Hostname: page-component-8448b6f56d-qsmjn Total loading time: 0 Render date: 2024-04-24T04:11:56.104Z Has data issue: false hasContentIssue false
  • English
  • Français

Degenerate p-Laplacian Operators and Hardy Type Inequalities on H-Type Groups

Published online by Cambridge University Press:  20 November 2018

Yongyang Jin  and
Genkai Zhang
Yongyang Jin*
Affiliation:
Department of Applied Mathematics, Zhejiang University of Technology, Hangzhou, 310032, China
Genkai Zhang*
Affiliation:
Mathematical Sciences, Chalmers University of Technology and Mathematical Sciences, Göteborg University, Göteborg, Sweden
*
yongyang@zjut.edu.cn
genkai@chalmers.se
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let 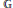$\mathbb{G}$ be a step-two nilpotent group of 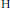$\text{H}$-type with Lie algebra 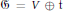$\mathfrak{G}\,=\,V\,\oplus \,\text{t}$. We define a class of vector fields $X\,=\,\left\{ {{X}_{j}} \right\}$ on 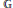$\mathbb{G}$ depending on a real parameter 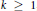$k\,\ge \,1$, and we consider the corresponding 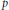$p$-Laplacian operator 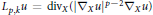${{L}_{p,\,k}}u\,=\,di{{v}_{X}}\left( {{\left| {{\nabla }_{X}}u \right|}^{p-2}}{{\nabla }_{X}}u \right)$. For 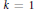$k\,=\,1$ the vector fields $X\,=\,\left\{ {{X}_{j}} \right\}$ are the left invariant vector fields corresponding to an orthonormal basis of $V$; for 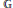$\mathbb{G}$ being the Heisenberg group the vector fields are the Greiner fields. In this paper we obtain the fundamental solution for the operator 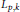${{L}_{p,\,k}}$ and as an application, we get a Hardy type inequality associated with 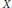$X$.

Keywords

35H3026D1022E25fundamental solutionsdegenerate LaplaciansHardy inequalityH-type groups
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 62 , Issue 5 , 01 October 2010 , pp. 1116 - 1130
Copyright
Copyright © Canadian Mathematical Society 2010

Footnotes

The first author’s research was supported by NNSF of China (10871180), NSF of Zhejiang province (Y6090359, Y6090383) and Department of Education, Zhejiang (Z200803357) The second author was supported by the Swedish Research Council and a STINT Institutional Grant.

References

[1] Beals, R., Gaveau, B., and Greiner, P., Uniforms hypoelliptic Green's functions. J. Math. Pures Appl. 77(1998), no. 3, 209–248.Google Scholar
[2] Capogna, L., Danielli, D., and Garofalo, N., Capacitary estimates and the local behavior of solutions of nonlinear subelliptic equations. Amer. J. Math. 118(1996), no. 6, 1153–1196. doi:10.1353/ajm.1996.0046Google Scholar
[3] D’Ambrozio, L., Some Hardy inequalities on the Heisenberg group. Differ. Uravn. 40(2004), no. 4, 509–521, 575.Google Scholar
[4] D’Ambrozio, L., Hardy-type inequalities related to degenerate elliptic differential operators. Ann. Sc. Norm. Super. Pisa Cl. Sci. 4(2005), no. 3, 451–486.Google Scholar
[5] Folland, G. B., A fundamental solution for a subelliptic operator. Bull. Amer. Math. Soc. 79(1973), 373–376. doi:10.1090/S0002-9904-1973-13171-4Google Scholar
[6] Folland, G. B., Subelliptic estimates and function spaces on nilpotent Lie groups. Ark. Mat. 13(1975), no. 2, 161–207. doi:10.1007/BF02386204Google Scholar
[7] Folland, G. B. and Stein, E. M., Hardy spaces on homogeneous groups. Mathematical Notes 28. Princeton University Press, Princeton, NJ, 1982.Google Scholar
[8] Garćıa Azorero, J. P. and Peral Alonso, I., Hardy inequalities and some critical elliptic and parabolic problems. J. Differential Equations 144(1998), no. 2, 441–476. doi:10.1006/jdeq.1997.3375Google Scholar
[9] Garofalo, N. and Lanconelli, E., Frequency functions on the Heisenberg group, the uncertainty principle and unique continuation. Ann. Inst. Fourier (Grenoble) 40(1990), no. 2, 313–356.Google Scholar
[10] Goldstein, J. A. and Kombe, I., Nonlinear degenerate parabolic equations on the Heisenberg group. Int. J. Evol. Equ. 1(2005), no. 1, 1–22.Google Scholar
[11] Goldstein, J. A. and Zhang, Q. S., On a degenerate heat equation with a singular potential. J. Funct. Anal. 186(2001), no. 2, 342–359. doi:10.1006/jfan.2001.3792Google Scholar
[12] Greiner, P. C., A fundamental solution for a nonelliptic partial differential operator. Canad. J. Math. 31(1979), no. 5, 1107–1120.Google Scholar
[13] Heinonen, J. and Holopainen, I., Quasiregular maps on Carnot groups. J. Geom. Anal. 7(1997), no. 1, 109–148.Google Scholar
[14] Hörmander, L., Hypoelliptic second order differential equations. Acta Math. 119(1967), 147–171. doi:10.1007/BF02392081Google Scholar
[15] Jin, Y., Hardy-type inequalities on H-type groups and anisotropic Heisenberg groups. Chin. Ann. Math. 29(2008), no. 5, 567–574.Google Scholar
[16] Kaplan, A., Fundamental solutions for a class of hypoelliptic PDE generated by composition of quadratic forms. Trans. Amer. Math. Soc. 258(1980), no. 1, 147–153. doi:10.2307/1998286Google Scholar
[17] Kohn, J. J., Hypoellipticity and loss of derivatives. Ann. of Math. 162(2005), no. 2, 943–986.With an appendix by Derridj, M. and Tartakoff, D. S.. doi:10.4007/annals.2005.162.943Google Scholar
[18] Nagel, A., Stein, E. M., and S.Wainger. Balls and metrics defined by vector fields. I. Basic properties. Acta Math. 155(1985), no. 1-2, 103–147. doi:10.1007/BF02392539Google Scholar
[19] Niu, P., Zhang, H., and Y.Wang, Hardy type and Rellich type inequalities on the Heisenberg group. Proc. Amer. Math. Soc. 129(2001), no. 12, 3623–3630. doi:10.1090/S0002-9939-01-06011-7Google Scholar
[20] Rothschild, L. P. and Stein, E. M., Hypoelliptic differential operators and nilpotent groups. Acta Math. 137(1976), no. 3-4,247–320. doi:10.1007/BF02392419Google Scholar
[21] Sánchez-Calle, A., Fundamental solutions and geometry of the sum of squares of vector fields. Invent. Math. 78(1984), no. 1, 143–160. doi:10.1007/BF01388721Google Scholar
[22] Stein, E. M., Harmonic analysis: real-variable methods, orthogonality, and oscillatory integrals. Princeton Mathematical Series 43. Princeton University Press, Princeton, NJ, 1993.Google Scholar
[23] Zhang, H. and Niu, P., Hardy-type inequalities and Pohozaev-type identities for a class of p-degenerate subelliptic operators and applications. Nonlinear Anal. 54(2003), 1, 165–186, 2003. doi:10.1016/S0362-546X(03)00062-2Google Scholar