Skip to main content
SpringerLink
Log in

Nonstandard Gauss—Lobatto quadrature approximation to fractional derivatives

  • Research Paper
  • Published:
Fractional Calculus and Applied Analysis Aims and scope Submit manuscript

Abstract

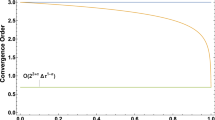
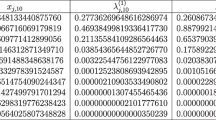
A family of nonstandard Gauss-Jacobi-Lobatto quadratures for numerical calculating integrals of the form ∫ 1-1 f′(x)(1-x)α dx, α > -1, is derived and applied to approximation of the usual fractional derivative. A software implementation of such quadratures was done by the recent Mathematica package OrthogonalPolynomials (cf. [A.S. Cvetković, G.V. Milovanović, Facta Univ. Ser. Math. Inform. 19 (2004), 17–36] and [G.V. Milovanović, A.S. Cvetković, Math. Balkanica 26 (2012), 169–184]). Several numerical examples are presented and they show the effectiveness of the proposed approach.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. G.E. Andrews, R. Askey, R. Roy, Special Functions. Encyclopedia of Mathematics and its Applications, 71, Cambridge University Press, Cambridge (1999).

    Book  MATH  Google Scholar 

  2. T.M. Atanacković, S. Pilipović, B. Stanković, D. Zorica, Fractional Calculus with Applications in Mechanics: Vibrations and Diffusion Processes. ISTE, London — Wiley, Hoboken (2014).

    Book  Google Scholar 

  3. D. Baleanu, K. Diethelm, E. Scalas, J. J. Trujillo, Fractional Calculus: Models and Numerical Methods. World Scientific, Singapore (2012).

    Google Scholar 

  4. H. Brass, K. Petras, Quadrature Theory: The Theory of Numerical Integration on a Compact Interval. American Mathematical Soc., Providence, RI. (2011).

    Book  Google Scholar 

  5. P.L. Butzer, U. Westphal, An introduction to fractional calculus, In: Applications of Fractional Calculus in Physics. World Sci. Publ., River Edge, NJ (2000), 1–85.

    Chapter  Google Scholar 

  6. M. Caputo, Linear models of dissipation whose Q is almost frequency independent — II. Geophysical Journal of the Royal Astronomical Society 13 (1967), 529–539.

    Article  Google Scholar 

  7. A.S. Cvetković, G.V. Milovanović, The Mathematica package ”OrthogonalPolynomials”. Facta Univ. Ser. Math. Inform. 9 (2014), 17–36.

    Google Scholar 

  8. K. Diethelm, The Analysis of Fractional Differential Equations. Springer-Verlag, Berlin, 2010.

    Book  MATH  Google Scholar 

  9. K. Diethelm, Error bounds for the numerical integration of functions with limited smoothness. SIAM J. Numer. Anal. 52, No 2 (2014), 877–879.

    Article  MATH  MathSciNet  Google Scholar 

  10. S. Esmaeili, M. Shamsi, M. Dehghan, Numerical solution of fractional differential equations via a Volterra integral equation approach. Centr. Eur. J. Phys. 11 (2013), 1470–1481.

    Article  Google Scholar 

  11. S. Esmaeili, M. Shamsi, Y. Luchko, Numerical solution of fractional differential equations with a collocation method based on Müntz polynomials. Comput. Math. Appl. 62 (2011), 918–929.

    Article  MATH  MathSciNet  Google Scholar 

  12. D. Funaro, Polynomial Approximation of Differential Equations. Springer-Verlag, Berlin (1992).

    MATH  Google Scholar 

  13. R. Garrappa, M. Popolizio, Evaluation of generalized Mittag-Leffler functions on the real line. Adv. Comput. Math. 39 (2013), 205–225.

    Article  MATH  MathSciNet  Google Scholar 

  14. W. Gautschi, Orthogonal Polynomials: Computation and Approximation. Oxford University Press, New York (2004).

    Google Scholar 

  15. G.H. Golub, J.H. Welsch, Calculation of Gauss quadrature rules. Math. Comp. 23 (1969), 221–230.

    Article  MATH  MathSciNet  Google Scholar 

  16. R. Gorenflo, J. Loutchko, Y. Luchko, Computation of the Mittag-Leffler function and its derivatives, Fract. Calc. Appl. Anal. 5 (2002), 491–518.

    MATH  MathSciNet  Google Scholar 

  17. R. Gorenflo, F. Mainardi, Fractional calculus: integral and differential equations of fractional order. In: Fractals and Fractional Calculus in Continuum Mechanics (Udine, 1996), CISMCourses and Lectures, 378, Springer, Vienna (1997), 223–276.

    Chapter  Google Scholar 

  18. N. Hale, A. Townsend, Fast and accurate computation of Gauss-Legendre and Gauss-Jacobi quadrature nodes and weights. SIAM J. Sci. Comput. 35 (2013), 652–674.

    Article  MathSciNet  Google Scholar 

  19. N. Hale, L.N. Trefethen, Chebfun and numerical quadrature. Sci. China Ser. A 55 (2012), 1749–1760.

    Article  MATH  MathSciNet  Google Scholar 

  20. T. Hasegawa, H. Sugiura, Uniform approximation to fractional derivatives of functions of algebraic singularity. J. Comput. Appl. Math. 228 (2009), 247–253.

    Article  MATH  MathSciNet  Google Scholar 

  21. R. Herrmann, Fractional Calculus: An Introduction for Physicists. World Scientific, Singapore (2011).

    Book  Google Scholar 

  22. A.A. Kilbas, H.M. Srivastava, J.J. Trujillo, Theory and Applications of Fractional Differential Equations. North-Holland Mathematics Studies, 204, Elsevier Science B.V., Amsterdam (2006).

    Book  MATH  Google Scholar 

  23. V. Kiryakova, Generalized Fractional Calculus and Applications. Pitman Research Notes in Math. Series, 301, Longman Scientific & Technical, Harlow; copubl. by John Wiley & Sons, Inc., New York (1994).

    MATH  Google Scholar 

  24. C. Li, F. Zheng, F. Liu, Spectral approximations to the fractional integral and derivative. Fract. Calc. Appl. Anal. 15 (2012), 383–406; DOI: 10.2478/s13540-012-0028-x; http://link.springer.com/article/10.2478/s13540-012-0028-x.

    Article  MATH  MathSciNet  Google Scholar 

  25. F. Mainardi, Fractional Calculus and Waves in Linear Viscoelasticity. An Introduction to Mathematical Models. Imperial College Press, London (2010).

    Book  MATH  Google Scholar 

  26. F. Mainardi, Fractional calculus: some basic problems in continuum and statistical mechanics. In: Fractals and Fractional Calculus in Continuum Mechanics (Udine, 1996), CISM Courses and Lectures, 378, Springer, Vienna (1997), 291–348.

    Chapter  Google Scholar 

  27. F. Mainardi, Y. Luchko, G. Pagnini, The fundamental solution of the space-time fractional diffusion equation. Fract. Calc. Appl. Anal. 4, No 2 (2001), 153–192.

    MATH  MathSciNet  Google Scholar 

  28. G. Mastroianni, G.V. Milovanović, Interpolation Processes: Basic Theory and Applications. Springer-Verlag, Berlin (2008).

    Book  Google Scholar 

  29. K.S. Miller, B. Ross, An Introduction to the Fractional Calculus and Fractional Differential Equations. A Wiley-Interscience Publication, John Wiley & Sons, Inc., New York (1993).

    MATH  Google Scholar 

  30. G.V. Milovanović, Müntz orthogonal polynomials and their numerical evaluation. In: Applications and Computation of Orthogonal Polynomials (W. Gautschi, G.H. Golub, and G. Opfer, Eds.), ISNM, Vol. 131, Birkhäuser, Basel (1999), 179–202.

    Chapter  Google Scholar 

  31. G.V. Milovanović, Chapter 23: Computer algorithms and software packages. In: Walter Gautschi: Selected Works and Commentaries, Volume 3 (C. Brezinski, A. Sameh, Eds.), Birkhäuser, Basel (2014), 9–10.

    Chapter  Google Scholar 

  32. G.V. Milovanović, A.S. Cvetković, Gaussian type quadrature rules for Müntz systems. SIAM J. Sci. Comput. 27 (2005), 893–913.

    Article  MATH  MathSciNet  Google Scholar 

  33. G.V. Milovanović, A.S. Cvetković, Nonstandard Gaussian quadrature formulae based on operator values. Adv. Comput. Math. 32 (2010), 431–486.

    Article  MATH  MathSciNet  Google Scholar 

  34. G.V. Milovanović, A.S. Cvetković, Special classes of orthogonal polynomials and corresponding quadratures of Gaussian type. Math. Balkanica 26, No 1–2 (2012), 169–184.

    MATH  MathSciNet  Google Scholar 

  35. P. Novati, Numerical approximation to the fractional derivative operator. Numer. Math. 127 (2014), 539–566.

    Article  MATH  MathSciNet  Google Scholar 

  36. I. Podlubny, Fractional Differential Equations. Academic Press, San Diego, CA (1999).

    MATH  Google Scholar 

  37. I. Podlubny, M. Kacenak, The Matlab mlf code. MATLAB Central File Exchange (2001–2012), File ID: 8738.

    Google Scholar 

  38. S.G. Samko, A.A. Kilbas, O.I. Marichev, Fractional Integrals and Derivatives. Theory and Applications. Edited and with a foreword by S.M. Nikol’skiĭ, Transl. from the 1987 Russian original, Revised by the authors. Gordon and Breach Science Publishers, Yverdon (1993).

  39. H. Sugiura, T. Hasegawa, Quadrature rule for Abel’s equations: uniformly approximating fractional derivatives. J. Comput. Appl. Math. 223 (2009), 459–468.

    Article  MATH  MathSciNet  Google Scholar 

  40. L.N. Trefethen, Approximation Theory and Approximation Practice, SIAM, Philadelphia, PA (2013).

    MATH  Google Scholar 

  41. D. Valério, J.J. Trujillo, M. Rivero, J.A.T. Machado, D. Baleanu, Fractional calculus: A survey of useful formulas. Eur. Phys. J. Special Topics 222 (2013), 1827–1846.

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Applied Mathematics, University of Kurdistan, P.O. Box 416, Sanandaj, Iran

    Shahrokh Esmaeili

  2. Mathematical Institute, Serbian Academy of Sciences and Arts, Kneza Mihaila 36, 11001, Belgrade, Serbia

    Gradimir V. Milovanović

Authors
  1. Shahrokh Esmaeili
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Gradimir V. Milovanović
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Shahrokh Esmaeili.

Additional information

Dedicated to Professor Ivan Dimovski on the occasion of his 80th anniversary

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Esmaeili, S., Milovanović, G.V. Nonstandard Gauss—Lobatto quadrature approximation to fractional derivatives. Fract Calc Appl Anal 17, 1075–1099 (2014). https://doi.org/10.2478/s13540-014-0215-z

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.2478/s13540-014-0215-z

MSC 2010

Key Words and Phrases

Search

Navigation