Abstract
The anomalous Hall effect in the noncollinear antiferromagnetic metals Mn3Ge and Mn3Sn has been observed after a theoretical prediction made by us (Kübler J. and Felser C., EPL, 108 (2014) 67001). The experimental values of the anomalous Hall conductivities (AHC) are large as are the theoretical values. Recently measured thermoelectric properties mirror the large AHC and clearly show that the transport is by quasiparticles at the Fermi energy. We here make an attempt to unravel the origin of the large AHC and show that both Mn3Sn and Mn3Ge host Weyl points, which were recently discovered in semimetals. For this purpose we determine the electronic structure ab initio in the local spin-density functional approximation. The Weyl points are found to occur below the Fermi energy and we argue that spots of large Berry flux ("hot spots") that are seen at the Fermi surface are produced by the Weyl nodes.
Export citation and abstract BibTeX RIS
Introduction
Recently we predicted the occurrence of an anomalous Hall effect (AHE) in the antiferromagnetic compounds Mn3Sn and Mn3Ge [1] and, indeed, this effect was subsequently discovered in Mn3Sn by Nakatsuji et al. [2] as well as in Mn3Ge by Nayak et al. [3] and independently by Kiyohara and Nakatsuji [4].
While the AHE commonly occurs in ferromagnets [5], it is expected to be absent in antiferromagnets, but Chen et al. [6] found that under certain symmetry conditions it is observable in non-collinear antiferromagnetic compounds. Extending the class of compounds possessing an AHE is of fundamental interest, but it might also be of importance for spintronics applications [7].
The compounds Mn3Sn and Mn3Ge can be grown in the hexagonal crystal structure having the symmetry  and a non-symmorphic spüace group. They have been shown to possess a variety of non-collinear antiferromagnetic orders [8–12], of which one kind is sketched in fig. 1(a), another one in fig. 6(a). Of all the possibilities these two cases have inverse chirality [11].
and a non-symmorphic spüace group. They have been shown to possess a variety of non-collinear antiferromagnetic orders [8–12], of which one kind is sketched in fig. 1(a), another one in fig. 6(a). Of all the possibilities these two cases have inverse chirality [11].
Fig. 1: (Colour online) The case of Mn3Sn. (a) Top view of the crystal structure indicating the directions of the magnetic moments by arrows, blue in the z = 0 plane and red in the 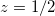 plane. The unit cell is enclosed by heavy solid lines, the corners of which are defined by the Sn sites in the z = 0 plane. (b) Hexagonal Brillouin zone.
plane. The unit cell is enclosed by heavy solid lines, the corners of which are defined by the Sn sites in the z = 0 plane. (b) Hexagonal Brillouin zone.
Download figure:
Standard imageWe present results of improved calculations of the anomalous Hall conductivity (AHC), changing the previously used outdated hexagonal coordinate system [1], so that we are now conform with ref. [3] and with the International Tables of Crystallography. Special attention is given to the electronic structure at general points in the Brillouin zone, in order to understand the physical origin of the large AHC. Here we show that topological objects, called Weyl points, might supply the answer. These points are singularities in momentum space, originating from band-crossings. They are understood as magnetic monopoles that are sources of Berry curvature. They come in pairs and give rise to characteristic surface properties, the so-called Fermi arcs, which have been predicted theoretically and verified experimentally [13–17]. The ensuing non-trivial topology has been studied in semimetals, but recently the topological approach has been extended to α-iron [18] and to the ferromagnetic Heusler compound Co2MnAl [19], which possesses a truly gigantic AHC. Recently, Mn3Sn and Mn3Ge were also seen to host Weyl points that were all found to be below the Fermi energy [20]. However, the electrical transport properties and especially the thermoelectric properties studied recently [21,22] are quasiparticle properties that reside at the Fermi surface as stressed by Haldane [23]. The purpose of this letter is to show under which conditions the effect of low-lying Weyl points might appear at the Fermi surface. Reference [20] contains a thorough study of the surface arcs of Mn3Sn and Mn3Ge but no attempt was made to relate the Weyl nodes to the bulk Fermi surface. Instead of using Haldane's mathematical theory [23], we here present descriptive arguments to show that the Weyl nodes cause the large anomalous Hall conductivity and the distinct thermoelectric effects through the non-zero Chern number of the bands at the Fermi surface. When we say large we have in mind values comparable to those of ferromagnetic α-iron, i.e., of order of 700S/cm, which is almost achieved in Mn3Ge.
The anomalous Hall effect is obtained by computing the Berry curvature (BC) in momentum space. The quantities responsible for the thermoelectric effects are related to the BC as well [23]. This is a vector, writing its p-component as 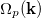 , and is obtained from the curl of the Berry connection, given by
, and is obtained from the curl of the Berry connection, given by 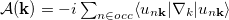 , where
, where 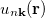 is the crystal-periodic eigenfunction having wave vector k and band index n,
is the crystal-periodic eigenfunction having wave vector k and band index n, 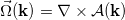 . The sum extends over the occupied states. For its evaluation we use the wave functions from density functional calculations [24] following ref. [25], where the numerical work is based on
. The sum extends over the occupied states. For its evaluation we use the wave functions from density functional calculations [24] following ref. [25], where the numerical work is based on ![$\Im \ln\det[\langle u_{n \bf k}|u_{m {\textbf{k}^{\prime}}}\rangle]$](https://content.cld.iop.org/journals/0295-5075/120/4/47002/revision1/epl18925ieqn7.gif) , which is directly related to the Berry connection [26]. Spin-orbit coupling (SOC) is treated in the second variation and is an essential ingredient. The anomalous Hall conductivity (AHC) follows from the BC by means of
, which is directly related to the Berry connection [26]. Spin-orbit coupling (SOC) is treated in the second variation and is an essential ingredient. The anomalous Hall conductivity (AHC) follows from the BC by means of

where 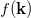 is the Fermi distribution function, the integral extends over the Brillouin zone,
is the Fermi distribution function, the integral extends over the Brillouin zone, 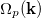 is the p-component of the BC for the wave vector k and the components
is the p-component of the BC for the wave vector k and the components 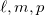 (for x, y,z) are to be chosen cyclic [27]. While this equation is commonly used for the computation of the AHC, it does not reveal that the transport takes place at the Fermi surface. Haldane [23], as said before, derived another version of eq. (1) that does just that, but will not be used here. We also need the definition of the Chern number [23], which is given by
(for x, y,z) are to be chosen cyclic [27]. While this equation is commonly used for the computation of the AHC, it does not reveal that the transport takes place at the Fermi surface. Haldane [23], as said before, derived another version of eq. (1) that does just that, but will not be used here. We also need the definition of the Chern number [23], which is given by

The extra index n appearing here is the state number, which appears in the BC if the sum over states is omitted. We state that the BZ-integral devided by 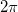 gives the Chern number of band n, Cn, times
gives the Chern number of band n, Cn, times 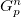 , the p-component of a reciprocal lattice vector. The topology of a crystal is non-trivial if the integer
, the p-component of a reciprocal lattice vector. The topology of a crystal is non-trivial if the integer 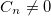 .
.
Mn3Sn
We begin with Mn3Sn and show the band structure along symmetry lines in fig. 2. It is in good agreement with the band structure shown in ref. [28], where the symmetry conditions are given for the anomalous Hall conductivity and the spin Hall conductivity. The integrated Berry curvature vector for the order shown in fig. 1(a) is in the x-direction and the calculated AHC is 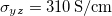 . The experimental value is obtained at 100 K and is
. The experimental value is obtained at 100 K and is 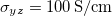 [2]. The value calculated in ref. [28] is
[2]. The value calculated in ref. [28] is 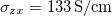 which is obtained for the magnetic order sketched in fig. 6(a). The difference is not due to the other magnetic order, but is accounted for by our numerical method. The BC together with contours of the Fermi surface are displayed in fig. 3(a) in the
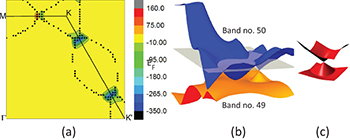
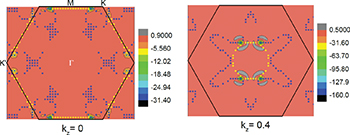
which is obtained for the magnetic order sketched in fig. 6(a). The difference is not due to the other magnetic order, but is accounted for by our numerical method. The BC together with contours of the Fermi surface are displayed in fig. 3(a) in the 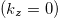 -plane. The BC is very large where the green spots appear. To appreciate the size of the local BC one should multiply the values given in the figure by about 1100 to convert to units S/cm of the Hall conductivity. The dominating values appear at locations of the Fermi surface where the curvature of the Fermi contours shown by the blue dots is large. Figure 3(b) is a two-dimensional rendering of the band structure and band No. 50 is seen to cut the Fermi energy (gray) thus defining the Fermi contours. The lower band No. 49 touches the higher band in two points in the Brillouin zone. Zooming in at one of the points we see the Weyl point that is graphed in part (c) of the figure. The band crossing is at the point K in the Brillouin zone where no signal of the Berry curvature is seen in fig. 3(a). Another Weyl point seen in fig. 3(b) is located at
-plane. The BC is very large where the green spots appear. To appreciate the size of the local BC one should multiply the values given in the figure by about 1100 to convert to units S/cm of the Hall conductivity. The dominating values appear at locations of the Fermi surface where the curvature of the Fermi contours shown by the blue dots is large. Figure 3(b) is a two-dimensional rendering of the band structure and band No. 50 is seen to cut the Fermi energy (gray) thus defining the Fermi contours. The lower band No. 49 touches the higher band in two points in the Brillouin zone. Zooming in at one of the points we see the Weyl point that is graphed in part (c) of the figure. The band crossing is at the point K in the Brillouin zone where no signal of the Berry curvature is seen in fig. 3(a). Another Weyl point seen in fig. 3(b) is located at 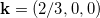 . There are all together 6 Weyl points of chirality
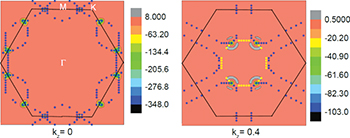
. There are all together 6 Weyl points of chirality 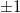 in the extended zone, which is displayed in fig. 4. We did not try to verify more points described in ref. [20] because they are too far below the Fermi energy. In fig. 4 we look at the Fermi contours in the extended zone to visualize the development of the BC in the
in the extended zone, which is displayed in fig. 4. We did not try to verify more points described in ref. [20] because they are too far below the Fermi energy. In fig. 4 we look at the Fermi contours in the extended zone to visualize the development of the BC in the 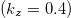 -plane from the BC in the
-plane from the BC in the 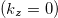 -plane. In the
-plane. In the 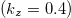 -plane a second contour appears (yellow dots) due to band No. 51. The contours due to band No. 50 (blue dots) deform and carry the BC spots at the location of large curvature of the Fermi contours. In fig. 5(a) the Berry curvature together with the Fermi contours is displayed in the
-plane a second contour appears (yellow dots) due to band No. 51. The contours due to band No. 50 (blue dots) deform and carry the BC spots at the location of large curvature of the Fermi contours. In fig. 5(a) the Berry curvature together with the Fermi contours is displayed in the 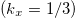 -plane which is sketched schematically in part (b) of the figure. The strong signal at the Fermi energy is a reflection of the Weyl point at K. The Fermi contour that shows up in the upper part of fig. 5(a) is due to band No. 51. There is no BC emanating from this contour.
-plane which is sketched schematically in part (b) of the figure. The strong signal at the Fermi energy is a reflection of the Weyl point at K. The Fermi contour that shows up in the upper part of fig. 5(a) is due to band No. 51. There is no BC emanating from this contour.
Fig. 2: The band structure of Mn3Sn plotted along symmetry lines. The labels are defined in fig. 1(b). The Fermi energy is at 0 eV.
Download figure:
Standard imageFig. 3: (Colour online) Mn3Sn: (a) Fermi surface contours in the  -plane from band No. 50 and the Berry curvature
-plane from band No. 50 and the Berry curvature 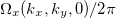 , special points of the Brillouin zone are marked. The dark-green contours are due to large values of the Berry curvature. (b) Bands Nos. 49 and 50 near the Fermi energy (gray) in a two-dimensional plot. (c) Enlarged Weyl point at the point K.
, special points of the Brillouin zone are marked. The dark-green contours are due to large values of the Berry curvature. (b) Bands Nos. 49 and 50 near the Fermi energy (gray) in a two-dimensional plot. (c) Enlarged Weyl point at the point K.
Download figure:
Standard imageFig. 4: (Colour online) Mn3Sn: View of the Fermi surface contours and the Berry curvatures in the extended zone for two planes; as fig. 3(a) 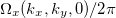 in the
in the 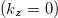 -plane and
-plane and 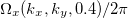 in the
in the 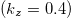 -plane. In the latter band No. 51 leads to the Fermi contours plotted in yellow.
-plane. In the latter band No. 51 leads to the Fermi contours plotted in yellow.
Download figure:
Standard imageFig. 5: (Colour online) Mn3Sn: (a) Fermi surface contours and the Berry curvature 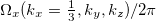 in the
in the 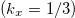 -plane. The green contours are due to large values of the Berry curvature. The circular contour graphed with green dots originates from band No. 51, black dots from band No. 50. (b) Schematic plot of the Brillouin zone showing the plane (yellow) used in (a).
-plane. The green contours are due to large values of the Berry curvature. The circular contour graphed with green dots originates from band No. 51, black dots from band No. 50. (b) Schematic plot of the Brillouin zone showing the plane (yellow) used in (a).
Download figure:
Standard imageFig. 6: (Colour online) The case of Mn3Ge. (a) Top view of the crystal structure and the directions of the magnetic moments as in fig. 1. (b) Band structure of Mn3Ge along symmetry lines.
Download figure:
Standard imageMn3Ge
We continue with Mn3Ge. The magnetic structure, shown in fig. 6(a), is different from that of Mn3Sn. One is obtained from the other by rotating all spins by 90° about the z-axis. Computationally the total energies of the two structures in either one of the systems are very close so that we rely on experimental information for the present assignment. The integrated Berry curvature vector in this case is in the y-direction and the calculated AHC amounts to 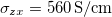 . This is to be compared with low-temperature values of
. This is to be compared with low-temperature values of 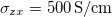 measured by both groups [3,4]. The calculated value given in ref. [28] is
measured by both groups [3,4]. The calculated value given in ref. [28] is 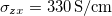 . The band structure along symmetry lines is given in fig. 6(b) and is in good agreement with ref. [28]. It is nearly identical to fig. 2, except for minute differences near the Fermi energy at K and M. These matter as can be seen in fig. 7(a), where Fermi contours are displayed together with the BC in the
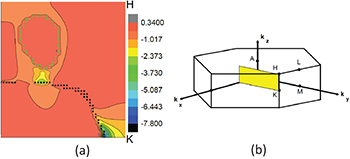
. The band structure along symmetry lines is given in fig. 6(b) and is in good agreement with ref. [28]. It is nearly identical to fig. 2, except for minute differences near the Fermi energy at K and M. These matter as can be seen in fig. 7(a), where Fermi contours are displayed together with the BC in the 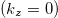 -plane. Now the Fermi surface is formed by two bands, viz. bands Nos. 70 and 71. A spot of large value of the BC appears as green and black contours near a Fermi contour that curve strongly below the line
-plane. Now the Fermi surface is formed by two bands, viz. bands Nos. 70 and 71. A spot of large value of the BC appears as green and black contours near a Fermi contour that curve strongly below the line 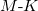 . Figure 7(b) gives the band structure near the Fermi energy in a two-dimensional plot. In fig. 8(a) we locate a band crossing between band Nos. 69 and 70. Zooming in near K we see the Weyl point shown in fig. 8(b). Another Weyl point appears at
. Figure 7(b) gives the band structure near the Fermi energy in a two-dimensional plot. In fig. 8(a) we locate a band crossing between band Nos. 69 and 70. Zooming in near K we see the Weyl point shown in fig. 8(b). Another Weyl point appears at 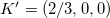 . As for Mn3Sn there are 6 Weyl points of chirality
. As for Mn3Sn there are 6 Weyl points of chirality 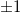 in the extended zone. The other Weyl points described in ref. [20] we ignored since they are too far below the Fermi energy. In fig. 9 as before we look at the Fermi contours in the extended zone to visualize the development of the BC in the
in the extended zone. The other Weyl points described in ref. [20] we ignored since they are too far below the Fermi energy. In fig. 9 as before we look at the Fermi contours in the extended zone to visualize the development of the BC in the 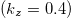 -plane from the BC in the
-plane from the BC in the 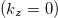 -plane. The contours due to band No. 70 (blue dots) deform and carry the BC spots at the location of large curvature of the Fermi contours, whereas the contours due to band No. 71 contract to the yellow circle. Figure 10(a), finally, shows Fermi contours together with the BC in the
-plane. The contours due to band No. 70 (blue dots) deform and carry the BC spots at the location of large curvature of the Fermi contours, whereas the contours due to band No. 71 contract to the yellow circle. Figure 10(a), finally, shows Fermi contours together with the BC in the 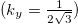 -plane, which is shown in part (b) of the figure. The green BC spot in (a) is a reflection of the Weyl points at K and
-plane, which is shown in part (b) of the figure. The green BC spot in (a) is a reflection of the Weyl points at K and 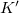 . The yellow Fermi surface contours near
. The yellow Fermi surface contours near 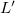 show no sizeable BC spots.
show no sizeable BC spots.
Fig. 7: (Colour online) Mn3Ge: (a) Fermi surface contours from band No. 70 (blue dots) and band No. 71 (yellow dots) and the berry curvature 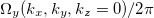 in the
in the 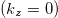 -plane. Symmetry points of the Brillouin zone are marked. The large value of the Berry curvature shows up as a green and black contour. (b) Bands Nos. 69 to 71 as well as the Fermi energy (yellow) in a two-dimensional graph.
-plane. Symmetry points of the Brillouin zone are marked. The large value of the Berry curvature shows up as a green and black contour. (b) Bands Nos. 69 to 71 as well as the Fermi energy (yellow) in a two-dimensional graph.
Download figure:
Standard imageFig. 8: (Colour online) Mn3Ge: (a) Two-dimensional bands from band No. 69 to 71 (blue) and the Fermi energy (grey). (b) The Weyl point at the K point.
Download figure:
Standard imageFig. 9: (Colour online) Mn3Ge: view of the Fermi surface contours and the Berry curvatures in the extended zone for two planes; as fig. 7(a) 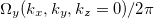 in the
in the 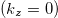 -plane and
-plane and 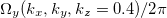 in the
in the 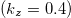 -plane. Band No. 71 leads to the Fermi contours plotted in yellow.
-plane. Band No. 71 leads to the Fermi contours plotted in yellow.
Download figure:
Standard imageFig. 10: (Colour online) Mn3Ge: (a) Fermi surface contours from band No. 70 (blue dots) and 71 (yellow dots) and the Berry curvature 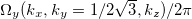 in the
in the 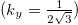 -plane. Large values of the Berry curvature show up as green contours. (b) Schematic plot of the Brillouin zone showing the surface (yellow) of the plane used in (a).
-plane. Large values of the Berry curvature show up as green contours. (b) Schematic plot of the Brillouin zone showing the surface (yellow) of the plane used in (a).
Download figure:
Standard imageDiscussion
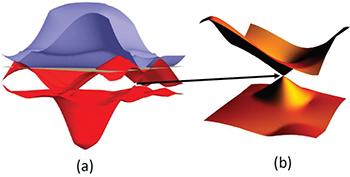
We now try to explain the spots of large BC, for short "hot spots", with the physical concepts developed for Weyl semimetals [15]. It is not a priori clear if these concepts also apply to non-collinear antiferromagnetic metals, but we assume they do. There is first the fact that the Chern number C of a band is non-zero between two Weyl points of opposite chirality. Thus, the band No. 50 for Mn3Sn (see fig. 3) and band No. 70 for Mn3Ge (see figs. 7 and 8) at the Fermi energy have  . That is the hot spots are a property of the non-zero Chern number. The next higher band, No. 51 for Mn3Sn and No. 71 for Mn3Ge, do produce uniform BC but no spots. A simple formula valid for a model Weyl semimetal relates the AHC to the difference vector between two Weyl points of opposite chirality [15]. Using the Weyl points obtained here, we obtain about
. That is the hot spots are a property of the non-zero Chern number. The next higher band, No. 51 for Mn3Sn and No. 71 for Mn3Ge, do produce uniform BC but no spots. A simple formula valid for a model Weyl semimetal relates the AHC to the difference vector between two Weyl points of opposite chirality [15]. Using the Weyl points obtained here, we obtain about 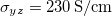 for Mn3Sn and
for Mn3Sn and 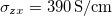 for Mn3Ge. It must be remarked that these values are fortuitously close to the observed ones, since none of the assumptions underlying their derivation are valid here, except for the Chern number of the bands. But the range of validity may be larger than assumed. This opens another question: Could it be that the chiral anomaly exists for non-collinear antiferromagnets? Presumably this question can only be answered by experiments. Concerning the thermoelectric effects it is to be seen whether they are as large in Mn3Ge as in Mn3Sn.
for Mn3Ge. It must be remarked that these values are fortuitously close to the observed ones, since none of the assumptions underlying their derivation are valid here, except for the Chern number of the bands. But the range of validity may be larger than assumed. This opens another question: Could it be that the chiral anomaly exists for non-collinear antiferromagnets? Presumably this question can only be answered by experiments. Concerning the thermoelectric effects it is to be seen whether they are as large in Mn3Ge as in Mn3Sn.