Normalized solutions to p-Laplacian equations with combined nonlinearities^*

In this paper, we study the p-Laplacian equation with a L^p-norm constraint: 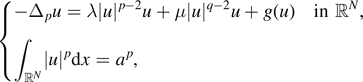$\begin{cases}-{{\Delta}}_{p}u=\lambda \vert u{\vert }^{p-2}u+\mu \vert u{\vert }^{q-2}u+g(u)\quad \text{in}\ {\mathbb{R}}^{N},\quad \hfill \\ {\int }_{{\mathbb{R}}^{N}}\vert u{\vert }^{p}\mathrm{d}x={a}^{p},\quad \hfill \end{cases}$ where N ⩾ 2, a > 0, 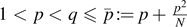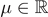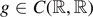$1< p< q\leqslant \bar{p}{:=}p+\frac{{p}^{2}}{N}$, $\mu \in \mathbb{R}$, $g\in C(\mathbb{R},\mathbb{R})$ and $\lambda \in \mathbb{R}$ 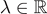is a Lagrange multiplier, which appears due to the mass constraint ||u||_p = a. We assume that g is odd and L^p-supercritical. When 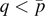$q< \bar{p}$ and μ > 0, we use Schwarz rearrangement and Ekeland variational principle to prove the existence of positive radial ground states for suitable μ. When 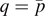$q=\bar{p}$ and μ > 0 or 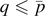$q\leqslant \bar{p}$ and μ ⩽ 0, with an additional condition of g, we obtain a positive radial ground state if μ lies in a suitable range, by the Schwarz rearrangement and minimax theorems. Via a fountain theorem type argument, with suitable $\mu \in \mathbb{R}$, we obtain infinitely many radial solutions for any N ⩾ 2 and establish the existence of infinitely many nonradial sign-changing solutions for N = 4 or N ⩾ 6. In any case mentioned above, the range of μ depends on the value of a: |μ| can be large if a > 0 is small.