Gevrey separation of fast and slow variables

We consider (not necessarily conservative) perturbations of a one phase Hamiltonian system written with action-angle variables

where is real analytic, of the form

where f, g are real analytic in all the variables and -periodic in . More generally we consider systems similar to system (*) with 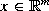. It is well known that, using an iterated averaging process, it is posssible to eliminate `formally in ' the phase by a formal transformation tangent to the identity

where are -periodic in .

Then one obtains a formal autonomous system

Fixing the normalization , we prove that the transform is Gevrey 1 in . As an application, we give a new proof of a result of Neishstadt: it is possible to represent the formal transformation by an actual transformation T (admitting as its asymptotic expansion) such that the transformation T reduces the system (*) to a system which is autonomous up to perturbations which are exponentially small in . It is possible to use a cut-off `at the smallest term' like Neishstadt but we prefer to use an incomplete Laplace transform. Then we obtain for T a nice dependence in and we improve Neishstadt's result. We will also give similar improvements for the basic adiabatic invariants theory.

Our main statement generalizes a theorem of D Sauzin conjectured by P Lochak.