Hostname: page-component-8448b6f56d-dnltx Total loading time: 0 Render date: 2024-04-23T17:24:26.328Z Has data issue: false hasContentIssue false
  • English
  • Français

Diapycnal diffusivity, turbulent Prandtl number and mixing efficiency in Boussinesq stratified turbulence

Published online by Cambridge University Press:  26 June 2015

Hesam Salehipour Open the ORCID record for Hesam Salehipour [Opens in a new window]  and
W. R. Peltier
Hesam Salehipour*
Affiliation:
Department of Physics, University of Toronto, Toronto, ON, M5S 1A7, Canada
W. R. Peltier
Affiliation:
Department of Physics, University of Toronto, Toronto, ON, M5S 1A7, Canada
*
†Email address for correspondence: h.salehipour@utoronto.ca
Get access Rights & Permissions [Opens in a new window]

Abstract

In order that it be correctly characterized, irreversible turbulent mixing in stratified fluids must distinguish between adiabatic ‘stirring’ and diabatic ‘mixing’. Such a distinction has been formalized through the definition of a diapycnal diffusivity, 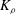$K_{{\it\rho}}$ (Winters & D’Asaro, J. Fluid Mech., vol. 317, 1996, pp. 179–193) and an appropriate mixing efficiency, $\mathscr{E}$ (Caulfield & Peltier, J. Fluid Mech., vol. 413, 2000, pp. 1–47). Equivalent attention has not been paid to the definitions of a corresponding momentum diffusivity 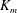$K_{m}$ and hence an appropriately defined turbulent Prandtl number 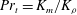$\mathit{Pr}_{t}=K_{m}/K_{{\it\rho}}$. In this paper, the diascalar framework of Winters & D’Asaro (1996) is first reformulated to obtain an ‘Osborn-like’ formula in which the correct definition of irreversible mixing efficiency $\mathscr{E}$ is shown to replace the flux Richardson number which Osborn (J. Phys. Oceanogr., vol. 10, 1980, pp. 83–89) assumed to characterize this efficiency. We advocate the use of this revised representation for diapycnal diffusivity since the proposed reformulation effectively removes the simplifying assumptions on which the original Osborn formula was based. We similarly propose correspondingly reasonable definitions for $K_{m}$ and 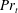$\mathit{Pr}_{t}$ by eliminating the reversible component of the momentum production term. To explore implications of the reformulations for both diapycnal and momentum diffusivity we employ an extensive series of direct numerical simulations (DNS) to investigate the properties of the shear-induced density-stratified turbulence that is engendered through the breaking of a freely evolving Kelvin–Helmholtz wave. The DNS results based on the proposed reformulation of 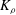$K_{{\it\rho}}$ are compared with available estimations due to the mixing length model, as well as both the Osborn–Cox and the Osborn models. Estimates based upon the Osborn–Cox formulation are shown to provide the closest approximation to the diapycnal diffusivity delivered by the exact representation. Through compilation of the complete set of DNS results we explore the characteristic dependence of $K_{{\it\rho}}$ on the buoyancy Reynolds number 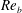$\mathit{Re}_{b}$ as originally investigated by Shih et al. (J. Fluid Mech., vol. 525, 2005, pp. 193–214) in their idealized study of homogeneous stratified and sheared turbulence, and show that the validity of their results is only further reinforced through analysis of the turbulence produced in the more geophysically relevant Kelvin–Helmholtz wave life-cycle ansatz. In contrast to the results described by Shih et al. (2005) however, we show that, besides 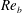$\mathit{Re}_{b}$, a vertically averaged measure of the gradient Richardson number 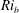$\mathit{Ri}_{b}$ may equivalently characterize the turbulent mixing at high 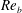$\mathit{Re}_{b}$. Based on the dominant driving processes involved in irreversible mixing, we categorize the intermediate (i.e. $\mathit{Re}_{b}=O(10^{1}{-}10^{2})$) and high (i.e. 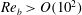$\mathit{Re}_{b}>O(10^{2})$) range of 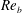$\mathit{Re}_{b}$ as ‘buoyancy-dominated’ and ‘shear-dominated’ mixing regimes, which together define a transition value of 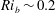$\mathit{Ri}_{b}\sim 0.2$. Mixing efficiency varies non-monotonically with both 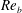$\mathit{Re}_{b}$ and 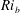$\mathit{Ri}_{b}$, with its maximum (on the order of 0.2–0.3) occurring in the ‘buoyancy-dominated’ regime. Unlike 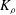$K_{{\it\rho}}$ which is very sensitive to the correct choice of $\mathscr{E}$ (i.e. 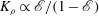$K_{{\it\rho}}\propto \mathscr{E}/(1-\mathscr{E})$), we show that $K_{m}$ is almost insensitive to the choice of $\mathscr{E}$ (i.e. 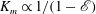$K_{m}\propto 1/(1-\mathscr{E})$) so long as 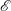$\mathscr{E}$ is not close to unity, which implies 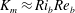$K_{m}\approx \mathit{Ri}_{b}\mathit{Re}_{b}$ for the entire range of $\mathit{Re}_{b}$. The turbulent Prandtl number is consequently shown to decrease monotonically with 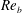$\mathit{Re}_{b}$ and may be (to first order) simply approximated by 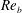$\mathit{Re}_{b}$ itself. 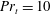Assuming $\mathit{Pr}_{t}=1$, or $\mathit{Pr}_{t}=10$ (as is common in large-scale numerical models of the ocean general circulation), is also suggested to be a questionable assumption.

JFM classification

Geophysical and Geological Flows: Ocean circulation Turbulent Flows: Stratified turbulence
Type
Papers
Information
Journal of Fluid Mechanics , Volume 775 , 25 July 2015 , pp. 464 - 500
Check for updates
Copyright
© 2015 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Barry, M. E., Ivey, G. N., Winters, K. B. & Imberger, J. 2001 Measurements of diapycnal diffusivities in stratified fluids. J. Fluid Mech. 442, 267–291.Google Scholar
Batchelor, G. K. 1953 The Theory of Homogeneous Turbulence. Cambridge University Press.Google Scholar
Bluteau, C. E., Jones, N. L. & Ivey, G. N. 2013 Turbulent mixing efficiency at an energetic ocean site. J. Geophys. Res. 118 (9), 4662–4672.Google Scholar
Bouffard, D. & Boegman, L. 2013 A diapycnal diffusivity model for stratified environmental flows. Dyn. Atmos. Oceans 61, 14–34.Google Scholar
Brethouwer, G., Billant, P., Lindborg, E. & Chomaz, J.-M. 2007 Scaling analysis and simulation of strongly stratified turbulent flows. J. Fluid Mech. 585, 343–368.CrossRefGoogle Scholar
Caulfield, C. P. & Peltier, W. R. 2000 The anatomy of the mixing transition in homogeneous and stratified free shear layers. J. Fluid Mech. 413, 1–47.CrossRefGoogle Scholar
Crawford, W. R. 1982 Pacific equatorial turbulence. J. Phys. Oceanogr. 12 (10), 1137–1149.Google Scholar
Danabasoglu, G., Bates, S. C., Briegleb, B. P., Jayne, S. R., Jochum, M., Large, W. G., Peacock, S. & Yeager, S. G. 2012 The CCSM4 ocean component. J. Clim. 25 (5), 1361–1389.Google Scholar
Davis, R. E. 1994 Diapycnal mixing in the ocean: the Osborn–Cox model. J. Phys. Oceanogr. 24 (12), 2560–2576.Google Scholar
Dunckley, J. F., Koseff, J. R., Steinbuck, J. V., Monismith, S. G. & Genin, A. 2012 Comparison of mixing efficiency and vertical diffusivity models from temperature microstructure. J. Geophys. Res. 117, C10008.Google Scholar
Ellison, T. H. 1957 Turbulent transport of heat and momentum from an infinite rough plane. J. Fluid Mech. 2 (5), 456–466.Google Scholar
Fernando, H. J. S. 1991 Turbulent mixing in stratified fluids. Annu. Rev. Fluid Mech. 23, 455–493.Google Scholar
Fischer, P. F. 1997 An overlapping schwarz method for spectral element solution of the incompressible Navier–Stokes equations. J. Comput. Phys. 133 (1), 84–101.Google Scholar
Fischer, P. F., Kruse, G. W. & Loth, F. 2002 Spectral element methods for transitional flows in complex geometries. J. Sci. Comput. 17 (1–4), 81–98.CrossRefGoogle Scholar
Fischer, P. F., Lottes, J. W. & Kerkemeier, S. G.2008 nek5000 Web page. http://nek5000.mcs.anl.gov.Google Scholar
Fleury, M. & Lueck, R. G. 1994 Direct heat flux estimates using a towed vehicle. J. Phys. Oceanogr. 24 (4), 801–818.Google Scholar
Gerz, T., Schumann, U. & Elghobashi, S. E. 1989 Direct numerical simulation of stratified homogeneous turbulent shear flows. J. Fluid Mech. 200, 563–594.Google Scholar
Gregg, M. C. 1987 Diapycnal mixing in the thermocline: a review. J. Geophys. Res. 92 (C5), 5249–5286.Google Scholar
Holt, S. E., Koseff, J. R. & Ferziger, J. H. 1992 A numerical study of the evolution and structure of homogeneous stably stratified sheared turbulence. J. Fluid Mech. 237, 499–539.CrossRefGoogle Scholar
Ivey, G. N. & Imberger, J. 1991 On the nature of turbulence in a stratified fluid. Part I: the energetics of mixing. J. Phys. Oceanogr. 21 (5), 650–658.Google Scholar
Ivey, G. N., Winters, K. B. & De Silva, I. P. D. 2000 Turbulent mixing in a sloping benthic boundary layer energized by internal waves. J. Fluid Mech. 418, 59–76.CrossRefGoogle Scholar
Ivey, G. N., Winters, K. B. & Koseff, J. R. 2008 Density stratification, turbulence, but how much mixing? Annu. Rev. Fluid Mech. 40, 169–184.CrossRefGoogle Scholar
Jackson, P. R. & Rehmann, C. R. 2003 Laboratory measurements of differential diffusion in a diffusively stable, turbulent flow. J. Phys. Oceanogr. 33 (8), 1592–1603.CrossRefGoogle Scholar
Klymak, J. M., Legg, S. & Pinkel, R. 2010 A simple parameterization of turbulent tidal mixing near supercritical topography. J. Phys. Oceanogr. 40 (9), 2059–2074.Google Scholar
Large, W. G., McWilliams, J. C. & Doney, S. C. 1994 Oceanic vertical mixing: a review and a model with a nonlocal boundary layer parameterization. Rev. Geophys. 32 (4), 363–403.Google Scholar
Ledwell, J. R., Montgomery, E. T., Polzin, K. L., St. Laurent, L. C., Schmitt, R. W. & Toole, J. M. 2000 Evidence for enhanced mixing over rough topography in the abyssal ocean. Nature 403, 179–182.Google Scholar
Linden, P. F. 1979 Mixing in stratified fluids. Geophys. Astrophys. Fluid Dyn. 13, 3–23.CrossRefGoogle Scholar
Lorenz, E. N. 1955 Available potential energy and the maintenance of the general circulation. Tellus 7, 157–167.Google Scholar
Lozovatsky, I. D. & Fernando, H. J. S. 2013 Mixing efficiency in natural flows. Phil. Trans. R. Soc. Lond. A 371, 20120213.Google Scholar
Maday, Y., Patera, A. T. & Rønquist, E. M. 1990 An operator-integration-factor splitting method for time-dependent problems: application to incompressible fluid flow. J. Sci. Comput. 5 (4), 263–292.Google Scholar
Martin, J. E. & Rehmann, C. R. 2006 Layering in a flow with diffusively stable temperature and salinity stratification. J. Phys. Oceanogr. 36 (7), 1457–1470.Google Scholar
Mashayek, A., Caulfield, C. P. & Peltier, W. R. 2013 Time-dependent, non-monotonic mixing in stratified turbulent shear flows: implications for oceanographic estimates of buoyancy flux. J. Fluid Mech. 736, 570–593.CrossRefGoogle Scholar
Mashayek, A. & Peltier, W. R. 2013 Shear induced mixing in geophysical flows: does the route to turbulence matter to its efficiency? J. Fluid Mech. 725, 216–261.CrossRefGoogle Scholar
Meyer, C. R. & Linden, P. F. 2014 Stratified shear flow: experiments in an inclined duct. J. Fluid Mech. 753, 242–253.Google Scholar
Moum, J. N. 1990 The quest for $K_{{\it\rho}}$ –preliminary results from direct measurements of turbulent fluxes in the ocean. J. Phys. Oceanogr. 20, 1980–1984.Google Scholar
Osborn, T. R. 1980 Estimates of the local rate of vertical diffusion from dissipation measurements. J. Phys. Oceanogr. 10, 83–89.Google Scholar
Osborn, T. R. & Cox, C. S. 1972 Oceanic fine structure. J. Geophys. Astrophys. Fluid Dyn. 3 (1), 321–345.Google Scholar
Peltier, W. R. & Caulfield, C. P. 2003 Mixing efficiency in stratified shear flows. Annu. Rev. Fluid Mech. 35, 135–167.Google Scholar
Peters, H., Gregg, M. C. & Toole, J. M. 1988 On the parameterization of equatorial turbulence. J. Geophys. Res. 93 (C2), 1199–1218.Google Scholar
Prandtl, L. 1925 Bericht über die entstehung der turbulenz. Z. Angew. Math. Mech. 5, 136–139.Google Scholar
Rehmann, C. R. & Koseff, J. R. 2004 Mean potential energy change in stratified grid turbulence. Dyn. Atmos. Oceans 37, 271–294.CrossRefGoogle Scholar
Salehipour, H., Peltier, W. R. & Mashayek, A. 2015 Turbulent diapycnal mixing in stratified shear flows: the influence of Prandtl number on mixing efficiency and transition at high Reynolds number. J. Fluid Mech. 773, 178–223.Google Scholar
Shih, L. H., Koseff, J. R., Ivey, G. N. & Ferziger, J. H. 2005 Parameterization of turbulent fluxes and scales using homogeneous sheared stably stratified turbulence simulations. J. Fluid Mech. 525, 193–214.Google Scholar
Simmons, H. L., Jayne, S. R., St. Laurent, L. C. & Weaver, A. J. 2004 Tidally driven mixing in a numerical model of the ocean general circulation. Ocean Model. 6 (3), 245–263.Google Scholar
Smyth, W. D., Carpenter, J. R. & Lawrence, G. A. 2007 Mixing in symmetric Holmboe waves. J. Phys. Oceanogr. 37, 1566–1583.Google Scholar
Smyth, W. D. & Moum, J. N. 2000a Anisotropy of turbulence in stably stratified mixing layers. Phys. Fluids 12 (6), 1343–1362.Google Scholar
Smyth, W. D. & Moum, J. N. 2000b Length scales of turbulence in stably stratified mixing layers. Phys. Fluids 12, 1327–1342.Google Scholar
Smyth, W. D., Moum, J. & Caldwell, D. 2001 The efficiency of mixing in turbulent patches: inferences from direct simulations and microstructure observations. J. Phys. Oceanogr. 31, 1969–1992.Google Scholar
Smyth, W. D., Nash, J. D. & Moum, J. N. 2005 Differential diffusion in breaking Kelvin–Helmholtz billows. J. Phys. Oceanogr. 35 (6), 1004–1022.Google Scholar
Stillinger, D. C., Helland, K. N. & Van Atta, C. W. 1983 Experiments on the transition of homogeneous turbulence to internal waves in a stratified fluid. J. Fluid Mech. 131, 91–122.Google Scholar
St. Laurent, L., Naveira Garabato, A. C., Ledwell, J. R., Thurnherr, A. M., Toole, J. M. & Watson, A. J. 2012 Turbulence and diapycnal mixing in drake passage. J. Phys. Oceanogr. 42 (12), 2143–2152.Google Scholar
St. Laurent, L. C., Simmons, H. L. & Jayne, S. R. 2002 Estimates of tidally driven enhanced mixing in the deep ocean. Geophys. Res. Lett. 29 (23), 2106.Google Scholar
Tailleux, R. 2009 On the energetics of stratified turbulent mixing, irreversible thermodynamics, boussinesq models and the ocean heat engine controversy. J. Fluid Mech. 638, 339–382.Google Scholar
Taylor, G. I. 1915 Eddy motion in the atmosphere. Phil. Trans. R. Soc. Lond. A 215, 1–26.Google Scholar
Venayagamoorthy, S. K. & Stretch, D. D. 2006 Lagrangian mixing in decaying stably stratified turbulence. J. Fluid Mech. 564, 197–226.CrossRefGoogle Scholar
Venayagamoorthy, S. K. & Stretch, D. D. 2010 On the turbulent Prandtl number in homogeneous stably stratified turbulence. J. Fluid Mech. 644, 359–369.Google Scholar
Waterhouse, A. F., MacKinnon, J. A., Nash, J. D., Alford, M. H., Kunze, E., Simmons, H. L., Polzin, K. L., St. Laurent, L. C, Sun, O., Pinkel, R., Talley, L. D., Whalen, C. B., Huussen, T. N., Carter, G. S., Fer, I., Waterman, S., Naveira Garabato, A. C., Sanford, T. B. & Lee, C. M. 2014 Global patterns of diapycnal mixing from measurements of the turbulent dissipation rate. J. Phys. Oceanogr 44, 1854–1872.Google Scholar
Winters, K. B. & D’Asaro, E. A. 1996 Diascalar flux and the rate of fluid mixing. J. Fluid Mech. 317, 179–193.Google Scholar
Winters, K. B., Lombard, P. N., Riley, J. J. & D’Asaro, E. A. 1995 Available potential energy and mixing in density-stratified fluids. J. Fluid Mech. 289, 115–128.Google Scholar
Wunsch, C. & Ferrari, R. 2004 Vertical mixing, energy, and the general circulation of the oceans. Annu. Rev. Fluid Mech. 36, 281–314.Google Scholar