Hostname: page-component-848d4c4894-nr4z6 Total loading time: 0 Render date: 2024-05-07T09:50:56.626Z Has data issue: false hasContentIssue false
  • English
  • Français

Almost everywhere convergence of ergodic series

Published online by Cambridge University Press:  06 October 2015

AIHUA FAN
AIHUA FAN*
Affiliation:
School of Mathematics and Statistics, Central China Normal University, 430079 Wuhan, China and
Get access Rights & Permissions [Opens in a new window]

Abstract

We consider ergodic series of the form 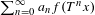$\sum _{n=0}^{\infty }a_{n}f(T^{n}x)$, where 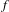$f$ is an integrable function with zero mean value with respect to a $T$-invariant measure $\unicode[STIX]{x1D707}$. Under certain conditions on the dynamical system $T$, the invariant measure $\unicode[STIX]{x1D707}$ and the function $f$, we prove that the series converges $\unicode[STIX]{x1D707}$-almost everywhere if and only if 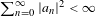$\sum _{n=0}^{\infty }|a_{n}|^{2}<\infty$, and that in this case the sum of the convergent series is exponentially integrable and satisfies a Khintchine-type inequality. We also prove that the system 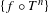$\{f\circ T^{n}\}$ is a Riesz system if and only if the spectral measure of 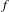$f$ is absolutely continuous with respect to the Lebesgue measure and the Radon–Nikodym derivative is bounded from above as well as from below by a constant. We check the conditions for Gibbs measures $\unicode[STIX]{x1D707}$ relative to hyperbolic dynamics $T$ and for Hölder functions 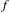$f$. An application is given to the study of differentiability of the Weierstrass-type functions 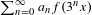$\sum _{n=0}^{\infty }a_{n}f(3^{n}x)$.

Type
Research Article
Information
Ergodic Theory and Dynamical Systems , Volume 37 , Issue 2 , April 2017 , pp. 490 - 511
Copyright
© Cambridge University Press, 2015 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Aistleitner, C.. Convergence of ∑c k f (kx) and the Lip 𝛼 class. Proc. Amer. Math. Soc. 140(11) (2012), 3893–3903.Google Scholar
Aistleitner, C.. Metric number theory, lacunary series and systems of dilated functions. Uniform Distribution and Quasi-Monte Carlo Methods (Radon Series on Computational and Applied Mathematics, 15) . de Gruyter, Berlin, 2014, pp. 1–16.Google Scholar
Alexits, G.. Convergence Problems of Orthogonal Series. Pergamon, New York, 1961.Google Scholar
Assani, I. and Lin, M.. On the one-sided ergodic Hilbert transform, ergodic theory and related fields. Contemp. Math. 430 (2007), 21–39.Google Scholar
Azuma, K.. Weighted sums of certain dependent random variables. Tohoku Math. J. (2) 19(3) (1967), 357–367.Google Scholar
Barański, K., Bárány, B. and Romanowska, J.. On the dimension of the graph of the classical Weierstrass functions. Adv. Math. 265 (2014), 32–59.Google Scholar
Berkes, I. and Weber, M.. On the Convergence of ∑c k f (n k x) (Memoirs of the American Mathematical Society, 201, No. 943) . American Mathematical Society, Providence, RI, 2009.Google Scholar
Berkes, I. and Weber, M.. On series ∑c k f (nx) and Khintchin’s conjecture. Israel J. Math. 201 (2014), 593–609.Google Scholar
Bowen, R.. Equilibrium States and the Ergodic Theory of Anosov Diffeomorphisms (Lecture Notes in Mathematics, 470) . Springer, Berlin, 1975.Google Scholar
Brémont, J.. Davenport and almost everywhere convergence. Q. J. Math. 62 (2011), 825–843.Google Scholar
Carleson, L.. On convergence and growth of partial sums of Fourier series. Acta Math. 116 (1966), 135–157.CrossRefGoogle Scholar
Cohen, G. and Lin, M.. Extensions of the Menchoff–Rademacher theorem with applications to ergodic theory. Israel J. Math. 148(1) (2005), 41–86.Google Scholar
Cotlar, M.. A unified theory of Hilbert transforms and ergodic theorems. Rev. Mat. Cuyana 1 (1955), 105–167.Google Scholar
Cuny, C.. On the a.s. convergence of the one-sided ergodic Hilbert transform. Ergod. Th. & Dynam. Sys. 29 (2009), 1781–1788.Google Scholar
Daubechies, I.. Ten Lectures on Wavelets (CBMS-NSF Regional Conference Series in Applied Mathematics, 61) . Society for Industrial and Applied Mathematics, Philadelphia, PA, 1992.Google Scholar
Derriennic, Y.. Lois “zéro ou deux” pour les processus de Markov. Applications aux marches aléatoires. Ann. Inst. Henri Poincaré Probab. Stat. 12(2) (1976), 111–129.Google Scholar
Derriennic, Y. and Lin, M.. Fractional Poisson equations and ergodic theorems for fractional coboundaries. Israel J. Math. 123 (2001), 93–130.Google Scholar
Fan, A. H.. Quelques propriété des produits de Riesz. Bull. Sci. Math. 117(4) (1993), 421–439.Google Scholar
Fan, A. H.. Sur les dimensions de mesures. Studia Math. 111 (1994), 1–17.Google Scholar
Fan, A. H. and Jiang, Y. P.. On Ruelle–Perron–Frobenius operators I. Comm. Math. Phys. 223(1) (2001), 125–141.Google Scholar
Fan, A. H. and Jiang, Y. P.. On Ruelle–Perron–Frobenius operators II. Comm. Math. Phys. 223(1) (2001), 143–159.Google Scholar
Fan, A. H. and Schmeling, J.. On fast Birkhoff averaging. Math. Proc. Cambridge Philos. Soc. 135 (2003), 443–467.Google Scholar
Fan, A. H. and Schmeling, J.. Everywhere divergence of one-sided ergodic Hilbert transform. Preprint, 2015.Google Scholar
Gaposhkin, V. F.. Lacunary series and independent functions. Uspekhi Mat. Nauk 21 (1966), 3–82 (in Russian).Google Scholar
Gaposhkin, V. F.. On series with respect to the system {𝜙(nx)}. Mat. Sb. 69(111) (1966), 328–353.Google Scholar
Gaposhkin, V. F.. Convergence and divergence systems. Math. Notes 4(3) (1968), 645–649.Google Scholar
Gaposhkin, V. F.. On the dependence of the convergence rate in the strong law of large numbers for stationary processes on the rate of decay of the correlation function. Theory Probab. Appl. 26 (1981), 706–720.Google Scholar
Gaposhkin, V. F.. Spectral criteria for existence of generalized ergodic transforms. Theory Probab. Appl. 41(2) (1996), 247–264.Google Scholar
Gröchenig, K. and Madych, W.. Multiresolution analysis, Haar basis and self-similar tilings. IEEE Trans. Inform. Theory 38(2) (1992), 558–568.CrossRefGoogle Scholar
Halmos, P.. A nonhomogeneous ergodic theorem. Trans. Amer. Math. Soc. 66 (1949), 284–288.Google Scholar
Hardy, G. H.. Weierstrass’s non-differentiable function. Trans. Amer. Math. Soc. 17(3) (1916), 301–325.Google Scholar
Hedenmalm, H., Lindqvist, P. and Seip, A.. A Hilbert space of Dirichlet series and systems of dilated functions in L 2(0, 1). Duke Math. J. 86(1) (1997), 1–37.Google Scholar
Izumi, S.. A non-homogeneous ergodic theorem. Proc. Imp. Acad. Tokyo 15 (1939), 189–192.Google Scholar
Kac, M., Salem, R. and Zygmund, A.. A gap theorem. Trans. Amer. Math. Soc. 63(2) (1948), 235–243.Google Scholar
Kachurovskii, A. G.. The rate of convergence in ergodic theorems. Russian Math. Surveys 51(4) (1996), 653–703.Google Scholar
Kahane, J. P.. Some Random Series of Functions (Cambridge Studies in Advanced Mathematics, 5) . 2nd edn. Cambridge University Press, Cambridge, 1985.Google Scholar
Kakutani, S. and Petersen, K.. The speed of convergence in the ergodic theorem. Monatsh. Math. 91 (1981), 11–18.Google Scholar
Katznelson, Y.. An Introduction to Harmonic Analysis. John Wiley, New York, 1968.Google Scholar
Krengel, U.. Ergodic Theorems. de Gruyter, Berlin, New York, 1982.Google Scholar
Lagarias, J. C. and Wang, Y.. Integral self-affine tiles in ℝ n II. J. Fourier Anal. Appl. 3(1) (1997), 83–102.Google Scholar
Móricz, F.. Moment inequalities and the strong law of large numbers. Z. Wahrscheinlichkeitstheor Verwandte 35 (1976), 299–314.Google Scholar
Nikishin, E. M. . Series of a system {𝛷(nx)}. Math. Notes 5(5) (1969), 316–319.Google Scholar
Parry, W. and Pollicott, M.. Zeta functions and the periodic orbit structure of hyperbolic dynamics. Astérisque 187–188 (1990), 268 pp.Google Scholar
Paszkiewicz, A.. A complete characterization of coefficients of a.e. convergent orthogonal series and majorizing measures. Invent. Math. 180 (2010), 55–110.Google Scholar
Petersen, K.. Ergodic Theory. Cambridge University Press, Cambridge, 1983.Google Scholar
Peyrière, J.. Almost everywhere convergence of lacunary trigonometric series with respect to Riesz products. J. Aust. Math. Soc. Ser. A 48(3) (1990), 376–383.Google Scholar
Polya, G. and Szegö, G.. Problems and Theorems in Analysis II. Springer, New York, 1976.Google Scholar
Ruelle, D.. Thermodynamic Formalism. Addison-Wesley, Reading, MA, 1978.Google Scholar
Sarig, O.. Subexponential decay of correlations. Invent. Math. 150 (2002), 629–653.Google Scholar
Walters, P.. An Introduction to Ergodic Theory (Graduate Texts in Mathematics, 79) . Springer, New York, 1982.Google Scholar
Weber, M.. Entropie métrique et convergence presque partout (Travaux en cours, 58) . Hermann, Paris, 1998.Google Scholar
Young, L. S.. Statistical properties of dynamical systems with some hyperbolicity. Ann. of Math. (2) 147(3) (1998), 585–650.Google Scholar
Young, L. S.. Recurrence times and rates of mixing. Israel J. Math. 110 (1999), 153–188.Google Scholar