Hostname: page-component-8448b6f56d-tj2md Total loading time: 0 Render date: 2024-04-24T18:45:33.468Z Has data issue: false hasContentIssue false
  • English
  • Français

CONSTRUCTING WADGE CLASSES

Part of: Set theory Connections with other structures, applications

Published online by Cambridge University Press:  26 January 2022

RAPHAËL CARROY ,
ANDREA MEDINI  and
SANDRA MÜLLER Open the ORCID record for SANDRA MÜLLER [Opens in a new window]
RAPHAËL CARROY
Affiliation:
DIPARTIMENTO DI MATEMATICA “GIUSEPPE PEANO” PALAZZO CAMPANA, UNIVERSITÁ DI TORINO VIA CARLO ALBERTO 10 10123TURIN, ITALYE-mail: raphael.carroy@unito.it
ANDREA MEDINI
Affiliation:
INSTITUT FÜR DISKRETE MATHEMATIK UND GEOMETRIE TECHNISCHE UNIVERSITÄT WIEN WIEDNER HAUPTSTRASSE 8-10/104 1040VIENNA, AUSTRIAE-mail: andrea.medini@tuwien.ac.atE-mail: sandra.mueller@tuwien.ac.atURL: http://muellersandra.github.io
SANDRA MÜLLER
Affiliation:
INSTITUT FÜR DISKRETE MATHEMATIK UND GEOMETRIE TECHNISCHE UNIVERSITÄT WIEN WIEDNER HAUPTSTRASSE 8-10/104 1040VIENNA, AUSTRIAE-mail: andrea.medini@tuwien.ac.atE-mail: sandra.mueller@tuwien.ac.atURL: http://muellersandra.github.io
Get access Rights & Permissions [Opens in a new window]

Abstract

We show that, assuming the Axiom of Determinacy, every non-selfdual Wadge class can be constructed by starting with those of level 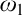$\omega _1$ (that is, the ones that are closed under Borel preimages) and iteratively applying the operations of expansion and separated differences. The proof is essentially due to Louveau, and it yields at the same time a new proof of a theorem of Van Wesep (namely, that every non-selfdual Wadge class can be expressed as the result of a Hausdorff operation applied to the open sets). The exposition is self-contained, except for facts from classical descriptive set theory.

Keywords

Wadge theorylevelexpansionseparated differencesdeterminacyHausdorff operationω-ary Boolean operation

MSC classification

Primary: 03E15: Descriptive set theory
Secondary: 54H05: Descriptive set theory (topological aspects of Borel, analytic, projective, etc. sets) 03E60: Determinacy principles
Type
Articles
Information
Bulletin of Symbolic Logic , Volume 28 , Issue 2 , June 2022 , pp. 207 - 257
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press on behalf of The Association for Symbolic Logic

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Andretta, A., The SLO principle and the Wadge hierarchy , Foundations of the formal sciences V. Infinite games (S. Bold, B. Löwe, T. Räsch, and J. van Benthem, editors), Studies in Logic, vol. 11, College Publications, London, 2007, pp. 1–38.Google Scholar
Andretta, A., Hjorth, G., and Neeman, I., Effective cardinals of boldface pointclasses . Journal of Mathematical Logic , vol. 7 (2007), no. 1, pp. 35–82.10.1142/S0219061307000615CrossRefGoogle Scholar
Andretta, A. and Martin, D. A., Borel-Wadge degrees . Fundamenta Mathematicae , vol. 177 (2003), no. 2, pp. 173–190.10.4064/fm177-2-5CrossRefGoogle Scholar
Carroy, R., Medini, A., and Müller, S., Every zero-dimensional homogeneous space is strongly homogeneous under determinacy . Journal of Mathematical Logic , vol. 20 (2020), no. 3, 2050015.10.1142/S0219061320500154CrossRefGoogle Scholar
van Engelen, F., Homogeneous Borel sets . Proceedings of the American Mathematical Society , vol. 96 (1986), pp. 673–682.10.1090/S0002-9939-1986-0826501-2CrossRefGoogle Scholar
van Engelen, A. J. M.. Homogeneous Zero-dimensional Absolute Borel Sets . CWI Tract, 27 (J. W. de Bakker, M. Hazewinkel, and J. K. Lenstra, editors), Stichting Mathematisch Centrum, Centrum voor Wiskunde en Informatica, Amsterdam, 1986. Available at http://ir.cwi.nl/pub/12724.Google Scholar
van Engelen, F., On Borel ideals . Annals of Pure and Applied Logic , vol. 70 (1994), no. 2, pp. 177–203.10.1016/0168-0072(94)90029-9CrossRefGoogle Scholar
Engelking, R., General Topology . Revised and completed edition. Sigma Series in Pure Mathematics, vol. 6, Heldermann Verlag, Berlin, 1989.Google Scholar
Harrington, L., Analytic determinacy and  ${0}^{\sharp }$ . Journal of Symbolic Logic , vol. 43 (1978), no. 4, pp. 685–693.10.2307/2273508CrossRefGoogle Scholar
Hausdorff, F., Gesammelte Werke. Band III: Mengenlehre (1927, 1935). Deskriptive Mengenlehre und Topologie (U. Felgner, H. Herrlich, M. Hušek, V. Kanovei, P. Koepke, G. Preuß, W. Purkert, and E. Scholz, editors), Springer, Berlin, 2010.Google Scholar
Howard, P. and Rubin, J. E., Consequences of the Axiom of Choice , Mathematical Surveys and Monographs, vol. 59, American Mathematical Society, Providence, Rhode Island, 1998.10.1090/surv/059CrossRefGoogle Scholar
Jech, T., Set Theory . The third millennium edition, revised and expanded. Springer Monographs in Mathematics, Springer-Verlag, Berlin, 2003.Google Scholar
Kanamori, A., The Higher Infinite . Second ed. Springer Monographs in Mathematics, Springer-Verlag, Berlin, 2009.Google Scholar
Kechris, A. S., Classical Descriptive Set Theory , Graduate Texts in Mathematics, vol. 156, Springer-Verlag, New York, 1995.10.1007/978-1-4612-4190-4CrossRefGoogle Scholar
Louveau, A., Effective descriptive set theory. Unpublished manuscript.Google Scholar
Louveau, A., Some results in the Wadge hierarchy of Borel sets , Wadge Degrees and Projective Ordinals. The Cabal Seminar Volume II (A. S. Kechris, B. Löwe, and J. R. Steel, editors), Lecture Notes in Logic, vol. 37, Association of Symbolic Logic, La Jolla, CA, 2012, pp. 3–23. Originally published in: Cabal seminar 79-81 , 28–55, Lecture Notes in Mathematics, vol. 1019, Springer, Berlin, 1983.Google Scholar
Louveau, A. and Saint-Raymond, J., Les propriétés de réduction et de norme pour les classes de Boréliens . Fundamenta Mathematicae , vol. 131 (1988), no. 3, pp. 223–243.10.4064/fm-131-3-223-243CrossRefGoogle Scholar
Louveau, A. and Saint-Raymond, J., The strength of Borel Wadge determinacy , Wadge degrees and projective ordinals. The Cabal Seminar. Volume II (A. S. Kechris, B. Löwe, and J. R. Steel, editors), Lecture Notes in Logic, vol. 37, Association of Symbolic Logic, La Jolla, CA, 2012, pp. 74–101. Originally published in: Cabal seminar 81-85 , 1–30, Lecture Notes in Mathematics, vol. 1333, Springer, Berlin, 1988.Google Scholar
Martin, D. A., Borel determinacy . Annals of Mathematics (2) , vol. 102 (1975), no. 2, pp. 363–371.10.2307/1971035CrossRefGoogle Scholar
Martin, D. A., A purely inductive proof of Borel determinacy , Recursion Theory (A. Nerode and R. A. Shore, editors), Proceedings of Symposia in Pure Mathematics, vol. 42, American Mathematical Society, Providence, RI, 1985, pp. 303–308.10.1090/pspum/042/791065CrossRefGoogle Scholar
Medini, A. and Zdomskyy, L., Between Polish and completely Baire . Archive for Mathematical Logic , vol. 54 (2015), no. 1–2, pp. 231–245.10.1007/s00153-014-0409-4CrossRefGoogle Scholar
Motto Ros, L., Borel-amenable reducibilities for sets of reals . Journal of Symbolic Logic , vol. 74 (2009), no. 1, pp. 27–49.Google Scholar
Müller, S., Schindler, R., and Woodin, W. H., Mice with finitely many Woodin cardinals from optimal determinacy hypotheses . Journal of Mathematical Logic , vol. 20 (2020), Suppl. 1, 1950013.10.1142/S0219061319500132CrossRefGoogle Scholar
Neeman, I., Determinacy in   $L(\mathbb{R})$ , Handbook of Set Theory (M. Foreman and A. Kanamori, editors), Springer, Dordrecht, 2010, pp. 1877–1950.10.1007/978-1-4020-5764-9_23CrossRefGoogle Scholar
Solovay, R. M., The independence of DC from AD, Cabal Seminar 76–77 , Lecture Notes in Mathematics, vol. 689, Springer, Berlin, Heidelberg, 1978, pp. 171–183.10.1007/BFb0069299CrossRefGoogle Scholar
Steel, J. R., Determinateness and subsystems of analysis , Ph.D. thesis, University of California, Berkeley, 1977.Google Scholar
Van Wesep, R. A., Subsystems of second-order arithmetic, and descriptive set theory under the axiom of determinateness, Ph.D. thesis, University of California, Berkeley, 1977.Google Scholar
Wadge, W. W., Reducibility and determinateness on the Baire space , Ph.D. thesis. University of California, Berkeley, 1984.Google Scholar
Wadge, W. W., Early investigations of the degrees of Borel sets , Wadge Degrees and Projective Ordinals. The Cabal Seminar. Volume II (A. S. Kechris, B. Löwe, and J. R. Steel, editors), Lecture Notes in Logic, vol. 37, Association of Symbolic Logic, La Jolla, CA, 2012, pp. 3–23.Google Scholar
Zafrany, S.. On the topological strength of Boolean set operations. Unpublished manuscript, 1989.Google Scholar