1 Introduction

Since the indirect debate between Schumpeter (1942) and Arrow (1962), the relationship between market structure and innovative activity has been of long-standing interest in industrial economics. Especially since the late ’70s of the past century a rapidly growing literature has tackled such relationship within a variety of models.Footnote 1 The main findings of such models are by now summarized also in advanced textbooks of Industrial Organization (see, e.g. Tirole 1988).

In this paper, we focus on a small but highly influential subset of that literature, dealing with the persistence of monopoly under technological progress and the threat of entry. More precisely, we shall focus on the models by Gilbert and Newbery (1982) and Reinganum (1983). Both papers investigate a setting which may take the structure of an auction concerning a patented innovation which the inventor wants to sell, not being endowed with any productive facilities. Gilbert and Newbery (1982) consider a deterministic auction for a product innovation protected by an everlasting patent between an incumbent monopolist (firm I) and a potential entrant (firm E). They prove that firm I has a greater incentive than firm E to obtain the patent for the new product, even a sleeping patent, to preempt entry. In their own words, this is because “the monopolist will spend more on R&D than the rival if entry results in any reduction of total profits below the joint maximization level ” (p. 516, italics in the original).

Reinganum (1983) models a stochastic race for a drastic innovation, showing that the entrant has a greater incentive than the incumbent to patent. She reformulates Gilbert and Newbery’s model considering “a case of cost reducing innovation in an industry with constant returns to scale” (p. 741, italics added). Notice that this is the setting which typically entered the textbook presentation of the debate on the persistence of monopoly (see, e.g., Tirole 1988, Chap. 10).

Apparently, the presence of uncertaintyFootnote 2 drives the opposite conclusion reached by Reinganum with respect to Gilbert and Newbery. Such a conclusion, however, would be misleading: other assumptions of their models play a key role and should be taken into account. We shall show indeed that, in a deterministic setting, the incumbent may lose the auction for the product innovation modelled in Gilbert and Newbery (1982) and win the race for the process innovation as modeled in Reinganum (1983). In fact, the presence of diseconomies of scope may disadvantage a multiproduct incumbent and prevent it from deterring entry by a single-product duopolist. This scenario is ruled out by Gilbert and Newbery’s assumption that “the monopolist suffers no diseconomies in the production of the substitute relative to production by rival firm” (p. 516). On the other hand, by considering diseconomies of scale in the production of the homogeneous good, even without uncertainty in the R&D technology, Reinganum’s conclusion may be reversed with the incumbent monopolist winning the patent race and strenghtening its leadership.

The remainder of the paper is organised as follows. In Sect. 2, we revisit Gilbert and Newbery’s model of product innovation, illustrating the consequences of diseconomies of scope. In Sect. 3, we explore the impact of decreasing returns to scale in Reinganum’s model of process innovation. Section 4 concludes.

2 Product innovation

Following Gilbert and Newbery (1982), we consider an industry in which the incumbent is a single-product firm operating at a constant marginal and average cost \(c\in \left( 0,a\right)\) and facing a linear demand for product 1, \(p_{1}=a-Q_{1},\) where \(p_{1}\in \left[ c,a\right)\) is market price, \(Q_{1}\) is the monopolist’s output and a is the reservation price.

A single,Footnote 3 exogenous and fully patentable innovation takes the form of a new variety (product 2) horizontally differentiated with respect to product 1, whereby the relevant post-innovation demand system is

$$\begin{aligned} p_{i}=a-q_{i}^{k}-sq_{j}^{\ell },\quad i,j=1,2; \quad k,\ell =E,I \end{aligned}$$
(1)

as in Singh and Vives (1984), irrespective of the innovator’s identity. In (1), \(q_{i}^{k}\) defines the output of firm k using technology i , and parameter \(s\in \left( 0,1\right]\) measures the degree of substitutability between the two varieties. We define (1) as a system of inverse demand functions, to mean that the eventual post-entry game takes place under Cournot competition.

The post-innovation profit function associated with the production of a single variety in isolation is

$$\begin{aligned} \pi _{i}^{k}=\left( p_{i}-c\right) q_{i}^{k}=\left( a-q_{i}^{k}-sq_{j}^{\ell }-c\right) q_{i}^{k} \end{aligned}$$
(2)

where marginal cost c is common to both varieties. If the incumbent innovates and becomes a multiproduct monopolist, its total cost function is \(C_{M}\left( 1,2\right) =c\left( q_{1}+q_{2}\right) +\beta q_{1}q_{2},\) where \(\beta\) is real and finite; if positive (negative), there exist diseconomies (economies) of scope. For the sake of brevity, and without further loss of generality, we pose \(c=0\). Accordingly, the profit function of the multiproduct monopolist is

$$\begin{aligned} \Pi _{M}\left( 1,2\right) =\left( a-q_{1}-sq_{2}\right) q_{1}+\left( a-q_{2}-sq_{1}\right) q_{2}-\beta q_{1}q_{2} \end{aligned}$$
(3)

The maximization problem of the multiproduct monopolist can be solved through the following first order condition (FOC):

$$\begin{aligned} \frac{\partial \Pi _{M}\left( 1,2\right) }{\partial q_{i}}=a-2q_{i}-\left( \beta +2s\right) q_{j}=0 \end{aligned}$$
(4)

so that the optimal quantity of each single variety is

$$\begin{aligned} q_{M}^{*}\left( 1,2\right) =\frac{a}{2\left( 1+s\right) +\beta } \end{aligned}$$
(5)

and the resulting equilibrium profits amount to

$$\begin{aligned} \Pi _{M}^{*}\left( 1,2\right) =\frac{a^{2}}{2\left( 1+s\right) +\beta } \end{aligned}$$
(6)

Then, note that the associated equilibrium price,

$$\begin{aligned} p_{M}^{*}\left( 1,2\right) =\frac{a\left( 1+s+\beta \right) }{2\left( 1+s\right) +\beta } \end{aligned}$$
(7)

is positive for all \(\beta >-\left( 1+s\right) ,\) which suffices to ensure the positivity of \(q_{M}^{*}\left( 1,2\right)\) and \(\Pi _{M}^{*}\left( 1,2\right)\). This is because, once \(\beta\) becomes negative (i.e., there exist economies of scope), optimal output expands at an increasing rate and shoots up to infinity in the limit, as \(\beta\) approaches \(-2\left( 1+s\right)\), which is the critical threshold of \(\beta\) at which the denominator of the three relevant magnitudes becomes nil. Well before doing so (since \(-\left( 1+s\right) >-2\left( 1+s\right)\)), the expansion of production drives the equilibrium price to zero, which coincides with the level of marginal cost.

In the Cournot-Nash equilibrium resulting from the entrant patenting the innovation, profits are

$$\begin{aligned} \pi _{1}^{I}=\pi _{2}^{E}=\frac{a^{2}}{\left( 2+s\right) ^{2}} \end{aligned}$$
(8)

Now we are in a position to compare \(\Pi _{M}^{*}\left( 1,2\right)\) with the sum of duopoly profits appearing in (8):

$$\begin{aligned} \Pi _{M}^{*}\left( 1,2\right) -\pi _{1}^{I}-\pi _{2}^{E}=\frac{ a^{2}\left( s^{2}-2\beta \right) }{\left( 2+s\right) ^{2}\left[ 2\left( 1+s\right) +\beta \right] } \end{aligned}$$
(9)

This seems to suggest that the incumbent will lose the auction if \(\beta >s^{2}/2\). However, one should consider the possibility for the incumbent to acquire the patent on product 2 without producing it. In such a case, the sum of duopoly profits \(\pi _{1}^{I}+\pi _{2}^{E}\) has to be compared with \(\Pi _{M}^{*}\left( 1\right) =a^{2}/4\):

$$\begin{aligned} \Pi _{M}^{*}\left( 1\right) -\pi _{1}^{I}-\pi _{2}^{E}=\frac{a^{2}\left[ s^{2}-4\left( 1-s\right) \right] }{4\left( 2+s\right) ^{2}}>0 \end{aligned}$$
(10)

for all \(s\in \left( 2\left( \sqrt{2}-1\right) ,1\right]\). The last step consists in checking if and when the incumbent has an incentive to become multiproduct, which requires evaluating the sign of

$$\begin{aligned} \Pi _{M}^{*}\left( 1,2\right) -\Pi _{M}^{*}\left( 1\right) =\frac{ a^{2}\left[ 2\left( 1-s\right) -\beta \right] }{4\left[ 2\left( 1+s\right) +\beta \right] }, \end{aligned}$$
(11)

the expression on the right hand side being positive for all \(\beta <2\left( 1-s\right)\).

Fig. 1
figure 1

Preemption vs entry

Full size image

Taken together, (9), (10) and (11) identify six regions in the space \(\left( s,\beta \right)\) as illustrated in Fig. 1:

  • In region \(\left( 1a\right)\), the incumbent prefers to sell a single variety and wins the auction.

  • In region \(\left( 1b\right)\), the incumbent still prefers to sell a single variety and wins the auction, but it would win even selling both varieties.

  • In region \(\left( 1c\right)\), it is efficient for the incumbent to sell both varieties, but it wins the auction in both scenarios, i.e., irrespective of whether product 2 is marketed or not.

  • In region \(\left( 1d\right)\), the only way for the incumbent to outbid the rival is to sell both varieties, which is also efficient from the incumbent’s standpoint.

  • In region \(\left( 1e\right)\), the incumbent would prefer to supply both varieties but it cannot win the auction.

  • In region \(\left( 1f\right)\), the incumbent prefers to supply one variety only but it doesn’t win the auction.

The above results deserve a few comments. In regions \(\left( 1a,1b\right) ,\) monopoly persists but the incumbent’s strict preference for a single variety makes the ex ante and ex post monopolies observationally equivalent. This can be interpreted as a situation in which the incumbent gets the patent to preempt entry but keeps it asleep; however, the monopolist might as well supply the new variety and drop the old one, and consumers would be totally unable to tell the difference because they face a single demand function for a homogeneous good. In regions \(\left( 1c,1d\right) ,\) the incumbent acquires the patent and the ex ante and ex post monopolies are different as the second is a multiproduct one. This happens because in region \(\left( 1d\right) ,\) given the extent of economies/diseconomies of scope, the presence of relatively high degree of differentiation favours, in line of principle, the entrant, so that in order to outbid the entrant the incumbent needs to become multiproduct, while this is not necessary in \(\left( 1c\right) ,\) as in this area varieties are closer substitutes. This also applies for any \(\beta \in \left( -\left( 1+s\right) ,0\right) ,\) i.e., as long as the economies of scope are not so large to push price below zero. Regions \(\left( 1e,1f\right)\) entail the emergence of duopoly, with the entrant outbidding the incumbent, the latter being hampered by a significant degree of diseconomies of scope.

As for the impact of scope diseconomies when products are very weak substitutes, notice that, as s becomes arbitrarily small, the duopoly tends to collapse to separate monopolies, i.e.,

$$\begin{aligned} \lim _{s\rightarrow 0}\pi _{1}^{I}=\lim _{s\rightarrow 0}\pi _{1}^{E}=\Pi _{M}^{*}\left( 1\right) \end{aligned}$$
(12)

and therefore

$$\begin{aligned} \lim _{s\rightarrow 0}\left( \pi _{1}^{I}+\pi _{1}^{E}\right) =2\Pi _{M}^{*}\left( 1\right) \end{aligned}$$
(13)

but in regions \(\left( 1e,1f\right) ,\) it happens that \(\Pi _{M}^{*}\left( 1,2\right) \in \left( \Pi _{M}^{*}\left( 1\right) ,2\Pi _{M}^{*}\left( 1\right) \right)\) for any s,  including \(s=0\). This of course implies that the joint presence of scope diseconomies and differentiation paves the way to an Arrovian outcome.

On the basis of the foregoing discussion, we may formulate the following

Proposition 1

In presence of a sufficiently high degree of product differentiation, any level of diseconomies of scope may allow the entrant to outbid the incumbent and opens the way to a duopoly equilibrium.

As we know, the externality generated by joint production is ruled out by assumption in Gilbert and Newbery’s model. Taking it into consideration, one realises that, while product differentiation enters all profit functions irrespective of market structure, diseconomies of scope can only appear in the multiproduct monopolist’s, in such a way that the latter may not be able to at least replicate the profit performance of a duopolistic industry.

3 Process innovation

As in Reinganum (1983), we examine firms’ incentive towards process innovationFootnote 4 under the assumption of convex costs taking the form of \(C_{i}^{k}=c_{i}\left( q_{i}^{k}\right) ^{2},\) where \(i=H,L\) and \(k=E,I,\) and \(0\le c_{L}<c_{H}<a\).

Moreover, we stipulate that firm(s) sell(s) the same homogeneous good whose inverse demand function is either (i) \(p=a-q_{L}^{M}\) if the incumbent innovates and uses the new technology only in the ex-post monopoly; or (ii) \(p=a-q_{L}^{M}-q_{H}^{M}\) if the incumbent innovates and uses both technologies in the ex-post monopoly; or (iii) \(p=a-q_{L}^{E}-q_{H}^{I}\) if the entrant innovates and a Cournot-Nash duopoly game is played.

Before proceeding any further, it is appropriate to discuss the explicit assumption underpinning the above definition of the relevant market demand functions, in particular the post-innovation demand structure. In principle, if the incumbent obtains the patent, it could use the new technology in two possible ways: the first would consist in opening up a second production line or plant flanking the previous one, as we stipulate in (ii); the second would consist in dismissing the old technology by converting the initial plant to the new technology, and complement it with an additional plant incorporating the innovation as well. The latter case would obviously an equilibrium confirming the persistence of monopoly, for the obvious reason that a multiplant monopoly with a lower marginal cost necessarily outperforms a duopoly made up by single-plant firms, one being hampered by a higher marginal cost.Footnote 5

Now we may characterise the equilibrium outcome of the scenario in which the incumbent, in view of the persistence of its monopoly power, has in mind to use both technologies (if convenient and/or necessary). In case (i), the monopolist’s profit function is \(\pi _{L}^{M}=\left( a-q_{L}^{M}\right) q_{L}^{M}-c_{L}\left( q_{L}^{M}\right) ^{2}.\) In (ii), it is

$$\begin{aligned} \pi _{HL}^{M}=\left( a-q_{H}^{M}-q_{L}^{M}\right) q_{H}^{M}-c_{H}\left( q_{H}^{M}\right) ^{2}+\left( a-q_{H}^{M}-q_{L}^{M}\right) q_{L}^{M}-c_{L}\left( q_{L}^{M}\right) ^{2} \end{aligned}$$
(14)

while in the duopoly scenario, i.e., case (iii), firms’ profit functions are

$$\begin{aligned} \begin{array}{c} \pi _{H}^{I}=\left( a-q_{L}^{E}-q_{H}^{I}\right) q_{H}^{I}-c_{H}\left( q_{H}^{I}\right) ^{2} \\ \\ \pi _{L}^{E}=\left( a-q_{L}^{E}-q_{H}^{I}\right) q_{L}^{E}-c_{L}\left( q_{L}^{E}\right) ^{2} \end{array} \end{aligned}$$
(15)

As a preliminary step, we compare the post-innovation profits of the incumbent when it remains a monopolist. Unsurprisingly, given the convexity of the cost function, it turns out that it is convenient for the innovating monopolist to operate with both technologies. The two equilibrium profits are

$$\begin{aligned} \pi _{L}^{M}=\frac{a^{2}}{4\left( 1+c_{L}\right) }; \quad \pi _{HL}^{M}=\frac{ a^{2}\left( c_{L}+c_{H}\right) }{4\left( c_{L}+c_{H}+c_{L}c_{H}\right) } \end{aligned}$$
(16)

and

$$\begin{aligned} \pi _{HL}^{M}-\pi _{L}^{M}=\frac{a^{2}c_{L}^{2}}{4\left( 1+c_{L}\right) \left( c_{L}+c_{H}+c_{L}c_{H}\right) }>0 \end{aligned}$$
(17)

This is the outcome of Jensen’s inequality whereby the monopolist finds it convenient to split any given volume of production (in this case, the optimal output) between two plants, in order to keep marginal cost as low as possible, even if the efficiency level of the plants is not the same. That is,

Lemma 2

Under quadratic production costs, the ex-post monopolist will always use the old and new technology in combination.

Equilibrium duopoly outputs and profits are, respectively,

$$\begin{aligned} q_{H}^{I}=\frac{a\left( 1+2c_{L}\right) }{3+4c_{H}+4c_{L}\left( 1+c_{H}\right) }; \quad q_{L}^{E}=\frac{a\left( 1+2c_{H}\right) }{ 3+4c_{H}+4c_{L}\left( 1+c_{H}\right) } \end{aligned}$$
(18)

and

$$\begin{aligned} \pi _{H}^{I}=\frac{a^{2}\left( 1+2c_{L}\right) ^{2}\left( 1+c_{H}\right) }{ \left[ 3+4c_{H}+4c_{L}\left( 1+c_{H}\right) \right] ^{2}}; \quad \pi _{L}^{E}= \frac{a^{2}\left( 1+2c_{H}\right) ^{2}\left( 1+c_{L}\right) }{\left[ 3+4c_{H}+4c_{L}\left( 1+c_{H}\right) \right] ^{2}} \end{aligned}$$
(19)

Now it is appropriate to recall that any reduction in marginal production cost qualifies as a drastic innovation if and only if the firm holding the rights upon that innovation can use it drive the rival(s) out of business and set the pure monopoly price. The expression of \(q_{L}^{E}\) in (18 ) is strictly positive, and this fact immediately imply the following, which is indeed a direct consequence of the convexity of the cost function:

Lemma 3

Under quadratic production costs, process innovation is never drastic.

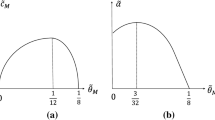
In other words, both firms are active in the post-innovation Cournot-Nash equilibrium, irrespective of the size of the cost reduction. On the basis of Lemma 2, we may anticipate that the incumbent will win the patent auction, as, according to the acquired wisdom from models without diseconomies (as in Gilbert and Newbery 1982), all else equal, a monopolist may at least replicate the profit performance of any more competitive industry structure. This means that \(\pi _{HL}^{M}>\pi _{H}^{I}+\pi _{L}^{E}\) for all \(a>c_{H}>c_{L}>0\).

Yet, what we are about to illustrate is that, behind this seemingly trivial conclusion, one may find out that there are relevant situations in which the incumbent must use the old technology to finance its bid (or, subsidise the acquisition of new technology) in order to prevent entry.

To see this, one has to solve the inequality \(\pi _{L}^{M}>\pi _{H}^{I}+\pi _{L}^{E}\) to ascertain that it is satisfied by all

$$\begin{aligned} c_{L}\in \left( 0,\min \left\{ c_{H},\overline{c}_{L}\right\} \right); \quad \overline{c}_{L}\equiv \frac{\sqrt{4c_{H}\left( 9c_{H}+13\right) +17} -2c_{H}-3}{8\left( 1+c_{H}\right) } \end{aligned}$$
(20)

and this is region A in Fig. 2. In region B,  if the incumbent’s bid relies on the profits stemming from the new technology only, the entrant acquires the patent and a duopoly obtains, because in that portion of the parameter space the new technology is only slightly more efficient than the old one. In other words, region B identifies the parameter constellation in which the incumbent must follow the prescription deriving from Jensen’s inequality, relying on both technologies to ensure the persistence of monopoly. By the way, this reinforces the result spelled out in Lemma 2 concerning the incumbent’s incentive to use both technologies systematically.

Fig. 2
figure 2

The incumbent’s incentives

Full size image

The foregoing analysis, which hinges upon Lemmata 2–3, delivers the following result:

Proposition 4

Under convex production costs and process innovation, the monopolist can always pre-empt entry. However, to do so, it may need to subsidise the acquisition of the patent by using a portion of the profits engendered by the old technology, which will never be discarded.

We may close this section with a few words to summarise the main finding: if marginal cost is increasing, the adoption of the new technology in isolation may not be conducive to the persistence of monopoly, which is instead warranted by the fact that for the incumbent it is optimal to use both technologies, and doing so the incumbent surely outbids the potential entrant.

4 Concluding remarks

We have revisited the debate on the persistence of monopoly originated by the pioneering contributions of Gilbert and Newbery (1982) and Reinganum (1983). Our findings can be summarised as follows. In the case of product innovation, the presence of diseconomies of scope in combination with product differentiation may give rise to (i) entry and (ii) persistence of monopoly with sleeping patents, a result which could not emerge from Gilbert and Newbery (1982) as they considered imperfect product substitutability without including the possible impact of scope economies/diseconomies into the picture. If instead firms bid for a process innovation and production costs are convex, the innovating incumbent always finds it profitable to use both technologies, and indeed may use a portion of the profits generated by the old technology to outbid the entrant and maintain monopoly power. This reveals that Reinganum’s (1983) assumption of constant returns to scale (coupled with the presence of uncertainty) is not sufficiently general, as a deterministic innovation reducing the slope of the production cost function may actually deliver a landscape in which the incumbent’s incentive to bid is systematically higher than the entrant’s.

The framework proposed in this paper can be extended along several avenues. An obvious one is to investigate the entry process in the case of product innovation, to understand whether there exists a limit structure of the oligopoly equilibrium, when the initial monopoly does not persists. Another, and wider extension would be to adapt both frameworks to discrete choice models describing horizontal and vertical differentiation in which the choice of products’ locations in the relevant space of characteristics is part of the firms’ strategy set.