Skip to main content
Springer Nature Link
Log in

Bell Non-Locality in Many-Body Quantum Systems with Exponential Decay of Correlations

  • Condensed Matter
  • Published:
Brazilian Journal of Physics Aims and scope Submit manuscript

A Correction to this article was published on 11 January 2022

This article has been updated

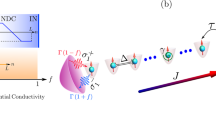
Abstract

Using Bell inequalities as a tool to explore non-classical physical behaviours, in this paper we analyse what one can expect to find in many-body quantum physics. Concretely, framing the usual correlation scenarios as a concrete spin lattice, we want to know whether or not it is possible to violate a Bell inequality restricted to this scenario. Using clustering theorems, we are able to show that a large family of quantum many-body systems behave almost-locally, violating Bell inequalities (if so) only by a non-significant amount. We also provide examples, explain some of our assumptions via counter-examples and present all the proofs for our results. We hope the paper is self-contained.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3

Similar content being viewed by others

Explore related subjects

Discover the latest articles and news from researchers in related subjects, suggested using machine learning.

Data Availability

All the necessary data and material are included in the main text.

Change history

References

  1. J.S. Bell, On the einstein podolsky rosen paradox. Physics Physique Fizika 1(3), 195–200 (1964)

  2. A. Fine, Hidden variables, joint probability, and the bell inequalities. Phys. Rev. Lett. 48, 291–295 (1982)

    Article  ADS  MathSciNet  Google Scholar 

  3. N. Brunner, D. Cavalcanti, S. Pironio, V. Scarani, S. Wehner, Bell nonlocality. Rev. Mod. Phys. 86, 419–478 (2014)

    Article  ADS  Google Scholar 

  4. J.F. Clauser, M.A. Horne, A. Shimony, R.A. Holt, Proposed experiment to test local hidden-variable theories. Phys. Rev. Lett. 23, 880–884 (1969)

    Article  ADS  Google Scholar 

  5. F. Altintas, R. Eryigit, Correlation and nonlocality measures as indicators of quantum phase transitions in several critical systems. Ann. Phys. (New York) 327, 12 (2012)

  6. J. Batle, M. Casas, Nonlocality and entanglement in the xy model. Phys. Rev. A 82, 062101 (2010)

  7. J. Batle, M. Casas, Nonlocality and entanglement in qubit systems. J. Phys. A: Math. Theor. 44(44), 445304 (2011)

  8. S. Campbell, M. Paternostro, Multipartite nonlocality in a thermalized Ising spin chain. Phys. Rev. A 82(4), 042324 (2010)

  9. T.R. de Oliveira, A. Saguia, M.S. Sarandy, Nonviolation of bell’s inequality in translation invariant systems. EPL (Europhysics Letters) 100(6), 60004 (2012)

  10. D.L. Deng, C. Wu, J.L. Chen, S.J. Gu, S. Yu, C.H. Oh, Bell nonlocality in conventional and topological quantum phase transitions. Phys. Rev. A 86, 032305 (2012)

  11. J.C. Getelina, T.R. de Oliveira, J.A. Hoyos, Violation of the bell inequality in quantum critical random spin-1/2 chains. Physics Letters A 382(39), 2799–2804 (2018)

    Article  ADS  MathSciNet  Google Scholar 

  12. L. Justino, T.R. de Oliveira, Bell inequalities and entanglement at quantum phase transitions in the XXZ model. Phys. Rev. A 85, 052128 (2012)

  13. E. Oudot, J.D. Bancal, P. Sekatski, N. Sangouard, Bipartite nonlocality with a many-body system. New J. Phys. 21(10), 103043 (2019)

  14. Z. Sun, Y. Wu, H. Huang, B. Wang, Bell inequality and nonlocality in an exactly soluble two-dimensional ising–heisenberg spin systems. Solid State Commun. 185, 30–34 (2014)

  15. Z.-Y. Sun, X. Guo, M. Wang, Multipartite quantum nonlocality in two-dimensional transverse-field ising models on n x n square lattices. The European Physical Journal B 92(4), 75 (2019)

    Article  ADS  MathSciNet  Google Scholar 

  16. Z.Y. Sun, M. Wang, Y.Y. Wu, B. Guo, Multipartite nonlocality and boundary conditions in one-dimensional spin chains. Phys. Rev. A 99(4), 042323 (2019)

  17. Z. Wang, S. Singh, M. Navascués, Entanglement and nonlocality in infinite 1d systems. Phys. Rev. Lett. 118(23), 230401 (2017)

  18. G.D. Chiara, A. Sanpera, Genuine quantum correlations in quantum many-body systems: a review of recent progress. Rep. Prog. Phys. 81(7), 074002 (2018)

  19. L. Babai, L. Fortnow, C. Lund, Non-deterministic exponential time has two-prover interactive protocols. Comput. Complex. 1(1), 3–40 (1991)

    Article  Google Scholar 

  20. M. Fadel, J. Tura, Bounding the set of classical correlations of a many-body system. Phys. Rev. Lett. 119, 230402 (2017)

  21. I. Frérot, T. Roscilde, Detecting many-body bell nonlocality by solving ising models. Phys. Rev. Lett. 126, 140504 (2021)

  22. A. Piga, A. Aloy, M. Lewenstein, I. Frérot, Bell correlations at ising quantum critical points. Phys. Rev. Lett. 123(17), 170604 (2019)

  23. J. Tura, R. Augusiak, A. Sainz, B. Lucke, C. Klempt, M. Lewenstein, A. Acin,. Nonlocality in many-body quantum systems detected with two-body correlators. Ann. Phys. 362, 370–423 (2015)

  24. J. Tura, R. Augusiak, A.B. Sainz, T. Vertesi, M. Lewenstein, A. Acin, Detecting nonlocality in many-body quantum states. Science 344(6189), 1256–1258 (2014)

    Article  ADS  Google Scholar 

  25. J. Tura, A.B. Sainz, T. Vértesi, A. Acín, M. Lewenstein, R. Augusiak, Translationally invariant multipartite bell inequalities involving only two-body correlators. J. Phys. A: Math. Theor. 47(42), 424024 (2014)

  26. Z. Wang, S. Singh, M. Navascués, Entanglement and nonlocality in infinite 1d systems. Phys. Rev. Lett. 118, 230401 (2017)

  27. J. Tura, G. De las Cuevas, R. Augusiak, M. Lewenstein, A. Acín, J.I. Cirac, Energy as a detector of nonlocality of many-body spin systems. Phys. Rev. X 7, 021005 (2017)

  28. R. Schmied, J.D. Bancal, B. Allard, M. Fadel, V. Scarani, P. Treutlein, N. Sangouard, Bell correlations in a bose-einstein condensate. Science 352(6284), 441–444 (2016)

  29. N.J. Engelsen, R. Krishnakumar, O. Hosten, M.A. Kasevich, Bell correlations in spin-squeezed states of 500000 atoms. Phys. Rev. Lett. 118, 140401 (2017)

  30. B.S. Tsirelson, Quantum generalizations of bell’s inequality. Letters in Mathematical Physics 4(2), 93–100 (1980)

    Article  ADS  MathSciNet  Google Scholar 

  31. A.F. Ducuara, P. Skrzypczyk, Operational interpretation of weight-based resource quantifiers in convex quantum resource theories. Phys. Rev. Lett. 125(11), 110401 (2020)

  32. M.B. Hastings, T. Koma, Spectral gap and exponential decay of correlations. Communications in Mathematical Physics 265(3), 781–804 (2006)

    Article  ADS  MathSciNet  Google Scholar 

  33. M. Kliesch, C. Gogolin, M.J. Kastoryano, A. Riera, J. Eisert, Locality of temperature. Phys. Rev. X 4, 031019 (2014)

  34. B. Nachtergaele, R. Sims, Lieb-robinson bounds and the exponential clustering theorem. Communications in Mathematical Physics 265(1), 119–130 (2006)

    Article  ADS  MathSciNet  Google Scholar 

  35. K. Fredenhagen, A remark on the cluster theorem. Communications in Mathematical Physics 97(3), 461–463 (1985)

    Article  ADS  MathSciNet  Google Scholar 

  36. E.H. Lieb, D.W. Robinson, The finite group velocity of quantum spin systems. Comm. Math. Phys. 28(3), 251–257 (1972)

    Article  ADS  MathSciNet  Google Scholar 

  37. E.G. Cavalcanti, R. Lal, On modifications of reichenbach’s principle of common cause in light of bell’s theorem. J. Phys. A Math. Theor. 47(42), 424018 (2014)

  38. A. Schrijver, Combinatorial Optimization: Polyhedra and Efficiency (Springer-Verlag, Algorithms and Combinatorics, 2004)

    MATH  Google Scholar 

  39. B. Nachtergaele, Y. Ogata, R. Sims, Propagation of correlations in quantum lattice systems. Journal of Statistical Physics 124(1), 1–13 (2006)

    Article  ADS  MathSciNet  Google Scholar 

  40. H. Wilming, M. Goihl, I. Roth, J. Eisert, Entanglement-ergodic quantum systems equilibrate exponentially well. Phys. Rev. Lett. 123(20), 200604 (2019)

  41. R.F. Werner, M.M. Wolf, Bell inequalities and entanglement. Quantum Inf. Comput. 1(3), 1–25 (2001)

    MathSciNet  MATH  Google Scholar 

  42. M. Junge, C. Palazuelos, Large Violation of Bell Inequalities with Low Entanglement. Communications in Mathematical Physics 306(3), 695–746 (2011)

    Article  ADS  MathSciNet  Google Scholar 

  43. G. Vallone, G. Lima, E.S. Gomez, G. Canas, J.A. Larsson, P. Mataloni, A. Cabello, Bell scenarios in which nonlocality and entanglement are inversely related. Phys. Rev. A 89(1), 012102 (2014)

  44. M.J. Kastoryano, J. Eisert, Rapid mixing implies exponential decay of correlations. J. Math. Phys. 54(10), 102201 (2013)

  45. D. Poulin, Lieb-Robinson bound and locality for general markovian quantum dynamics. Phys. Rev. Lett. 104(19), 190401 (2010)

Download references

Acknowledgements

Many thanks to R. Rabelo for all the stimulating discussion.

Funding

This paper is a result of the Brazilian National Institute of Science and Technology on Quantum Information. This work is supported by the Brazilian agencies Conselho Nacional de Desenvolvimento Científico e Tecnológico, Coordenação de Aperfeiçoamento de Pessoal de Nível Superior, FAEPEX, and by the National Research, Development and Innovation Office of Hungary (NKFIH) through the Quantum Information National Laboratory of Hungary and through the grant FK 135220. This project/research was supported by grant number FQXi-RFP-IPW-1905 from the Foundational Questions Institute and Fetzer Franklin Fund, a donor advised fund of Silicon Valley Community Foundation. CD was supported by a fellowship from the Grand Challenges Initiative at Chapman University.

Author information

Authors and Affiliations

  1. Departamento de Matemática Aplicada, Instituto de Matemática, Estatística e Computação Cientıfica, Universidade Estadual de Campinas, 13083-859, Campinas, São Paulo, Brazil

    Carlos H. S. Vieira & Marcelo Terra Cunha

  2. School of Physics and Astronomy, University of Leeds, LS2 9JT, Leeds, Brazil

    Cristhiano Duarte

  3. International Institute of Physics, Federal University of Rio Grande do Norte, 59070-405, Natal, Brazil

    Cristhiano Duarte

  4. Wigner Research Centre for Physics, H-1121, Budapest, Hungary

    Cristhiano Duarte

  5. Departamento de Matemática, Instituto de Ciências Exatas, Universidade Federal de Minas Gerais, 30123-970, Minas Gerais, Belo Horizonte, Brazil

    Raphael C. Drumond

Authors
  1. Carlos H. S. Vieira
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Cristhiano Duarte
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Raphael C. Drumond
    View author publications

    You can also search for this author inPubMed Google Scholar

  4. Marcelo Terra Cunha
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Carlos H. S. Vieira.

Ethics declarations

Conflict of Interest/Competing Interests

The authors declare that there is no conflict of interest whatsoever with the publication and in the elaboration of this work.

Additional information

Publisher’s Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

The original online version of this article was revised: The copyright holder was corrected.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Vieira, C.H.S., Duarte, C., Drumond, R.C. et al. Bell Non-Locality in Many-Body Quantum Systems with Exponential Decay of Correlations. Braz J Phys 51, 1603–1616 (2021). https://doi.org/10.1007/s13538-021-00998-1

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s13538-021-00998-1

Keywords