Abstract
Although mycorrhiza has been identified to be of major importance for plant nutrition and ecosystem stability, existing C- and N- simulation models on the ecosystem scale do not explicitly consider the feedbacks between ectomycorrhizal fungi and plants. We present a simple dynamic feedback model which allows estimating the main C- and N- flows between ectomycorrhizal fungi and tree roots in order to test the sensitivity of the system fungus-tree to environmental parameters and to assess the fungal contribution to plant N nutrition. Sensitivity tests carried out showed that the model responses to variations of model parameters, particularly with regard to N availability, are in agreement with published results from field and laboratory studies. However, there are still some processes and parameters which are not well constrained. Fungal N uptake rates and the ratio between mycelium, hartig net, and mantle biomass are parameters which significantly affect model results but for which published data are scarce or missing. Nevertheless, the model is already providing a platform to test our understanding of the importance of mycorrhiza for forest stand nutrition. Future coupling to a mechanistic ecosystem model will allow simulating the importance of mycorrhization for e.g. stand growth and C and N retention.
Similar content being viewed by others
Avoid common mistakes on your manuscript.
Introduction
Mycorrhiza and ecosystem C- and N-cycling
The significance of mycorrhizal symbiosises for plant nutrition and ecosystem C- and N- turnover has been acknowledged in numerous studies on ecto- and arbuscular mycorrhizal associations (EM, AM) (e.g. Marschner and Dell 1994; Smith and Read 1997). Ectomycorrhizal fungi form mutualistic relationships with trees in order to receive carbohydrates from the plants and in return supply the plant with important nutrients. Fungi receive between 1–25% of plant photoassimilates (for details see: AM: Jakobsen and Rosendahl 1990; Staddon et al. 2003; EM: Ek 1997; Hobbie 2006; Leake 2007) and constitute the major pathway for soil carbon sequestration (Godbold et al. 2006). AM associations are typical for grassland ecosystems, but are scarce in boreal and temperate forests. Therefore, AM associations are not in the focus of this study.
EM fungi cover their carbon demand mainly from host assimilates. However, they can also exist in separation from their host trees and gain carbon by heterotrophic decomposition of plant litter and soil organic matter (Nehls 2008; Treseder et al. 2007; Malcolm et al. 2008). Genome analysis revealed that almost the whole suite of genes for saprophytic enzymes was present in Laccaria bicolor, a typical EM fungus of the basidiomycetes (Martin et al. 2008). However, currently evidence is lacking that EM fungi feed on litter when functioning in a well established symbiosis. Therefore, it can be assumed that the carbon demand of EM in a mutualistic relationship is almost exclusively covered by the host.
The plant benefits from mycorrhizal colonization by an improved vitality since the fungi supplies the plant with important micro- and macronutrients. Mycorrhiza uptake and transfer of inorganic nutrients to the plant has been shown for NH +4 , NO -3 , P, K, Ca, SO4, Cu, Fe, and Zn (Marschner and Dell 1994; Ek 1997; Ames et al. 1983). In addition, EM can take up organic nitrogen in form of amino acids from the soil (Martin et al. 1988). Mycorrhizal fungi have been estimated to contribute about 80% of plant P as well as 80% of plant N uptake (van der Heijden et al. 2008). EM fungi are particularly important for N nutrition in temperate and boreal forests, where N is a limiting resource (Read and Perez-Moreno 2003).
Mycorrhiza is assumed to be an adaptation to nutrient-poor conditions (Read 1991), evolved to improve nutrient transfer to the plant. For this purpose, EM fungi ensheath the root tip by a thick mantle-like structure (hyphal mantle). The function of the hyphal mantle is believed to be that of a physical barrier (Taylor and Peterson 2005) and of a store for carbohydrates and lipids (Jordy et al. 1998; Laczko et al. 2003). Outside the mantle thin hyphae emanate into the soil, where they can explore pores which are inaccessible to roots. Furthermore, the hyphae exude enzymes which degrade P- and N-containing polymers and thus, make these resources available to plants (Pritsch et al. 2004; Finlay 2008). The hyphae of some EM species also form thick, long cords (rhizomorphs) enabling long-distance transfer of nutrients. According to the distance from which soil nutrients can be gathered, different mycorrhiza exploration types have been distinguished (Agerer 2001). Altogether, these structures outside the mantle are called extra-radical mycelium (ERM). The ERM substantially increases the surface for nutrient and water uptake compared with that of roots and, therefore, is highly beneficial for the plant (Read and Perez-Moreno 2003). Inside the mantle, at the root surface, the fungal hyphae penetrate the cell wall and grow between but not inside the cells. Thereby, they form a large interface with root cells for nutrient exchange. This structure inside the plant is called “hartig net”.
EM fungi have important roles in soil C and N sequestration and cycling. The inorganic N uptake rates of many mycorrhizal fungi species are measured to be more than 10-fold higher than root uptake rates (Plassard et al. 1991). Baath and Söderström (1979) estimated the N stored in the fungal extraradical mycelium (ERM) to make up 20% of total soil N in a given horizon. Altogether, the significance of mycorrhiza for ecosystem nutrient cycling is currently fully acknowledged but still barely understood.
Despite their importance, mycorrhizas are hardly considered in ecosystem models which are used for simulating C- and N- cycling and plant growth. The scarcity of data about explicit allocation rates of C and N between the symbiotic partners makes model implementation of mycorrhiza very difficult. There is a range of mathematical models describing fungal or hyphal growth and distribution in the soil matrix. Halley et al. (1994) presented a dynamic cellular automation model which describes the dynamics of a colony by the propagation of an individual cell, providing a better understanding of community population dynamics. Other mycelium growth models simulate growth as a function of nutrient resources. Boswell et al. (2003) focussed on the growth of saprophytic hyphae and the interaction with their environment. They describe nutrient transport in the ERM and exchange with its heterotrophic environment as a function of hyphal density, inactive hyphal density, and hyphal tip density. A model applicable to simulate mycorrhizal symbiosis was developed by Neuhauser and Fargione (2004) and is based on the classical Lotka-Volterra approach. They considered symbiosis between a host and a fungus as a mutualism-parasitism model and focused on modelling the symbionts’ behaviour. However, nutrient turnover was not considered and mycorrhiza was regarded as a system which turns from mutualism to parasitism when soil fertility rises. To our knowledge, the only models dealing with the importance of mycorrhiza for plant nutrition were developed by Jolicouer et al. (2002), Schnepf and Roose (2006), Schnepf et al. (2008), and Deressa and Schenk (2008). Jolicoeur et al. (2002) described a growth behaviour model for root dry mass and fungal spore number driven by the regulation of the nutritional status of the symbiont using internal phosphate and sugar concentrations as regulating key nutrients. The first model to describe fungal contribution to plant nutrition was developed by Schnepf and Roose (2006). On the basis of a single root model for simulating solute uptake they developed a model for hyphal solute uptake by adding a volumetric sink term representing uptake by extraradical hyphae. Schnepf et al. (2008) presented a different model for arbuscular mycorrhiza by describing growth and distribution of fungal ERM by developmental processes like branching and anastomosis. In both models they focused on modelling fungal contribution to plant phosphate nutrition and described distribution of mycelia in soil explicitly. The model of Deressa and Schenk (2008) also focused only on P nutrition mediated by AM associations.
Fungal growth and nutrient flows between fungal hyphae and the neighbouring environment have been described explicitly in the above mentioned models. Still, no model exists whose main focus is on plant and fungal interaction describing C- and N- exchange and allocation routines. Such a model would allow to estimate fungal contribution to ecosystem C- and N- cycling. We therefore present a simple feedback model which captures the main allocation routines of C and N between a tree root and the fungal network. The model was used to test the sensitivity of the mycorrhizal symbiosis to environmental parameters such as variation in temperature, carbon availability, and nitrogen supply. In future, it can be linked to ecosystem or plant physiology models to allow site specific simulation of mycorrhizal growth and thus give an estimation of the contribution of this important ecosystem component to nutrient cycling.
Factors influencing mycorrhizal growth – a basis for model development
Developing a mycorrhiza feedback model requires consideration of the impact of changes in environmental parameters or plant growth on mycorrhiza development. Environmental parameters can have direct or indirect effects of fungal metabolism, e.g. direct temperature effects on respiration or indirect effects via plant metabolism, following e.g. changes in photosynthesis or plant C allocation (Table 1). Increased temperature in general leads to a higher C allocation from the photosynthetically active plant parts to the roots and therefore to higher C transfer to the fungus. The fungus can use the C resources for biomass production or N assimilation. Increased fungal biomass as a consequence of higher temperature has been reported for AM and EM by Gavito et al. (2005), Hawkes et al. (2008), and Heinemeyer et al. (2006). Under these conditions higher root colonization and hyphal lengths have also been found (Heinemeyer and Fitter 2004). In addition to these indirect effects, direct effects on fungal metabolism have been shown. Baath and Wallander (2003), Hawkes et al. (2008), and Malcom et al. (2008) reported increased respiration of EM as a response to increased temperatures. However, some species show a fast temperature acclimation and reduce respiration after days or weeks (Heinemeyer et al. 2007; Malcolm et al. 2008). In consequence, this would lead to a C accumulation in hyphae when C is still supplied at higher rate to the fungus. Malcolm et al. (2008) tested 12 isolated mycorrhiza fungi for their temperature response. Only three of them clearly acclimated to temperature, i.e. showed no increased metabolism with increasing temperature. This is in agreement with studies by Moyano et al. (2007) and Heinemeyer et al. (2007), who observed a temperature insensitivity of mycorrhizal respiration in AM and EM communities, respectively.
With respect to the impact of soil moisture on the growth and vitality of mycorrhiza contradictory results have been reported. Boone et al. (1998) and Moyano et al. (2007) found the response to humidity to be very small or non-existent, whereas Heinemeyer et al. (2007) found that EM fungi were more sensitive to soil moisture than to temperature changes.
A clear correlation between pH and EM biomass has been detected (Högberg et al. 2003; Blagodatskaya and Anderson 1998). Marx (1990) showed that fungal growth was reduced by more than 50% when the pH was increased from 4.8 to 6.8. Högberg et al. (2003) could attribute a decrease of the fungal marker PLFA 18:2w6.9 by 10 mol% with rising pH from 3.8 to 6.5 to a decrease of the ectomycorrhizal community by means of a girdling experiment (Högberg and Högberg 2002). Despite some contradictory results (Baath and Wallander 2003), soils with pH values <5.5 generally have higher ectomycorrhizal biomass than soils with neutral pH values.
Despite many studies, the relation between mycorrhiza and elevated atmospheric CO2 concentrations is still unclear. Staddon et al. (2002) stated that there were no effects other than plant mediated ones, meaning that higher CO2 assimilation by plants resulted in higher belowground C allocation and, thus, a proportional increase in fungal growth (see e.g. Gavito et al. 2000 Godbold et al. 1997; Wiemken et al. 2001). This view is also supported from earlier studies by Lewis et al. (1994) and Runion et al. (1994). However, this may not always be true because growth under elevated CO2 increased N uptake but under certain conditions also N use efficiency (Finzi et al. 2007). Nevertheless, increases in ambient CO2 in general increase mycorrhizal growth (Treseder 2004).
N availability is one important factor regulating mycorrhizal colonization (Table 2). A higher N availability, e.g. as a consequence of increased atmospheric N deposition, has been found to decrease growth of mycorrhiza (Wallenda and Kottke 1998), whereas low amounts of N can cause a stimulating effect (Reid et al. 1983; Wallander and Nylund. 1992; Gorisson et al. 1993). High amounts of N led to a significant decrease in fungal biomass (Wallander and Nylund 1991; Wallander and Nylund 1992; Wallander 1995), a decrease in mycelia production (Arnebrandt 1994; Nilsson 2003; Nilsson 2007), and mycorrhizal frequency (Richards 1965; Reid et al. 1983; Beckjord 1985; Gorisson et al. 1993; Treseder 2004). Most studies are laboratory experiments, while only few were carried out in the field but also these revealed an average reduction of mycorrhization of 15% with N fertilization (Treseder 2004). The effects of increased N levels on plant-fungal interactions are still not completely clarified. An early explanation from Björkmann (Björkmann, 1942) was that the host plant would allocate less C to its fungal partner due to an increased demand of C for its own shoot growth caused by higher N uptake. However, Wallander (1995) and Wallander and Nylund (1991) found an increase in root C pools during high levels of N availability although fungal production was decreased. Wallander (1995) concluded that with increasing N availability in soil the fungus cannot avoid taking up available N and, thus, a large part of available C is used for N assimilation and amino acid production, whereas less C is used for growth. This view is supported by experiments form Martin et al. (1988) who demonstrated that around 38% of applied Glucose enter the amino acid pool which identifies N assimilation into amino acids as an important C sink.
Objectives
In this paper we describe the development of a simple dynamic feedback model simulating uptake and allocation of C and N between ectomycorrhizas and tree roots. Arbuscular mycorrhiza is explicitly not considered here. The aim of this model is to explore the dynamics of ectomycorrhiza abundance and its importance for forest C- and N- turnover under changing environmental conditions. The main emphasis is to capture principal exchange mechanisms of C and N between fungi and tree roots and to analyze possible feedback mechanisms. The focus of the model applications is on the exploration of the sensitivity of the fungus-tree-relationship to changes in environmental conditions such as changes in N availability. We will not consider mycorrhiza diversity on the community level nor differentiate exchange of different N-forms between roots and fungus. Also, interactions with the microbial community in the soil are not considered at this stage. Since experimental results are not conclusive or can not be quantified, we are not able to consider all environmental factors which possibly affect mycorrhiza in our model development. Therefore, we focus on changes in N availability and temperature on EM-root interactions of C- and N- exchange. In the future, our model can also be linked to forest-ecosystem and/or plant physiology models in order to allow site-specific simulations of mycorrhizal growth and to assess its importance for forest C- and N cycling. Thereby, predictions about the importance of mycorrhiza for forest development under changing environmental conditions can be obtained.
Material and methods
Model description
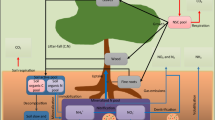
Principal mechanisms and nutrient flows are displayed in Fig. 1. For model development and parameter estimation we use the software VENSIM@PLE 2006. The simulation model VENSIM@PLE offers different solution methods for the resulting systems of differential-algebraic-equations based on explicit integration schemes like the Euler method or the Runge-Kutta method. All methods take advantage of adaptive time step sizes in order to maintain stability when dealing with stiff equations systems. All available numerical methods have been evaluated for our system in order to ensure consistent integration of the obtained equation system.
The model’s emphasis is on the simulation of EM, even though we sometimes use results obtained for AM parameterization. In these cases no reports were available for EM. The model describes the dynamics of four compartments: root carbon and nitrogen pools and fungi carbon and nitrogen pools. Prescribed plant physiological and environmental conditions are:
-
1.
Plant photosynthesis is prescribed dynamically:
For model development we followed a simplified approach and have not coupled the mycorrhiza model to a plant physiology model, yet. Therefore, in our approach we assume that plant assimilates CO2 at maximum a rate of 0.01 kg-C m-2d-1. Plant assimilation is modified by the seasonality of temperature and light, which in the model is expressed as the annual factor (Fig. 2) (Granier et al. 2000 Verbeeck et al. 2008). The relationship is based on gross primary production data for beech forests from Verbeeck et al. (2008).
-
2.
Total nitrogen availability in soil is assumed to be constant. Typical soil inorganic and organic N concentrations were taken from observations of Dannenmann et al. (2006) for a beech site in Tuttlingen, South Germany.
Carbon allocation of plant and fungus and C release by respiration and turnover
An overview about parameters and their sources is given in Table 3 and in Table 5 in the appendix. The C as originating from photosynthesis (PS) and allocated to roots is the only C source for roots and fungi. We are aware that some EM species are able to cover a certain amount of their C demand by uptake from decomposition of organic matter, too (Langley and Hungate 2003; Treseder et al. 2007). However, this is regarded a minor contribution, which is probably insignificant when the fungus exists in symbiosis. Moreover, there are hardly data available which quantify fungal C uptake due to decomposition of organic matter. Therefore, this component was not considered in the model.
Carbohydrates feed root growth and maintenance including storage. They are allocated from the shoot to the root and account for a constant fraction (froot) of the C assimilated by photosynthesis. Root C content therefore is calculated by:
-
Cr = froot PS
-
[Cr = C allocation to root and mycorrhiza, Crto = C loss by turnover, Crresp = C loss by respiration, Callo = C allocation to the fungus]
Since C storage in roots is between 15 and 90 mg grootDW-1 (A. Polle, unpublished data) we assumed C storage (Cst) to be 3.33% of root C. Root C losses are due to respiration, allocation to the fungus, and root turnover. Furthermore, root C losses may arise from exudation. But since exudation is not considered in the context of model development it is not further discussed here. Respiration (Crresp) depends on temperature and is calculated after Thornley and Cannell (2000), who distinguish between residual-, growth-, and N-uptake respiration (Appendix). The temperature optimum for residual respiration is 15°C. Root turnover (Crto) is calculated based on root biomass (Croot) and a specific death rate which depends on mycorrhizal colonisation. Non-mycorrhizal roots have a turnover (dr_r) of 365 days (Bauhus and Bartsch 1996), although much slower decomposition rates have also been reported (Matamala et al. 2003). Mycorrhizal colonisation decreases the death rate of roots. Therefore, we assumed a turnover of mycorrhized roots (dr_m) of 625 days (Langley et al. 2006).
The mycorrhization degree (m) is the average fraction of the fine root tip biomass covered by the fungal mantle (Smith and Read 1997). Root C turnover is then calculated as:
The left part of the equation describes turnover of non-covered roots: the turnover rate dr_r affects the part of roots which are not mycorrhized and are described by the term “(1- m)· Croot”. The right part of the equation describes turnover of root tips which are mycorrhized (m). Therefore dr_m affects the fraction of root tips which are mycorrhized and are described by the term “m ·Croot”.
The fungal C content is dependent on C transfer from the root and C losses due to respiration and turnover. Therefore fungal C is calculated by:
-
[Cfung = fungal C content, Callo = C transfer from root to fungus, Cfresp = fungal respiration, Cfto = fungal turnover]
The calculation of C transfer from root to fungus is calculated by means of an optimum ratio between fungal and root biomass (CfCropt) and is based on the assumption that a certain amount of root biomass requires a certain amount of fungal biomass in order to form an optimum mycorrhization. We acknowledge that this assumption is a strong simplification because biomass fractionation between mantle, ERM, and root biomass is highly species and site dependent. The transfer of C from root to the fungus covers total fungal C demand as long as transfer does not exceed a certain fraction of plant assimilated C (PS) (Jakobsen and Rosendahl 1990; Staddon 2003). Furthermore, C allocation from the roots to the fungus depends on fungal (Cfung) and root (Croot) C content and is then calculated as:
This minimum function denotes that total fungal C demand (right side) is satisfied as long as the fungal C demand does not exceed a certain fraction of plant assimilated C (left side). Fact_allomax defines this maximum possible fraction and is set to 20% as long as N availability (Nav) is <0.01 kg-N kg -1soil . Otherwise it is calculated by:
This is based on the assumption that with increasing N concentration in soil the root increases its own N uptake. This will lead to increased root C demand since the available N is converted into amino acids (Wallander 1995). In consequence, less C is available for fungal growth, so that Callo is reduced.
The scaling factor fact_allo in Eq. 4 reduces C allocation if the fungal N transfer (Nallo) to the root is not sufficient to cover 50% of the root N demand:
-
[Nupt_root = total root N uptake, Nallo = N allocation from fungus to root]
This equation directly links fungal C demand to root N demand and represents the assumption that the plant will only cover fungal C demand as long as the plant is supplied with a significant amount of N (Nehls 2008). Eq. 6 is based on the suggestion of Nehls (2008) who stated that the plant is able to control the activation status of certain transporters regulating C transfer to the fungus as a reaction to the amount of nutrients which is provided by the fungus.
C allocation from root to fungus (Callo) is directly linked to plant photosynthetic rate and, thus, decreases to zero during wintertime when photosynthesis stops. To avoid death of mycorrhiza during winter, a minimum C allocation (Cmin) is transferred from the root C storage (Cst) at a daily rate of 5% of Cst (see Appendix Table 5).
Fungal C losses are caused by respiration and turnover. There are contrasting reports about the temperature response of fungal and root respiration. On the one hand Heinemeyer et al. (2007) reported that fungal and root respiration differs with regard to temperature changes, whereas on the other hand Baath and Wallander (2003) did not find any significant difference. For simplicity and in view of the scarcity of literature data on this topic we assumed that temperature response of respiration does not differ between the fungus and the root. Fungal respiration was therefore calculated like root respiration and follows Thornley and Cannell (2000) (see Appendix Table 5). C loss by turnover (Cfto) is a linear function assuming different turnover times for mantle and ERM material. ERM turnover ranges between 10 days to 100 days, while mantle turnover time is 625 days (Langley and Hungate 2006). The biomass fractionation between ERM and mantle biomass, which is necessary to describe fungal turnover but also for nutrient uptake, is given by the ratio myc_m. The parameter myc_m describes the fractionation between the three fungal organs ERM, mantle, and hartig net biomass. The hartig net only serves as an interface for root-fungal interactions while the fungal mantle, similar to the ERM, probably also contributes to nutrient uptake. Though, a clear biomass fractionation between the hartig net and the mantle is not possible due to scarcity of data. Therefore, no differentiation is made between hartig net and mantle biomass and both are assumed not to contribute to fungal N uptake. Therefore, in the following myc_m refers to the ratio between ERM and mantle (including hartig net) biomass. Mycorrhization (m) is defined as the ratio between current fungal C content and potential C content which is required to cover all root tips. It is calculated by using the optimum fungal biomass ratio CfCropt:
The ratio between mantle and ERM biomass, myc_m, is assumed to be 0.5 (Wallander 2001; Wallander and Nylund 1992), which is an average value since very different forms of fungal networks are known (Agerer 1987-2002).
N uptake processes and fungal contribution to plant N nutrition
The fungal N reservoir is defined by N gains from fungal N uptake and N losses caused by turnover and allocation to the root:
-
[NH4fupt = fungal NH4 uptake, NO3fupt = fungal NO3 uptake, Norgfupt = fungal uptake of dissolved organic N]
Fungal N gains
Gains of the fungal N reservoir derive from uptake of NH4, NO3, and organic N from the soil, whereas N is lost by turnover and allocation to the root system. N uptake is calculated by:
-
[Nxyfupt = total uptake of each N-form (xy) (kg-Nxy m-2), NxyUPTF = constant uptake rate of each N- form (kg-Nxy kg dw.-1 d-1), xy = NH4/NO3/org.N, myc_m = ratio between ERM and mantle (+ hartig net) biomass]
N uptake of all forms is calculated with a constant, though N-form specific, rate (Table 3) which is modified by N availability (factN, Fig. 3) and a term representing fungal N demand, \( 1 - \frac{{NC_{fung} }}{{NC_{fungopt} }} \). Fungal N demand depends on fungal C and N status and, thus, on the NC ratio. The more the fungal NC content approaches an optimum fungal NC ratio (NCfungopt), the less N is taken up and vice versa.
myc_m, as explained above, is the ratio between ERM and mantle biomass whereas mantle biomass also comprises the hartig net since differentiation between these is not possible. In our model, only the ERM is responsible for N uptake even when uncertainties might occur because N might be also taken up by the fungal mantle. This can not be regarded since data are too scarce to derive uptake routines. Thus, fungal N uptake directly depends on the ratio between ERM and mantle (including hartig net) biomass, myc_m.
The scaling factor factN increases N uptake with increasing N availability in soil reflecting the assumption that fungi increase N uptake effectiveness with increasing N availability (Wallander 1995). Owing to a lack of data which would allow us to develop an explicit N uptake - N availability relationship function, we assume a simple exponential function for factN (Fig. 3).
A differentiation of fungal uptake for different N forms is very difficult since this is highly species and site dependent and published uptake rates differ very much with regard to N forms (Plassard et al. 1991; Chalot et al. 1995; Smith and Read 1997). Some fungal species grow very well on organic N, while others only assimilate inorganic N sources (Read 1991; Smith and Read 1997). In general, fungi seem to prefer NH4 > NO3 > organic N which is considered by respective specific rates (Table 3).
N losses of the fungal network and N allocation to the root system
N loss caused by fungal turnover (Nfto) is proportional to C loss, i.e. we assumed that there is no retranslocation of N in surviving fungal parts (Gordon and Jackson 2000), and is calculated by means of the NC ratio. N losses to the plant (Nallo) are assumed to be transferred as elemental N, independent of the form of fungal N uptake, since the form in which N is transferred to the root is not clear. It could be transported as amino acid, most probably glutamine (Smith and Smith 1990; Carrodus 1966), but loading into root cells involved mainly ammonium transporters (Müller et al. 2007; Nehls 2008) suggesting that NH4 is the preferred form for the plant. Allocation of N from the fungal network to the root is described as:
-
[Nallo = N transfer to the root, Nmax = maximum N allocation , NCrootopt = optimum root NC, NCroot = current root NC]
\( 1 - \frac{{NC_{root} }}{{NC_{rootopt} }} \) is a demand term which is controlled by the plant and is therefore sink-controlled. NCrootopt is a constant reflecting the optimum NC ratio of the plant root. The more the NC ratio of the root approaches NCrootopt, the less N is allocated from the fungus to the root and vice versa. N is allocated from the fungal N reservoir, meaning that N is assimilated by the fungus and in the following transferred to the plant as N. Only little is known about the explicit transfer rates. Owing to lack of data about allocation rates, N allocation rate was estimated from sensitivity analysis by varying the value from 0.1 to 0.9 and using the value which produced the most reasonable results. N allocation to the plant is coupled to the fungal NC ratio which restricts allocation when NC is lower than 0.025 which is a CN ratio of 40.
Root N uptake and loss by turnover and allocation to the shoot system
The root N reservoir is calculated from N gains by root N uptake and fungal N allocation and N losses caused by turnover and allocation of N to upper plant parts. It is derived from:
-
[Nroot = root N content, Nallo = N transfer from fungus, NH4/NO3rupt = root uptake of NH4 and NO3, Nrto = root N turnover, Nshoot = N transfer to the shoot]
In our model, non-mycorrhizal roots take up N as NH4 and NO3. The uptake of organic N sources is regarded as insignificant and is therefore neglected but can be included in the model, if required. The N uptake is demand controlled and described by:
-
[Croot = root C content, NCrootopt = optimum root NC ratio (=0.033), NCroot = current NC ratio, Nallo = N transfer from fungus to root]
factN is the scaling factor already mentioned for fungal N uptake (Fig. 3, Eq. 9). The term “Croot ·(NCoptroot - NCopt) - Nallo” of the equation calculates root N demand reduced by the amount of N already supplied from the fungus, while the factor factN modifies N uptake according to N availability. The maximum root uptake rate Nxyruptmax is restricted to root zones which are not mycorrhized:
-
[Nxyruptmax = total maximum uptake for each N form (kg-Nxy m-2 d-1), NxyUPTR = maximum N uptake rate for each N-form (kg-Nxy kgDW-1d-1), m = relative mycorrhizal colonization, \( \frac{{C_{root} }}{0.45} \)= conversion of root C into root biomass assuming a C content of dry matter of 45%]
The preference of root N uptake for NH4 and NO3 is controlled by the ratio between the uptake rates, respectively.
Root N losses are caused by turnover and allocation to upper plant parts. N loss is proportional to root C loss assuming that there is no N-retranslocation (Gordon and Jackson 2000). Root N losses by turnover are equivalent to C losses and are therefore calculated as:
N allocation to upper plants is dependent on the C available for shoot growth and is furthermore influenced by the root NC ratio. The higher the root NC, the more N is available for shoot growth:
-
[Nshoot = N allocation to shoot, PS = C production by photosynthesis, froot = fraction of C assimilates which is allocated belowground]
Sensitivity analysis
In order to assess the functionality and the structure of the model and to demonstrate its’ general behaviour, we used a detailed sensitivity analysis (Brugnach 2005). The main aspect of this sensitivity analysis is to assess the feedbacks of changes in environmental (soil N availability, temperature) and plant and fungal physiological parameters (e.g. photosynthesis, allocation) on the C and N flow between root and fungus. Sensitivity tests were done in such a way that one factor was varied while the others were kept constant. Results were analyzed with respect to their relative change to a defined baseline scenario. Conditions of the baseline scenario correspond to the conditions of a site in the Swabian Jura, Germany, (8°45’E, 47°59’N), with a mean annual air temperature of 6.6°C and a mean N soil concentration of 0.006 kg-N kg soil-1 (Dannenmann et al. 2006).
Model sensitivity was tested for variations of model drivers like temperature and photosynthesis-rate as well as for model parameters describing the a) share of carbohydrates from gross primary production allocated from the shoot to the root (froot), b) optimum ratio between fungal and root biomass (CfCropt), c) maximum C allocation rate to the fungus (Cmax), d) concentration of N in the soil (Nav), root and fungal N-uptake rates and here the e) maximum N allocation rate from the fungus to the plant root (Nmax), f) ratio between extra-radical mycelium and the mantle (myc_m), g) turnover rate of the fungal mantle covering the root (dr_m), h) turnover rate of the fungal extra-radical mycelium (dr_myc), and rates for i) fungal (Nupt_fung) and j) root (Nupt_root ) N uptake. Simulation runs cover three years and, if not stated otherwise, results are sums of the last year for all parameters except NC ratios and mycorrhization which are averages.
Results
Results of the sensitivity analysis for all model parameters are shown in Table 4. Root and fungal biomass predictions of the baseline scenario are calculated from C content assuming a C content in dry matter of 45%. Simulated maximum biomass values were 0.16 kg DW m-2 for fungal and 0.5 kg DW m-2 for root biomass (<2 mm) (Fig. 4). The fungal contribution to total root N uptake was defined as the fraction of fungal N allocation of the sum of root N uptake and fungal allocation. In our baseline scenario, 99% of total plant N uptake originate from the fungus. Since it is generally assumed that mycorrhiza has a positive feedback on photosynthesis rate, we assumed an increase in the photosynthetic activity of +50% and +100% in the framework of the sensitivity analysis. The increase in values of fungal and root C content (Cfung and Croot ) and root and fungal respiration was directly proportional to the increase in photosynthesis rate, i.e. 50% and 100%, respectively. The same applies for root and fungal N uptake rates, since photosynthesis influences root and fungal biomass directly proportional and therefore dependent parameters also increase directly proportional (Table 4). The increase in photosynthesis rate did affect neither the NC ratio of the fungus nor the root.
The influence of a variation in the fraction of plant assimilated C allocated to the roots (froot) on output parameters is not directly proportional to changes in photosynthesis rate. froot directly affects root N transfer to the shoot, since higher C allocation from the shoot to the root will result in a lower N transfer from the root to the shoot (Eq. 15). By that, root N content and therefore N allocation from the fungus are affected and consequently fungal N uptake and fungal respiration will change. On the other hand, the decreased N transfer from the root to the shoot following increased C allocation is transient until a new equilibrium is reached, since a higher C allocation increases root biomass and root N demand (Table 4).
While the variation of the C allocation belowground (froott) directly affects available C for root and fungus, variations in the C root to fungus allocation rate (Cmax) or in the optimum ratio between fungal and root biomass (CfCropt) have only indirect effects on root C but direct effects on fungal output parameters such as biomass (Table 4). With a 50% decrease in CfCropt, fungal C decreases by about 22% while a 50% increase in CfCrop only leads to a C gain of 10% (Table 4, Fig. 5).
One of the major factors controlling C and N allocation between fungus and plant is the soil N concentration Nav. The effects of changes in Nav were tested for -50 to +500% (Fig. 6). In general, at higher soil N concentrations the importance of root N uptake increases, whereas the importance of fungal N uptake for plant N nutrition decreases. An increase in soil N concentration compared to the baseline value by 200% is followed by a decline in C allocation from the plant to the fungus by 65% which causes a significant reduction in fungal C by 66% (Fig. 6). Under increased soil N availability, the root covers a higher percentage of its N demand by own N uptake, so that the dependence on fungal N supply decreases. Furthermore, the root also uses its C assimilates for its own N assimilation and conversion into amino acids rather than for supplying the fungus with C. Consequently, C allocation to the fungus declines. This mechanism in our model is driven by the factor fact_allomax which affects C allocation if soil N concentration is higher than 0.01 kg-N kg -1soil .
Simulated seasonal variations in root and fungal respiration (kg-C m-2 d-1). The sharp decrease in fungal respiration around day 135 is due to a step-decrease in the fungal NC ratio which lowers C demand. This decrease in the fungal NC ratio is caused by a loss in fungal N which is due to the onset of fungal N transfer to the root, which is driven by the onset of plant growth at the start of the vegetation period
The decrease in fungal C supply and consequently the decrease in biomass for the +200% scenario results in (i) a reduction of mycorrhizal colonisation from 84% to 19% and in (ii) a decrease in fungal N uptake and N allocation by about 70% as compared to the baseline scenario. At the same time, fungal contribution to root N nutrition decreases from 99% to 21%. With a further increase in N availability to 400% the fungal biomass declines almost completely. In contrast to fungal N uptake, root N uptake increases some 10-fold under elevated soil N concentrations which corresponds to the reduction of N supplied by fungal allocation. Lowering of soil N concentrations by 50% hardly affects fungal as well as root N uptake in the simulations.
For fungal and root N uptake, constant uptake rates for each N-form were used for root and fungus, respectively. These are maximum potential uptake rates which are lowered by the factors myc_m (only fungal uptake) and factN (root and fungal uptake). N uptake rates were varied in a range of -50% and +50% of the baseline level for each N-form except for fungal and root NH4. Fungal NH4 uptake was varied according to the range published in Plassard et al. (1991), root NH4 uptake was derived from Göransson et al.(2006b), Wallenda (2000), and Carrodus (1966). To avoid that in our simulations the change in the fungal or root NH4 uptake rate is compensated by increased fungal/ root uptake of nitrate or organic N (only fungus), we limited NO3 and organic N uptake to the values of the baseline scenario. The same applies when variations of fungal/root N uptake rates for the other N forms were tested.
Model results are most sensitive to changes in fungal NH4 uptake (Table 4). A decrease of the fungal NH4 uptake rate from the baseline value to 0.008 kg-NH4 kgDW-1 results in a reduction of total fungal N uptake by 49% and a reduction of fungal N allocation to the plant by 48%. The root responds with a 12-fold increase in N uptake which is too ineffective to cover root N demand. Therefore root NC falls by 23%.
An increase in fungal NH4 uptake rate has only little effect on fungal and root growth (Table 4). The reason for this is that fungal N demand is already covered by the baseline value so that total N uptake for the higher NH4 uptake rate is down-regulated by the term \( (1 - \frac{{NC_{fung} }}{{NC_{fungopt} }}) \) (Eq. 9). Changes in the other N-form uptake rates result in a similar pattern but differences of root and fungal C and N to the baseline value at most 3% (Table 4). Fungal contribution to plant N nutrition varies between 71% and 99% for minimum and maximum NH4 uptake rate, respectively. Changes in root N uptake rates have only insignificant effects. A decrease in root NH4 uptake rate by 90% leads to a decrease of total root N uptake by 88%. The reduced plant N uptake is compensated by an increase in fungal N allocation to the root so that root NC as well as fungal and root biomass are not influenced.
The maximum N allocation rate from the fungal ERM to the root system (Nmax) has only little impact on root and fungal biomass. For a 50% higher allocation rate, root and fungal C are altered by less than 1%, the same applies for a 50% lower allocation rate. The reason for this insignificant effect is that fungal contribution to root N is above 98% for the baseline scenario already and increases only little for the +50% scenario. At this high contribution, C allocation between fungus and root is not affected by any changes in N transfer (see Eq. 6). The parameters which are significantly affected are root and fungal N uptake. A 50% reduction of N transfer leads to a 6% lower fungal N uptake since fungus has fewer N losses. The root in contrast needs to increase its N uptake by 7% to compensate the lacking N supply from the fungus. As the root N uptake is less effective than fungal uptake and, moreover, root N uptake is limited by the fungal mantle cover, root NC decreases by 6%. An increase in maximum N allocation shows less severe effects since it is controlled by root N demand. Since root NC is already at its almost optimum at the baseline value, the higher N transfer rate is down-regulated by the term \( 1 - \frac{{{\text{NC}}_{\text{root}} }}{{{\text{NC}}_{\text{rootopt}} }} \) (Eq. 10).
myc_m is the ratio between ERM and mantle (including hartig net) biomass and has strong influence on nutrient uptake and fungal turnover. With a 50% increase in myc_m, the fraction of ERM in total fungal biomass increases from 50% und 75%. Model sensitivity was tested for -50% and +50%. A 50% increase of myc_m leads to a decrease of fungal C by 21%. A decline in fungal C despite a higher fraction of ERM and thus a potentially higher nutrient uptake area seems at a first glance surprising but can be explained with fungal biomass turnover. Total fungal turnover is enhanced since ERM turnover, which is increased due to higher myc_m, is about 12 times higher than mantle turnover. Therefore fungal C demand is increased but cannot be completely compensated by increasing C transfer from the root. Root growth is negatively affected since less fungal biomass leads to a reduction of fungal N uptake and thus, N allocation to the root. Moreover, root C decreases due to an increase in the C allocation to the fungus. Although mycorrhization is about 25% lower than the baseline value, the root increases N uptake only marginally since root biomass is lower than the baseline value and root N uptake is inefficient compared to fungal N uptake. A decrease in myc_m by 50% has only positive effects on root and fungal growth since total fungal turnover is lowered and therefore fungal C demand is reduced. Due to higher fungal biomass, N uptake and N allocation to the root rise and favour root growth.
Fungal and root turnover rates have significant effects on model output. Turnover rates can be distinguished between turnover of roots which are not covered by the fungal sheath (dr_r), the turnover of the fungal mantle which covers fine roots (dr_m), and the turnover of fungal ERM (dr_myc). Changes in turnover rates cause a significant variation in model results, e.g. a change of mantle turnover of -50% to 50% leads to a change in fungal C of -8% to +9% and a change in root C of -11% to +10% compared to the baseline value (Table 4).
Discussion
The use of published data from field and laboratory experiments brings about uncertainty as experimental conditions are highly variable and often do not reflect natural conditions completely. Furthermore, model parameters are strongly generalized. Hence, we used a sensitivity analysis to demonstrate the general behaviour of the model and to provide a better understanding of the importance of the parameters.
Results of the model prediction cannot be directly validated with data from field studies as the model only captures feedback processes and is not used with site specific input data such as plant growth or measured data on soil temperature. However, our model results seem to be well in-line with data from field studies. Simulated maximum biomass values for fungal and root biomass (1600 kg DW. ha-1 and 5000 kg DW. ha-1) are within the range reported for different field experiments. Göransson et al. (2006a) measured 792 kg DW ha-1 for total fungal biomass and Wallander et al. (2001) and Wallander et al. (2004) estimated fungal biomass to be 1000 kg DW ha-1 in the organic horizon of a pine-spruce forest and 4800 kg DW ha-1 in the whole soil profile of a pine forest. Somewhat lower values were reported by Högberg and Högberg (2002) who found fungal biomass in the range of 160 kg DW ha-1. Modelled fine root biomass (<2 mm) values are similar to reported values, e.g. from Stober et al. (2000) who measured root biomass (<1 mm) of beech stands in Germany and France to be 1880 kg DW ha-1 and 4000 kg DW ha -1, respectively. Scarascia-Mugnozza et al. (2000) found beech fine root biomass (<1 mm) at different European sites to vary between 1500 and 3800 kg DW ha-1. Since the generally lower published data refer to fine roots smaller than 1 mm and model data refer to fine roots smaller 2 mm we can assume that the model data give a reasonable estimation of fine root biomass. In our baseline scenario the fungal contribution to plant N nutrition was 99%. This value is little higher than findings from Hobbie (2006) and Hobbie and Hobbie (2008) who estimated that the fungal contribution to N nutrition of Arctic Tundra vegetation was about 61 – 81% by means of N isotope patterns. Also van der Heijden et al. (2008) stated that mycorrhizal contribution to plant N nutrition can reach 80% (van der Heijden et al. 2008). That model results are higher than published data might result from uncertainties in root and fungal N uptake rates. Since plant roots are practically isolated from soil solution because they are almost completely covered by the fungal mantle (Taylor and Alexander 2005; Hobbie and Hobbie 2008) we can assume that with a mycorrhization of almost 100% nearly all N must pass the fungal mantle before entering the plant and, thus, a very high fungal contribution to plant N nutrition is quite reasonable.
Plant photosynthetic activity depends mainly on temperature, water availability, and ambient CO2 concentrations, and is also affected by mycorrhizal fungi. Indirectly, increased photosynthetic activity affects C sequestration in soil due to increased above and belowground plant litter production, but also due to increased C transfer into the soil via mycorrhizal fungi (Godbold et al. 2006). Mycorrhizal fungi stimulate photosynthesis probably by increasing sink activity and by providing support for an improved plant N nutrition via increased fungal N allocation. Reid et al. (1983) reported that mycorrhizal pine had a 2.1 fold higher photosynthetic (PS) rate per gram dry weight than non-mycorrhizal trees 10 weeks after inoculation with EM fungi. In another study, mycorrhizal beech seedling displayed an about 1.5 fold higher photosynthesis rate than non-mycorrhizal beech seedlings (Pena et al. 2008). This shows that mycorrhizal colonization has a significant positive influence on plant CO2 assimilation. In our simulations increases in photosynthetic activity were mirrored proportionally in increases in fungal and root biomass (Cfung and Croot) and increases in root and fungal N uptake. Model results are in agreement with field and laboratory studies. Reid et al (1983) found that a 2.1-fold PS-rate in mycorrhized plants in general caused a higher C allocation to belowground plant parts and increased respiration per unit root weight 3.6-fold. According to model results (Table 4), an increase in PS-rate of 100% leads to a directly proportional increase in C allocation belowground and also to an increase in root and fungal respiration by 100% (Fig. 7). Although the increase in respiration is less than the result from Reid et al. (1983), we can assume that the model captures the main mechanisms of C allocation quite well. Our sensitivity study also revealed that an increase in the optimum ratio between root and fungal biomass (CfCropt) does not proportionally affect fungal biomass. This is due to the fact that the allocation mechanism is controlled by the product “CfCropt · Croot” (Eq. 4). With a 50% higher CfCropt, root C content (Croot) falls by 17% compared to the baseline value since more C is allocated to the fungus and thus, the product is not proportionally higher than the baseline value. Due to higher C allocation to the fungus, the root C is reduced by 17%, meaning that at a higher CfCropt ratio the plant invests more in mycorrhization than in its own root system. At the same time, N allocation from fungus to root is little reduced compared to the baseline scenario and root N uptake is increased by about 100%. This seems at a first glance surprising because higher C investment into the fungus should favour plant growth instead of increasing root N uptake. This can be explained by the C allocation mechanism. With a 50% increase in CfCropt, more fungal C is necessary to build the optimum mycorrhization. Since the C allocation from the root is not sufficient for the fungus to increase its mantle cover, mycorrhization is only 73% for the +50% scenario (Fig. 5). Therefore less fine root biomass is covered by the fungal mantle and root N uptake rises. The fungal contribution to plant N nutrition is only little affected by that (-1%) since root N uptake rates are quite small compared to fungal N uptake rates. Though, the N allocation from root to the shoot is increased by 2% and thus, the shoot N supply is improved. It seems therefore that a higher plant C investment in fungal growth is disadvantageous for the plant since fungal contribution to plant nutrition decreases and the root increases its N uptake significantly. The reason for this is that the model is not directed at simulating the feedback processes between aboveground and belowground plant parts. With a 50% higher CfCropt ratio, the plant N allocation to the shoot rise only little by 2%. A higher N supply to the shoot, however, would lead to increased shoot growth and in return to a higher photosynthesis rate which again would increase root N demand. As a consequence, the N transfer from the fungus would rise and so the fungal contribution to plant N. Since the model does not capture this feedback process yet, model output seems unreasonable, but can be solved in coupling the MYCOFON model to a plant growth model which describes all major plant physiological processes.
Simulated increases in soil N concentration resulted in increased N uptake by plant roots and a decrease of the importance of fungal N supply to the plant to values close to zero at soil N concentrations being 400% as high as assumed for the baseline scenario. Root N uptake is increased due to three effects: a) at higher soil N concentration fungal biomass and thus, fungal N uptake and N allocation are decreased due to the effect of the factor fact_allomax which restricts C allocation to the fungus when N availability increases above 0.01 kg-N kg -1soil . Due to the lack of fungal N transfer, the root has an increased N demand and increases its own N uptake, b) the reduction in fungal biomass leads to a decrease of mycorrhizal colonization and thus, a smaller fraction of fine roots is covered by the fungal mantle. Therefore, c) a higher fraction of fine roots is available for nutrient absorption and N uptake rises. Root C content increases by 53% due to lowered C allocation to the fungus which seems unreasonable since root biomass in general is known to decrease with increasing N availability. Here, our model fails to simulate the relative decrease of root biomass compared to shoot biomass which is a well-known effect of high N loads on plant growth. The reason again is that in our model the feedback processes between belowground and aboveground biomass, like e.g. root N uptake and photosynthesis rate, can not be simulated. However, the problem would be solved in linking the MYCOFON model to a plant physiology model.
The model simulations on the effects of an increase in N availability are in general in good agreement with observations from field experiments. For a 200% increase in the soil N availability (Nav), model results predict a decrease in mycorrhization of 65%. Reid et al. (1983) found a reduction of mycorrhizal root tips by 67% following an increase in soil N concentration due to fertilization by 400%. Similar results were reported by Gorrisson et al. (1993) who measured mycorrhizal frequency after fertilization with 5 kg N ha-1 and 200 kg N ha-1 and found a decrease in mycorrhization of 60 and 87% after 6 and 18 months, respectively. Wallander and Nylund (1992) exposed different types of EM fungi to excess N (10 and 20-fold). This resulted in a reduction of ERM of 68% for Laccaria bicolor and 90% for Suillus bovinus. It is obvious that the intensity of mycorrhizal reduction caused by N fertilization is species- and site-dependent (Table 2). A model prediction can therefore just provide a rough estimation. Nevertheless, the principal mechanisms of the effect of N fertilization on mycorrhiza are captured quite well in our model. The highest uncertainty is caused by the lack of data which would allow us to specify the threshold of soil N concentration above which negative effects on mycorrhizal growth occur. The reason for this is that most studies on effects of soil N availability were conducted in laboratory experiments with unrealistic high amounts of N fertilization so that the response of mycorrhiza to a gradual increase of N availability caused e.g. by atmospheric N deposition has rarely been studied. Although there are some field studies on the response of mycorrhiza to natural gradients of N availability (e.g. Lilleskov et al. 2001; Högberg et al. 2003) the response of fungal biomass or C content to natural increases in N loads is difficult to deduce since studies consider either only changes in community composition or total fungal biomass, or data about N loads are insufficient to be assigned for modelling. In our model though, with few more input data like the optimum N concentration for fungal growth, we can adapt model sensitivity to N availability by the response curves implemented in the model.
Our simulations revealed that changes in the root N uptake rate hardly affected fungal N uptake. For the simulated environmental conditions, root surfaces are nearly fully covered by the mycorrhiza mantle. Therefore, changes in root N uptake rates have only minor impact, since for these conditions fungal N uptake rates are some 100-fold higher as root N uptake, so that changes in those can easily be compensated.
The maximum N allocation to the root (Nmax) is a very uncertain factor since almost no data about precise allocation rates are available. It is not completely clear yet, whereby this allocation is driven, i.e. if N allocation is a root controlled or a fungus controlled process. In our model, the N allocation is driven by sink-strength, i.e. by root N demand although an upper transfer limit is given by the fungal NC (Eq. 10). Findings from Hawkes et al. (2008) indicate that N allocation might, in fact, be source controlled, i.e. controlled by the fungus. They suggested that C allocation to the fungus is driven by the C sink strength, i.e. by the size of the ERM, and is therefore not depending on the amount of N which is supplied to the root as suggested by Nehls (2008). This would imply that N allocation to the root is in fact a source driven process which depends on the size of the fungal N content or fungal NC ratio. Hence, although Nmax is a very uncertain factor, the uncertainty range is below 1% for fungal and root C and below 9% and for fungal and root N uptake which simplifies error estimation.
Our sensitivity results demonstrate that variations in the ratio between ERM and fungal mantle (myc_m) strongly affect simulation results. Since myc_m is a parameter which is varying very much across fungal species, it would be helpful for further model development and site specific simulations if mycorrhizal “cadastres” were available. This means that for sites with certain soil and vegetation characteristics like humidity, pH, soil N, and other nutrient or pollutant concentration, lists of characteristic mycorrhizal fungi should be assembled. With knowledge about the characteristics of these fungal communities, like the ratio between ERM and mantle biomass (myc_m) and turnover rates, these cadastres could be used as model input in order to improve site specific simulations of C and N cycling.
Also, for reducing uncertainties in fungal and fine root turnover site specific data are needed. Even though some data about fungal and root turnover rates are available in literature, the huge species variability and effects of environmental conditions are resulting in a huge uncertainty. Therefore, site specific simulations cadastres as already mentioned above, including average turnover rates of characteristic species, would be helpful to reduce model uncertainties.
Conclusion
In this paper we introduce a simple dynamic feedback model which allows estimating the main C- and N- flows between ectomycorrhizal fungi and trees, to test the sensitivity of the system fungus-tree to environmental parameters, and to assess the fungal contribution to plant N nutrition. It uses only few input parameters and is therefore easily attachable to ecosystem models.
The sensitivity study showed that the model responses to variations of model parameters are in agreement with results from field and laboratory studies. Especially simulated variations in N availability or photosynthesis rate resulted in similar patterns of plant-fungal C- and N- exchange as found in field and laboratory experiments.
For the development of this mycorrhiza model we used published information about certain processes, e.g. the C allocation to the root system and to the fungus. Still, there are some processes and parameters, which are not well known. Fungal and root N uptake rates, the ratio between the ERM and the fungal mantle (myc_m), and the optimum ratio between root and fungal biomass (CfCropt) are parameters which significantly affect model results, but for which published data are scarce or missing. Especially the high diversity of fungal species and their potentially varying preferences for different N-forms hamper model evaluation.
Besides uncertainties of model parameters, the model has yet no link to belowground soil processes and stand growth. For example, changes in root N supply do not feed back on plant CO2 assimilation and nutrient uptake by roots or ERM does not affect nutrient availability. These restrictions were necessary for model development and testing. In order to couple belowground and aboveground processes and to evaluate the role of mycorrhiza for plant nutrition, the MYCOFON model will have to be linked to a plant physiology or forest-growth model and to a soil processes model. The first would have to provide a nutrient-dependent assimilation routine and represent allocation of C and N to different parts of the aboveground biomass which then feeds back to the carbon supply of the root-mycorrhiza system. The second would be required to update the concentration of different nitrogen forms in the soil solution considering supply from decomposing litter and dead mycorrhiza. Such a coupling provides means for estimating the importance of mycorrhiza to ecosystem C- and N cycling under different environmental conditions such as climate change and deposition regime.
The MYCOFON model clearly shows the complexity of the root-fungus relation and increases the understanding of the feedback between e.g. root-fungus C allocation and fungus-root N supply. However, it also reveals the huge gaps which still prevail in our knowledge in mycorrhizal research. Much effort has been put into the research about fungal N uptake and transfer by 15 N analysis. But especially for ectomycorrhiza still no reliable estimation of the precise N transfer rates expressed as a fraction of N taken up by the fungus is available. Therefore data from studies of AM have to be considered for model development. Even more severe, although many different fungal species and their structural and physiological characteristics have been identified (Agerer 2001), no species classification according to functional groups has been developed which would facilitate model development. Such a classification should attribute species to certain classes, e.g. with regard to biomass (formation of mycelium and mantle, biomass content related to root biomass, fraction of photosynthetic C received from tree partner) and symbiotic and site characteristics (typical symbiotic partners, occurrence depending on site characteristics like pH). For further mycorrhiza model development it would be very progressive if future field research would consider theses aspects and by that deliver the necessary database for model improvements.
References
Agerer R (1987-2002) Colour Atlas of Ectomycorrhizae. [1st-12th delivery].Einhorn, Schwäbisch-Gmünd
Agerer R (2001) Exploration types of ectomycorrhizae. A proposal to classify ectomycorrhizal mycelial systems according to their patterns of differentiation and putative ecological importance. Mycorrhiza 11:107–114. doi:10.1007/s005720100108
Ames RN, Reid CPP, Porter LK, Cambardella C (1983) Hyphal uptake and transport of nitrogen from two 15 N labelled sources by Glomus mosseae, a vesicular-arbuscular mycorrhizal fungus. New Phytol 116:381–396. doi:10.1111/j.1469-8137.1983.tb03506.x
Arnebrandt K (1994) Nitrogen amendments reduce the growth of extramatrical mycelium. Mycorrhiza 5:7–15. doi:10.1007/BF00204014
Baath E, Söderström B (1979) Fungal biomass and fungal immobilization of plant nutrients in Swedish forest soils. Rev Eco Bot 16:477–489
Baath E, Wallander H (2003) Soil and rhizosphere microorganisms have the same Q(10) for respiration in a model system. Glob Change Biol 9:1788–1791. doi:10.1046/j.1365-2486.2003.00692.x
Bauhus J, Bartsch N (1996) Fine-root growth in beech (Fagus sylvatica) forest gaps. Can J For Res 26:2153–2159. doi:10.1139/x26-244
Beckjord P (1985) Effects of nitrogen and phosphorus fertilization on growth and formation of ectomycorrhizae of Quercus alba and Q.rubra by Pisolithus tinctorius and Scleroderma auranteum. Can J Bot 63:1677–1680
Björkmann E (1942) Über die Bedingungen der Mykorrhizabildung bei Kiefer und Fichte. Symb Bot Ups 6:2
Blagodatskaya EV, Anderson T-H (1998) Interactive effects of pH and substrate quality on the fungal-to-bacterial ratio and QCO2 of microbial communities in forst soils. Soil Biol Biochem 30:1269–1274. doi:10.1016/S0038-0717(98)00050-9
Boone RD, Nadelhoffer KJ, Canary JD, Kaye JP (1998) Roots exert a strong influence on the temperature sensitivity of soil respiration. Nature 396:570–572. doi:10.1038/25119
Boswell GP, Jacobs H, Davidson FA, Gadd GM, Ritz K (2003) Growth and function of fungal mycelia in heterogeneous environments. Bull Math Biol 65:447–477. doi:10.1016/S0092-8240(03)00003-X
Brugnach M (2005) Process level sensitivity analysis for complex ecological models. Ecol Modell 187:99–120. doi:10.1016/j.ecolmodel.2005.01.044
Cannell MGR, Thornley JHM (2000) Modelling the components of plant respiration: some guiding principles. Ann Bot (Lond) 85:55–67. doi:10.1006/anbo.1999.0996
Carrodus BB (1966) Absorption of nitrogen by mycorrhizal roots of beech II factors affecting the assimilation of nitrogen. New Phytol 65:358–371. doi:10.1111/j.1469-8137.1966.tb06372.x
Chalot M, Kytöviita MM, Brun A, Finlay RD, Söderström B (1995) Factors affecting amino acid uptake by the ectomycorrhizal fungus Paxillus involutus. Mycol Res 99:1131–1138. doi:10.1016/S0953-7562(09)80784-3
Dannenmann M, Gasche R, Ledebuhr A, Papen H (2006) Effects of forest management on soil N cycling in beech forests stocking on calcareous soils. Plant Soil 287:279–300. doi:10.1007/s11104-006-9077-4
Deressa TG, Schenk MK (2008) Contribution of roots and hyphae to phosphorus uptake of mycorrhizal onion (Allium cepa.L) - a mechanistic modelling approach. J Plant Nutr Soil Sci 171:810–820. doi:10.1002/jpln.200700209
EK H (1997) The influence of nitrogen fertilization on the carbon economy of Paxillus involutus in ectomycorrhizal association with Betula pendula. New Phytol 135:133–142. doi:10.1046/j.1469-8137.1997.00621.x
Finlay RD (2008) Ecological aspects of mycorrhizal symbiosis: with special emphasis on the function diversity of interactions involving the extraradical mycelium. J Exp Bot 59:1115–1126. doi:10.1093/jxb/ern059
Finzi AC, Norby RJ, Calfapietra C, Gallet-Budyneka A, Gielen B, Holmes WE, Hoosbeek MR, Iverseng CM, Jackson RB, Kubiske ME, Ledford J, Liberloo M, Oren R, Polle A, Pritchard S, Zak DR, Schlesinger WH, Ceulemans R (2007) Increases in nitrogen uptake rather than nitrogen-use efficiency support higher rates of temperature forest productivity under elevated CO2. Proc Natl Acad Sci USA 104:14014–14019. doi:10.1073/pnas.0706518104
Gagnon J, Langlois CG, Bouchard D, Le Tacon F (1995) Growth and ectomycorrhizal formation of container-grown Douglas-fir seedlings inoculated with Laccaria biocolor under four levels of nitrogen fertilization. Can J For Res 25:1953–1961. doi:10.1139/x95-210
Garcia MO, Ovasapyan T, Greas M, Treseder KK (2008) Mycorrhizal dynamics under elevated CO2 and nitrogen fertilization in a warm temperate forest. Plant Soil 303:301–310. doi:10.1007/s11104-007-9509-9
Gavito ME, Curtis PS, Mikkelsen TN, Jakobsen I (2000) Atmospheric CO2 and mycorrhiza effetcs on biomass allocation and nutrient uptake of nodulated pea (Pisum sativum L.) plants. J Exp Bot 51:1931–1938. doi:10.1093/jexbot/51.352.1931
Gavito ME, Olsson PA, Rouhier H, Medina-Penafiel A, Jakobsen I, Bago A, Azcon-Aguilar C (2005) Temperature constraints on the growth and functioning of root organ cultures with arbuscular mycorrhizal fungu. New Phytol 168:179–188. doi:10.1111/j.1469-8137.2005.01481.x
Godbold DL, Berntson GM, Bazzaz FA (1997) Growth and mycorrhizal colonization of three North American tree species under elevated atmospheric CO2. New Phytol 137:433–440. doi:10.1046/j.1469-8137.1997.00842.x
Godbold DL, Berntson GM (1997) Elevated atmospheric CO2 concentration changes ectomycorrhizal morphotype assemblages in Betula papyrifera. Tree Physiol 17:347–350
Godbold DL, Hoosbeek MR, Lukac M, Cotrufo MF, Janssens IA, Ceulemans R, Polle A, Velthorst EJ, Scarascia-Mugnozza G, De Angelis P, Miglietta F, Peressotti A (2006) Mycorrhizal hyphal turnover as a dominant process for carbon input into soil organic matter. Plant Soil 281:15–24. doi:10.1007/s11104-005-3701-6
Göransson H, Wallander H, Ingerslev M, Rosengreen U (2006a) Estimating the relative nutrient uptake from different soil depths in Quercus robur, Fagus sylvatica and Picea abies. Plant Soil 286:87–98. doi:10.1007/s11104-006-9028-0
Göransson H, Wallander H, Ingerslev M, Rosengreen U (2006b) Nutrient acquisition from different soil depth by pedunculate oak. Trees (Berl) 20:292–298. doi:10.1007/s00468-005-0034-2
Gordon WS, Jackson RB (2000) Nutrient concentrations in fine roots. Ecology 81:275–280
Gorisson A, Jansen AE, Olsthoorn AFM (1993) Effects of a two-year application of ammonium sulphate on growth, nutrient uptake, and rhizosphere microflora of juvenile Douglas fir. Plant Soil 157:41–50
Granier A, Ceschia E, Damesin C, Dufrene E, Epron D, Gross P, Lebaube S, Le Dantec V, Le Goff N, Lemoine D, Lucot E, Ottorini JM, Pontailler JY, Saugier B (2000) The carbon balance of a young beech forest. Funct Ecol 14:312–325. doi:10.1046/j.1365-2435.2000.00434.x
Halley JM, Comins HN, Lawton JH, Hassell MP (1994) Competition, succession and pattern in fungal communities: towards a cellular automaton model. Oikos 70:435–442. doi:10.2307/3545783
Hawkes CV, Hartley IP, Ineson P, Fitter AH (2008) Soil temperature affects allocation within arbuscular mycorrhizal networks and carbon transport from plant to fungus. Glob Change Biol 14:1–10. doi:10.1111/j.1365-2486.2007.01535.x
Heinemeyer A, Fitter AH (2004) Impact of temperature on the arbuscular mycorrhizal (AM) symbiosis: growth responses of the host plant and its AM fungal partner. J Exp Bot 55:525–534. doi:10.1093/jxb/erh049
Heinemeyer A, Ineson P, Ostle N, Fitter AH (2006) Respiration of the external mycelium in the arbuscular mycorrhizal symbiosis shows strong dependence on recent photosynthates and acclimation to temperature. New Phytol 171:159–170. doi:10.1111/j.1469-8137.2006.01730.x
Heinemeyer A, Hartley IP, Evans SP, Carreira de la fuentes JA, Ineson P (2007) Forest soil CO2 flux: uncovering the contribution and environmental responses of ectomycorrhizas. Glob Change Biol 13:1786–1797. doi:10.1111/j.1365-2486.2007.01383.x
Hobbie EA (2006) Carbon allocation to ectomycorrhizal fungi correlates with belowground allocation in culture studies. Ecology 87:563–569. doi:10.1890/05-0755
Hobbie EA, Hobbie JE (2008) Natural abundance of 15 N in nitrogen-limited forests and tundra can estimate nitrogen cycling through mycorrhiza fungi: a review. Ecosystems (N Y, Print) 11:815–830. doi:10.1007/s10021-008-9159-7
Högberg M, Baath E, Nordgren A, Arnebrandt K, Högberg P (2003) Contrasting effects of nitrogen availability on plant carbon supply to mycorrhizal fungi and saprotrophs - a hypothesis based on field observations in boreal forest. New Phytol 160:225–238. doi:10.1046/j.1469-8137.2003.00867.x
Högberg MN, Högberg P (2002) Extramatrical ectomycorrhizal mycelium contributes one-third of microbial biomass and produces, together with associated roots, half the dissolved organic carbon in a forest soil. New Phytol 154:791–795. doi:10.1046/j.1469-8137.2002.00417.x
Jakobsen I, Rosendahl L (1990) Carbon flow into soil and external hyphae from roots of mycorrhizal cucumber. New Phytol 115:77–83. doi:10.1111/j.1469-8137.1990.tb00924.x
Johnson D, Ijdo M, Genney DR, Anderson IC, Alexander IJ (2005) How do plants regulate the function, community structure, and diversity of mycorrhizal fungi? J Exp Bot 417:1751–1760. doi:10.1093/jxb/eri192
Jolicoeur M, Bouchard-Marchand E, Bécard G, Perrier M (2002) Regulation of mycorrhizal symbiosis:development of a structured nutritional dual model. Ecol Modell 158:121–142. doi:10.1016/S0304-3800(02)00168-0
Jordy MN, Azemar-Lorentz S, Brun A, Botton B, Pargney JC (1998) Cytolocalization of glycogen, starch, and other insoluble polyssacharides during ontogeny of Paxillus involutus - Betula pendula ectomycorrhizas. New Phytol 140:331–341. doi:10.1046/j.1469-8137.1998.00254.x
Kamminga-van Wijk C, Prins HBA, Kuiper PJC (1992) Mycorrhizal and non-mycorrhizal Douglas fir grown in hydroculture.The effect of nutrient concentration on the formation and functioning of mycorrhiza. Acta Bot Neerl 41:481–495
Kreutzer K, Weiss T (1998) The Höglwald field experiments - aims, concept and basic data. Plant Soil 199:1–10. doi:10.1023/A:1004384909535
Laczko E, Boller T, Wiemken V (2003) Lipids in roots of Pinus sylvestris seedlings and in mycelia of Pisolithus tinctorius during ectomycorrhiza formation:changes in the fatty acid and sterol composition. Plant Cell Environ 27:27–40. doi:10.1046/j.0016-8025.2003.01122.x
Langley JA, Hungate BA (2003) Mycorrhizal controls on belowground litter quality. Ecology 84:2302–2312. doi:10.1890/02-0282
Langley JA, Chapman SK, Hungate BA (2006) Ectomycorrhizal colonization slows roots decomposition:the post portem fungal legacy. Ecol Lett 9:955–959. doi:10.1111/j.1461-0248.2006.00948.x
Leake JR (2007) Mycorrhizas and the terrestrial carbon cycle: roles in global carbon sequestration and plant community composition. In: Gadd GM, Watkinson SC, Dyer PS (eds) Fungi in the environment. Cambridge University Press, Cambridge, pp 161–185
Lewis JD, Thomas RB, Strain BR (1994) Effect of elevated CO2 on mycorrhizal colonization on lobolly pine (Pinus taeda L.) seedlings. Plant Soil 165:81–88. doi:10.1007/BF00009965
Litton CM, Raich JW, Ryan MG (2007) Carbon allocation in forest ecosystems. Glob Change Biol 13:2089–2109. doi:10.1111/j.1365-2486.2007.01420.x
Malcolm G, Lopez-Gutierrez J, Koide RT, Eissenstat DM (2008) Acclimation to temperature and temperature sensivity of metabolism by ectomycorrhizal fungi. Glob Change Biol 14:1169–1180. doi:10.1111/j.1365-2486.2008.01555.x
Marschner H, Dell B (1994) Nutrient uptake in mycorrhizal symbiosis. Plant Soil 159:89–102
Martin F, Ramstedt M, Söderhäll K, Canet D (1988) Carbohydrate and amino acid metabolisms in the ectomycorrhizal ascomycete Sphaerosporella brunnea during glucose utilization. Plant Physiol 86:935–940. doi:10.1104/pp. 86.3.935
Martin F, Aerts A, Ahren D, Brun A, Danchin EGJ, Duchaussoy F, Gibon J, Kohler A, Lindquist E, Pereda V, Salamov A, Shapiro HJ, Wuyts J, Blaudez D, Buee M, Brokstein P, Canback B, Cohen D, Courty PE, Coutinho PM, Delaruelle C, Detter JC, Deveau A, DiFazio S, Duplessis S, Fraissinet-Tachet L, Lucic E, Frey-Klett P, Fourrey C, Feussner I, Gay G, Grimwood J, Hoegger PJ, Jain P, Kilaru S, Labbe J, Lin YC, Legue V, Le Tacon F, Marmeisse R, Melayah D, Montanini B, Muratet M, Nehls U, Niculita-Hirzel H, Oudot-Le Secq MP, Peter M, Quesneville H, Rajashekar B, Reich M, Rouhier N, Schmutz J, Yin T, Chalot M, Henrissat B, Kues U, Lucas S, de Peer YV, Podila GK, Polle A, Pukkila PJ, Richardson PM, Rouze P, Sanders IR, Stajich JE, Tunlid A, Tuskan G, Grigoriev IV (2008) The genome of Laccaria bicolor provides insights into mycorrhizal symbiosis. Nature 452:88–92. doi:10.1038/nature06556
Marx DH (1990) Soil pH and nitrogen influence Pisolithus ectomycorrhizal development and growth of lobolly pine seedlings. For Sci 36:224–245
Matamala R, Gonzalez-Meler MA, Jastrow JD, Norby RJ, Schlesinger WH (2003) Impacts of fine root turnover on forest NPP and soil C sequestration potential. Science 302:1385–1387. doi:10.1126/science.1089543
Moyano FE, Kutsch WL, Schulze ED (2007) Response of mycorrhizal, rhizosphere and soil basal respiration to temperature and photosynthesis in a barley field. Soil Biol Biochem 39:843–863. doi:10.1016/j.soilbio.2006.10.001
Müller T, Avolio M, Olivi M, Benjdia M, Rikirsch E, Kasaras A, Fitz M, Chalot M, Wipf D (2007) Nitrogen transport in the ectomycorrhiza association: the Hebeloma cylindrosporum-Pinus pinaster model. Phytochemistry 68:41–51. doi:10.1016/j.phytochem.2006.09.021
Nehls U (2008) Mastering ectomycorrhizal symbiosis: the impact of carbohydrates. J Exp Bot 59:1097–1108. doi:10.1093/jxb/erm334
Neuhauser C, Fargione JE (2004) A mutualism-parasitism continuum model and its applictaion to plant-mycorrhizae interactions. Ecol Modell 177:337–352. doi:10.1016/j.ecolmodel.2004.02.010
Newton AC, Pigott CD (1991) Mineral nutrition and mycorrhizal infection of seedling oak and birch. Nova Hedwigia 117:45–52
Nilsson LO, Wallander H (2003) Production of external mycelium by ectomycorrhizal fungi in a norway spruce forest was reduced in response to nitrogen fertilization. New Phytol 158:409–416. doi:10.1046/j.1469-8137.2003.00728.x
Nilsson LO, Baath E, Falkengren-Grerup U, Wallander H (2007) Growth of ectomycorrhizal mycelia and composition of soil microbial communities in oak forest soils along a nitrogen deposition gradient. Oecologia 153:375–384. doi:10.1007/s00442-007-0735-x
Pena R, Offermann C, Simon J, Naumann PS, Geßler A, Holst J, Mayer H, Kögel-Knabner I, Rennenberg H, Polle A (2008) Carbon limitations after girdling affect ectomycorrhizal diversity and reveal functional differences of EM community composition in a mature beech forest (Fagus sylvatica). New.Phytol. (subm.)
Plassard C, Schroemm P, Mouisan D, Salsac L (1991) Assimilation of mineral nitrogen and ion balance in the two partners of ectomycorrhizal symbiosis: data and hypothesis. Experimentia 47:340–349. doi:10.1007/BF01972075
Pritsch K, Raidl S, Marksteiner E, Blaschke H, Agerer R, Schloter M, Hartmann A (2004) A rapid and highly sensitive method for measuring enzyme activities in single mycorrhizal tips using 4-methylumbelliforme-labelled fluorogenic substrates in a microplate system. J Microbiol Methods 58:233–241. doi:10.1016/j.mimet.2004.04.001
Read DJ (1991) Mycorrhizas in Ecosystems. Experimentia 47:376–391. doi:10.1007/BF01972080
Read DJ, Perez-Moreno J (2003) Mycorrhizas and nutrient cycling in ecosystems - a journey towards relevance? New Phytol 157:475–492. doi:10.1046/j.1469-8137.2003.00704.x
Reid CPP, Kidd FA, Ekwebelam SA (1983) Nitrogen nutrition, photosynthesis and carbon allocation in ectomycorrhizal pine. Plant Soil 71:415–432. doi:10.1007/BF02182683
Richards BN (1965) Mycorrhiza development of loblolly pine seedlings in relation to soil reaction and the supply of nitrate. Plant Soil 22:187–199. doi:10.1007/BF01373990
Runion GB, Curl EA, Rogers HH, Backman PA, Rodríguez-Kábana R, Helms BE (1994) Effects of free-air CO2 enrichment on microbial populations in the rhizosphere and phyllosphere of cotton. Agric For Meteorol 70:117–130. doi:10.1016/0168-1923(94)90051-5
Schnepf A, Roose T (2006) Modelling the contribution of arbuscular mycorrhizal fungi to plant phosphate uptake. New Phytol 171:669–682
Schnepf A, Roose T, Schweiger P (2008) Growth model for arbuscular mycorrhizal fungi. J.R. Soc. Lond. Interface 5:773–784
Smith SE, Smith FA (1990) Structure and function of the interfaces in biotrophic symbioses as they relate to nutrient transport. New Phytol 114:1–38. doi:10.1111/j.1469-8137.1990.tb00370.x
Smith SE, Read DJ (1997) Mycorrhizal symbiosis. Academic Press, London
Staddon PL, Heinemeyer A, Fitter AH (2002) Mycorrhizas and global environmental change: research at different scales. Plant Soil 244:253–261. doi:10.1023/A:1020285309675
Staddon PL, Ramsey CB, Ostle N, Ineson P, Fitter AH (2003) Rapid turnover of hyphae of mycorrhizal fungi determined by AMS microanalysis of C-14. Science 300:1138–1140. doi:10.1126/science.1084269
Stober C, George E, Persson H (2000) Root growth and Response to Nitrogen. In: Schulze ED (ed) Carbon and Nitrogen cycling in European forest ecosystems. Springer Verlag, Berlin, pp 99–121
Taylor AF, Alexander I (2005) The ectomycorrhizal symbiosis: life in the real world. Mycologist 19:102–112. doi:10.1017/S0269915X05003034
Taylor JH, Peterson CA (2005) Ectomycorrhizal impacts on nutrient uptake pathways in woody roots. New For 30:203–214. doi:10.1007/s11056-005-0489-4
Thornley JHM, Cannell MGR (2000) Modelling the components of plant respiration: representation and realism. Ann Bot (Lond) 85:55–67. doi:10.1006/anbo.1999.0997
Treseder KK (2004) A meta-analysis of mycorrhizal responses to nitrogen, phosphorus, and atmospheric CO2 in field studies. New Phytol 164:347–355. doi:10.1111/j.1469-8137.2004.01159.x
Treseder KK, Turner KM, Mack MC (2007) Mycorrhizal responses to nitrogen fertilization in boreal ecosystems: potential consequences for soil carbon storage. Glob Change Biol 13:78–88. doi:10.1111/j.1365-2486.2006.01279.x
Tyler G (1985) Macrofungal flora of swedish beech forest related to soil organic matter and acidity characteristics. For Ecol Manage 10:13–29. doi:10.1016/0378-1127(85)90011-8
van der Heijden MGA, Bardgett RD, van Straalen NM (2008) The unseen majority: soil microbes as drivers of plant diversity and productivity in terrestrial ecosystems. Ecol Lett 11:296–310. doi:10.1111/j.1461-0248.2007.01139.x
Verbeeck H, Samson R, Granier A, Montpied P, Lemeur R (2008) Multi-year model analysis of GPP in a temperate beech forest in France. Ecol Modell 210:85–103. doi:10.1016/j.ecolmodel.2007.07.010
Wallander H, Nylund JE (1991) Effects of excess nitrogen on carbohydrate concentration and mycorrhizal development of Pinus sylvestris L. seedlings. New Phytol 119:405–411. doi:10.1111/j.1469-8137.1991.tb00040.x
Wallander H, Nylund JE (1992) Effects of excess nitrogen and phosphorus starvation on the extramatrical mycelium of ectomycorrhizas. New Phytol 120:495–503. doi:10.1111/j.1469-8137.1992.tb01798.x
Wallander H (1995) A new hypothesis to explain allocation of dry matter between mycorrhizal fungi and pine seedlings in relation to nutrient supply. Plant Soil 168–169:243–248. doi:10.1007/BF00029334
Wallander H, Arnebrandt K, Dahlberg A (1999) Relationsships between fungal uptake of ammonium, fungal growth and nitrogen availability in ectomycorrhizal Pinus sylvestris seedlings. Mycorrhiza 8:215–223. doi:10.1007/s005720050237
Wallander H, Nilsson LO, Hagerberg D, Baath E (2001) Estimation of the biomass and seasonal growth of external mycelium of ectomycorrhizal fungi in the field. New Phytol 151:753–760. doi:10.1046/j.0028-646x.2001.00199.x
Wallander H, Nilsson LO (2003) Direct estimates of C:N ratios of ectomycorrhizal mycelia collected from Norway spruce forest soils. Soil Biol Biochem 35:997–999. doi:10.1016/S0038-0717(03)00121-4
Wallander H, Göransson H, Rosengreen U (2004) Production, standing biomass and natural abundance of 15 N and 13C in ectomycorrhizal mycelia collected at different soil depth in two forest types. Oecologia 139:89–97. doi:10.1007/s00442-003-1477-z
Wallenda T, Kottke I (1998) Nitrogen deposition and ectomycorrhizas. New Phytol 139:169–187. doi:10.1046/j.1469-8137.1998.00176.x
Wallenda T, Stober C, Högböm L, Schinkel H, George E, Högberg P, Read DJ (2000) Nitrogen uptake processes in roots and mycorrhizas. In: Schulze ED (ed) Carbon and nitrogen cycling in european forest ecosystems. Springer Verlag, Heidelberg, pp 122–143
Wiemken V, Ineichen K, Boller T (2001) Development of ectomycorrhizas in model beech-spruce ecosystems on siliceous and calcareous soil: a 4-year experiment with atmospheric CO2 enrichment and nitrogen fertilization. Plant Soil 234:99–10. doi:10.1023/A:1010500400263
Acknowledgements
This work was funded by the Deutsche Forschungsgemeinschaft (DFG) within the Beech Research Group and the EU funded integrated project NitroEurope.
Author information
Authors and Affiliations
Corresponding author
Additional information
Responsible Editor: Ute Skiba.
An erratum to this article can be found at http://dx.doi.org/10.1007/s11104-009-0157-0
Appendix
Appendix
Rights and permissions
Open Access This is an open access article distributed under the terms of the Creative Commons Attribution Noncommercial License ( https://creativecommons.org/licenses/by-nc/2.0 ), which permits any noncommercial use, distribution, and reproduction in any medium, provided the original author(s) and source are credited.
About this article
Cite this article
Meyer, A., Grote, R., Polle, A. et al. Simulating mycorrhiza contribution to forest C- and N cycling-the MYCOFON model. Plant Soil 327, 493–517 (2010). https://doi.org/10.1007/s11104-009-0017-y
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11104-009-0017-y