Skip to main content
SpringerLink
Log in

A magnetic control method for large-deformation vibration of cantilevered pipe conveying fluid

  • Original paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

Abstract

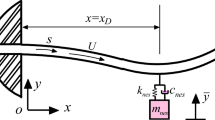
Soft active materials have the ability to undergo large deformation in response to stimuli such as light, heat, magnetic, and electric fields. Due to their promising applications in the fields of soft robots, flexible electronics, and biomedicine engineering, they have attracted tremendous attention from different disciplines and developed rapidly in the past decades basing on mutual efforts. Recently, a new class of soft active materials, known as hard-magnetic soft (HMS) materials is successfully developed. By applying magnetic fields, unprecedented mechanical behaviors of HMS structures have been observed. To further explore the potential applications of HMS materials, this work will investigate the dynamical behaviors of fluid-conveying pipes made of HMS materials for the first time. By considering the exactly geometric nonlinearities due to the bending deformation of the pipe, the governing equation of a cantilevered HMS pipe conveying fluid is derived based on Hamilton’s principle. The analyses of the stability, static deformation, and nonlinear vibration of the HMS pipe are conducted by solving the obtained governing equation. It is found that there is a critical flow velocity for the dynamic instability of the pipe. When the flow velocity is below this value, the HMS pipe may undergo a large static deformation in a stable state. However, the pipe would periodically oscillate with a large amplitude when the flow velocity is beyond the critical flow velocity. Results also indicate the mechanical responses including static deformation, loss of stability, and vibration of the HMS pipe conveying fluid can be effectively controlled by applying an external magnetic field.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17

Similar content being viewed by others

Data availability

The datasets generated during and/or analyzed during the current study are available from the corresponding author on reasonable request.

References

  1. Yang, J., Yabuno, H., Yanagisawa, N., Yamamoto, Y., Matsumoto, S.: Measurement of added mass for an object oscillating in viscous fluids using nonlinear self-excited oscillations. Nonlinear Dyn. 102(4), 1987–1996 (2020)

    Article  Google Scholar 

  2. Zhang, Z., Zhang, X., Ge, Y.: Motion-induced vortex shedding and lock-in phenomena of a rectangular section. Nonlinear Dyn. 102(4), 2267–2280 (2020)

    Article  MathSciNet  Google Scholar 

  3. Chen, W., Dai, H.L., Wang, L.: Enhanced stability of two-material panels in supersonic flow: optimization strategy and physical explanation. AIAA J. 57(12), 5553–5565 (2019)

    Article  Google Scholar 

  4. Lu, Z.Q., Zhang, K.K., Ding, H., Chen, L.Q.: Nonlinear vibration effects on the fatigue life of fluid-conveying pipes composed of axially functionally graded materials. Nonlinear Dyn. 100(2), 1091–1104 (2020)

    Article  Google Scholar 

  5. Reddy, R.S., Panda, S., Natarajan, G.: Nonlinear dynamics of functionally graded pipes conveying hot fluid. Nonlinear Dyn. 99(3), 1989–2010 (2020)

    Article  Google Scholar 

  6. Thomsen, J.J., Fuglede, N.: Perturbation-based prediction of vibration phase shift along fluid-conveying pipes due to Coriolis forces, nonuniformity, and nonlinearity. Nonlinear Dyn. 99(1), 173–199 (2020)

    Article  MATH  Google Scholar 

  7. Rousselet, J., Herrmann, G.: Dynamic behavior of continuous cantilevered pipes conveying fluid near critical velocities. J. Appl. Mech. 48(4), 943–947 (1981)

    Article  Google Scholar 

  8. Wang, L.: Flutter instability of supported pipes conveying fluid subjected to distributed follower forces. Acta Mech. Solida Sin. 25(1), 46–52 (2012)

    Article  Google Scholar 

  9. Sugiyama, Y., Tanaka, Y., Kishi, T., Kawagoe, H.: Effect of a spring support on the stability of pipes conveying fluid. J. Sound Vib. 100(2), 257–270 (1985)

    Article  Google Scholar 

  10. Païdoussis, M.P., Semler, C.: Nonlinear and chaotic oscillations of a constrained cantilevered pipe conveying fluid: a full nonlinear analysis. Nonlinear Dyn. 4(6), 655–670 (1993)

    Article  Google Scholar 

  11. Ghayesh, M.H., Païdoussis, M.P., Modarres-Sadeghi, Y.: Three-dimensional dynamics of a fluid-conveying cantilevered pipe fitted with an additional spring-support and an end-mass. J. Sound Vib. 330(12), 2869–2899 (2011)

    Article  Google Scholar 

  12. Païdoussis, M.P., Sundararajan, C.: Parametric and combination resonances of a pipe conveying pulsating fluid. J. Appl. Mech. 42(4), 780–784 (1975)

    Article  MATH  Google Scholar 

  13. Bai, Y., Xie, W., Gao, X., Wu, X.: Dynamic analysis of a cantilevered pipe conveying fluid with density variation. J. Fluids Struct. 81, 638–655 (2018)

    Article  Google Scholar 

  14. Dehrouyeh-Semnani, A.M., Nikkhah-Bahrami, M., Yazdi, M.R.H.: On nonlinear vibrations of micropipes conveying fluid. Int. J. Eng. Sci. 117, 20–33 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  15. Ghayesh, M.H., Farajpour, A., Farokhi, H.: Viscoelastically coupled mechanics of fluid-conveying microtubes. Int. J. Eng. Sci. 145, 103139 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  16. Bourrières, F.J.: Sur un phénomène d’oscillation auto-entretenue en mécanique des fluides réels. Publications Scientifiques et Techniques du Ministère de l’Air, No. 147 (1939)

  17. Feodos’ev, V.P.: Vibrations and stability of a pipe when liquid flows through it. Inzhenernyi Sbornik 10, 169–170 (1951)

    Google Scholar 

  18. Housner, G.W.: Bending vibrations of a pipe line containing flowing fluid. J. Appl. Mech. 19, 205–208 (1952)

    Article  Google Scholar 

  19. Niordson, F.I.: Vibrations of a cylindrical tube containing flowing fluid. Kungliga Tekniska Hogskolans Handlingar (Stockholm), No. 73 (1953)

  20. Qian, Q., Wang, L., Ni, Q.: Instability of simply supported pipes conveying fluid under thermal loads. Mech. Res. Commun. 36(3), 413–417 (2009)

    Article  MATH  Google Scholar 

  21. Kheiri, M., Païdoussis, M.P., Del Pozo, G.C., Amabili, M.: Dynamics of a pipe conveying fluid flexibly restrained at the ends. J. Fluids Struct. 49, 360–385 (2014)

    Article  Google Scholar 

  22. Bahaadini, R., Saidi, A.R.: Stability analysis of thin-walled spinning reinforced pipes conveying fluid in thermal environment. Eur. J. Mech. A/Solids 72, 298–309 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  23. Ritto, T.G., Soize, C., Rochinha, F.A., Sampaio, R.: Dynamic stability of a pipe conveying fluid with an uncertain computational model. J. Fluids Struct. 49, 412–426 (2014)

    Article  Google Scholar 

  24. Ni, Q., Zhang, Z.L., Wang, L.: Application of the differential transformation method to vibration analysis of pipes conveying fluid. Appl. Math. Comput. 217(16), 7028–7038 (2011)

    MathSciNet  MATH  Google Scholar 

  25. Benjamin, T.B.: Dynamics of a system of articulated pipes conveying fluid. II. Experiments. Proc. R. Soc. Lond. A 261, 487–499 (1961)

    Article  MathSciNet  MATH  Google Scholar 

  26. Gregory, R.W., Païdoussis, M.P.: Unstable oscillation of tubular cantilevers conveying fluid, II. Experiments. Proc. R. Soc. Lond. A 293, 528–542 (1966)

    Article  Google Scholar 

  27. Benjamin, T.B.: Dynamics of a system of articulated pipes conveying fluid, I Theory. Proc. R. Soc. Lond. A 261, 457–486 (1961)

    Article  MathSciNet  MATH  Google Scholar 

  28. Gregory, R.W., Païdoussis, M.P.: Unstable oscillation of tubular cantilevers conveying fluid, I Theory. Proc. R. Soc. Lond. A 293, 512–527 (1966)

    Article  MATH  Google Scholar 

  29. Li, Q., Liu, W., Lu, K., Yue, Z.: Nonlinear parametric vibration of a fluid-conveying pipe flexibly restrained at the ends. Acta Mech. Solida Sin. 33(3), 327–346 (2020)

    Article  Google Scholar 

  30. Liu, Z.Y., Wang, L., Sun, X.P.: Nonlinear forced vibration of cantilevered pipes conveying fluid. Acta Mech. Solida Sin. 31(1), 32–50 (2018)

    Article  Google Scholar 

  31. Lundgren, T.S., Sethna, P.R., Bajaj, A.K.: Stability boundaries for flow induced motions of tubes with an inclined terminal nozzle. J. Sound Vib. 64, 553–571 (1979)

    Article  MATH  Google Scholar 

  32. Rousselet, J., Herrmann, G.: Dynamic behaviour of continuous cantilevered pipes conveying fluid near critical velocities. J. Appl. Mech. 48, 943–947 (1981)

    Article  Google Scholar 

  33. Païdoussis, M.P.: Fluid-Structure Interactions: Slender Structures and Axial Flow. Academic Press, London (1998)

    Google Scholar 

  34. Holmes, P.J.: Bifurcations to divergence and flutter in flow-induced oscillations: a finite-dimensional analysis. J. Sound Vib. 53, 471–503 (1977)

    Article  MathSciNet  MATH  Google Scholar 

  35. Ch’ng, E. & Dowell, E.H.: A theoretical analysis of nonlinear effects on the flutter and divergence of a tube conveying fluid. In Flow-Induced Vibrations (eds S.S. Chen & M.D. Bernstein), pp. 65–81. New York: ASME (1979)

  36. Bajaj, A.K., Sethna, P.R., Lundgren, T.S.: 1980 Hopf bifurcation phenomena in tubes carrying fluid. SIAM J. Appl. Math. 39, 213–230 (1980)

    Article  MathSciNet  MATH  Google Scholar 

  37. Rousselet, J., Herrmann, G.: Dynamic behaviour of continuous cantilevered pipes conveying fluid near critical velocities. J. Appl. Mech. 48(4), 943–947 (1981)

    Article  Google Scholar 

  38. Semler, C., Li, G.X., Païdoussis, M.P.: The nonlinear equations of motion of pipes conveying fluid. J. Sound Vib. 169, 577–599 (1994)

    Article  MATH  Google Scholar 

  39. Païdoussis, M.P., Semler, C.: Non-linear dynamics of a fluid-conveying cantilevered pipe with a small mass attached at the free end. Int. J. Nonlin. Mech. 33(1), 15–32 (1998)

    Article  MATH  Google Scholar 

  40. Sarkar, A., Païdoussis, M.P.: , MP A compact limit-cycle oscillation model of a cantilever conveying fluid. J. Fluids Struct. 17(4), 525–539 (2003)

    Article  Google Scholar 

  41. Zhou, K., Xiong, F.R., Jiang, N.B., Dai, H.L., Yan, H., Wang, L., Ni, Q.: Nonlinear vibration control of a cantilevered fluid-conveying pipe using the idea of nonlinear energy sink. Nonlinear Dyn. 95(2), 1435–1456 (2019)

    Article  Google Scholar 

  42. Wadham-gagnon, M., Païdoussis, M.P., Semler, C.: Dynamics of cantilevered pipes conveying fluid. Part 1: Nonlinear equations of three-dimensional motion. J. Fluids Struct. 23, 545–567 (2007)

    Article  Google Scholar 

  43. Ghayesh, M.H., Païdoussis, M.P.: Three-dimensional dynamics of a cantilevered pipe conveying fluid, additionally supported by an intermediate spring array. Int. J. Nonlin. Mech. 45(5), 507–524 (2010)

    Article  Google Scholar 

  44. Chang, G.H., Modarres-Sadeghi, Y.: Flow-induced oscillations of a cantilevered pipe conveying fluid with base excitation. J. Sound Vib. 333(18), 4265–4280 (2014)

    Article  Google Scholar 

  45. Païdoussis, M.P., Semler, C.: Nonlinear dynamics of a fluid-conveying cantilevered pipe with an intermediate spring support. J. Fluids Struct. 7(3), 269–298 (1993)

    Article  Google Scholar 

  46. Tang, D.M., Dowell, D.H.: Chaotic oscillations of a cantilevered pipe conveying fluid. J. Fluids Struct. 2(3), 263–283 (1998)

    Article  Google Scholar 

  47. Chen, W., Dai, H.L., Jia, Q.Q., Wang, L.: Geometrically exact equation of motion for large-amplitude oscillation of cantilevered pipe conveying fluid. Nonlinear Dyn. 98(3), 2097–2114 (2019)

    Article  MATH  Google Scholar 

  48. Chen, W., Hu, Z., Dai, H.L., Wang, L.: Extremely large-amplitude oscillation of soft pipes conveying fluid under gravity. Appl. Math. Mech. 41(9), 1381–1400 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  49. Kim, Y., Parada, G.A., Liu, S., Zhao, X.: Ferromagnetic soft continuum robots. Sci. Robot. 4(33), eaax7329 (2019)

    Article  Google Scholar 

  50. Zhou, K., Dai, H.L., Wang, L., Liu, Z.Y., Zhang, L.B., Jiang, T.L., Chen, W., Lin, S.X., Yi, H.R.: A underwater bionic robot based on the actuation of flow-induced vibration, Chinese Patent for Invention CN109367746A (2019) (In Chinese)

  51. Yang, T., Liu, T., Tang, Y., Hou, S., Lv, X.: Enhanced targeted energy transfer for adaptive vibration suppression of pipes conveying fluid. Nonlinear Dyn. 97(3), 1937–1944 (2019)

    Article  Google Scholar 

  52. Ding, H., Ji, J., Chen, L.Q.: Nonlinear vibration isolation for fluid-conveying pipes using quasi-zero stiffness characteristics. Mech. Syst. Signal Pr. 121, 675–688 (2019)

    Article  Google Scholar 

  53. Kim, Y., Yuk, H., Zhao, R., Chester, S.A., Zhao, X.: Printing ferromagnetic domains for untethered fast-transforming soft materials. Nature 558(7709), 274–279 (2018)

    Article  Google Scholar 

  54. Venkiteswaran, V.K., Samaniego, L.F.P., Sikorski, J., Misra, S.: Bio-inspired terrestrial motion of magnetic soft millirobots. IEEE Robot. Autom. Let. 4(2), 1753–1759 (2019)

    Article  Google Scholar 

  55. Chen, W., Wang, L.: Theoretical modeling and exact solution for extreme bending deformation of hard-magnetic soft beams. J. Appl. Mech. 87(4), 041002 (2020)

    Article  Google Scholar 

  56. Wang, L., Kim, Y., Guo, C.F., Zhao, X.: Hard-magnetic elastica. J. Mech. Phys. Solids 142, 104045 (2020)

    Article  MathSciNet  Google Scholar 

  57. Chen, W., Yan, Z., Wang, L.: Complex transformations of hard-magnetic soft beams by designing residual magnetic flux density. Soft Matter 16, 6379–6388 (2020)

    Article  Google Scholar 

  58. Chen, W., Yan, Z., Wang, L.: On mechanics of functionally graded hard-magnetic soft beams. Int. J. Eng. Sci. 157, 103391 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  59. Chen, W., Wang, L., Yan, Z., Luo, B.: Three-dimensional large-deformation model of hard-magnetic soft beams. Compos. Struct. 266, 113822 (2021)

    Article  Google Scholar 

  60. Wu, S., Hamel, C.M., Ze, Q., Yang, F., Qi, H.J., Zhao, R.: Evolutionary algorithm-guided voxel-encoding printing of functional hard-magnetic soft active materials. Adv. Intell. Syst. 2(8), 2000060 (2020)

    Article  Google Scholar 

  61. Stoker, J.J.: Nonlinear elasticity. Gordon and Breach Science Publishers, New York (1968)

    MATH  Google Scholar 

  62. Zhao, R., Kim, Y., Chester, S.A., Sharma, P., Zhao, X.: Mechanics of hard-magnetic soft materials. J. Mech. Phys. Solids 124, 244–263 (2019)

    Article  MathSciNet  Google Scholar 

  63. Snowdon, J.C.: Vibration and Shock in Damped Mechanical System. Wiley, New York (1968)

    Google Scholar 

  64. Rothon, R.: Particulate-Filled Polymer Composites, pp. 361–362. Smithers Rapra Publishing, Shrewsbury (2003)

    Google Scholar 

Download references

Acknowledgements

The authors are grateful for the support from the National Natural Science Foundation of China (NSFC) through grant numbers 12072119, 11902120 and 11972167.

Author information

Authors and Affiliations

  1. Department of Engineering Mechanics, School of Aerospace Engineering, Huazhong University of Science and Technology, Wuhan, 430074, China

    Wei Chen, Lin Wang & Zerui Peng

  2. Hubei Key Laboratory for Engineering Structural Analysis and Safety Assessment, Wuhan, 430074, China

    Wei Chen, Lin Wang & Zerui Peng

Authors
  1. Wei Chen
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Lin Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Zerui Peng
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding authors

Correspondence to Lin Wang or Zerui Peng.

Ethics declarations

Conflict of interest

The authors have no conflict of interest.

Ethical standard

All procedures performed in studies involving human participants were in accordance with the ethical standards of the institutional and/or national research committee and with the 1964 Helsinki Declaration and its later amendments or comparable ethical standards.

Human and animal rights

This article does not contain any studies with animals performed by any of the authors.

Informed consent

Informed consent was obtained from all individual participants included in the study.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendix 1

Appendix 1

The convergence of the Galerkin discretization will be examined in this part. The evolution of the critical flow velocity υcr for various values of α as P increases from 0 to 15 is given in Fig. 

Fig. 18
figure 18

Convergence test of the Galerkin discretization for a the critical flow velocity of stability analysis and b the bifurcation diagram of nonlinear vibration analysis of the fluid-conveying HMS pipe, where S = 1, P = 10, α = π/3, β = 0.142, γ = 18.9 and μ = 5 × 10–3 are employed

Full size image

18a. Note that the results of N = 3 and N = 4 are shown. It can be seen that the values of υcr obtained by using N = 3 agree very well with that obtained by using N = 4. Furthermore, Fig. 18b shows the bifurcation diagrams of θ1 for N = 3 and 4 when S = 1, P = 10, α = π/3, β = 0.142, γ = 18.9 and μ = 5 × 10–3. It can be found that there is a good agreement between the bifurcation diagram by using N = 3 and the counterpart by using N = 4. Therefore, the Galerkin discretization of N = 3 is valid for the problem at hand and all the results given in Sect. 3 are obtained by using N = 3.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Chen, W., Wang, L. & Peng, Z. A magnetic control method for large-deformation vibration of cantilevered pipe conveying fluid. Nonlinear Dyn 105, 1459–1481 (2021). https://doi.org/10.1007/s11071-021-06662-2

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-021-06662-2

Keywords

Search

Navigation