Saturation-based active controller for vibration suppression of a four-degree-of-freedom rotor–AMB system

Abstract

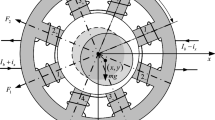
In this paper, a four-degree-of-freedom (4-DOF) rotor–AMB system including saturation-based active controller is considered that is used to suppress the vibrations of the rotor–AMB system at primary resonance excitation and the presence of 1:1 and 1:2 internal resonances. We obtained an approximate solution applying the multiple scales perturbation method. Then we conducted bifurcation analyses for both the open and closed loop systems. The stability of the system is investigated applying the Lyapunov first method. The effects of different controller parameters on the main system’s behavior are studied. Optimum working conditions of the system are extracted to be used in the design of such systems. Finally, numerical simulations are performed to validate the saturation control law. We found that all predictions from analytical solutions are in close agreement to the numerical simulation. At the end of the work, a comparison with the available published work is included.

Appendix

Introducing nondimensional parameters $u = c_{0}\hat{u}$, $v = c_{0}\hat{v}$, $i_{u} = I_{0}\hat{i}_{u}$, $i_{v} = I_{0}\hat{i}_{v}$, and $t = \zeta\hat{t}$, $\varOmega= \zeta^{ - 1}\hat{\varOmega}$, omitting Hat for brevity, Eqs. (5) can be rearranged as

$$\begin{aligned} f_{u} &= \frac{I_{0}^{2}\mu_{0}N^{2}A\cos(\phi )}{4c_{0}^{2}} \bigl[ - 8\zeta c_{0}k_{d} \cos(\alpha)\dot{u} \\ &\quad+ 8 \bigl( 1 - c_{0}k_{p}\cos(\alpha ) \bigr)u + \bigl( 16 \bigl( \cos^{4}(\alpha) \\ &\quad+ \sin^{4}( \alpha) \bigr) - 24c_{0}k_{p}\cos^{3}(\alpha) \\ &\quad+ 8c_{0}^{2}k_{p}^{2}\cos^{2}( \alpha) \bigr)u^{3} + \bigl( 8c_{0}^{2}k_{p}^{2} \sin^{2}(\alpha) \\ &\quad- 72c_{0}k_{p}\cos(\alpha) \sin^{2}(\alpha) \\ &\quad+ 96\cos^{2}(\alpha)\sin^{2}( \alpha) \bigr)uv^{2} + \bigl( 16c_{0}^{2}\zeta k_{p}k_{d}\cos^{2}(\alpha) \\ &\quad- 24\zeta c_{0}k_{d}\cos^{3}(\alpha) \bigr)u^{2} \dot{u} \\ &\quad- \bigl( 24\zeta c_{0}K_{d}\cos(\alpha) \sin^{2}(\alpha) \bigr)\dot{u}v^{2} \\ &\quad+ 8\zeta^{2}c_{0}^{2}k_{d}^{2} \sin^{2}(\alpha)u\dot{v}^{2} + 8\zeta^{2}c_{0}^{2}k_{d}^{2} \cos^{2}(\alpha)u\dot{u}^{2} \\ &\quad+ \bigl( - 48\zeta c_{0}k_{d}\cos(\alpha)\sin^{2}(\alpha) \\ &\quad+ 16 \zeta c_{0}^{2}k_{d}k_{p} \sin^{2}(\alpha) \bigr)uv\dot{v} \\ &\quad+ \bigl( 8c_{0}^{2} \psi_{1}\cos(\alpha)x^{2} \bigr) \bigr] \end{aligned}$$

(64)

$$\begin{aligned} f_{v} &= \frac{I_{0}^{2}\mu_{0}N^{2}A\cos(\phi )}{4c_{0}^{2}} \bigl[ - 8\zeta c_{0}k_{d} \cos(\alpha)\dot{v} \\ &\quad+ 8 \bigl( 1 - c_{0}k_{p}\cos(\alpha ) \bigr)v \\ &\quad+ \bigl( 16 \bigl( \cos^{4}(\alpha) + \sin^{4}( \alpha) \bigr) - 24c_{0}k_{p}\cos^{3}(\alpha) \\ &\quad+ 8c_{0}^{2}k_{p}^{2}\cos^{2}( \alpha) \bigr)v^{3} + \bigl( 8c_{0}^{2}k_{p}^{2} \sin^{2}(\alpha) \\ &\quad- 72c_{0}k_{p}\cos(\alpha) \sin^{2}(\alpha) \\ &\quad+ 96\cos^{2}(\alpha)\sin^{2}( \alpha) \bigr)vu^{2} + \bigl( 16c_{0}^{2}\zeta k_{p}k_{d}\cos^{2}(\alpha) \\ &\quad- 24\zeta c_{0}k_{d}\cos^{3}(\alpha) \bigr)v^{2} \dot{v} \\ &\quad- \bigl( 24\zeta c_{0}K_{d}\cos(\alpha) \sin^{2}(\alpha) \bigr)\dot{v}u^{2} \\ &\quad + 8\zeta^{2}c_{0}^{2}k_{d}^{2} \sin^{2}(\alpha)v\dot{u}^{2} + 8\zeta^{2}c_{0}^{2}k_{d}^{2} \cos^{2}(\alpha)v\dot{v}^{2} \\ &\quad + \bigl( - 48\zeta c_{0}k_{d}\cos(\alpha)\sin^{2}(\alpha) \\ &\quad+ 16 \zeta c_{0}^{2}k_{d}k_{p} \sin^{2}(\alpha) \bigr)vu\dot{u} + \bigl( 8c_{0}^{2} \psi_{2}\cos(\alpha)y^{2} \bigr) \bigr] \end{aligned}$$

(65)

We can rewrite Eq. (7) as

$$\begin{aligned} &c_{0}\zeta^{2}\ddot{u} - \frac{1}{m}f_{u} + \frac{cc_{0}\zeta}{m}\dot{u} = e\cos(\varOmega t) \end{aligned}$$

(66)

$$\begin{aligned} &c_{0}\zeta^{2}\ddot{v} - \frac{1}{m}f_{v} + \frac{cc_{0}\zeta}{m}\dot{v} = e\sin(\varOmega t) \end{aligned}$$

(67)

Substituting Eq. (64) into (66) and (65) into (67), respectively, we get

$$\begin{aligned} &\ddot{u} - \frac{I_{0}^{2}\mu_{0}N^{2}A\cos(\phi )}{4mc_{0}^{3}\zeta^{2}} \bigl[ - 8\zeta c_{0}k_{d} \cos(\alpha)\dot{u} \\ &\qquad+ 8 \bigl( 1 - c_{0}k_{p}\cos(\alpha ) \bigr)u + \bigl( 16 \bigl( \cos^{4}(\alpha) \\ &\qquad+ \sin^{4}( \alpha) \bigr) - 24c_{0}k_{p}\cos^{3}(\alpha) + 8c_{0}^{2}k_{p}^{2}\cos^{2}( \alpha) \bigr)u^{3} \\ &\qquad+ \bigl( 8c_{0}^{2}k_{p}^{2} \sin^{2}(\alpha) - 72c_{0}k_{p}\cos(\alpha) \sin^{2}(\alpha) \\ &\qquad+ 96\cos^{2}(\alpha)\sin^{2}( \alpha) \bigr)uv^{2} + \bigl( 16c_{0}^{2}\zeta k_{p}k_{d}\cos^{2}(\alpha) \\ &\qquad- 24\zeta c_{0}k_{d}\cos^{3}(\alpha) \bigr)u^{2} \dot{u} \\ &\qquad- \bigl( 24\zeta c_{0}K_{d}\cos(\alpha) \sin^{2}(\alpha) \bigr)\dot{u}v^{2} \\ &\qquad+ 8\zeta^{2}c_{0}^{2}k_{d}^{2} \sin^{2}(\alpha)u\dot{v}^{2} + 8\zeta^{2}c_{0}^{2}k_{d}^{2} \cos^{2}(\alpha)u\dot{u}^{2} \\ &\qquad+ \bigl( - 48\zeta c_{0}k_{d}\cos(\alpha)\sin^{2}(\alpha) \\ &\qquad+ 16 \zeta c_{0}^{2}k_{d}k_{p} \sin^{2}(\alpha) \bigr)uv\dot{v} \\ &\qquad+ \bigl( 8c_{0}^{2} \psi_{1}\cos(\alpha)x^{2} \bigr) \bigr] + \frac{c}{m\zeta} \dot{u} \\ &\quad = \frac{e}{c_{0}\zeta^{2}}\cos(\varOmega t) \end{aligned}$$

(68)

$$\begin{aligned} & \ddot{v} - \frac{I_{0}^{2}\mu_{0}N^{2}A\cos(\phi )}{4mc_{0}^{3}\zeta^{2}} \bigl[ - 8\zeta c_{0}k_{d} \cos(\alpha)\dot{v} \\ &\qquad+ 8 \bigl( 1 - c_{0}k_{p}\cos(\alpha ) \bigr)v + \bigl( 16 \bigl( \cos^{4}(\alpha) \\ &\qquad+ \sin^{4}( \alpha) \bigr) - 24c_{0}k_{p}\cos^{3}(\alpha) \\ &\qquad+ 8c_{0}^{2}k_{p}^{2}\cos^{2}( \alpha) \bigr)v^{3} + \bigl( 8c_{0}^{2}k_{p}^{2} \sin^{2}(\alpha) - 72c_{0}k_{p} \\ &\qquad\times\cos(\alpha) \sin^{2}(\alpha) + 96\cos^{2}(\alpha)\sin^{2}( \alpha) \bigr)vu^{2} \\ &\qquad+ \bigl( 16c_{0}^{2}\zeta k_{p}k_{d}\cos^{2}(\alpha) - 24\zeta c_{0}k_{d}\cos^{3}(\alpha) \bigr)v^{2} \dot{v} \\ &\qquad- \bigl( 24\zeta c_{0}K_{d}\cos(\alpha) \sin^{2}(\alpha) \bigr)\dot{v}u^{2} + 8\zeta^{2}c_{0}^{2}k_{d}^{2} \\ &\qquad\times \sin^{2}(\alpha)v\dot{u}^{2} + 8\zeta^{2}c_{0}^{2}k_{d}^{2} \cos^{2}(\alpha)v\dot{v}^{2} \\ &\qquad+ \bigl( - 48\zeta c_{0}k_{d}\cos(\alpha)\sin^{2}(\alpha) \\ &\qquad+ 16 \zeta c_{0}^{2}k_{d}k_{p} \sin^{2}(\alpha) \bigr)vu\dot{u} + \bigl( 8c_{0}^{2} \psi_{2}\cos(\alpha)y^{2} \bigr) \bigr] \\ &\qquad+ \frac{c}{m\zeta} \dot{v} \\ &\quad= \frac{e}{c_{0}\zeta^{2}}\sin(\varOmega t) \end{aligned}$$

(69)

Putting $\zeta^{2} = \frac{I_{0}^{2}\mu_{0}N^{2}A\cos(\phi )}{4mc_{0}^{3}}$, p=c ₀ k _p , d=ζc ₀ k _d , $c_{1} = \frac{c}{m\zeta}$, $f = \frac{e}{c_{0}\zeta^{2}}$ at Eqs. (68) and (69), we get

$$\begin{aligned} &\ddot{u} + 2\mu\dot{u} + \omega u - \bigl(\alpha_{1}u^{3} + \alpha_{2}uv^{2} \\ &\qquad+ \alpha_{3}u^{2} \dot{u} + \alpha_{4}\dot{u}v^{2} + \alpha_{5}u \dot{v}^{2} + \alpha_{6}u\dot{u}^{2} + \alpha_{7}uv\dot{v}\bigr) \\ &\quad= f\cos(\varOmega t) + \gamma_{1}x^{2} \end{aligned}$$

(70)

$$\begin{aligned} &\ddot{v} + 2\mu\dot{v} + \omega v - \bigl(\alpha_{1}v^{3} + \alpha_{2}vu^{2} + \alpha_{3}v^{2} \dot{v} + \alpha_{4}\dot{v}u^{2} \\ &\qquad+ \alpha_{5}v \dot{u}^{2} + \alpha_{6}v\dot{v}^{2} + \alpha_{7}vu\dot{u}\bigr) \\ &\quad= f\sin(\varOmega t) + \gamma_{2}y^{2} \end{aligned}$$

(71)

where

$$\begin{aligned} &2\mu= c_{1} + 8d\cos(\alpha),\qquad \omega= 8 \bigl( p\cos (\alpha) - 1 \bigr) \\ & \begin{aligned}[t] \alpha_{1} &= 16\bigl(\cos^{4}(\alpha) + \sin^{4}(\alpha)\bigr) - 24p\cos^{3}(\alpha) \\ &\quad+ 8p^{2}\cos^{2}(\alpha) \end{aligned} \\ & \begin{aligned}[t] \alpha_{2} &= 8p^{2}\sin^{2}(\alpha) - 72p\cos( \alpha)\sin^{2}(\alpha)\\ &\quad + 96\cos^{2}(\alpha) \sin^{2}(\alpha) \end{aligned} \\ &\alpha_{3} = 16pd\cos^{2}(\alpha) - 24d\cos^{3}( \alpha) \\ & \alpha_{4} = - 24d\cos(\alpha)\sin^{2}(\alpha),\qquad \alpha_{5} = 8d^{2}\sin^{2}(\alpha) \\ & \alpha_{6} = 8d^{2}\cos^{2}(\alpha),\\ & \alpha_{7} = - 48d\cos(\alpha)\sin^{2}(\alpha) + 16pd \sin^{2}(\alpha) \\ &\gamma_{1} = 8c_{0}^{2}\psi_{1}\cos( \alpha),\qquad \gamma_{2} = 8c_{0}^{2}\psi_{2} \cos(\alpha) \end{aligned}$$