Skip to main content
SpringerLink
Log in

Nonlinear modes of cylindrical panels with complex boundaries. R-function method

  • Published:
Meccanica Aims and scope Submit manuscript

Abstract

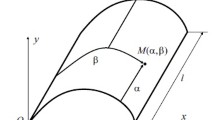
Nonlinear vibrations of cylindrical panels with complex base are analyzed. The Donnell-Mushtari-Vlasov equations with respect to displacements are used to study vibrations of shallow shell with geometrical nonlinearity. R-function method is applied to satisfy the panel boundary conditions. The Rayleigh-Ritz method is used to obtain the linear vibrations eigenmodes, which contain R-function. The nonlinear vibrations of panel are expanded by using these eigenmodes. The harmonic balance method and nonlinear normal modes are used to study the free nonlinear vibrations.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Grigolyuk EI (1955) Vibrations of circular cylindrical panels subjected to finite deflection. Prikl Mat Meh 19:376–382. (In Russian)

    MATH  Google Scholar 

  2. Cummings BE (1964) Large-amplitude vibration and response of curved panels. AIAA J 2:709–716

    Article  Google Scholar 

  3. Leissa AW, Kadi AS (1971) Curvature effects on shallow shell vibrations. J Sound Vib 16:173–187

    Article  ADS  MATH  Google Scholar 

  4. Vol’mir AS, Logvinskaya AA, Rogalevich VV (1973) Nonlinear natural vibrations of rectangular plates and cylindrical panels. Sov Phys Dokl 17:720–721

    ADS  Google Scholar 

  5. Amabili M (2005) Non-linear vibrations of doubly curved shallow shells. Int J Non-Linear Mech 40:683–710

    Article  MATH  Google Scholar 

  6. Amabili M (2005) Nonlinear vibrations of circular cylindrical panels. J Sound Vib 281:509–535

    Article  ADS  Google Scholar 

  7. Amabili M (2006) Theory and experiments for large-amplitude vibrations of circular cylindrical panels with geometric imperfections. J Sound Vib 298:43–72

    Article  ADS  Google Scholar 

  8. Popov AA, Thompson JMT, Croll JGA (1998) Bifurcation analyses in the parametrically excited vibrations of cylindrical panels. Nonlinear Dyn 17:205–225

    Article  MATH  MathSciNet  Google Scholar 

  9. Baumgarten R, Kreuzer E (1996) Bifurcations and subharmonic resonances in multi-degree-of-freedom panel’s models. Meccanica 31:309–322

    Article  MATH  MathSciNet  Google Scholar 

  10. Zhang W, Liu Z, Yu P (2001) Global dynamics of a parametrically excited thin plate. Nonlinear Dyn 24:245–268

    Article  MATH  MathSciNet  Google Scholar 

  11. Anlas G, Elbeyli O (2002) Nonlinear vibrations of a simply supported rectangular metallic plate subjected to transverse harmonic excitation in the presence of a one-to-one internal resonance. Nonlinear Dyn 30:1–28

    Article  MATH  Google Scholar 

  12. Amabili M, Paıdoussis MP (2003) Review of studies on geometrically nonlinear vibrations and dynamics of circular cylindrical shells and panels, with and without fluid-structure interaction. Appl Mech Rev 56:349–381

    Article  ADS  Google Scholar 

  13. Rvachev VL (1982) Theory R-function and its application. Naukova Dumka, Kiev

    Google Scholar 

  14. Sheiko TI, Shapiro V, Tsukanov I (2000) On completeness of RFM solutions structure. Comput Mech 25:305–316

    Article  MATH  MathSciNet  Google Scholar 

  15. Kurpa L, Pilgun G, Amabili M (2007) Nonlinear vibrations of shallow shells with complex boundary: R-functions methods and experiments. J Sound Vib 306:580–600

    Article  ADS  Google Scholar 

  16. Kurpa LV, Rvachev VL, Ventsel E (2003) The R-function method for the free vibration analysis of thin orthotropic plates of arbitrary shape. J Sound Vib 261:109–122

    Article  ADS  Google Scholar 

  17. Kurpa LV, Pilgun G, Ventsel E (2005) Application of the R-function method to nonlinear vibrations of thin plates of arbitrary shape. J Sound Vib 284:379–392

    Article  ADS  Google Scholar 

  18. Kurpa LV, Lyubitska KI, Shmatko AV (2005) Solution of vibration problems for shallow shells of arbitrary form by the R-function method. J Sound Vib 279:1071–1084

    Article  ADS  Google Scholar 

  19. Vol’mir AS (1972) Nonlinear dynamics of plates and shells. Nauka, Moscow. (In Russian)

    Google Scholar 

  20. Avramov KV, Pellicano F (2007) Linear and nonlinear dynamics of a circular cylindrical shell connected to a rigid disk. Commun Nonlinear Sci Numer Simul 12:496–518

    Article  ADS  MATH  Google Scholar 

  21. Rvachev VL, Shevchenko AN, Veretel’nik VV (1994) Numerical integration software for projection and projection-grid methods. Cybern Syst Anal 30(1):154–158

    Article  MathSciNet  Google Scholar 

  22. Luft B, Shapiro V, Tsukanov I (2008) Geometrically adaptive numerical integration. In: Proceedings of the ACM solid and physical modeling symposium (SPM 2008), pp 147–158

  23. Wolfram S (1996) The mathematica book. Cambridge University Press, Cambridge

    MATH  Google Scholar 

  24. Avramov KV, Pierre C, Shyriaieva N (2007) Flexural-flexural-torsional nonlinear vibrations of pre-twisted rotating beams with asymmetric cross-section. J Vib Control 13:329–364

    Article  MATH  MathSciNet  Google Scholar 

  25. Avramov KV, Mikhlin YV, Kurilov G (2007) Asymptotic analysis of nonlinear dynamics of simply supported cylindricall shells. Nonlinear Dyn 47:331–352

    Article  MATH  MathSciNet  Google Scholar 

  26. Vakakis A, Manevich LI, Mikhlin YuV, Pilipchuk VN, Zevin AA (1996) Normal modes and localization in nonlinear systems. Wiley, New York

    Book  MATH  Google Scholar 

  27. Shaw SW, Pierre C (1993) Normal modes for nonlinear vibratory systems. J Sound Vib 164:58–124

    Article  MathSciNet  Google Scholar 

  28. Shaw SW, Pierre C, Pesheck E (1999) Modal analysis-based reduced-order models for nonlinear structure—an invariant manifolds approach. Shock Vib Dig 31:3–16

    Article  Google Scholar 

  29. Avramov KV (2008) Analysis of forced vibrations by nonlinear modes. Nonlinear Dyn 53:117–127

    Article  MATH  MathSciNet  Google Scholar 

  30. Jiang D, Pierre C, Shaw SW (2005) The construction of non-linear normal modes for systems with internal resonance. Int J Non-Linear Mech 40:729–746

    Article  MATH  Google Scholar 

  31. Parker TS, Chua LO (1989) Practical numerical algorithms for chaotic systems. Springer, New York

    Book  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Nonstationary Vibrations, A.N. Podgorny Institute for Mechanical Engineering Problems, National Academy of Sciences of Ukraine, 2/10 Dm. Pozharskoho St., 61046, Kharkiv, Ukraine

    I. D. Breslavsky & K. V. Avramov

Authors
  1. I. D. Breslavsky
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. K. V. Avramov
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to K. V. Avramov.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Breslavsky, I.D., Avramov, K.V. Nonlinear modes of cylindrical panels with complex boundaries. R-function method. Meccanica 46, 817–832 (2011). https://doi.org/10.1007/s11012-010-9340-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11012-010-9340-x

Keywords

Search

Navigation