Skip to main content
SpringerLink
Log in

Exact solutions in MHD natural convection of a Bingham fluid: fully developed flow in a vertical channel

  • Published:
Journal of Thermal Analysis and Calorimetry Aims and scope Submit manuscript

Abstract

In nature, many fluid-like materials exhibit a yield stress below which they behave like a solid. The Bingham model aims to describe such materials. This paper draws some mathematical considerations on the flow of a Bingham fluid in a vertical channel. The situation due to the presence of an external magnetic field and natural convection is analyzed: the external magnetic field, which is orthogonal to the walls of the channel, generates the Lorentz forces that influence the motion through the Hartmann number. The behavior of the velocity, the induced magnetic field and the thickness of the plug regions are discussed and presented graphically. We find that the velocity is a decreasing function of the Bingham and Hartmann numbers. In particular, the presence of the external magnetic field increases the thickness of the plug region. The modulus of the induced magnetic field is not monotone when the Hartmann number changes, but it is a decreasing function of the Bingham number.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14

Similar content being viewed by others

Abbreviations

b :

Thermal diffusivity      (\(\hbox{m}^2 \,{\rm s}^{-1}\))

B :

Bingham number (dimensionless parameter)

C :

Constant such that \(P^*\) or \(p^* \,=\,-Cx_1 +p_0\)    \({\text{(Nm}}^{{ - 3}} )\)

\(\widetilde{C}_0\) :

Integration constant     (\(\hbox{N}\, \hbox{m}^{-2}\))

\(C_0\) :

Dimensionless integration constant

\(\mathbf{D}\) :

Stretching tensor     (\(\hbox{s}^{-1}\))

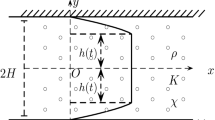
2d :

Distance between the walls     (m)

\({\mathbf{E}}\) :

Electric field     (\(\hbox{V}\, \hbox{m}^{-1}\)), V = volt

\((\mathbf{e}_1,\mathbf{e}_2,\mathbf{e}_3)\) :

Orthonormal basis of \({{\mathbb {R}}}^3\)     (dimensionless)

\(\mathbf{g}\) :

Gravity acceleration     (\(\hbox{m}\, \hbox{s}^{-2}\))

\(\mathbf{H}\) :

Total magnetic field     (\(\hbox{A}\, \hbox{m}^{-1}\)),

h(y):

Dimensionless function describing induced magnetic field

\(H_0\mathbf{e_2}\) :

External uniform magnetic field     (\(\hbox{A}\, \hbox{m}^{-1}\))

\(H_1\mathbf{e_1}\) :

Induced magnetic field     (\(\hbox{A}\, \hbox{m}^{-1}\))

\(\mathbf{I}\) :

Identity tensor     (dimensionless)

k :

Thermal conductivity     (\(\hbox{W}\, \hbox{m}^{-1} \hbox{K}^{-1}\))

\(k_{1,2}\) :

Heat transfer coefficients at \(\Pi _{1,2}\)    (\(\hbox{W}\, \hbox{m}^{-2} \hbox{K}^{-1}\))

M :

Hartmann number     (dimensionless parameter)

\(Nu_{1,2}\) :

Nusselt numbers at the walls \(\Pi _{1,2}\)     (dimensionless parameters)

p :

Pressure     (\(\hbox{N}\,\hbox{m}^{-2}\))

\(p^*\) :

Modified pressure in the absence of an external magnetic field     (\(\hbox{N}\,\hbox{m}^{-2}\))

\(P^*\) :

Modified pressure in the presence of an external magnetic field     (\(\hbox{N}\,\hbox{m}^{-2}\))

\(p_0\) :

Some constant     (\(\hbox{N}\,\hbox{m}^{-2}\)

\({\mathbf{{q}}}\) :

Heat flux vector     (\(\hbox{W}\, \hbox{m}^{-2}\))

T :

Temperature     (K)

\(\mathbf{T}\) :

Stress tensor     (\(\hbox{N}\, \hbox{m}^{-2}\))

\({\mathbf{T}}^\text{D}\) :

Deviatoric part of \(\mathbf{T}\)     (\(\hbox{N}\, \hbox{m}^{-2}\))

\(T^\text{D}_\text{ij}\) :

Components of \({\mathbf{T}}^\text{D}\)    (\(\hbox{N}\, \hbox{m}^{-2}\))

\(t_{12}\) :

Dimensionless component of \({\mathbf{T}}^\text{D}\)

\(T_0\) :

Reference temperature     (K)

\(T_{1,2}\) :

Uniform temperatures at the walls \(\Pi _{1,2}\)     \((T_2 > T_1)\) (K)

\(\mathbf{v}\) :

Velocity field     (\(\hbox{m}\,\hbox{s}^{-1}\))

v :

Dimensionless velocity

\(V_0\) :

Characteristic velocity     (\(\hbox{m}\,\hbox{s}^{-1}\))

\(v_1\) :

Velocity component in \(x_1-\)direction    (\(m\,s^{-1}\))

\(x_1,\,x_2,\,x_3\) :

Cartesian coordinates     (m)

y :

Dimensionless transverse coordinate

\(y_{0,1,2}\) :

Dimensionless boundaries of the plug regions.

\(\alpha\) :

Thermal expansion coefficient     (\(\hbox{K}^{-1}\))

\(\eta _\text{e}\) :

Magnetic diffusivity \(\displaystyle \left( \eta _\text{e}=\frac{1}{\mu _\text{e}\sigma _\text{e}}\right)\)     (\(\hbox{m}^2\,\hbox{s}^{-1}\))

\(\vartheta\) :

Dimensionless temperature

\({\mu}\) :

Dynamic viscosity coefficient    (\(\hbox{N}\,\hbox{m}^{-2}\,\hbox{s}\))

\({\mu _\text{e}}\) :

Magnetic permeability     (\(\hbox{H}\, \hbox{m}^{-1}\))

\(\rho _0\) :

Mass density at the temperature \(T_0\)     (\(\hbox{Kg}\,\hbox{m}^{-3}\))

\(\sigma _\text{e}\) :

Electrical conductivity     (\(\hbox{S}\,\hbox{m}^{-1}\))

\(\tau\) :

Yield stress    (\(\hbox{N}\, \hbox{m}^{-2}\))

\({\varvec{\tau }}_{1,2}\) :

Skin frictions at the walls \(\Pi _{1,2}\)    (\(\hbox{N}\, \hbox{m}^{-2}\))

References

  1. Bingham E. C., Fluidity and Plasticity, McGraw-Hill Book Company, Inc., 1922.

  2. Obando B., Takahashi T., Existence of weak solutions for a Bingham fluid-rigid body system, Ann. I. H. Poincar\(\acute{e}\) 36 (2019) 1281-1309.

  3. Patel N, Ingham DB. Analytic solution for the mixed convection flow of non-Newtonian fluids in parallel plate ducts. Int Comm Heat Mass Trans. 1994;21:75–84.

    Article  CAS  Google Scholar 

  4. Nouar C, Frigaard IA. Nonlinear stability of Poiseuille flow of a Bingham fluid; theoretical results and comparison with phenomenological criteria. J Non-Newt Fluid Mech. 2001;100:127–49.

    Article  CAS  Google Scholar 

  5. Borrelli A, Patria MC, Piras E. Spatial decay estimate in the problem of entry flow for a Bingham fluid filling a pipe. Mat Comp Model. 2004;40:23–42.

    Article  Google Scholar 

  6. Barletta A, Mayari E. Buoyant Couette-Bingham flow between vertical parallel plates. Int J Thermal Sci. 2008;47:811–9.

    Article  CAS  Google Scholar 

  7. Chen YL, Zhu KQ. Couette-Poiseuille flow of Bingham fluids between two porous parallel plates with slip conditions. J Non-Newt Fluid Mech. 2008;153:1–11.

    Article  CAS  Google Scholar 

  8. Barletta A. Laminar mixed convection with viscous dissipation in a vertical channel. Int J Heat Mass Trans. 1998;41:3501–13.

    Article  CAS  Google Scholar 

  9. Borrelli A, Giantesio G, Patria MC. Magnetoconvection of a micropolar fluid in a vertical channel. Int J Heat Mass Transfer. 2015;80:614–25.

    Article  Google Scholar 

  10. Borrelli A, Giantesio G, Patria MC. Reverse Flow in Magnetoconvection of Two Immiscible Fluids in a Vertical Channel. J Fluid Eng. 2017;139:101203.

    Article  Google Scholar 

  11. Sheikholeslami M, et al. CuO nanomaterial two-phase simulation within a tube with enhanced turbulator. Powder Technol. 2020;373:1–13.

    Article  CAS  Google Scholar 

  12. Ho CJ, Liu Y-C, Ghambalaz M, Yan W-M. Forced convection heat transfer of nano-encapsulated phase change material (NEPCM) suspension in a mini-channel heatsink. Int J Heat Mass Trans. 2020;155:119858.

    Article  CAS  Google Scholar 

  13. Ho CJ, et al. Convective heat transfer of nano-encapsulated phase change material suspension in a divergent minichannel heatsink. Int J Heat Mass Trans. 2021;165:120717.

    Article  CAS  Google Scholar 

  14. Ghalambaz M, et al. Thermo-hydraulic performance analysis on the effects of truncated twisted tape inserts in a tube heat exchanger. Symmetry. 2020;12(10):1652.

    Article  CAS  Google Scholar 

  15. Ghalambaz M. Investigation of overlapped twisted tapes inserted in a double-pipe heat exchanger using two-phase nanofluid. Nanomaterials. 2020;10(9):1656.

    Article  CAS  Google Scholar 

  16. Yang W-J, Yeh H-C. Free convective flow of a Bingham plastic between two vertical plates. J Heat Trans. 1965;87:319–32.

    Article  Google Scholar 

  17. Bayazitoglu Y, Paslay PR, Cenocky P. Laminar Bingham fluid flow between vertical parallel plates. Int J Thermal Sci. 2007;46:349–57.

    Article  CAS  Google Scholar 

  18. Karimfazli I, Frigaard IA. Natural convection flows of a Bingham fluid in a long vertical channel. J Non-Newt Fluid Mech. 2013;201:39–55.

    Article  CAS  Google Scholar 

  19. Misra JC, Adhikary SD. Flow of Bingham fluid in a porous bed under the action of a magnetic field: application to magneto-hemorheology. Eng Sci Tech Int J. 2017;20:973–81.

    Google Scholar 

  20. Pourjafar M, Malmir F, Bazargan S, Sadeghy K. Magnetohydrodynamic flow of Bingham fluids in a plane channel: a theoretical study. J Non-Newton Fluid Mech. 2019;264:1–18.

    Article  CAS  Google Scholar 

  21. Sheikholeslami M, et al. Numerical simulation of wavy porous enclosure filled with hybrid nanofluid involving Lorentz effect. Phys Scr. 2020;95:115701.

    Article  CAS  Google Scholar 

  22. Shah Z, et al. Modeling of entropy optimization for hybrid nanofluid MHD flow through a porous annulus involving variation of Bejan number. Sci Rep. 2020;10:12821.

    Article  CAS  Google Scholar 

  23. Sheikholeslami M, et al. Lorentz force impact on hybrid nanofluid within a porous tank including entropy generation. Int Commun Heat Mass Transf. 2020;116:104635.

    Article  CAS  Google Scholar 

  24. Ghalambaz M, et al. Analysis of melting behavior of PCMs in a cavity subject to a non-uniform magnetic field using a moving grid technique. Appl Math Model. 2020;77:1936–53.

    Article  Google Scholar 

  25. Borrelli A, Giantesio G, Patria MC. Magnetoconvection of a micropolar fluid in a vertical channel. Int J Heat Mass Trans. 2015;80:614–25.

    Article  Google Scholar 

  26. Ferraro VCA, Plumpton A. An introduction to magneto-fluid mechanics. London-New York: Oxford University Press; 1961.

    Google Scholar 

  27. Rajagopal VR, Ruzicka MM, Srinivasa AR. On the Oberbeck-Boussinesq approximation. Math Models Methods Appl Sci. 1996;6:1157–67.

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Dipartimento di Matematica e Informatica, Università di Ferrara, via Machiavelli 30, 44121, Ferrara, Italy

    A. Borrelli & M. C. Patria

  2. Dipartimento di Matematica e Fisica, Università Cattolica del Sacro Cuore, via Musei 41, 25121, Brescia, Italy

    G. Giantesio

Authors
  1. A. Borrelli
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. G. Giantesio
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. M. C. Patria
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to G. Giantesio.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Borrelli, A., Giantesio, G. & Patria, M.C. Exact solutions in MHD natural convection of a Bingham fluid: fully developed flow in a vertical channel. J Therm Anal Calorim 147, 5825–5838 (2022). https://doi.org/10.1007/s10973-021-10882-4

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10973-021-10882-4

Keywords

Mathematics Subject Classification

Search

Navigation