Skip to main content
SpringerLink
Log in

On dual-phase-lag magneto-thermo-viscoelasticity theory with memory-dependent derivative

  • Technical Paper
  • Published:
Microsystem Technologies Aims and scope Submit manuscript

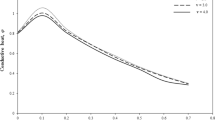
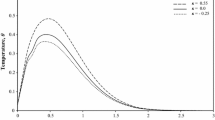
Abstract

A new mathematical model of generalized magneto-thermo-viscoelasticity theories with memory-dependent derivatives (MDD) of dual-phase-lag heat conduction law is developed. The equations for one-dimensional problems including heat sources are cast into matrix form using the state space and Laplace transform techniques. The resulting formulation is applied to a problem for the whole space with a plane distribution of heat sources. It is also applied to a perfect conducting semi-space problem with a traction-free surface and plane distribution of heat sources located inside the medium. The inversion of the Laplace transforms is carried out using a numerical approach. Numerical results for the temperature, displacement, stress and heat flux distributions as well as the induced magnetic and electric fields are given and illustrated graphically. A comparison is made with the results obtained in the coupled theory. The impacts of the MDD heat transfer parameter and Alfven velocity on a viscoelastic material, for example, poly (methyl methacrylate) (Perspex) are discussed.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

Abbreviations

t :

Time

\( \varvec{x} \) :

Space coordinates

\( u_{i} \) :

Components of displacement vector

\( q_{i} \) :

Components of heat flux

\( e_{ij} \) :

Components of strain tensor

C o :

\( \sqrt {\frac{\lambda \, + \,\;2\,\mu }{\rho }} \), Speed of propagation of isothermal elastic waves

\( K_{o} \) :

\( \lambda \; + (2/3)\,\mu \), bulk modulus

\( C_{E} \) :

Specific heat at constant strain

\( \sigma_{o} \) :

The electrical conductivity

\( \varvec{H} \) :

Magnetic intensity vector

\( \varvec{E} \) :

Electric intensity vector

\( \mu_{o} \) :

Magnetic permeability

\( \varepsilon_{o} \) :

Electric permeability

\( \varvec{B} \) :

\( \mu_{o} \varvec{H}, \), magnetic induction vector

J :

Conduction current density vector

\( e \) :

Dilatation

\( k \) :

Thermal conductivity

\( T \) :

Absolute thermodynamic temperature

\( T_{ \circ } \) :

Reference temperature

\( \vartheta \) :

\( T - T_{o} \); \( \left| {\,\frac{\vartheta }{{T_{o} }}} \right| \ll 1 \)

\( \gamma \) :

\( (3\lambda + 2\mu )\alpha_{T} \)

\( \delta (.) \) :

Dirac delta function

\( \delta_{ij} \) :

Kronecker’s delta

\( \varepsilon \) :

Thermal coupling parameter

\( \varepsilon_{ij} \) :

Components of strain tensor

\( \sigma_{ij} \) :

Components of stress tensor

\( S_{ij} \) :

Components of stress deviator tensor

\( \lambda ,\;\mu \) :

Lame’ constants

\( \rho \) :

Mass Density

\( \eta_{o} \) :

\( \frac{{\rho \,C_{E} }}{k} \)

\( \varepsilon \) :

\( \frac{{ \, \gamma^{2} T_{o} }}{{k\eta_{o} \rho C_{o}^{2} }} \), Thermal coupling parameter

\( \alpha_{o} \) :

\( \sqrt {\frac{{\mu_{o} \,H_{o}^{2} }}{\rho }} \), Alfven velocity

\( \tau \) :

Relaxation time

\( \tau_{q} ,\tau_{\theta } \) :

Phase-lags

\( {\varGamma (}\text{.)} \) :

Gamma function

Q :

Strength of applied heat source per unit mass

References

  • Abbas IA (2015) Eigenvalue approach to fractional order generalized magneto-thermoelastic medium subjected to moving heat source. J Magn Magn Mater 377:452–459

    Article  Google Scholar 

  • Abbas IA, Kumar R (2016) 2D Deformation in initially stressed thermoelastic half-space with voids. Steel Compos Struct 20:1103–1117

    Article  Google Scholar 

  • Adolfsson K, Enelund M, Olsson P (2005) On the fractional order model of viscoelasticity. Mech Time-Depend Mater 9:15–34

    Article  Google Scholar 

  • Anwar M, Sherief HH (1993) State space formulation for generalized thermoelasticity with one relaxation time including heat sources. J Therm Stress 16:163–180

    Article  MathSciNet  Google Scholar 

  • Atkinson C, Craster RV (1995) Theoretical aspects of fracture mechanics. Prog Aerospace Sci 31:1–83

    Article  Google Scholar 

  • Bartle RG, Sherbert DR (2000) Introduction to real analysis, 209, 3rd edn. Wiley, New York

    MATH  Google Scholar 

  • Biot M (1956) Thermoelasticity and irreversible thermo-dynamics. J Appl Phys 27:240–253

    Article  MathSciNet  MATH  Google Scholar 

  • Caputo M (1974) Vibrations of an infinite viscoelastic layer with a dissipative memory. J Acoust Soc Am 56:897–904

    Article  MATH  Google Scholar 

  • Caputo M, Mainardi F (1971) Linear model of dissipation in an elastic solids. Riv Nuovo Cimento 1:161–198

    Article  Google Scholar 

  • Chandrasekharaiah D (1998) Hyperbolic thermoelasticity: a review of recent literature. Appl Mech Rev 51:705–729

    Article  Google Scholar 

  • El-Karamany AS, Ezzat MA (2002) On the boundary integral formulation of Thermo-viscoelasticity theory. Int J Eng Sci 40:1943–1956

    Article  MathSciNet  MATH  Google Scholar 

  • El-Karamany AS, Ezzat MA (2011a) On fractional thermoelastisity. Math Mech Solids 16:334–346

    Article  MathSciNet  MATH  Google Scholar 

  • El-Karamany AS, Ezzat MA (2011b) Convolutional variational principle, reciprocal and uniqueness theorems in linear fractional two-temperature thermoelasticity. J Therm Stress 34:264–284

    Article  Google Scholar 

  • Ezzat MA (2001) Free convection effects on perfectly conducting fluid. Int J Eng Sci 39:799–819

    Article  MATH  Google Scholar 

  • Ezzat MA (2006) The relaxation effects of the volume properties of electrically conducting viscoelastic material. Mater Sci Eng, B 130:11–23

    Article  Google Scholar 

  • Ezzat MA (2011a) Magneto-thermoelasticity with thermoelectric properties and fractional derivative heat transfer. Phys B 406:30–35

    Article  Google Scholar 

  • Ezzat MA (2011b) Thermoelectric MHD with modified Fourier’s law. Int J Therm Sci 50:449–455

    Article  Google Scholar 

  • Ezzat MA, Abd-Elaal MZ (1997) State space approach to viscoelastic fluid flow of hydromagnetic fluctuating boundary-layer through a porous medium. ZAMM 77:197–207

    Article  MathSciNet  MATH  Google Scholar 

  • Ezzat MA, Awad ES (2010) Constitutive relations, uniqueness of solution, and thermal shock application in the linear theory of micropolar generalized thermoelasticity involving two temperatures. J Therm Stress 33:226–250

    Article  Google Scholar 

  • Ezzat MA, El-Bary AA (2015) Memory-dependent derivatives theory of thermo-viscoelasticity involving two-temperature. J Mech Sci Tech 29:4273–4279

    Article  Google Scholar 

  • Ezzat MA, El-Bary AA (2017a) Application of fractional order theory of magneto-thermoelasticity to an infinite perfect conducting body with a cylindrical cavity. Micro Sys Tech 23:2447–2458

    Article  Google Scholar 

  • Ezzat MA, El-Bary AA (2017b) Generalized fractional magneto-thermo-viscoelasticity. Micro Sys Tech 23:1767–1777

    Article  Google Scholar 

  • Ezzat MA, El-Bary AA (2017c) A functionally graded magneto-thermoelastic half space with memory-dependent derivatives heat transfer. Steel Compos Struct 25:177–186

    Google Scholar 

  • Ezzat MA, El-Karamany AS (2002) The uniqueness and reciprocity theorems for generalized thermo-viscoelasticity with two relaxation times. Int J Eng Sci 40:1275–1284

    Article  MathSciNet  MATH  Google Scholar 

  • Ezzat MA, El-Karamany AS (2011a) Theory of fractional order in electro-thermoelasticity. Eur J Mech A/Solids 30:491–500

    Article  MATH  Google Scholar 

  • Ezzat MA, El-Karamany AS (2011b) Fractional order heat conduction law in magneto-thermoelasticity involving two temperatures. ZAMP 62:937–952

    MathSciNet  MATH  Google Scholar 

  • Ezzat MA, El-Karamany AS, Samaan AA (2011a) State-space formulation to generalized thermoviscoelasticity with thermal relaxation. J Therm Stress 24:823–846

    Article  Google Scholar 

  • Ezzat MA, Othman MI, El-Karamany AS (2011b) State space approach to generalized thermo-viscoelasticity with two relaxation times. Int J Eng Sci 40:283–302

    Article  MathSciNet  Google Scholar 

  • Ezzat MA, El-Karamany AS, El-Bary AA (2014) Generalized thermo-viscoelasticity with memory-dependent derivatives. Int J Mech Sci 89:470–475

    Article  Google Scholar 

  • Ezzat MA, El-Karamany AS, El-Bary AA (2015) A novel magneto-thermoelasticity theory with memory-dependent derivative. J Electromag Waves Applic 29:1018–1031

    Article  Google Scholar 

  • Ezzat MA, El-Karamany AS, El-Bary AA (2016) Modeling of memory-dependent derivative in generalized thermoelasticity. Euro Phys J Plus 131:372

    Article  Google Scholar 

  • Ezzat MA, El-Karamany AS, El-Bary AA (2017) Thermoelectric viscoelastic materials with memory-dependent derivative. Smart Struct Sys 19:539–551

    Article  Google Scholar 

  • Gross B (1953) Mathematical structure of the theories of viscoelasticity. Hermann, Paris

    MATH  Google Scholar 

  • Hetnarski RB, Ignaczak J (1999) Generalized thermo-elasticity. J Therm. Stress 22:451–476

    Google Scholar 

  • Honig G, Hirdes U (1984) A method for the numerical inversion of the Laplace transform. J Comput Appl Math 10:113–132

    Article  MathSciNet  MATH  Google Scholar 

  • Ilioushin A, Pobedria B (1970) Fundamentals of the mathematical theory of thermal viscoelasticity. Nauka, Moscow In Russian

    Google Scholar 

  • Kumar R, Mukhopadhyay S (2009) Effects of three phase lags on generalized thermoelasticity for an infinite medium with a cylindrical cavity. J Therm Stress 32:1149–1165

    Article  Google Scholar 

  • Lata P, Kumar R, Sharma N (2016) Plane waves in an anisotropic thermoelastic. Steel Compos Struct 22:567–587

    Article  Google Scholar 

  • Lord H, Shulman Y (1967) A generalized dynamical theory of thermoelasticity. J Mech Phys Solid 15:299–309

    Article  MATH  Google Scholar 

  • Nowacki W (1959a) Two one-dimensional problem of the thermoelasticity. Arch Mech Stos 11:333–346

    MathSciNet  MATH  Google Scholar 

  • Nowacki W (1959b) Some dynamic problems of thermoelasticity. Arch Mech Stos 11:259–283

    MathSciNet  MATH  Google Scholar 

  • Ogata K (1967) State space analysis control system, vol 6. Prentice-Hall, Englewood Cliffs

    MATH  Google Scholar 

  • Povstenko Y (2009) Thermoelasticity that uses fractional heat conduction equation. J. Math Sci 162:296–305

    Article  MathSciNet  Google Scholar 

  • Povstenko Y (2011) Fractional Cattaneo-type equations and generalized thermoelasticity. J Therm Stress 34:97–114

    Article  Google Scholar 

  • Rajagopal KR, Saccomandi G (2007) On the Dynamics of non-linear viscoelastic solids with material moduli that depend upon pressure. Int J Eng Sci 45:41–54

    Article  MathSciNet  MATH  Google Scholar 

  • Roychoudhuri SK (2007) On a thermoelastic three-phase-lag model. J Therm Stress 30:231–238

    Article  Google Scholar 

  • Sherief HH, Raslan WE (2016) 2D problem for a long cylinder in the fractional theory of thermoelasticity. Latin Am J Solids Struct 13:1596–1613

    Article  Google Scholar 

  • Sherief HH, El-Sayed AM, Abd El-Latief AM (2010) Fractional order theory of Thermoelasticity. Int J Solids Struct 47:269–275

    Article  MATH  Google Scholar 

  • Sherief H, Allam M, El-Hagary M (2011) Generalized theory of thermo viscoelasticity and a half-space problem. Int J Thermophys 32:1271–1295

    Article  Google Scholar 

  • Sherief HH, El-Maghraby NM, Allam AA (2017) Stochastic thermal shock problem and study of wave propagation in the theory of generalized thermoelastic diffusion. Math Mech Solids 22:1767–1789

    Article  MathSciNet  MATH  Google Scholar 

  • Tanner R (1988) Engineering rheology. Oxford University Press, Oxford

    MATH  Google Scholar 

  • Tiwari R, Mukhopadhyay S (2018) Analysis of wave propagation in the presence of a continuous line heat source under heat transfer with memory dependent derivatives. Mat Mech Solids 23:820–834

    Article  MathSciNet  MATH  Google Scholar 

  • Tschegl NW (1997) Time dependence in material properties: an Overview. Mech Time-Depend Mater 1:3–31

    Article  Google Scholar 

  • Tzou DY (1995) A unified filed approach for heat conduction from macro to macro Scales. ASME Heat Transf 117:8–16

    Article  Google Scholar 

  • Tzou DY (1997) Macro- to microscale heat transfer: the lagging behavior. Taylor & Francis, Washington

    Google Scholar 

  • Wang JL, Li HF (2011) Surpassing the fractional derivative: concept of the memory-dependent derivative. Compu Math Applic 62:1562–1567

    Article  MathSciNet  MATH  Google Scholar 

  • Youssef HM, Abbas IA (2014) Fractional order generalized thermoelasticity with variable thermal conductivity. J Vibroeng 16:4077–4087

    Google Scholar 

  • Yu Y-J, Hu W, Tian X-G (2014) A novel generalized thermoelasticity model based on memory- dependent derivative. Int J Eng Sci 81:123–134

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The authors gratefully acknowledge the approval and the support of this research study by the Grant No. SCI-2017-1-8-F-7322 from the Deanship of Scientific Research in Northern Border University, Arar, KSA.

Author information

Authors and Affiliations

  1. Department of Mathematics, Faculty of Science, Northern Border University, Arar, Saudi Arabia

    Dalia A. Aldawody & Mohamed H. Hendy

  2. Department of Mathematics, Faculty of Science, Port Said University, Port Said, Egypt

    Dalia A. Aldawody

  3. Department of Mathematics, Faculty of Science, Al Arish University, Al Arish, Egypt

    Mohamed H. Hendy

  4. Department of Mathematic, Faculty of Education, Alexandria University, Alexandria, Egypt

    Magdy A. Ezzat

Authors
  1. Dalia A. Aldawody
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Mohamed H. Hendy
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Magdy A. Ezzat
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Magdy A. Ezzat.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Aldawody, D.A., Hendy, M.H. & Ezzat, M.A. On dual-phase-lag magneto-thermo-viscoelasticity theory with memory-dependent derivative. Microsyst Technol 25, 2915–2929 (2019). https://doi.org/10.1007/s00542-018-4194-6

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00542-018-4194-6

Search

Navigation