Skip to main content
Springer Nature Link
Log in

Dynamical characteristics of the tip vortex from a four-bladed rotor in hover

  • Research Article
  • Published:
Experiments in Fluids Aims and scope Submit manuscript

Abstract

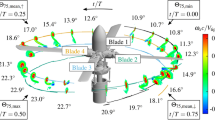
Dynamical characteristics of tip vortices shed from a 1 m diameter, four-bladed rotor in hover are investigated using various aperiodicity correction techniques. Data are acquired by way of stereo-particle image velocimetry and comprises measurements up to 260° vortex age with 10° offsets. The nominal operating condition of the rotor corresponds to Re c  = 248,000 and M = 0.23 at the blade tip. With the collective pitch set to 7.2° and a rotor solidity of 0.147, blade loading (C T/σ) is estimated from blade element momentum theory to be 0.042. The findings reveal a noticeable, anisotropic, aperiodic vortex wandering pattern over all vortex ages measured. These findings are in agreement with recent observations of a full-scale, four-bladed rotor in hover operating under realistic blade loading. The principal axis of wander is found to align itself perpendicular to the slipstream boundary. Likewise, tip vortices trailing from different blades show a wandering motion that is in phase instantaneously with respect to one another, in every direction and at every wake age in the measurement envelope.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16

Similar content being viewed by others

Explore related subjects

Discover the latest articles and news from researchers in related subjects, suggested using machine learning.

Abbreviations

b :

Number of blades

c :

Blade chord

C T :

Coefficient of thrust

d r :

Pixel dimension

d s :

Diffraction limited spot diameter

H ψ :

Normalized-helicity

l :

Turbulent length scale

L m :

Measurement resolution

M :

Blade tip Mach number

Q :

Q method

r c :

Vortex core radius

R :

Blade radius

Re c :

Blade tip Reynolds number based on chord

T :

Rotor thrust

u = u, v, w :

Velocity vector with components in y, r, ϕ

u l :

Turbulent velocity scale

V tip :

Blade tip velocity

V θ :

Swirl velocity

V θ,m :

Peak swirl velocity

y, r, ϕ:

Fixed frame coordinates

\(y^{\prime},r^{\prime}\) :

Vortex frame coordinates

\(y^\star,r^\star\) :

Coordinates with respect to mean vortex center

α:

Wander orientation with respect to slipstream slope

β:

Helix pitch angle

\(\varGamma_1\) :

\(\varGamma_1\) method

\(\varepsilon\) :

Rate of energy dissipation

θ1deg :

Blade twist

\(\Uplambda\) :

Symmetric part of velocity gradient tensor

λ2 :

λ2 method

∇:

Gradient operator

ν:

Kinematic viscosity

\(\Uppi\) :

Skew-symmetric part of velocity gradient tensor

ρ f :

Fluid density

ρ p :

Particle density

σ:

Rotor solidity

σi :

Standard of deviation of wander in i direction

σy :

Standard of deviation of axial wander

σr :

Standard of deviation of radial wander

τ:

Kolmogorov time scale

ψ:

Wake age in angular coordinates

ψv :

Vortex age in angular coordinates

\(\Upomega\) :

Rotor speed

ω ψ :

ψ component of vorticity vector

References

  • Bailey SCC, Tavoularis JG (2008) Measurements of the velocity field of a wing-tip vortex, wandering in grid turbulence. J Fluid Mech 601:281–315

    Article  MATH  Google Scholar 

  • Baker GR, Barker SJ, Bofah KK, Saffman PG (1974) Laser anemometer measurements of trailing vortices in water. J Fluid Mech 62:325–336

    Article  Google Scholar 

  • Beresh SJ, Henfling JF, Spillers RW (2010) Meander of a fin trailing vortex and the origin of its turbulence. Exp Fluids 49:599–611

    Article  Google Scholar 

  • Bhagwat MJ, Leishman JG (2000) Correlation of helicopter rotor tip vortex measurements. AIAA J 38(2):301–308

    Article  Google Scholar 

  • Bhagwat MJ, Leishman JG (2000) Stability analysis of helicopter rotor wakes in axial flight. J Am Helic Soc 45(3):165–178

    Article  Google Scholar 

  • Bhagwat MJ, Ramasamy M (2012) Effect of tip vortex aperiodicity on measurement uncertainty. Exp Fluids 53:1191–1202

    Article  Google Scholar 

  • Chakraborty P, Balachandar S, Adrian RJ (2005) On the relationships between local vortex identification schemes. J Fluid Mech 535:189–214

    Article  MathSciNet  MATH  Google Scholar 

  • Cucitore R, Quadrio M, Baron A (1999) On the effectiveness and limitations of local criteria for the identification of the vortex. Eur J Mech B/Fluids 18(2):261–282

    Article  MathSciNet  MATH  Google Scholar 

  • Devenport WJ, Rife MC, Liapis SI, Follin GJ (1996) The structure and development of a wing-tip vortex. J Fluid Mech 312:67–106

    Article  MathSciNet  Google Scholar 

  • Graftieaux L, Michard M, Grosjean N (2001) Combining PIV, POD and Vortex identification algorithms for the study of unsteady turbulent swirling flows. Meas Sci Technol 12(9):1422–1429

    Article  Google Scholar 

  • Grant I (1997) Particle image velocimetry: A review. Proceedings of the institution of mechanical engineers 211(C):55–76

    Google Scholar 

  • Green SI, Acosta AJ (1991) Unsteady flow in trailing vortices. J Fluid Mech 227:107–134

    Article  Google Scholar 

  • Gursul I, Xie A (1999) Origin of vortex wandering over delta wings. J Aircraft 37(2):348–350

    Article  Google Scholar 

  • Han YO, Leishman JG, Coyne AJ (1997) Measurements of the velocity and turbulence structure of a rotor tip vortex. AIAA J 35(3):477–485

    Article  Google Scholar 

  • Heyes AL, Jones RF, Smith DAR (2004) Wandering of wing-tip vortex. In: Proc 12th Int Symp Appl Laser Tech Fluid Mech, Lisbon, Portugal

  • Hunt JCR, Wray AA, Moin P (1988) Eddies, stream, and convergence zones in turbulent flows. Center for Turbulence Research Report: CTR-S88:193–208

  • Iversen JD, Corsiglia VR, Park S, Backhus DR, Brickman RA (1978) Hot-wire, laser-anemometer, and force measurements of interacting trailing vortices. J Aircraft 16(7):448–454

    Article  Google Scholar 

  • Jacquin L, Fabre D, Geffroy P, Coustols E (2001) The properties of a transport aircraft wake in the extended near field: an experimental study. AIAA Paper 2001–1038

  • Jeong J, Hussain F (1995) On the identification of a vortex. J Fluid Mech 285:69–94

    Article  MathSciNet  MATH  Google Scholar 

  • Karpatne A, Sirohi J, Mula SM, Tinney CE (2012) Investigation of tip vortex aperiodicity in hover. In: American helicopter society 68th annual forum, Fort Worth, USA, May 1–3

  • Kindler K, Mulleners K, Richard H, van der Wall BG, Raffel M (2010) Aperiodicity in the near field of full-scale rotor blade tip vortices. Exp Fluids 50(6):1601–1610

    Article  Google Scholar 

  • Landgrebe AJ (1972) The wake geometry of a hovering helicopter rotor and its influence on rotor performance. J Am Helic Soc 17(4):3–15

    Article  Google Scholar 

  • Leishman JG (1998) Measurements of the aperiodic wake of a hovering rotor. Exp Fluids 25:352–361

    Article  Google Scholar 

  • Leishman JG, Baker A, Coyne A (1996) Measurements of rotor tip vortices using three-component laser doppler velocimetry. J Am Helic Soc 41(4):342–353

    Article  Google Scholar 

  • Levy Y, Degani D, Seginer A (1990) Graphical visualization of vortical flows by means of helicity. AIAA J 28(8):1347–1352

    Article  Google Scholar 

  • Martin PB, Leishman JG, Pugliese GJ, Anderson SL (2000) Stereoscopic PIV measurements in the wake of a hovering rotor. In: American helicopter society 56th annual forum, Virginia, USA, May 2–4

  • McAlister KW (2003) Rotor wake development during the first revolution. In: American helicopter society 59th annual forum, Phoenix, USA, May 6–8

  • Melling A (1997) Tracer particles and seeding for particle image velocimetry. Meas Sci Technol 8(12):1406–1416

    Article  Google Scholar 

  • Mula SM, Stephenson J, Tinney CE, Sirohi J (2011) Vortex jitter in hover. In: American helicopter society southwest region technical specialists meeting, Fort Worth, USA, February 23–25

  • Mula SM, Stephenson J, Tinney CE, Sirohi J (2012) Dynamical and evolutionary characteristics of the tip vortex from a four-bladed rotor in hover. In: American helicopter society 68th annual forum, Fort Worth, USA, May 1–3

  • Ohanian CV, McCauley GJ, Savaş O (2012) A visual study of vortex instabilities in the wake of a rotor in hover. J Amer Heli Soc 57(042005):1–8

    Article  Google Scholar 

  • Raffel M, Willert C, Kompenhans J (1998) Particle image velocimetry - A practical guide. Springer, Berlin

  • Ramasamy M, Johnson B, Huismann T, Leishman JG (2009) Digital particle image velocimetry measurements of tip vortex characteristics using an improved aperiodicity correction. J Am Helic Soc 54(012004):1–13

    Google Scholar 

  • Ramasamy M, Paetzel R, Bhagwat WJ (2011) Aperiodicity correction for rotor tip vortex measurements. In: American helicopter society 67th annual forum, Virginia Beach, USA, May 3–5

  • Richard H, Bosbach J, Henning A, Raffel M, Willert C, van der Wall G (2006) 2C and 3C PIV measurements on a rotor in hover condition. In: Proc 13th Int Symp Appl Laser Tech Fluid Mech, Lisbon, Portugal

  • Sirohi J, Lawson MS (2012) Measurement of helicopter rotor blade deformation using digital image correlation. J Optical Eng 51(4):43603

    Article  Google Scholar 

  • Stephenson JH, Mula SM, Tinney CE, Sirohi J (2012) Far wake rotorcraft vortex tumbling. In: 50th AIAA Aerospace sciences meeting and exposition, Nashville, USA, 2012–0425.

  • Thompson TL, Komerath NM, Gray RB (1988) Visualization and measurement of the tip vortex of a rotor blade in hover. J Aircraft 25(12):1113–1121

    Article  Google Scholar 

  • Tinney CE, Glauser MN, Ukeiley LS (2008) Low-dimensional characteristics of a transonic jet. Part 1: Proper orthogonal decomposition. J Fluid Mech 612:107–141

    Article  MATH  Google Scholar 

  • Tung C, Pucci SL, Caradonna FX, Morse HA (1981) The structure of trailing vortices generated by model rotor blades. NASA Technical Memorandum 81316

  • van der Wall BG, Richard R (2006) Analysis methodology for 3C-PIV data of rotary wing vortices. Exp Fluids 40:798–812

    Article  Google Scholar 

  • Westerweel J (1998) Effect of sensor geometry on the performance of PIV interrogation. In: Proc 9th Int Symp Appl Laser Tech Fluid Mech, Lisbon, Portugal

  • Widnall SE (1972) The stability of a helical vortex filament. J Fluid Mech 54(4):641–663

    Article  MATH  Google Scholar 

Download references

Acknowledgments

The work was supported by the Army/Navy/NASA Vertical Lift Research Center of Excellence (VLRCOE) led by the University of Maryland with Dr. Mike Rutkowski as Technical Monitor; Grant No. W911W6-11-2-0012. The authors also wish to acknowledge constructive discussions with Dr. Sheila Widnall.

Author information

Authors and Affiliations

  1. Aerospace Engineering and Engineering Mechanics Department, The University of Texas at Austin, 1 University Station, C0600, Austin, Texas, 78712, USA

    Swathi M. Mula, James H. Stephenson, Charles E. Tinney & Jayant Sirohi

Authors
  1. Swathi M. Mula
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. James H. Stephenson
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Charles E. Tinney
    View author publications

    You can also search for this author inPubMed Google Scholar

  4. Jayant Sirohi
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Swathi M. Mula.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Mula, S.M., Stephenson, J.H., Tinney, C.E. et al. Dynamical characteristics of the tip vortex from a four-bladed rotor in hover. Exp Fluids 54, 1600 (2013). https://doi.org/10.1007/s00348-013-1600-9

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s00348-013-1600-9

Keywords