Abstract
The continuous Hull of a repetitive tiling T in ℝd with the Finite Pattern Condition (FPC) inherits a minimal ℝd-lamination structure with flat leaves and a transversal  which is a Cantor set. This class of tiling includes the Penrose & the Amman Benkker ones in 2D, as well as the icosahedral tilings in 3D. We show that the continuous Hull, with its canonical ℝd-action, can be seen as the projective limit of a suitable sequence of branched, oriented and flat compact d-manifolds. As a consequence, the longitudinal cohomology and the K-theory of the corresponding C*-algebra
which is a Cantor set. This class of tiling includes the Penrose & the Amman Benkker ones in 2D, as well as the icosahedral tilings in 3D. We show that the continuous Hull, with its canonical ℝd-action, can be seen as the projective limit of a suitable sequence of branched, oriented and flat compact d-manifolds. As a consequence, the longitudinal cohomology and the K-theory of the corresponding C*-algebra  are obtained as direct limits of cohomology and K-theory of ordinary manifolds. Moreover, the space of invariant finite positive measures can be identified with a cone in the d
th homology group canonically associated with the orientation of ℝd. At last, the gap labeling theorem holds: given an invariant ergodic probability measure μ on the Hull the corresponding Integrated Density of States (IDS) of any selfadjoint operators affiliated to
are obtained as direct limits of cohomology and K-theory of ordinary manifolds. Moreover, the space of invariant finite positive measures can be identified with a cone in the d
th homology group canonically associated with the orientation of ℝd. At last, the gap labeling theorem holds: given an invariant ergodic probability measure μ on the Hull the corresponding Integrated Density of States (IDS) of any selfadjoint operators affiliated to  takes on values on spectral gaps in the ℤ-module generated by the occurrence probabilities of finite patches in the tiling.
takes on values on spectral gaps in the ℤ-module generated by the occurrence probabilities of finite patches in the tiling.
Similar content being viewed by others
References
Anderson, J.E., Putnam, I.: Topological invariants for substitution tilings and their associated C*-algebra. Erg. Th. Dynam. Syst. 18, 509–537 (1998)
Atiyah, M. F.: K-Theory, Harvard University Lecture Notes, 1964
Bellissard, J.: Schrödinger's operators with an almost periodic potential: an overview, Lecture Notes in Phys., n°153, Berlin-Heidelberg-NewYork, Springer Verlag, 1982, pp. 356–363
Bellissard, J.: K-Theory of C*-algebras in Solid State Physics. In: Statistical Mechanics and Field Theory, Mathematical Aspects, T.C. Dorlas, M.N. Hugenholtz, M. Winnink, eds., Lecture Notes in Physics, n°257, Berlin-Heidelberg-NewYork, Springer, 1986, pp. 99-156
Bellissard, J.: Gap labeling Theorems for Schrödinger's Operators. In From Number Theory to Physics, Les Houches March 89, J.M. Luck, P. Moussa, M. Waldschmidt eds., Berlin-Heidelberg-NewYork, Springer, (1993), pp.538-630
Bellissard, J., Bovier, A. Ghez, J.-M.: Gap labeling Theorems for One Dimensional Discrete, Schrödinger Operators. Rev. Math. Phys. 4, 1–37 (1992)
Bellissard, J., Contensou, E., Legrand, A.: K-théorie des quasi-cristaux, image par la trace: le cas du réseau octogonal. C. R. Acad. Sci. (Paris), t.327, Série I, 197-200 (1998)
Bellissard, J., Hermmann, D., Zarrouati, M.: Hull of Aperiodic Solids and Gap labeling Theorems. In: Directions in Mathematical Quasicrystals, CRM Monograph Series, Volume 13, M.B. Baake, R.V. Moody, eds., Providence, RI: AMS, 2000, pp. 207–259
Bellissard J., Kellendonk, J., Legrand, A.: Gap-labeling for three dimensional aperiodic solids. C. R. Acad. Sci. (Paris), t.332, Série I, 521–525 (2001)
Bellissard, J.: Noncommutative Geometry of Aperiodic Solids. In: Proceedings of the 2001 Summer School of Theoretical Physics, Geometry, Topology and Quantum Field Theory, Villa de Leyva, Colombia, 7–30 July 2001, River Edge NJ: World Scientific, 2003
Benameur, M., Oyono, H.: Private communication.
Benedetti, R., Gambaudo, J.-M.: On the dynamics of
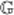 -solenoids. Applications to Delone sets. Ergod. Th. & Dynam. Sys. 23, 673–691 (2003)
-solenoids. Applications to Delone sets. Ergod. Th. & Dynam. Sys. 23, 673–691 (2003)Benedetti, R., Petronio, C.: Lectures on Hyperbolic Geometry, Berlin-Heidelberg-New York: Springer,1992
Benedetti, R., Petronio, C.: Branched Standard Spines of 3-Manifolds. LNM 1653, Berlin-Heidelberg-New York: Springer, 1997
Blackadar, B.: K-Theory for Operator Algebras. MSRI Publications, Vol.5, Berlin-Heidelberg-New York: Springer-Verlag, 1986
Connes, A.: Sur la théorie non commutative de l'intégration. In: Algèbres d'Opérateurs, Lecture Notes in Mathematics, 725, Berlin: Springer, Berlin 1979 pp. 19–143
Connes, A.: ``An analogue of the Thom isomorphism for crossed products of a C*-algebra by an action of R''. Adv. in Math. 39, 31–55 (1981)
Connes, A.: Cyclic cohomology and the transverse fundamental class of a foliation. Pitman Res. Notes in Math. 123, London: Longman Harlow, 1986, pp. 52–144
Connes, A.: Noncommutative Geometry. San Diego: Academic Press, 1994
Ghys, E.: Laminations par surfaces de Riemann. In: Dynamique et géométrie complexes, Panoramas & Synthèses 8, 49–95 (1999)
Forrest, A., Hunton, J.: The cohomology and K-theory of commuting homeomorphisms of the Cantor set. Erg. Th. Dynam. Syst. 19, 611–625 (1999)
Forrest, A., Hunton, J., Kellendonk, J.: Cohomology of canonical projection tilings. Commun. Math. Phys. 226, 289–322 (2002); Topological invariants for projection point patterns. Memoirs of the Amer. Math. Soc. Vol I, 159/758 providence RI Amer. Math. Soc., 2002; Cohomology groups for projection point patterns. In: Proceedings of the International Congress of Mathematical Physics 2000, Boston MA: Int press 2001, pp. 333–339
van Elst, A.: Gap labeling Theorems for Schrödinger's Operators on the Square and Cubic Lattice. Rev. Math. Phys. 6, 319–342 (1994)
Gähler F., Kellendonk, J.: Cohomology groups for projection tilings of codimension 2. Mat. Sci. Eng. A 294–296, 438–440 (2000)
Gambaudo, J.-M., Martens, M.: Algebraic topology for minimal Cantor sets. Preprint, Dijon (2000), at http://www.dim.uchile. cl/gambaudo/GM.pdf
Gottschalk, W., Hedlund, G.A.: Topological Dynamics. providence RI: Amer. Math. Soc., 1955
Griffiths, P, Harris, J.: Principles of Algebraic Geometry. New York: Wiley 1978
Hippert, F., Gratias, D. eds.: Lectures on Quasicrystals. Les Ulis: Editions de Physique, 1994
Johnson, R., Moser, J.: The rotation number for almost periodic potentials. Commun. Math. Phys. 84 403–438 (1982)
Kaminker, J., Putnam, I.F.: Private communication.
Kellendonk, J., Putnam, I.F.: Tilings, C*-algebras and K-theory. In: Directions in Mathematical Quasicrystals, CRM Monograph Series, Volume 13, M.P. Baake, R.V. Moody, eds., Providence, RI: AMS, 2000, pp. 177–206
Lagarias, J.C., Pleasants, P.A.B.: Repetitive Delone sets and quasicrystals. Ergod. Th. & Dynam. Sys. 23, 831–867 (2003)
Milnor, J.W., Stasheff, J.D.: Characteristic Classes. Princeton, NJ: Princeton University Press, 1974
Moore, C.C., Schochet, C.: Global Analysis on Foliated Spaces. Math. Sci. Res. Inst. Publ. No. 9, New York: Springer-Verlag, 1988
Pedersen, G.: C* -algebras. and their Automorphism Group. London: Academic Press, 1979
Pimsner, M.V., Voiculescu, D.: Exact sequences for K-groups and ext-groups of certain cross-product of C*-algebra. J. Operator Theory 4, 93–118 (1980)
Pimsner, M.V.: Range of traces on K 0 of reduced crossed products by free groups. In: Operator Algebras and their Connections with Topology and Ergodic Theory, Lecture Notes in Math., n°1132, Berlin-Heidelberg-New York: Springer Verlag, 1983, pp. 374–408
Putnam, I.F.: The C ★ algebras associated with minimal homeomorphisms of the Cantor set. Pac. J. Math. 136, 329–352 (1989)
Radin, C.: Miles of Tiles. Student Mathematical Library, Vol 1 providence RI: Amer. Math. Soc., 1999; see also Radin, C.: The pinwheel tilings of the plane. Ann. Math. 139, 661–702 (1994)
Sadun, L., Williams, R.F.: Tiling spaces are Cantor fiber bundles Ergith and Dya. Systs. 23, 307–316(2003)
Senechal, M.: Crystalline Symmetries : An informal mathematical introduction, Institute of Physics, London: Alan Hilger, Ltd., 1990
Serre, J.-P.: Modules projectifs et espaces fibrés à fibre vectorielle, (1958) Séminaire P. Dubreil, M.-L. Dubreil-Jacotin et C. Pisot, 1957/58, Fasc. 2, Exposé 23, Paris: Secrétariat mathématique, 18 pp.
Spanier, E.H.: Algebraic Topology, New York: McGraw-Hill, 1966
Sullivan, D.: Cycles for the dynamical study of foliated manifolds and complex manifolds. Invent. Math. 89, 225–255 (1976)
Swan, R.G.: Vector bundles and projective modules. Trans. Amer. Math. Soc. 105, 264–277 (1962)
Thurston, W.: Geometry and Topology of 3-manifolds. princeton, NJ: Princeton University Press 1979
Versik, A.M.: A Theorem on Periodical Markov Approximation in Ergodic Theory. In: Ergodic Theory and Related Topics (Vitte, 1981), Math. Res., 12, Berlin: Akademie-Verlag, 1981, pp. 195–206
Williams, R.F.: Expanding attractors. Publ. IHES 43 169–203 (1974)
Author information
Authors and Affiliations
Additional information
Communicated by A. Connes
Accepted in Revised Form: 7 May 2005
Rights and permissions
About this article
Cite this article
Bellissard, J., Benedetti, R. & Gambaudo, JM. Spaces of Tilings, Finite Telescopic Approximations and Gap-Labeling. Commun. Math. Phys. 261, 1–41 (2006). https://doi.org/10.1007/s00220-005-1445-z
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00220-005-1445-z




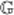 -solenoids. Applications to Delone sets. Ergod. Th. & Dynam. Sys. 23, 673–691 (2003)
-solenoids. Applications to Delone sets. Ergod. Th. & Dynam. Sys. 23, 673–691 (2003)