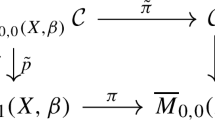Skip to main content
SpringerLink
Log in

Counting curves on surfaces in Calabi–Yau 3-folds

  • Published:
Mathematische Annalen Aims and scope Submit manuscript

Abstract

Motivated by S-duality modularity conjectures in string theory, we define new invariants counting a restricted class of two-dimensional torsion sheaves, enumerating pairs \(Z\subset H\) in a Calabi–Yau threefold \(X\). Here \(H\) is a member of a sufficiently positive linear system and \(Z\) is a one-dimensional subscheme of it. The associated sheaf is the ideal sheaf of \(Z\subset H\), pushed forward to \(X\) and considered as a certain Joyce–Song pair in the derived category of \(X\). We express these invariants in terms of the MNOP invariants of \(X\).

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

Notes

  1. We use straight \(I\)s to denote ideal sheaves on \(H\) and curly \(\mathcal {I}\)s for ideal sheaves on \(X\).

  2. Isomorphism is meant in the strict sense: two families of stable pairs \((\mathcal {E}_i,s_i)\) are isomorphic if there is an isomorphism \(\mathcal {E}_1\rightarrow \mathcal {E}_2\) taking \(s_1\) to \(s_2\). Stable pairs have no automorphisms so there is no need to tensor by line bundles pulled back from the base (as one does to define isomorphism for families of stable sheaves, for example: stable sheaves have Aut \(=\mathbb {C}^*\)).

  3. In fact it is all of \(M_{c}(X)\) when \(H^2(X,\mathbb {Z})\) is torsion-free.

  4. For instance this is the case when all members of the linear system \(|H|\) are reduced and irreducible. The hyperplane sections of the quintic threefold have this property by the Lefschetz hyperplane theorem.

  5. In [15] Toda studies some different but remarkable wall crossings. These relate the counting of torsion sheaves to the counting of both ideal sheaves [11] and stable pairs [13]. There may be some connection: a hint is provided by the fact that Fujita’s conjecture (which would determine which \(H\) are sufficiently positive in the sense of Definition 2.2) enters into his analysis. Manschot [10] also studies another wall crossing involving these invariants.

References

  1. Behrend, K.: Donaldson–Thomas invariants via microlocal geometry. Ann. Math. 170, 1307–1338 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  2. Cheng, M., DeBoer, J., Dijkgraaf, R., Manschot, J., Verlinde, E.: A farey tail for attractor black holes. J. High Energy Phys. 2006(11), 1–28 (2006)

  3. Denef, F., Moore, G.: Split states, entropy enigmas, holes and halos. J. High Energy Phys. 2011(11), 1–152 (2011)

  4. Gaiotto, D., Strominger, A., Yin, X.: The M5-Brane elliptic genus: modularity and BPS states. J. High Energy Phys. 2007(8), 1–18 (2007)

  5. Gaiotto, D., Yin, X.: Examples of M5-Brane elliptic genera. J. High Energy Phys. 2007(11), 1–12 (2007)

    Google Scholar 

  6. Huybrechts, D., Thomas, R.P.: Deformation-obstruction theory for complexes via Atiyah and Kodaira–Spencer classes. Math. Ann. 346(3), 545–569 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  7. Joyce, D., Song, Y.: A theory of generalized Donaldson–Thomas invariants. Memoirs AMS 217(1020), iv+199 (2011). ISBN 978-0-8218-5279-8

  8. Kollár, J.: Projectivity of complete moduli. J. Differ. Geom. 32, 235–268 (1990)

    MATH  Google Scholar 

  9. Maldacena, J., Strominger, A., Witten, E.: Black hole entropy in M-theory. J. High Energy Phys. 1997(12), 1–16 (1997)

  10. Manschot, J.: Wall-crossing of D4-branes using flow trees. Adv. Theor. Math. Phys. 15(1), 1–42 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  11. Maulik, D., Nekrasov, N., Okounkov, A., Pandharipande, R.: Gromov–Witten theory and Donaldson–Thomas theory. I. Compos. Math. 11, 1263–1285 (2006)

    Article  MathSciNet  Google Scholar 

  12. Ooguri, H., Strominger, A., Vafa, C.: Black hole attractors and the topological string. Phys. Rev. D 70(10), 106–119 (2001)

    MathSciNet  Google Scholar 

  13. Pandharipande, R., Thomas, R.P.: Curve counting via stable pairs in the derived category. Invent. Math. 178, 407–447 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  14. Thomas, R.P.: A holomorphic Casson invariant for Calabi–Yau 3-folds, and bundles on K3 fibrations. J. Differ. Geom. 54, 367–438 (2000)

    MATH  Google Scholar 

  15. Toda, Y.: Bogomolov–Gieseker type inequality and counting invariants. J. Topol. 6(1), 217–250 (2013)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgments

We thank Kai Behrend, Vincent Bouchard, Jim Bryan, Tudor Dimofte, Dagan Karp, Jan Manschot, Davesh Maulik and Yukinobu Toda for useful discussions. The second author would like to thank the University of British Columbia, the Max Planck Institute, Bonn and the Isaac Newton Institute for Mathematical Sciences, Cambridge for their hospitality. The third author is supported by the EPSRC programme Grant EP/G06170X/1.

Author information

Authors and Affiliations

  1. University of Maryland, College Park, MD , 20742-4015, USA

    Amin Gholampour

  2. Department of mathematics, Ohio State University, 600 Math Tower, 231 West 18th Avenue, Columbus, OH , 43210-1174, USA

    Artan Sheshmani

  3. Imperial College London, 180 Queen’s Gate, London , SW7 2AZ, UK

    Richard Thomas

Authors
  1. Amin Gholampour
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Artan Sheshmani
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Richard Thomas
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Amin Gholampour.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Gholampour, A., Sheshmani, A. & Thomas, R. Counting curves on surfaces in Calabi–Yau 3-folds. Math. Ann. 360, 67–78 (2014). https://doi.org/10.1007/s00208-014-1035-5

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00208-014-1035-5

Keywords

Search

Navigation