Skip to main content
Springer Nature Link
Log in

Sensitivity analysis of structural response uncertainty propagation using evidence theory

  • Research Paper
  • Published:
Structural and Multidisciplinary Optimization Aims and scope Submit manuscript

Abstract

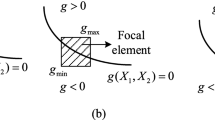
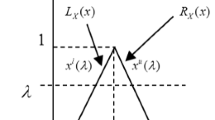
Sensitivity analysis for the quantified uncertainty in evidence theory is developed. In reliability quantification, classical probabilistic analysis has been a popular approach in many engineering disciplines. However, when we cannot obtain sufficient data to construct probability distributions in a large-complex system, the classical probability methodology may not be appropriate to quantify the uncertainty. Evidence theory, also called Dempster–Shafer Theory, has the potential to quantify aleatory (random) and epistemic (subjective) uncertainties because it can directly handle insufficient data and incomplete knowledge situations. In this paper, interval information is assumed for the best representation of imprecise information, and the sensitivity analysis of plausibility in evidence theory is analytically derived with respect to expert opinions and structural parameters. The results from the sensitivity analysis are expected to be very useful in finding the major contributors for quantified uncertainty and also in redesigning the structural system for risk minimization.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  • Arora JS (1989) Introduction to optimum design. McGraw-Hill, New York

    Google Scholar 

  • Bae H-R, Grandhi RV, Canfield RA (2003) Uncertainty quantification of structural response using evidence theory. AIAA J 41(10):2062–2068

    Google Scholar 

  • Dempster AP, Laird NM, Rubin DB (1977) Maximum likelihood from incomplete data via the EM algorithm. J Stat Soc 39:1–38

    MathSciNet  Google Scholar 

  • Dong W, Shah H (1987) Vertex method for computing functions on fuzzy variables. Fuzzy Sets Syst 24:65–78

    Article  MathSciNet  Google Scholar 

  • Elishakoff I (1999) Whys and hows of uncertainty modelling: probability, fuzziness and anti-optimization. Springer, Berlin Heidelberg New York

    Google Scholar 

  • Li C, Rao SS (1998) A modified Dempster–Shafer theory for multicriteria optimization. Eng Optim 30:177–201

    Google Scholar 

  • Oberkampf WL, Helton JC, Sentz K (2001) Mathematical representation of uncertainty. Proceedings of the 41st AIAA/ASME/ASCE/AHS/ASC structures, structural dynamics and materials conference, AIAA-2001-1645, Seattle, 16–19 April 2001

  • Savage LJ (1972) The foundations of statistics. Dover, New York

    Google Scholar 

  • Sentz K, Ferson S (2002) Combination of evidence in Dempster–Shafer theory. Report SAND2002-0835, Sandia National Laboratories, Albuquerque

  • Shafer G (1976) A mathematical theory of evidence. Princeton University Press, Princeton

    Google Scholar 

  • Walpole RE (1998) Probability and statistics for engineers and scientists. Prentice Hall, Upper Saddle River

    Google Scholar 

  • Yager RR (1987) On the Dempster–Shafer framework and new combination rules. Info Sci 41:93–137

    MATH  MathSciNet  Google Scholar 

  • Yager RR, Kacprzyk J, Fedrizzi M (1994) Advances in the Dempster–Shafer theory of evidence. Wiley, New York

    Google Scholar 

  • Zadeh L (1965) Fuzzy sets. Inf Control 8:338–353

    Article  MATH  MathSciNet  Google Scholar 

  • Zadeh L (1984) Review of Shafer's a mathematical theory of evidence. Artif Intell Mag 5:81–83

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mechanical and Material Engineering, Wright State University, Dayton, OH, 45435, USA

    H.-R. Bae & R. V. Grandhi

  2. Department of Aeronautics and Astronautics, Air Force Institute of Technology, WPAFB, OH, 45433, USA

    R. A. Canfield

Authors
  1. H.-R. Bae
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. R. V. Grandhi
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. R. A. Canfield
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to R. V. Grandhi.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Bae, HR., Grandhi, R.V. & Canfield, R.A. Sensitivity analysis of structural response uncertainty propagation using evidence theory. Struct Multidisc Optim 31, 270–279 (2006). https://doi.org/10.1007/s00158-006-0606-9

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00158-006-0606-9

Keywords