Abstract
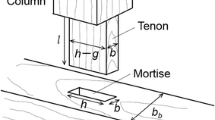
The existence of gaps between tenon and mortise in traditional timber structures is very common. Accurate estimation of its probability distribution is essential for the stress and reliability analysis. This paper presents the probability distributions for the horizontal and vertical gaps based on measurement. The gap data were collected from four traditional timber buildings in a heritage building complex by manual on-site measurement. Statistical analysis of data for each building was performed. Results show that the statistical features of all four buildings are similar. All the measured gap data were then categorized into two groups in horizontal and vertical directions. The optimal distribution to represent these gap data was obtained using the probability plot correlation coefficient method and Anderson–Darling test. Results demonstrate that the Weibull distribution is the most appropriate model for fitting both the horizontal and vertical gaps. Parameters of the distributions were obtained from the maximum likelihood estimate. A finite-element (FE) numerical investigation for a joint was conducted. Gaps were randomly created using the obtained probability model in the joints. Static analysis results show that the gaps will weaken the mechanical behavior of the tenon–mortise joint, especially the initial stiffness. Compared with the horizontal gaps, the vertical gaps affect the mechanical properties of the joint more directly.








Similar content being viewed by others
Explore related subjects
Discover the latest articles and news from researchers in related subjects, suggested using machine learning.References
Anderson T, Darling D (1952) Asymptotic theory of certain “goodness of fit” criteria based on stochastic processes. Ann Math Stat 23:193–212. https://doi.org/10.1214/aoms/1177729437
Blom G (1958) Statistical estimates and transformed beta variables. Wiley, New York, pp 53–55
Calderoni C, Matteis GD, Giubileo C, Mazzolani FM (2010) Experimental correlations between destructive and non-destructive tests on ancient timber elements. Eng Struct 32:442–448. https://doi.org/10.1016/j.engstruct.2009.10.006
Chang WS, Hsu MF (2007) Rotational performance of traditional Nuki joints with gap II: the behavior of butted Nuki joint and its comparison with continuous Nuki joint. J Wood Sci 53:401–407. https://doi.org/10.1007/s10086-007-0880-1
Chang WS, Hsu MF, Komatsu K (2006) Rotational performance of traditional Nuki joints with gap I: theory and verification. J Wood Sci 52:58–62. https://doi.org/10.1007/s10086-005-0734-7
Chen CC (2016) Integral mechanics property analysis and safety evaluation of ancient timber structures. Dissertation, Southeast University
Cunnane C (1978) Unbiased plotting position—a review. J Hydrol 37:205–222. https://doi.org/10.1016/0022-1694(78)90017-3
Dias AMPG, Kuilen JWVD, Lopes S, Cruz H (2007) A non-linear 3D FEM model to simulate timber–concrete joints. Adv Eng Softw 38:522–530. https://doi.org/10.1016/j.advengsoft.2006.08.024
Feng K (2016) Study on The seismic performance of the damaged ancient buildings. Dissertation, Taiyuan University of Technology
Filliben JJ (1975) The probability plot correlation coefficient test for normality. Technometrics 17:111–117. https://doi.org/10.2307/1268008
Freedman D, Diaconis P (1981) On the histogram as a density estimator: L2 theory. Probab Theory Rel 57:453–476. https://doi.org/10.1007/BF01025868
Gao DF (2007) A research on the structure and their aseismatic characteristics of ancient Chinese timber buildings. Dissertation, Xi’an University of Architecture and Technology
Gringorten II (1963) A plotting rule for extreme probability paper. J Geophys Res 68:813–814. https://doi.org/10.1029/JZ068i003p00813
Hill R (1948) A theory of the yielding and plastic flow of anisotropic metals. Proc R Soc Lond 193:281–297. https://doi.org/10.1098/rspa.1948.0045
Jiang SF, Wu MH, Tang WJ, Liu XM (2016) Multi-scale modeling method and seismic behavior analysis for ancient timber structures. J Build Struct 37:44–53. https://doi.org/10.14006/j.jzjgxb.2016.10.006
Kang K, Qiao GF, Chen JY, Niu QF, Li TY, Wei JW (2016) The influence of gap between tenon and mortise on the bearing behavior of the dovetail joints in ancient timber structure by finite element analysis. China Sciencepap 11:38–42. https://doi.org/10.3969/j.issn.2095-2783.2016.01.009
Li TY (2004) The main structural damages and damage mechanism analysis on Yingxian Wooden Tower. Dissertation, Taiyuan University of Technology
Ma BJ (2003) Techniques on Chinese ancient wood structure building, 2nd edn. Science Press, Beijing, pp 120–139 (167, 176–181)
Oudjene M, Khelifa M (2009) Finite element modelling of wooden structures at large deformations and brittle failure prediction. Mater Des 30:4081–4087. https://doi.org/10.1016/j.matdes.2009.05.024
Pan GX, He JZ (2005) Interpretation of Ying-tsao-fa-shih. Southeast University Press, Nanjing, pp 65–75
Pan Y, Wang C, Tang L, Li LG (2015) Study on mechanical model of straight-tenon joints in ancient timber structures. Eng Mech 32:82–89. https://doi.org/10.6052/j.issn1000-4750,2013.08.0728
Qiang XH, Bijlaard FSK, Kolstein H, Jiang X (2014) Behaviour of beam-to-column high strength steel endplate connections under fire conditions-part 1: experimental study. Eng Struct 64:23–38. https://doi.org/10.1016/j.engstruct.2014.01.028
Reddy JN (2004) An introduction to non-linear finite element analysis. Oxford University Press, New York, pp 91–102
Xia HL (2015) Experimental study on seismic behavior of through-tenon joints under different degree of looseness in ancient wooden buildings. Dissertation, Xi’an University of Architecture and Technology
Xu BH, Bouchaïr A, Racher P (2015) Mechanical behavior and modeling of dowelled steel-to-timber moment-resisting connections. J Struct Eng 141:04014165. https://doi.org/10.1061/(asce)st.1943-541x.0001119
Xue JY, Li YZ, Xia HL, Sui Y (2016) Experimental study on seismic performance of dovetail joints with different loose degrees in ancient building. J Build Struct 37:73–79. https://doi.org/10.14006/j.jzjgxb.2016.04.010
Acknowledgements
The National Key R&D Program of China (2017YFC0703505), The Overseas Expertise Introduction Project for Discipline Innovation (B13002), National Natural Science Foundation of China (51338001, 51408038) and Beijing Natural Science Foundation of China (8151003).
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Yang, Q., Gao, C., Wang, J. et al. Probability distribution of gaps between tenon and mortise of traditional timber structures. Eur. J. Wood Prod. 78, 27–39 (2020). https://doi.org/10.1007/s00107-019-01472-1
Received:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00107-019-01472-1