Abstract
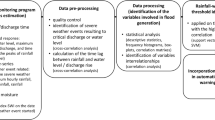
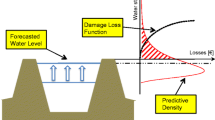
Operational real time flood forecasting systems generally require a hydrological model to run in real time as well as a series of hydro-informatics tools to transform the flood forecast into relatively simple and clear messages to the decision makers involved in flood defense. The scope of this chapter is to set forth the possibility of providing flood warnings at given river sections based on the direct comparison of the quantitative precipitation forecast with critical rainfall thresholdvalues, without the need of an on-line real time forecasting system. This approach leads to an extremely simplified alert system to be used by non technical stakeholders and could also be used to supplement the traditional flood forecasting systems in case of system failures. The critical rainfall threshold values, incorporating the soil moisture initial conditions, result from statistical analyses using long hydrological time series combined with a Bayesian utility function minimization. In the chapter, results of an application of the proposed methodology to the Sieve river, a tributary of the Arno river in Italy, are given to exemplify its practical applicability.
Preview
Unable to display preview. Download preview PDF.
Similar content being viewed by others
References
Annunziati, A., A. Focardi, P. Focardi, S. Martello, and P. Vannocci, 1999: Analysis of the rainfall thresholds that induced debris flows in the area of Apuan Alps – Tuscany, Italy, Plinius Conference ’99: Mediterranean Storms. Ed. Bios. pp. 485–493.
Benjamin, J. R. and C. A. Cornell, 1970: Probability, Statistics, and Decision For Civil Engineers, McGraw-Hill, New York.
Berger, J. O., 1986: Statistical Decision Theory and Bayesian Analysis, Springer-Verlag, New York.
Beven, K., 1987: Towards the use of catchment geomorphology in flood frequency predictions. Earth Surf Processes Landforms, 12, 6–82.
Bras, R. L., D. R. Gaboury, D. S. Grossman, and G. J. Vicen, 1985: Spatially varying rainfall and flood risk analysis. J. Hydraul. Eng., 111, 754–773.
Cameron, D. S., K. J. Beven, J. Tawn, S. Blazkova, and P. Naden, 1999: Flood frequency estimation by continuous simulation for a gauged upland catchment (with uncertainty). J. Hydrol., 219, 169–187.
Carpenter, T. M., J. A. Sperfslage, K. P. Georgakakos, T. Sweeney, and D. L. Fread, 1999: National threshold runoff estimation utilizing GIS in support of operational flash flood warning systems. J. Hydrol., 224, 21–44.
Ciarapica, L. and Todini E., 2002: TOPKAPI: a model for the representation of the rainfall-runoff process at different scales. Hydrological Processes, 16(2), 207–229.
Cowpertwait, P. S. P., 1991: Further developments of the Neyman-Scott clustered point processes for modelling rainfall. Water Resour. Res., 27(7), 1431–1438.
Cowpertwait, P. S. P., P. E. O’Connell, A. V. Metcalfe, and J. A. Mawdsley, 1996: Stochastic point process modelling of rainfall. I. Single site fitting and validation. J. Hydrology, 175, 17–46.
Crosta, G. B. and P. Frattini, 2000: Rainfall thresholds for soil slip and debris flow triggering, Proceedings of the EGS 2nd Plinius Conference on Mediterranean Storms, Ed. Bios.
Crosta, G. B. and P. Frattini, 2001: Coupling empirical and physically based rainfall thresholds for shallow landslides forecasting, Proceedings of the EGS 3rd Plinius Conference on Mediterranean Storms, Ed. Bios.
Crosta, G. B. and P. Frattini, 2003: Distributed modelling of shallow landslides triggered by intense rainfall. Nat. Hazards Earth Sci., 3, 81–93.
EFFS: Gouweleeuw B., P. Reggiani, and A. de Roo (eds.), 2004: A European Flood Forecasting System. Full Report (Contract no. EVG1-CT-1999-00011, http://effs.wldelft.nl.
De Vita, P. and P. Reichenbach, 1998: Rainfall triggered landslides: a reference list. Environ. Geol., 35(2/3), 219–233.
Gandin, L. S. and A. H. Murphy, 1992: Equitable skill scores for categorical forecasts. Mon. Weather Rev., 120, 361–370.
Georgakakos, K. P., 2006: Analytical results for operational flash flood guidance. J. Hydrology, in press. 317, 81–103.
Gray, D. D., P. G. Katz, S. M. deMonsabert, and N. P. Cogo, 1982: Antecedent moisture condition probabilities, J. Irrig. Drain. Engrg. Div., ASCE, 108(2), 107–114.
Hawkins, R. H., A. T. Hjelmfelt, and A. W. Zevenberger, 1985: Runoff probability, storm depth, and curve numbers. J. Irrig. Drain. Engrg., ASCE, 111(4), 330–340.
Hennrich, K., 2000: Modelling CriticalWater Contents for Slope Stability and Associated Rainfall Thresholds using Computer Simulations. In: Bromhead, E., Dixon, N. and Ibsen, M.-L. (eds.) Landslides in Research, Theory and Practice – Proceedings of the 8th International Symposium on Landslides, Cardiff/UK, Thomas Telford Ltd.
Iverson, R. M., 2000: Landslide triggering by rain infiltration. Water Resour. Res., 36(7), 1897– 1910.
Kelly, K. S. and R. Krzysztofowicz, 1997: A bivariate meta-Gaussian density for use in Hydrology. Stoch. Hydrol. Hydraul., 11, 17–31.
Liu, Z. and E. Todini, 2002: Towards a comprehensive physically-based rainfall-runoff model. Hydrol. Earth Syst. Sci., 6, 859–881.
Mancini, M., P. Mazzetti, S. Nativi, D. Rabuffetti, G. Ravazzani, P. Amadio, and R. Rosso, 2002: Definizione di soglie pluviometriche di piena per la realizzazione di un sistema di allertamento in tempo reale per il bacino dell’Arno a monte di Firenze, Proc. XXVII Convegno di idraulica e Costruzione Idrauliche, Vol. II:497–505 (In Italian).
Mason, S. J., 1982: A model for assessment of weather forecasts. Aust. Meteor. Mag., 30, 291–303.
Mason, S. J. and N. E. Graham, 1999: Conditional probabilities, relative operating characteristics and relative operating levels. Weather Forecast., 14, 713–725.
Moore, R. J. and R. T. Clarke, 1981: A distribution function approach to rainfall runoff modelling. Water Resour. Res., 17(5), 1367–1382.
Neary, D .G. and L. W. Swift, 1987: Rainfall thresholds for triggering a debris flow avalanching event in the southern Appalachian Mountains. Rew. Eng. Geology, VII, 81–95.
Nelsen, R. B., 1999: An Introduction to Copulas, Springer-Verlag, New York.
Reed, S., D. Johnson, and T. Sweeney, 2002: Application and national geographic information system database to support two-year flood and threshold runoff estimates. J. Hydrol. Eng., 7(3), 209–219, May 1.
Rodriguez-Iturbe, I., D. R. Cox, and Isham V., 1987a : Some models for rainfall based on stochastic point processes. Proc. R. Soc. London, Ser. A, 410, 269–288.
Rodriguez-Iturbe, I., B. Febres De Power, and J. B. Valdes, 1987b: Rectangular Pulses point processes models for rainfall: analysis of empirical data. J. Geophys. Res., 92(D8), 9645–9656.
SCS – Soil Conservation Service, 1986: National Engineering Handbook, Section 4, Hydrology, Rev. Ed., USDA, Washington DC, USA.
Sweeney, T. L., 1992: Modernized areal flash flood guidance. NOAA Technical Report NWS HYDRO 44, Hydrologic Research Laboratory, National Weather Service, NOAA, Silver Spring, MD, October, 21pp. and an appendix.
Todini, E., 1996: The ARNO rainfall-runoff model. J. Hydro., 175, 339–382.
Todini, E. and L. Ciarapica, 2002: The TOPKAPI model. In: Singh, V. P., D. K. Frevert, and S. P. Meyer, (eds.) Mathematical Models of Large Watershed Hydrology. Water Resources Publications, Littleton, Colorado, Chapter 12, 471–506.
Van derWaerden, B. L., 1952: Order tests for two-sample problem and their power. Indagat.Math., 14, 253–458.
Wang, C. T., V. K. Gupta and E. Waymire, 1981: A geomorphologic synthesis of nonlinearity in surface runoff. Water Resour. Res. 17(3), 545–554.
Zhao, R. J., 1977: Flood Forecasting Method for Humid Regions of China, East China College of Hydraulic Engineering, Nanjing.
Author information
Authors and Affiliations
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2009 Springer Science+Business Media B.V.
About this chapter
Cite this chapter
Martina, M., Todini, E., Libralon, A. (2009). Rainfall Thresholds for Flood Warning Systems: A Bayesian Decision Approach. In: Sorooshian, S., Hsu, KL., Coppola, E., Tomassetti, B., Verdecchia, M., Visconti, G. (eds) Hydrological Modelling and the Water Cycle. Water Science and Technology Library, vol 63. Springer, Berlin, Heidelberg. https://doi.org/10.1007/978-3-540-77843-1_9
Download citation
DOI: https://doi.org/10.1007/978-3-540-77843-1_9
Publisher Name: Springer, Berlin, Heidelberg
Print ISBN: 978-3-540-77842-4
Online ISBN: 978-3-540-77843-1
eBook Packages: Earth and Environmental ScienceEarth and Environmental Science (R0)