Skip to main content
SpringerLink
Log in

A Morse-theoretical proof of the Hartogs extension theorem

  • Published:
The Journal of Geometric Analysis Aims and scope Submit manuscript

Abstract

One hundered years ago exactly, in 1906, Hartogs published a celebrated extension phenomenon (birth of Several Complex Variables), whose global counterpart was understood later: Holomorphic functions in a connected neighborhood V(∂Ω) of a connected boundary ∂Ω ⋐ℂn ≥ 2) do extend holomorphically and uniquely to the domain ό. Martinelli, in the early 1940’s, and Ehrenpreis in 1961 obtained a rigorous proof, using a new multidimensional integral kernel or a short\(\bar \partial \) argument, but it remained unclear how to derive a proof using only analytic discs, as did Hurwitz (1897), Hartogs (1906), and E. E. Levi (1911) in some special, model cases. In fact, known attempts (e.g., Osgood, 1929, Brown, 1936) struggled for monodromy against multivaluations, but failed to get the general global theorem.

Moreover, quite unexpectedly, in 1998, Fornœss exhibited a topologically strange (nonpseudoconvex) domain όF ⊂ ℂ2 that cannot befitted in by holomorphic discs, when one makes the additional requirement that discs must all lie entirely inside όF. However, one should point out that the standard, unrestricted disc method usually allows discs to go outside the domain (just think of Levi pseudoconcavity).

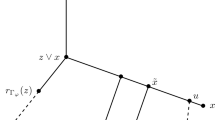
Using the method of analytic discs for local extensional steps and Morse-theoretical tools for the global topological control of monodromy, we show that the Hartogs extension theorem can be established in such a way.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Andreotti, A. and Grauert, H. Théorèmes de finitude pour la cohomologie des espaces complexes,Bull. Soc. Math. France 90, 193–259, (1962).

    MathSciNet  MATH  Google Scholar 

  2. Andreotti, A. and Hill, C. D. E. E. Levi convexity and the Hans Lewy problem, I and II,Ann. Scuola Norm. Sup. Pisa, Sci. Fis. Mat. 26, 325–363, 747–806, (1972).

    MathSciNet  MATH  Google Scholar 

  3. Bedford, E. Review of [10],Mathematical Reviews, available on MathScinet.

  4. Bochner, S. Analytic and meromorphic continuation by means of Green’s formula,Ann. of Math. (2) 44, 652–673, (1943).

    Article  MathSciNet  Google Scholar 

  5. Brown, A. B. On certain analytic continuations and analytic homeomorphisms,Duke Math. J. 2(1), 20–28, (1936).

    Article  MathSciNet  MATH  Google Scholar 

  6. Chirka, E. M. and Stout, E. L. Removable singularities in the boundary. Contributions to complex analysis and analytic geometry,Aspects Math, E26, Vieweg, Braunschweig, 43–104, (1994).

  7. Demailly, J.-P. Analytic and algebraic geometry, lecture notes available at: http://www- - fourier. ujf - - grenoble. fr/∼demailly.

  8. Ehrenpreis, L. A new proof and an extension of Hartogs’ theorem,Bull. Amer. Math. Soc. 67, 507–509, (1961).

    Article  MathSciNet  MATH  Google Scholar 

  9. Fichera, G. Caratterizzazione della traccia, sulla frontiera di un campo, di una funzione analitica di più variabili complesse,Atti Accad. Naz. Lincei Rend. Cl. Sci. Fis. Mat. Nat. (8) 22, 706–715, (1957).

    MathSciNet  MATH  Google Scholar 

  10. Fomæss, J. E. The disc method,Math. Z 227(4), 705–709, (1998).

    Article  MathSciNet  Google Scholar 

  11. Fueter, R. Über einen Hartogs’schen Satz,Comm. Math. Helv. 12, 75–80, (1939).

    Article  MathSciNet  MATH  Google Scholar 

  12. Gompf, R. E. and Stipsicz, A. E.4-Manifolds and Kirby Calculus, Graduate Studies in Mathematics, Vol. 20, AMS Providence, Rhode Island, (1999).

    Google Scholar 

  13. Grauert, H. Theory of q-convexity and q-concavity, inSeveral Complex Variables VII, Grauert, H., Peternell, Th., Remmert, R., Eds., Springer-Verlag, (1994).

  14. Hartogs, F.Einige Folgerungen aus der Cauchyschen Integralformel bei Funktionen mehrerer Veränderlichen, Bayerische Akademie der Wissenschaften. Mathematish-Physikalisch Klasse,36, 223–292, (1906).

    Google Scholar 

  15. Henkin, G. M.The Method of Integral Representations in Complex Analysis, (Russian), Current problems in mathematics. Fundamental directions7, 23–124, 258, Itogi Nauki i Tekhniki, Akad. Nauk SSSR, Vsesoyuz. Inst. Nauchn. i Tekhn. Inform, Moscow, (1985).

  16. Henkin, G. M. and Leiterer, J. Theory of functions on complex manifolds,Mon. Math. 79, Birkhäuser, Basel-Boston, Mass., 226 pp, (1984).

    Google Scholar 

  17. Henkin, G. M. and Leiterer, J. Andreotti-Grauert theory by integral formulas,Progr. Math. 74, Birkhäuser, Boston-Basel, (1988).

    Google Scholar 

  18. Henkin, G. M. and Michel, V. Principe de Hartogs dans les variétés CR,J. Math. Pures Appl. (9) 81(12), 1313–1395, (2002).

    MathSciNet  MATH  Google Scholar 

  19. Hirsch, M. W.Differential Topology, Graduate Texts in Mathematics,33, Springer-Verlag, Berlin, 222, (1976).

    Google Scholar 

  20. Hörmander, L.An Introduction to Complex Analysis in Several Variables, Van Nostrand, Princeton 1966, 3rd ed., North Holland Publ., Amsterdam-New York, (1990).

    Google Scholar 

  21. Hurwitz, A. Überdie Entwicklung der allgemeinen Theorie der analytischen Funktionen in neuerer Zeit, in Proc. 1st International Congress of Mathematicians, Zürich, 91–112, (1897–1898).

  22. Jarnicki, J. and Pflug, P.Extension of Holomorphic Functions, De Gruyter Expositions, in Mathematics, 34, De Gruyter, Berlin, 487, (2000).

  23. Jöricke, B. and Porten, E.Hulls and analytic extension from nonpseudoconvex boundaries, U.U.M.D. Report2002(19), Uppsala University, 38 pp, (2002).

  24. Kneser, H. Die Randwerte einer analytischen Funktion zweier Veränderlichen,Monats. f. Math. u. Phys. 43, 364–380, (1936).

    Article  MathSciNet  MATH  Google Scholar 

  25. Levi, E. E. Sulle ipersuperficie dello spazio a 4 dimensioni che possono essere frontiera del campo di esistenza di una funzione analitica di due variabili complesse,Annali di Mat. (3) 18, 69–79, (1911).

    Google Scholar 

  26. Lieb, I. and Michel,J. The Cauchy-Riemann Complex. Integral Formulae and Neumann Problem, Aspects of Mathematics, E34. FriEd., Vieweg & Sohn, Braunschweig, 362, (2002).

  27. Martinelli, E. Alcuni teoremi integrali per le funzioni per le funzioni analitiche di più variabili complesse,Mem. delta R. Accad. d’Italia 9, 269–283, (1938).

    Google Scholar 

  28. Martinelli, E. Sopra una dimostrazione di R. Fueter per un teorema di Hartogs,Comm. Math. Helv. 15, 340–349, (1942–1943).

    Article  MathSciNet  Google Scholar 

  29. Merker, J. and Porten, P.Holomorphic Extension of CR Functions, Envelopes of Holomorphy and Removable Singularities, International Mathematics Research Surveys, Vol.2006, Article ID 28925, 287, math. CV/ 0701531.

  30. Merker, J. and Porten, P. The Hartogs extension theorem on (n −1)complete complex spaces, http:// arxiv.org/abs/0704.3216.

  31. Milnor, J.Morse Theory, Based on lectures notes by M. Spivak and R. Wells, Annals of mathematical Studies, No. 51, Princeton University Press, Princeton, NJ. 153, (1963).

    Google Scholar 

  32. Osgood, W. F.Lehrbuch der Funktionentheorie, Bd II, B.G. Teubner, Leipzig, (1929).

    Google Scholar 

  33. Range, M.Holomorphic Functions and Integral Representations in Several Complex Variables, Graduate Texts in Mathematics,108, Springer-Verlag, New York, 386, (1986).

    Google Scholar 

  34. Range, M. Extension phenomena in multidimensional complex analysis: Correction of the historical record,Math. Intelligencer 24(2), 4–12, (2002).

    Article  MathSciNet  Google Scholar 

  35. Struppa, D.The First Eighty Years of Hartogs’ Theorem, Geometry Seminars, 1987–1988, Bologna 1987–1988, 127–209, Univ. Stud. Bologna, Bologna, (1988).

Download references

Author information

Authors and Affiliations

  1. Département de Mathématiques et Applications, UMR 8553 du CNRS, École Normale Supérieure, 45 rue d’Ulm, F-75230, Paris Cedex 05, France

    Joël Merker & Egmont Porten

  2. Department of Engineering, Physics and Mathematics, Mid Sweden University, Campus Sundsvall, S-85170, Sundsvall, Sweden

    Joël Merker & Egmont Porten

Authors
  1. Joël Merker
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Egmont Porten
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Joël Merker.

Additional information

Communicated by Steven Krantz

Rights and permissions

Reprints and permissions

About this article

Cite this article

Merker, J., Porten, E. A Morse-theoretical proof of the Hartogs extension theorem. J Geom Anal 17, 513–546 (2007). https://doi.org/10.1007/BF02922095

Download citation

  • Received:

  • Issue Date:

  • DOI: https://doi.org/10.1007/BF02922095

Math Subject Classifications

Key Words and Phrases

Search

Navigation