Mathematical Modeling of a Carrier-Mediated Transport Process in a Liquid Membrane

Abstract

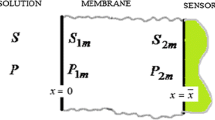
An analysis of the reaction diffusion in a carrier-mediated transport process through a membrane is presented. A simple approximate analytical expression of concentration profiles is derived in terms of all dimensionless parameters. Furthermore, in this work we employ the homotopy perturbation method to solve the nonlinear reaction–diffusion equations. Moreover, the analytical results have been compared to the numerical simulation using the Matlab program. The simulated results are comparable with the appropriate theories. The results obtained in this work are valid for the entire solution domain.

Appendices

Appendix 1: Approximate Analytical Solution of the Concentration Species Using the Homotopy Perturbation Method

In this appendix, we derive the solution of nonlinear reaction Eqs. 8–10 using He’s HPM. To illustrate the basic concepts of this method, we consider the following nonlinear differential equation (Ghori et al. 2007; Ozis and Yildirim 2007; Li and Liu 2006; Mousa and Ragab 2008):

$$L(u) + N(u) - f(r) = 0 $$

(16)

where L is a linear operator, N is a nonlinear operator and f(r) is a given continuous function. We construct a homotopy $\Upomega \times [0,1] \to R$ which satisfies

$$(1 - p)\,\frac{{d^{2} C_{S}^{ * } \,}}{{dX^{2} }} + p(\frac{{d^{2} C_{S}^{ * } }}{{dX^{2} }} - MC_{S}^{ * } C_{L}^{ * } + NC_{LS}^{ * } ) = 0 $$

(17)

$$(1 - p)\,\frac{{d^{2} C_{L}^{ * } \,}}{{dX^{2} }} + p(\frac{{d^{2} C_{L}^{ * } }}{{dX^{2} }} - MC_{S}^{ * } C_{L}^{ * } + NC_{LS}^{ * } ) = 0 $$

(18)

$$(1 - p)\,(\frac{{d^{2} C_{LS}^{ * } }}{{dX^{2} }} - NC_{LS}^{ * } ) + p(\frac{{d^{2} C_{LS}^{ * } }}{{dX^{2} }} + MC_{S}^{ * } C_{L}^{ * } - NC_{LS}^{ * } ) = 0 $$

(19)

Suppose the approximate solutions of Eqs. 17–19 have the form

$$\left\{ \begin{gathered} C_{S}^{ * } \, = \, C_{S0}^{ * } + \, pC_{S1}^{ * } \, + \, p^{2} C_{S2}^{ * } + \ldots \hfill \\ C_{L}^{ * } \, = \, C_{L,\,0}^{ * } \, + \, pC_{L,\,1}^{ * } \, + \, p^{2} C_{L\,2}^{ * } + \ldots \hfill \\ C_{LS}^{ * } \, = \, C_{LS0}^{ * } + \, pC_{LS\,1}^{ * } + \, p^{2} C_{LS\,2}^{ * } + \ldots \hfill \\ \end{gathered} \right. $$

(20)

Substituting Eq. 20 into Eqs. 17–19 and equating the terms with the identical powers of p, we obtain

$$p^{0} :\frac{{d^{2} C_{S,\,0}^{ * } }}{{dX^{2} }} = 0 $$

(21)

$$p^{1} :\frac{{d^{2} C_{S,\,1}^{ * } }}{{dX^{2} }} - MC_{S,\,1}^{ * } C_{L,\,0}^{ * } + NC_{LS,\,0}^{ * } = 0 $$

(22)

and

$$p^{0} :\frac{{d^{2} C_{L,\,0}^{ * } }}{{dX^{2} }} = 0 $$

(23)

$$p^{1} :\frac{{d^{2} C_{L,\,1}^{ * } }}{{dX^{2} }} - MC_{S,\,0}^{ * } C_{L,\,0}^{ * } \, + NC_{LS,\,0}^{ * } = 0 $$

(24)

and

$$p^{0} :\frac{{d^{2} C_{LS,\,0}^{ * } }}{{dX^{2} }} - NC_{LS,\,0}^{ * } = 0 $$

(25)

$$p^{1} :\frac{{d^{2} C_{LS,\,1}^{ * } }}{{dX^{2} }} + MC_{S,\,0}^{ * } C_{L,\,0}^{ * } - NC_{LS,\,1}^{ * } = 0 $$

(26)

The initial conditions are as follows:

$$C_{S,0}^{ * } (X = 0) = 1; \, C_{S,0}^{ * } (X = 1) = 0 \, $$

(27)

$$C_{{L,{ 0}}}^{ * } (X = 0) = 0; \, C_{{L,{ 0}}}^{ * } (X = 1) = 1 \, $$

(28)

$$C_{{LS,{ 0}}}^{ * } (X = 0) = 1; \, C_{{LS,{ 0}}}^{ * } (X = 1) = 0 \, $$

(29)

and

$$C_{{S,{\text{ i}}}}^{ * } (X = 0 )= 0; \, C_{{S,{\text{ i}}}}^{ * } (X = 1 )= 0\;{\text{for all }}i = { 1},{ 2},{ 3} \ldots $$

(30)

$$C_{{L,{\text{ i}}}}^{ * } (X = 0 )= 0; \, C_{{L,{\text{ i}}}}^{ * } (X = 1 )= 0\;{\text{for all }}i = { 1},{ 2},{ 3} \ldots $$

(31)

$$C_{{LS,{\text{ i}}}}^{ * } (X = 0 )= 0; \, C_{{LS,{\text{ i}}}}^{ * } (X = 1 )= 0\;{\text{for all }}i = { 1},{ 2},{ 3} \ldots $$

(32)

Solving Eqs. 21, 23 and 25 and using the boundary condition Eqs. 27–29, we get

$$C_{S,\,0}^{ * } (X) = 1 - X $$

(34)

$$C_{L,\,0}^{ * } (X) = X $$

(35)

$$C_{LS,\,0}^{*} (X) = \frac{\sinh \sqrt N (1 - X)}{\sinh \sqrt N } $$

(36)

Substituting the above values of $C_{S,0}^{*} ,\,C_{L,0}^{*}$ and $C_{LS,\,0}^{ * }$ and solving Eqs. 22, 24 and 26 using the boundary condition Eqs. 30–32, we obtain the following results:

$$C_{S,1}^{*} (X) = {\kern 1pt} M\left( {\frac{{X^{3} }}{6} - \frac{{X^{4} }}{12}} \right) - \frac{\sinh \sqrt N (1 - X)}{\sinh \sqrt N } - {\kern 1pt} \left( {\frac{M}{2} + 1} \right)X + 1 $$

(37)

$$C_{L,1}^{*} (X) = {\kern 1pt} M\left( {\frac{{X^{3} }}{6} - \frac{{X^{4} }}{4}} \right) - \frac{\sinh \sqrt N (1 - X)}{\sinh \sqrt N } - {\kern 1pt} \left( {\frac{M}{2} + 1} \right)X + 1 $$

(38)

$$C_{L,S,1}^{*} (X)\, = \,{\kern 1pt} \frac{2M}{{N^{2} \sinh \sqrt N }}\left( {\sinh \sqrt N (1 - X) + \sinh \sqrt N X} \right) + \frac{M}{N}\left( {X - X^{2} - \frac{2}{N}} \right) $$

(39)

Adding Eqs. 34 and 37, we get Eq. 13 in the text. Similarly, we can get Eqs. 14 and 15.

Appendix 2: Matlab Program to Find the Numerical Solution of Equations 8–10

function pdex4

m = 0;

x = linspace(0,1);

t = linspace(0,1000);

sol = pdepe(m,@pdex4pde,@pdex4ic,@pdex4bc,x,t);

u1 = sol(:,:,1);

u2 = sol(:,:,2);

u3 = sol(:,:,3);

figure

plot(x,u1(end,:))

title(‘u1(x,t)’)

% ———————————————–

function (c,f,s) = pdex4pde(x,t,u,DuDx)

M = 0.1;

N = 1;

c = (1; 1; 1);

f = (1; 1; 1).* DuDx;

F1 = –M*u(1)*u(2) + N*u(3);

F2 = –M*u(1)*u(2) + N*u(3);

F3 = M*u(1)*u(2)–N*u(3);

s = (F1; F2; F3);

% ———————————————–

function u0 = pdex4ic(x);

u0 = (1; 0;0);

% ———————————————–

function (pl,ql,pr,qr) = pdex4bc(xl,ul,xr,ur,t)

pl = (ul(1)–1; ul(2);ul(3)–1);

ql = (0;0; 0);

pr = (ur(1); ur(2)–1; ur(3));

qr = (0; 0; 0);