No CrossRef data available.
Article contents
The Ample Cone of the Kontsevich Moduli Space
Published online by Cambridge University Press: 20 November 2018
Abstract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
We produce ample (resp. NEF, eventually free) divisors in the Kontsevich space
${{\overline{\mathcal{M}}}_{\text{0,}n}}\left( {{\mathbb{P}}^{r}},d \right)$
of 
$n$-pointed, genus 0, stable maps to 
${{\mathbb{P}}^{r}}$, given such divisors in
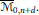${{\overline{\mathcal{M}}}_{\text{0,}n+d}}$ We prove that this produces all ample (resp. NEF, eventually free) divisors in ${{\overline{\mathcal{M}}}_{\text{0,}n}}\left( {{\mathbb{P}}^{r}},\,d \right)$ As a consequence, we construct a contraction of the boundary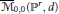 
$\,\,\mathop{\bigcup }_{k=1}^{\left\lfloor {d}/{2}\; \right\rfloor }\,{{\Delta }_{k,d-k}}$ in 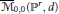${{\overline{\mathcal{M}}}_{\text{0,}0}}\left( {{\mathbb{P}}^{r}},d \right)$
analogous to a contraction of the boundary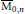 
$\mathop{\bigcup }_{k=3}^{\left\lfloor {n}/{2}\; \right\rfloor }\,{{\widetilde{\Delta }}_{k,n-k}}$ in 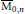${{\overline{\mathcal{M}}}_{\text{0,}n}}$ first constructed by Keel and McKernan.
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2009
References
[CHS]
Coskun, I., Harris, J., and Starr, J., The effective cone of the Kontsevich moduli space.
Canad. Math. Bull., to appear. http://www.math.uic.edu/˜coskun/reveff.pdf
Google Scholar
[dJS]
Jong, A.J. de and Starr, J., Divisor classes and the virtual canonical bundle.
http://arxiv.org/abs/math/0602642
Google Scholar
[Ful]
Fulton, W., Intersection Theory, Second edition. Ergebnisse der Mathematik und ihrer Grenzgebiete. 3. Folge. A Series of Modern Surveys in Mathematics. Springer-Verlag, Berlin, 1998.Google Scholar
[Ha]
Hartshorne, R.,, Algebraic Geometry. Graduate Texts in Mathematics 52, Springer-Verlag, New York, 1977.Google Scholar
[Has]
Hassett, B.,Moduli spaces of weighted pointed stable curves. Adv. Math. 173 (2003), no. 2, 316–352.Google Scholar
[Kap]
Kapranov, M. M., Veronese curves and Grothendieck-Knudsen moduli space M0,n. J. Algebraic Geom. 2 (1993), no. 2, 239–262.Google Scholar
[Kaw]
Kawamata, Y., Subadjunction of log canonical divisors for a subvariety of codimension 2. In: Birational Algebraic Geometry. Contemp. Math. 207, American Mathematical Society, Providence, RI, 1997, pp. 79–88.Google Scholar
[KM]
Keel, S. and McKernan, J., Contractible extremal rays on M0,n. Preprint, 1996. http://arxiv.org/abs/alg-geom/9607009.
Google Scholar
[Pa]
Pandharipande, R., Intersections of
Q
-divisors on Kontsevich's moduli space M0,n(P
r, d) and enumerative geometry. Trans. Amer. Math. Soc. 351 (1999), 1481–1505.Google Scholar
[Par]
Parker, A., An elementary GIT construction of the moduli space of stable maps. Ph.D. thesis, University of Texas at Austin, 2005.Google Scholar
You have
Access