Skip to main content
SpringerLink
Log in

Chaos and Hyperchaos in Two Coupled Identical Hindmarsh – Rose Systems

  • Published:
Regular and Chaotic Dynamics Aims and scope Submit manuscript

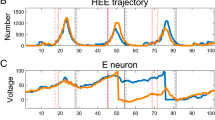
Abstract

The dynamics of two coupled neuron models, the Hindmarsh – Rose systems, are studied. Their interaction is simulated via a chemical coupling that is implemented with a sigmoid function. It is shown that the model may exhibit complex behavior: quasi-periodic, chaotic and hyperchaotic oscillations. A phenomenological scenario for the formation of hyperchaos associated with the appearance of a discrete Shilnikov attractor is described. It is shown that the formation of these attractors leads to the appearance of in-phase bursting oscillations.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7

Similar content being viewed by others

Notes

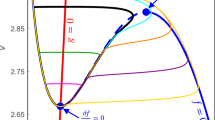
  1. Using the symbol \(C\)(m,n) we denote the limit cycle, the indices in brackets indicate the dimension of the stable (m) and unstable (n) manifolds of the corresponding point in the Poincaré section

  2. In our numerical experiments, we used the fixed initial conditions: \(x_{10}=1.12\), \(y_{10}=2.67\), \(z_{10}=-0.42\), \(x_{20}=-0.91\), \(y_{20}=3.54\), \(z_{20}=-0.38\)

References

  1. Kuramoto, Y., Chemical Oscillations, Waves and Turbulence, Berlin: Springer, 1984.

    Google Scholar 

  2. Litvak, A. G. and Tokman, M. D., Electromagnetically Induced Transparency in Ensembles of Classical Oscillators, Phys. Rev. Lett., 2002, vol. 88, no. 9, 095003, 4 pp.

    ADS  CAS  PubMed  Google Scholar 

  3. Moskalenko, O. I., Koronovskii, A. A., Hramov, A. E., and Boccaletti, S., Generalized Synchronization in Mutually Coupled Oscillators and Complex Networks, Phys. Rev. E, 2012, vol. 86, no. 3, 036216, 9 pp.

    ADS  Google Scholar 

  4. Zakharova, A., Chimera Patterns in Networks: Interplay between Dynamics, Structure, Noise, and Delay, Cham: Springer, 2020.

    Google Scholar 

  5. Pikovsky, A., Rosenblum, M., and Kurths, J., Synchronization: A Universal Concept in Nonlinear Sciences, Cambridge Nonlinear Sci. Ser., vol. 12, New York: Cambridge Univ. Press, 2001.

    Google Scholar 

  6. Mosekilde, E., Maistrenko, Yu., and Postnov, D., Chaotic Synchronization: Applications to Living Systems, World Sci. Ser. Nonlinear Sci. Ser. A Monogr. Treatises, vol. 42, River Edge, N.J.: World Sci. Publ., 2002.

    Google Scholar 

  7. González-Miranda, J. M., Synchronization and Control of Chaos: An Introduction for Scientists and Engineers, London: Imperial College Press, 2004.

    Google Scholar 

  8. Rössler, O. E., An Equation for Hyperchaos, Phys. Lett. A, 1979, vol. 71, no. 2, pp. 155–157.

    ADS  MathSciNet  Google Scholar 

  9. Cannas, B. and Cincotti, S., Hyperchaotic Behaviour of Two Bi-Directionally Coupled Chua Circuits, Int. J. Circuit Theory Appl., 2002, vol. 30, no. 6, pp. 625–637.

    Google Scholar 

  10. Rasmussen, J., Mosekilde, E., and Reick, C., Bifurcations in Two Coupled Rössler Systems, Math. Comput. Simul., 1996, vol. 40, no. 3–4, pp. 247–270.

    Google Scholar 

  11. Postnov, D., Vadivasova, T., Sosnovtseva, O., Balanov, A., Anishchenko, V., and Mosekilde, E., Role of Multistability in the Transition to Chaotic Phase Synchronization, Chaos, 1999, vol. 9, no. 1, pp. 227–232.

    ADS  PubMed  Google Scholar 

  12. Yanchuk, S. and Kapitaniak, T., Chaos–Hyperchaos Transition in Coupled Rössler Systems, Phys. Lett. A, 2001, vol. 290, no. 3–4, pp. 139–144.

    ADS  CAS  Google Scholar 

  13. Čenys, A., Tamaševičius, A., Baziliauskas, A., Krivickas, R., and Lindberg, E., Hyperchaos in Coupled Colpitts Oscillators, Chaos Solitons Fractals, 2003, vol. 17, no. 2–3, pp. 349–353.

    ADS  Google Scholar 

  14. Stankevich, N. V., Dvorak, A., Astakhov, V., Jaros, P., Kapitaniak, M., Perlikowski, P., and Kapitaniak, T., Chaos and Hyperchaos in Coupled Antiphase Driven Toda Oscillators, Regul. Chaotic Dyn., 2018, vol. 23, no. 1, pp. 120–126.

    ADS  MathSciNet  Google Scholar 

  15. Perlikowski, P., Yanchuk, S., Wolfrum, M., Stefanski, A., Mosiolek, P., and Kapitaniak, T., Routes to Complex Dynamics in a Ring of Unidirectionally Coupled Systems, Chaos, 2010, vol. 20, no. 1, 013111, 10 pp.

    ADS  MathSciNet  CAS  PubMed  Google Scholar 

  16. Stankevich, N. and Volkov, E., Chaos–Hyperchaos Transition in Three Identical Quorum-Sensing Mean-Field Coupled Ring Oscillators, Chaos, 2021, vol. 31, no. 10, 103112, 12 pp.

    ADS  MathSciNet  CAS  PubMed  Google Scholar 

  17. Stankevich, N. V., Kuznetsov, A. P., and Seleznev, E. P., Chaos and Hyperchaos Arising from the Destruction of Multifrequency Tori, Chaos Solitons Fractals, 2021, vol. 147, Paper No. 110998, 8 pp.

    MathSciNet  Google Scholar 

  18. Rinzel, J., A Formal Classification of Bursting Mechanisms in Excitable Systems, in Mathematical Topics in Population Biology, Morphogenesis and Neurosciences, E. Teramoto, M. Yumaguti (Eds.), Lecture Notes in Biomath., vol. 71, Berlin: Springer, 1987.

    Google Scholar 

  19. Izhikevich, E. M., Neural Excitability, Spiking and Bursting, Internat. J. Bifur. Chaos Appl. Sci. Engrg., 2000, vol. 10, no. 6, pp. 1171–1266.

    MathSciNet  Google Scholar 

  20. Shilnikov, A. and Cymbalyuk, G., Transition between Tonic Spiking and Bursting in a Neuron Model via the Blue-Sky Catastrophe, Phys. Rev. Lett., 2005, vol. 94, no. 4, 048101, 4 pp.

    ADS  PubMed  Google Scholar 

  21. Shilnikov, A., Calabrese, R. L., and Cymbalyuk, G., Mechanism of Bistability: Tonic Spiking and Bursting in a Neuron Model, Phys. Rev. E (3), 2005, vol. 71, no. 5, 056214, 9 pp.

    ADS  MathSciNet  Google Scholar 

  22. Izhikevich, E. M., Dynamical Systems in Neuroscience: The Geometry of Excitability and Bursting, Cambridge, Mass.: MIT Press, 2007.

    Google Scholar 

  23. Pankratova, E. V. and Kalyakulina, A. I., Environmentally Induced Amplitude Death and Firing Provocation in Large-Scale Networks of Neuronal Systems, Regul. Chaotic Dyn., 2016, vol. 21, no. 7–8, pp. 840–848.

    ADS  MathSciNet  Google Scholar 

  24. Koronovskii, A. A., Hramov, A. E., Grubov, V. V., Moskalenko, O. I., Sitnikova, E. Yu., and Pavlov, A. N., Coexistence of Intermittencies in the Neuronal Network of the Epileptic Brain, Phys. Rev. E, 2016, vol. 93, no. 3, 032220, 5 pp.

    ADS  MathSciNet  PubMed  Google Scholar 

  25. Nikitin, D., Omelchenko, I., Zakharova, A., Avetyan, M., Fradkov, A. L., and Schöll, E., Complex Partial Synchronization Patterns in Networks of Delay-Coupled Neurons, Philos. Trans. Roy. Soc. A, 2019, vol. 377, no. 2153, 20180128, 19 pp.

    ADS  MathSciNet  CAS  Google Scholar 

  26. Ruzzene, G. Omelchenko, I., Sawicki, J., Zakharova, A., Schöll, E., and Andrzejak, R. G., Remote Pacemaker Control of Chimera States in Multilayer Networks of Neurons, Phys. Rev. E, 2020, vol. 102, no. 5, 052216, 9 pp.

    ADS  MathSciNet  CAS  PubMed  Google Scholar 

  27. Pankratova, E. V., Kalyakulina, A. I., Stasenko, S. V., Gordleeva, S. Yu., Lazarevich, I. A., and Kazantsev, V. B., Neuronal Synchronization Enhanced by Neuron-Astrocyte Interaction, Nonlinear Dyn., 2019, vol. 97, no. 1, pp. 647–662.

    Google Scholar 

  28. Belykh, I. and Shilnikov, A., When Weak Inhibition Synchronizes Strongly Desynchronizing Networks of Bursting Neurons, Phys. Rev. Lett., 2008, vol. 101, no. 7, 078102, 4 pp.

    ADS  PubMed  Google Scholar 

  29. Jalil, S., Belykh, I., and Shilnikov, A., Fast Reciprocal Inhibition Can Synchronize Bursting Neurons, Phys. Rev. E (3), 2010, vol. 81, no. 4, 045201, 4 pp.

    ADS  MathSciNet  Google Scholar 

  30. Reimbayev, R. and Belykh, I., When Transitions between Bursting Modes Induce Neural Synchrony, Internat. J. Bifur. Chaos Appl. Sci. Engrg., 2014, vol. 24, no. 8, 1440013, 9 pp.

    MathSciNet  Google Scholar 

  31. Belykh, I., Reimbayev, R., and Zhao, K., Synergistic Effect of Repulsive Inhibition in Synchronization of Excitatory Networks, Phys. Rev. E (3), 2015, vol. 91, no. 6, 062919, 10 pp.

    ADS  MathSciNet  Google Scholar 

  32. Reimbayev, R., Daley, K., and Belykh, I., When Two Wrongs Make a Right: Synchronized Neuronal Bursting from Combined Electrical and Inhibitory Coupling, Philos. Trans. Roy. Soc. A, 2017, vol. 375, no. 2096, 20160282, 19 pp.

    ADS  MathSciNet  Google Scholar 

  33. Chay, T. R., Effects of Extracellular Calcium on Electrical Bursting and Intracellular and Luminal Calcium Oscillations in Insulin Secreting Pancreatic Beta-Cells, Biophys. J., 1997, vol. 73, no. 3, pp. 1673–1688.

    ADS  CAS  PubMed  PubMed Central  Google Scholar 

  34. Zeldenrust, F., Wadman, W. J., and Englitz, B., Neural Coding with Bursts-Current State and Future Perspectives, Front. Comput. Neurosci., 2018, vol. 12, Art. 8, 14 pp.

    PubMed  PubMed Central  Google Scholar 

  35. Gibson, J. R., Beierlein, M., and Connors, B. W., Two Networks of Electrically Coupled Inhibitory Neurons in Neocortex, Nature, 1999, vol. 402, no. 6757, pp. 75–79.

    ADS  CAS  PubMed  Google Scholar 

  36. Hindmarsh, J. L. and Rose, R. M., A Model of Neuronal Bursting Using Three Coupled First Order Differential Equations, Proc. R. Soc. Lond. Ser. B Biol. Sci., 1984, vol. 221, no. 1222, pp. 87–102.

    ADS  CAS  Google Scholar 

  37. Shilnikov, A. and Kolomiets, M., Methods of the Qualitative Theory for the Hindmarsh – Rose Model: A Case Study. A Tutorial, Internat. J. Bifur. Chaos Appl. Sci. Engrg., 2008, vol. 18, no. 8, pp. 2141–2168.

    MathSciNet  Google Scholar 

  38. Barrio, R., Angeles Martínez, M., Serrano, S., and Shilnikov, A., Macro- and Micro-Chaotic Structures in the Hindmarsh – Rose Model of Bursting Neurons, Chaos, 2014, vol. 24, no. 2, 023128, 11 pp.

    ADS  MathSciNet  PubMed  Google Scholar 

  39. Garashchuk, I. R., Asynchronous Chaos and Bifurcations in a Model of Two Coupled Identical Hindmarsh – Rose Neurons, Russian J. Nonlinear Dyn., 2021, vol. 17, no. 3, pp. 307–320.

    MathSciNet  Google Scholar 

  40. Garashchuk, I. R. and Sinelshchikov, D. I., Excitation of a Group of Two Hindmarsh – Rose Neurons with a Neuron-Generated Signal, Russian J. Nonlinear Dyn., 2023, vol. 19, no. 1, pp. 19–34.

    MathSciNet  Google Scholar 

  41. Benettin, G., Galgani, L., Giorgilli, A., and Strelcyn, J.-M., Lyapunov Characteristic Exponents for Smooth Dynamical Systems and for Hamiltonian Systems: A Method for Computing All of Them: P. 1: Theory, Meccanica, 1980, vol. 15, no. 1, pp. 9–20.

    ADS  Google Scholar 

  42. Ermentrout, G. B., Simulating, Analyzing, and Animating Dynamical Systems: A Guide to XPPAUT for Researchers and Students, Software, Environments, and Tools, vol. 14, Philadelphia, Pa.: SIAM, 2002.

    Google Scholar 

  43. Gonchenko, A. S., Gonchenko, S. V., and Shilnikov, L. P., Towards Scenarios of Chaos Appearance in Three-Dimensional Maps, Nelin. Dinam., 2012, vol. 8, no. 1, pp. 3–28 (Russian).

    Google Scholar 

  44. Gonchenko, A. S., Gonchenko, S. V., Kazakov, A. O., and Turaev, D. V., Simple Scenarios of Onset of Chaos in Three-Dimensional Maps, Internat. J. Bifur. Chaos Appl. Sci. Engrg., 2014, vol. 24, no. 8, 1440005, 25 pp.

    MathSciNet  Google Scholar 

  45. Garashchuk, I. R., Sinelshchikov, D. I., Kazakov, A. O., and Kudryashov, N. A., Hyperchaos and Multistability in the Model of Two Interacting Microbubble Contrast Agents, Chaos, 2019, vol. 29, no. 6, 063131, 16 pp.

    ADS  PubMed  Google Scholar 

  46. Stankevich, N., Kuznetsov, A., Popova, E., and Seleznev, E., Chaos and Hyperchaos via Secondary Neimark – Sacker Bifurcation in a Model of Radiophysical Generator, Nonlinear Dyn., 2019, vol. 97, no. 4, pp. 2355–2370.

    Google Scholar 

  47. Stankevich, N., Kazakov, A., and Gonchenko, S., Scenarios of Hyperchaos Occurrence in 4D Rössler System, Chaos, 2020, vol. 30, no. 12, 123129, 16 pp.

    ADS  MathSciNet  CAS  PubMed  Google Scholar 

  48. Sataev, I. R. and Stankevich, N. V., Cascade of Torus Birth Bifurcations and Inverse Cascade of Shilnikov Attractors Merging at the Threshold of Hyperchaos, Chaos, 2021, vol. 31, no. 2, Paper No. 023140, 8 pp.

    ADS  MathSciNet  CAS  PubMed  Google Scholar 

  49. Shykhmamedov, A., Karatetskaia, E., Kazakov, A., and Stankevich, N., Scenarios for the Creation of Hypercaotic Attractors in 3D Maps, Nonlinearity, 2023, vol. 36, no. 7, pp. 3501–3541.

    ADS  MathSciNet  Google Scholar 

  50. French, A. P., The Superposition of Periodic Motions, in Vibrations and Waves, Boca Raton, Fla.: CRC, 1971, pp. 19-39.

    Google Scholar 

  51. Rulkov, N. F. and Lewis, C. T., Subharmonic Destruction of Generalized Chaos Synchronization, Phys. Rev. E, 2001, vol. 63, no. 6, 065204, 4 pp.

    ADS  CAS  Google Scholar 

  52. Palmer, K., Ridgway, T., Al-Rawi, O., Johnson, I., and Poullis, M., Lissajous Figures: An Engineering Tool for Root Cause Analysis of Individual Cases: A Preliminary Concept, J. Extra Corpor. Technol., 2011, vol. 43, no. 3, pp. 153–156.

    PubMed  PubMed Central  Google Scholar 

  53. Stankovski, T., Analogue Simulation and Synchronization Analysis of Non-Autonomous Oscillators, in Tackling the Inverse Problem for Non-Autonomous Systems: Application to the Life Sciences, Cham: Springer, 2014, pp. 109-117.

    Google Scholar 

Download references

ACKNOWLEDGMENTS

The authors thank Dr. Alexey Kazakov and Prof. Igor Belykh for useful discussions of this problem.

Funding

This work is supported by the Russian Science Foundation (project no. 20-71-10048, Sections 2.2, 3, 5). NVS, AAB are partially supported by the Laboratory of Dynamical Systems and Applications NRU HSE, grant of the Ministry of Science and Higher Education of the RF, ag. no. 075-15-2022-1101 (Sections 2.1, 4).

Author information

Authors and Affiliations

  1. HSE University, ul. Bolshaya Pecherskaya 25/12, 603155, Nizhny Novgorod, Russia

    Nataliya V. Stankevich, Andrey A. Bobrovskii & Natalya A. Shchegoleva

Authors
  1. Nataliya V. Stankevich
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Andrey A. Bobrovskii
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Natalya A. Shchegoleva
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding authors

Correspondence to Nataliya V. Stankevich, Andrey A. Bobrovskii or Natalya A. Shchegoleva.

Ethics declarations

The authors declare that they have no conflicts of interest.

Additional information

PUBLISHER’S NOTE

Pleiades Publishing remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

MSC2010

65P20, 92B25

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Stankevich, N.V., Bobrovskii, A.A. & Shchegoleva, N.A. Chaos and Hyperchaos in Two Coupled Identical Hindmarsh – Rose Systems. Regul. Chaot. Dyn. 29, 120–133 (2024). https://doi.org/10.1134/S1560354723540031

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1134/S1560354723540031

Keywords

Search

Navigation