Hostname: page-component-848d4c4894-ttngx Total loading time: 0 Render date: 2024-05-21T17:58:17.428Z Has data issue: false hasContentIssue false
  • English
  • Français

A CONJECTURE OF MERCA ON CONGRUENCES MODULO POWERS OF 2 FOR PARTITIONS INTO DISTINCT PARTS

Part of: Combinatorics Additive number theory; partitions

Published online by Cambridge University Press:  27 March 2023

JULIA Q. D. DU  and
DAZHAO TANG Open the ORCID record for DAZHAO TANG [Opens in a new window]
JULIA Q. D. DU
Affiliation:
School of Mathematical Sciences, Hebei International Joint Research Center for Mathematics and Interdisciplinary Science, Hebei Normal University, Shijiazhuang 050024, PR China e-mail: qddu@hebtu.edu.cn
DAZHAO TANG*
Affiliation:
School of Mathematical Sciences, Chongqing Normal University, Chongqing 401331, PR China
*
e-mail: dazhaotang@sina.com
Get access Rights & Permissions [Opens in a new window]

Abstract

Let 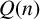$Q(n)$ denote the number of partitions of n into distinct parts. Merca [‘Ramanujan-type congruences modulo 4 for partitions into distinct parts’, An. Şt. Univ. Ovidius Constanţa 30(3) (2022), 185–199] derived some congruences modulo $4$ and $8$ for 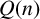$Q(n)$ and posed a conjecture on congruences modulo powers of 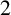$2$ enjoyed by 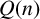$Q(n)$. We present an approach which can be used to prove a family of internal congruence relations modulo powers of 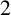$2$ concerning 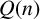$Q(n)$. As an immediate consequence, we not only prove Merca’s conjecture, but also derive many internal congruences modulo powers of 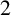$2$ satisfied by 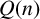$Q(n)$. Moreover, we establish an infinite family of congruence relations modulo 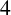$4$ for 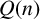$Q(n)$.

Keywords

congruencesinternal congruencespartitionsdistinct partsmodular forms

MSC classification

Primary: 11P83: Partitions; congruences and congruential restrictions
Secondary: 05A17: Partitions of integers
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 109 , Issue 1 , February 2024 , pp. 26 - 36
Check for updates
Copyright
© The Author(s), 2023. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

The first author was partially supported by the National Natural Science Foundation of China (No. 12201177), the Natural Science Foundation of Hebei Province (No. A2021205018), the Science and Technology Research Project of Colleges and Universities of Hebei Province (No. BJK2023092), the Doctor Foundation of Hebei Normal University (No. L2021B02), the Program for Foreign Experts of Hebei Province and the Program for 100 Foreign Experts Plan of Hebei Province. The second author was partially supported by the National Natural Science Foundation of China (No. 12201093), the Natural Science Foundation Project of Chongqing CSTB (No. CSTB2022NSCQ–MSX0387), the Science and Technology Research Program of Chongqing Municipal Education Commission (No. KJQN202200509) and the Doctoral Start-up Research Foundation (No. 21XLB038) of Chongqing Normal University.

References

Andrews, G. E. and Berndt, B. C., Ramanujan’s Lost Notebook, Part II (Springer-Verlag, New York, 2005).CrossRefGoogle Scholar
Atkin, A. O. L., ‘Proof of a conjecture of Ramanujan’, Glasg. Math. J. 8 (1967), 14–32.CrossRefGoogle Scholar
Berndt, B. C., Ramanujan’s Notebook, Part III (Springer-Verlag, New York, 1991).CrossRefGoogle Scholar
Berndt, B. C. and Ono, K., ‘Ramanujan’s unpublished manuscipt on the partition and tau functions with proofs and commentary’, Sém. Lothar. Combin. 42 (1999), Article no. B42c.Google Scholar
Cui, S.-P. and Gu, N. S. S., ‘Arithmetic properties of $\ell$ -regular partitions’, Adv. in Appl. Math. 51(4) (2013), 507–523.CrossRefGoogle Scholar
Diamond, F. and Shurman, J., A First Course in Modular Forms, Graduate Texts in Mathematics, 228 (Springer-Verlag, New York, 2005).Google Scholar
Euler, L., Introductio in Analysin Infinitorum, Vol. 2 (MM Bousquet Hanson, Lausanne, 1748).Google Scholar
Gordon, B. and Ono, K., ‘Divisibility of certain partition functions by powers of primes’, Ramanujan J. 1(1) (1997), 25–34.CrossRefGoogle Scholar
Merca, M., ‘Ramanujan-type congruences modulo 4 for partitions into distinct parts’, An. Şt. Univ. Ovidius Constanţa 30(3) (2022), 185–199.Google Scholar
Ono, K., The Web of Modularity: Arithmetic of the Coefficients of Modular Forms and $q$ -Series , CBMS Regional Conference Series in Mathematics, 102 (American Mathematical Society, Providence, RI, 2004).Google Scholar
Paule, P. and Radu, S., ‘Partition analysis, modular functions, and computer algebra’, in: Recent Trends in Combinatorics, IMA Volumes in Mathematics and its Applications, 159 (eds. Beveridge, A., Griggs, J. R., Hogben, L., Musiker, G. and Tetali, P.) (Springer, Cham, 2016), 511–543.CrossRefGoogle Scholar
Radu, C.-S., ‘An algorithmic approach to Ramanujan–Kolberg identities’, J. Symbolic Comput. 68(1) (2015), 225–253.CrossRefGoogle Scholar
Smoot, N. A., ‘On the computation of identities relating partition numbers in arithmetic progressions with eta quotients: an implementation of Radu’s algorithm’, J. Symbolic Comput. 104 (2021), 276–311.CrossRefGoogle Scholar
Sturm, J., ‘On the congruence of modular forms’, in: Number Theory (eds. D. V. Chudnovsky, G. V. Chudnovsky, H. Cohn and M. B. Nathanson) (Springer, Berlin, 1987) 275–280.CrossRefGoogle Scholar
Watson, G. N., ‘Ramanujan’s vermutung über zerfällungsanzahlen’, J. reine angew. Math. 179 (1938), 97–128.CrossRefGoogle Scholar