Skip to main content
SpringerLink
Log in

Closed form solution of a periodically forced logistic model

  • Published:
ANNALI DELL'UNIVERSITA' DI FERRARA Aims and scope Submit manuscript

Abstract

We give the exact closed form solution of the following ordinary differential equation:

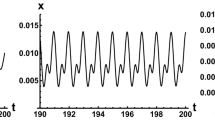
$$\left\{ \begin{array}{ll} \dot{x}(t)&=a\,x(t)-b\,x^{2}(t)+c\, {\rm cos} (\omega t)-h,\\ x(0)&=x_{0} \end{array}\right.$$

which is a modified logistic one, wherein x(t) is the population of a homogeneous species x at time t. Other than integrating the above nonlinear differential equation by means of Mathieu functions of the first kind, we also provide a condition of a couple of inequalities involving a, b, c, h and x 0 whose fulfillment is sufficient to ensure that a bounded solution for x(t) there exists.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  • Abramowitz M. and Stegun I.A. (1970). Handbook of Mathematical Functions. Dover, New York

    Google Scholar 

  • Banks R.B. (1994). Growth and Diffusion Phenomena. Springer Verlag, Berlin

    MATH  Google Scholar 

  • Bass F.M. (1969). A new product growth model for consumer durables. Manag. Sci. 15: 215–227

    Article  Google Scholar 

  • Boyce W.E. and Di Prima R.C. (1997). Elementary Differential Equations and Boundary Value Problems. Wiley, New York

    Google Scholar 

  • Campbell, R.: Théorie génerale de l’Èquation de Mathieu. Masson et Cie, Paris (1955)

  • Clark C.W. (1976). Mathematical Bioeconomics: The Optimal Management of Renewable Resources. Wiley, New York

    MATH  Google Scholar 

  • Cooke K.L. and Witten M. (1986). One-dimensional linear and logistic harvesting models. Math. Mod. 7: 301–340

    Article  MathSciNet  MATH  Google Scholar 

  • Erdèlyi A., Magnus W., Oberhettinger F. and Tricomi F.G. (1953). Higher Transcendental Functions. McGraw-Hill, New York

    Google Scholar 

  • Hale, J.K., Koçak, H.: Dynamics and Bifurcations. Berlin (1991)

  • Gause G.F., Smaragdova N.P. and Witt A.A. (1936). Further studies of interaction between predators and prey. J. Anim. Ecol. 5: 1–18

    Article  Google Scholar 

  • Hartman P. (2002). Ordinary Differential Equations. 2nd edn. SIAM, Philadelphia

    Google Scholar 

  • Hirsch M.W. and Smale S. (1974). Differential Equations, Dynamical Systems and Linear Algebra. Academic Press, New York

    MATH  Google Scholar 

  • Mathieu E. (1868). Mémoire sur le mouvement vibratoire d’une membrane de forme elliptique. J. Math. pure Appl. 13: 137–203

    Google Scholar 

  • McLachlan N.V. (1947). Theory and Application of Mathieu Functions. Clarendon Press, Oxford

    MATH  Google Scholar 

  • Sansone, G.: Equazioni Differenziali nel Campo Reale, Parte Seconda. Bologna (1949)

  • Verhulst, F.: Nonlinear Differential Equations and Dynamical Systems. Berlin (1990)

  • Verhulst P.F. (1838). Notice sur la loi que la population suit dans son accroissement. Correspondance Mathématique et Physique 10: 113–121

    Google Scholar 

  • Wang, Z.X., Guo, D.R.: Special Functions. World Scientific, Singapore (1989)

Download references

Author information

Authors and Affiliations

  1. Dipartimento di Matematica per le scienze economiche e sociali, Università di Bologna, viale Filopanti, 5, 40126, Bologna, Italy

    Giovanni Mingari Scarpello & Daniele Ritelli

Authors
  1. Giovanni Mingari Scarpello
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Daniele Ritelli
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Daniele Ritelli.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Mingari Scarpello, G., Ritelli, D. Closed form solution of a periodically forced logistic model. Ann. Univ. Ferrara 54, 85–94 (2008). https://doi.org/10.1007/s11565-008-0035-3

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11565-008-0035-3

Keywords

Mathematics Subject Classification (2000)

Search

Navigation