Abstract
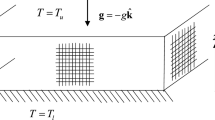
The linear stability of a viscoelastic fluid saturated densely packed horizontal porous layer heated from below and cooled from above is investigated by considering the Oldroyd-B type fluid. A generalized Darcy model, which takes into account the viscoelastic properties, is employed as momentum equation and a two-field model is used for energy equation each representing solid and fluid phases separately. Linear stability analysis suggests that, there is a competition between the processes of viscous relaxation and thermal diffusion that causes the first convective instability to be oscillatory rather than stationary. Analytical expression for the occurrence of oscillatory onset is obtained, and it is found that the necessary condition for the existence of the same is Λ < 1. Besides, the effect of viscoelastic parameters and the thermal non-equilibrium on the stability of the system is analyzed.
Similar content being viewed by others
Explore related subjects
Discover the latest articles and news from researchers in related subjects, suggested using machine learning.References
M.A. Alves P.J. Oliveira F.T. Pinho (2003) ArticleTitleBenchmark solutions for the flow of Oldroyd-B and PTT fluids in planar contractions J. Non-Newtonian Fluid Mech. 110 45–75 Occurrence Handle10.1016/S0377-0257(02)00191-X
Y. Anis (2003) ArticleTitleOn modeling the multidimensional complex fluid flow and heat or mass transport in porous media Int. J. Heat Mass Transfer 46 367–379 Occurrence Handle10.1016/S0017-9310(02)00264-8
N. Banu D.A.S. Rees (2002) ArticleTitleOnset of Darcy–Benard convection using a thermal non-equilibrium model Int. J. Heat Mass Transfer 45 2221–2228 Occurrence Handle10.1016/S0017-9310(01)00331-3
P.Y. Huang H.H. Hu D.D. Joseph (1998) ArticleTitleDirect simulation of sedimentation of elliptic particles in Oldroyd-B fluids J. Fluid Mech. 362 297–326 Occurrence Handle10.1017/S0022112098008672
D.B. Ingham I. Pop (1998) Transport Phenomena in Porous Media Pergamon Oxford
A.R.A. Khaled K. Vafai (2003) ArticleTitleThe role of porous media in modeling flow and heat transfer in biological tissues Int. J. Heat Mass Transfer 46 4989–5003 Occurrence Handle10.1016/S0017-9310(03)00301-6
B. Khuzhayorov J.L. Auriault P. Royer (2000) ArticleTitleDerivation of macroscopic filtration law for transient linear viscoelastic fluid flow in porous media Int. J. Eng. Sci. 38 487–504 Occurrence Handle10.1016/S0020-7225(99)00048-8
M.C. Kim S.B. Lee S. Kim B.J. Chung (2003) ArticleTitleThermal instability of viscoelastic fluids in porous media Int. J. Heat Mass Transfer 46 5065–5072 Occurrence Handle10.1016/S0017-9310(03)00363-6
Kuznetsov, A. V.: 1998, in D. B. Ingham and I. Pop (eds.), Thermal Non-equilibrium Forced Convection in Porous Media, Pergamon, Oxford, pp. 103–130
Z. Li R.E. Khayat (2005) ArticleTitleFinite-amplitude Rayleigh–Benard convection and pattern selection for viscoelastic fluids J. Fluid Mech. 529 221–251 Occurrence Handle10.1017/S0022112005003563
A. Lozinski R.G. Owens (2003) ArticleTitleAn energy estimate for the Oldroyd-B model: theory and applications J. Non-Newtonian Fluid Mech. 112 161–176 Occurrence Handle10.1016/S0377-0257(03)00096-X
M.S. Malashetty I.S. Shivakumara K. Sridhar (2005a) ArticleTitleThe onset of Lapwood–Brinkman convection using a thermal non-equilibrium model Int. J. Heat Mass Transfer 48 1155–1163 Occurrence Handle10.1016/j.ijheatmasstransfer.2004.09.027
M.S. Malashetty I.S. Shivakumara K. Sridhar (2005b) ArticleTitleThe onset of convection in an anisotropic porous layer using a thermal non-equilibrium model Transport Porous Media 60 199–215 Occurrence Handle10.1007/s11242-004-5130-z
D.A. Nield A. Bejan (1999) Convection in Porous Media EditionNumber2 Springer-Verlag New York
T.N. Phillips A.J. Williams (2002) ArticleTitleComparison of creeping and inertial flow of an Oldroyd-B fluid through planar and axisymmetric contractions J. Non-Newtonian Fluid Mech. 108 25–47 Occurrence Handle10.1016/S0377-0257(02)00123-4
A. Postelnicu D.A.S. Rees (2003) ArticleTitleThe onset of Darcy–Brinkman convection in a porous layer using a thermal non-equilibrium model – Part I: Stress free boundaries Int. J. Energy Res. 27 961–973 Occurrence Handle10.1002/er.928
K.R. Rajagopal M. Ruzicka A.R. Srinivas (1998) ArticleTitleOn the Oberbeck – Boussinesq approximation Math. Model Meth. Appl. Sci. 16 1157–1167
D.A.S. Rees I. Pop (2000) ArticleTitleVertical free convective boundary layer flow in a porous medium using a thermal non-equilibrium model J. Porous Media 3 31–44
D.A.S. Rees I. Pop (2002) ArticleTitleVertical free convective boundary layer flow in a porous medium using a thermal non-equilibrium model: elliptic effects J. Appl. Math. Phys. 53 1–12 Occurrence Handle10.1007/s00033-002-8137-8
N. Rudraiah P.N. Kaloni P.V. Radhadevi (1989) ArticleTitleOscillatory convection in a viscoelastic fluid through a porous layer heated from below Rheol. Acta 28 48–53 Occurrence Handle10.1007/BF01354768
Tan Wenchang Masuoka Takashi (2005) ArticleTitleStokes’ first problem for an Oldroyd-B fluid in a porous half space Phys. Fluids 17 23101–23107 Occurrence Handle10.1063/1.1850409
K. Vafai (2000) Handbook of Porous Media Marcel Dekker New York
D.Y. Yoon M.C. Kim C.K. Choi (2004) ArticleTitleThe onset of oscillatory convection in a horizontal porous layer saturated with viscoelastic liquid Transport Porous Media 55 275–284 Occurrence Handle10.1023/B:TIPM.0000013328.69773.a1
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Malashetty, M.S., Shivakumara, I.S., Kulkarni, S. et al. Convective Instability of Oldroyd-B Fluid Saturated Porous Layer Heated from Below using a Thermal Non-equilibrium Model. Transp Porous Med 64, 123–139 (2006). https://doi.org/10.1007/s11242-005-1893-0
Received:
Accepted:
Issue Date:
DOI: https://doi.org/10.1007/s11242-005-1893-0