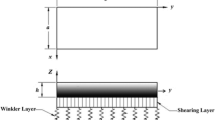
The static response of simply supported functionally graded plates (FGP) subjected to a transverse uniform load (UL) or a sinusoidally distributed load (SL) and resting on an elastic foundation is examined by using a new hyperbolic displacement model. The present theory exactly satisfies the stress boundary conditions on the top and bottom surfaces of the plate. No transverse shear correction factors are needed, because a correct representation of the transverse shear strain is given. The material properties of the plate are assumed to be graded in the thickness direction according to a simple power-law distribution in terms of volume fractions of material constituents. The foundation is modeled as a two-parameter Pasternak-type foundation, or as a Winkler-type one if the second parameter is zero. The equilibrium equations of a functionally graded plate are given based on the hyperbolic shear deformation theory of plates presented. The effects of stiffness and gradient index of the foundation on the mechanical responses of the plates are discussed. It is established that the elastic foundations significantly affect the mechanical behavior of thick functionally graded plates. The numerical results presented in the paper can serve as benchmarks for future analyses of thick functionally graded plates on elastic foundations.
Similar content being viewed by others
References
M. Koizumi, “FGM activities in Japan,” Composites, 28, 14 (1997).
Reddy J.N., “Analysis of functionally graded plates,” Int. J. Num. Meth. Eng., 47, 663–84 (2000).
Z. Q. Cheng and R. C. Batra, “Deflection relationships between the homogeneous Kirchhoff plate theory and different functionally graded plate theories,” Arch. Mech., 52, 143–58 (2000).
Z. Q. Cheng and R. C. Batra, “Exact correspondence between eigenvalues of membranes and functionally graded simply supported polygonal plates,” J. Sound Vibrat., 229, 879–95 (2000).
C. T. Loy, K. Y. Lam, and J. N. Reddy, “Vibration of functionally graded cylindrical shells,” Int. J. Mech. Sci., 41, 309–24 (1999).
A. M. Zenkour, “Generalized shear deformation theory for bending analysis of functionally graded materials,” Appl. Math. Model., 30, 67–4 (2006).
A. D. Kerr, “Elastic and viscoelastic foundation models,” ASME J. Appl. Mech., 31, No. 3, 491–98 (1964).
B. N. Zhemochkin and A. P. Sinitsyn, Practical Methods of Calculation of Foundation Beams and Slabs on Elastic Foundation [in Russian], Stroiizdat, Moscow (1947).
M. M. Filonenko-Borodich, Some Approximate Theories of Die Elastic Foundation [in Russian], Uch. Zapiski Mosk. Gos. Univ. Mekhanika., 46, 3–18 (1940).
M. Hetényi, Beams on Elastic Foundations, The Univ. Michigan Press, Ann Arbor, Michigan (1946).
M. Hetényi, “A general solution for the bending of beams on an elastic foundation of arbitrary continuity,” J. Appl. Phys., 21, 55–58 (1950).
V. Z. Vlasov, Structural Mechanics of Thin Walled Three-Dimensional Systems [in Russian], Stroiizdat (1949).
M. I. Gorbunov-Posadov, Beams and Plates on Elastic Foundation [in Russian], Gosstroiizdat (1949).
P. L. Pasternak, On a New Method of Analysis of an Elastic Foundation by Means of Two Foundation Constants [in Russian], Gosstroiizdat, Moscow (1954).
H. S. Shen, “Postbuckling analysis of composite laminated plates on two-parameter elastic foundations,” Int. J. Mech. Sci., 37, No. 12, 1307–1316 (1995).
M. H. Omurtag, A. Ozutok, and A. Y. Akoz, “Free vibration analysis of Kirchhoff plates resting on elastic foundation by mixed finite element formulation based on Gateaux differential,” Int. J. Num. Meth. Eng., 40, No. 2, 295–317 (1997).
H. Matsunaga, “Vibration and stability of thick plates on elastic foundations,” ASCE. J. Eng. Mech., 126, No. 1, 27–34 (2000).
C. P. Filipich and M. B. Rosales, “A further study about the behavior of foundation piles and beams in a Winkler–Pasternak soil,” Int. J. Mech. Sci., 44, No. 1, 21–36 (2002).
D. Zhou, Y. K. Cheung, S. H. Lo, and F. T. K. Au, “Three-dimensional vibration analysis of rectangular thick plates on Pasternak foundations,” Int. J. Num. Meth. Eng., 59, No. 10, 1313–1334 (2004).
Z. Y. Huang, C. F. Lu, and W. Q. Chen, “Benchmark solutions for functionally graded thick plates resting on Winkler–Pasternak elastic foundations,” Compos. Struct., 85, 95–104 (2008).
Z. Q. Cheng and S. Kitipornchai, “Membrane analogy of buckling and vibration of inhomogeneous plates,” J. Eng. Mech., ASCE, 125, No. 11, 1293–1297 (1999).
Z. Q. Cheng and B. C. Batra, “Exact correspondence between eigenvalues of membranes and functionally graded simply supported polygonal plate,” J. Sound. Vibrat., 229, No. 4, 879–895 (2000).
J. N. Reddy and Z. Q. Cheng, “Frequency correspondence between membranes and functionally graded spherical shallow shells of polygonal plan form,” Int. J. Mech. Sci., 44, No. 5, 967–985 (2002).
S. S. Akavci, “Analysis of thick laminated composite plates on an elastic foundation with the use of various plate theories” Mech. Compos. Mater., 44, No. 5, 445–460 (2005).
F. Delale and F. Erdogan, “The crack problem for a nonhomogeneous plane,” J. Appl. Mech., 50, 609–614 (1983).
H. Kobayashi and K. Sonoda, “Rectangular Mindlin plates on elastic foundations,” Int. J. Mech. Sci., 31, 679–692 (1989).
K. Y. Lam, C. M. Wang, and X. Q. He, “Canonical exact solutions for Levy-plates on two-parameter foundation using Green’s functions,” Eng. Struct., 22, 364–378 (2000).
Author information
Authors and Affiliations
Corresponding author
Additional information
Russian translation published in Mekhanika Kompozitnykh Materialov, Vol. 46, No. 4, pp. 621–636, July–August, 2010.
Rights and permissions
About this article
Cite this article
Benyoucef, S., Mechab, I., Tounsi, A. et al. Bending of thick functionally graded plates resting on Winkler–Pasternak elastic foundations. Mech Compos Mater 46, 425–434 (2010). https://doi.org/10.1007/s11029-010-9159-5
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11029-010-9159-5