Skip to main content
SpringerLink
Log in

Equivalence of Ensembles, Condensation and Glassy Dynamics in the Bose–Hubbard Hamiltonian

  • Published:
Journal of Statistical Physics Aims and scope Submit manuscript

Abstract

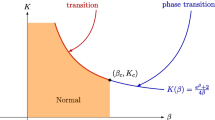
We study mathematically the equilibrium properties of the Bose–Hubbard Hamiltonian in the limit of a vanishing hopping amplitude. This system conserves the energy and the number of particles. We establish the equivalence between the microcanonical and the grand-canonical ensembles for all allowed values of the density of particles \(\rho \) and density of energy \(\varepsilon \). Moreover, given \(\rho \), we show that the system undergoes a transition as \(\varepsilon \) increases, from a usual positive temperature state to the infinite temperature state where a macroscopic excess of energy condensates on a single site. Analogous results have been obtained by Chatterjee [6] for a closely related model. We introduce here a different method to tackle this problem, hoping that it reflects more directly the basic understanding stemming from statistical mechanics. We discuss also how, and in which sense, the condensation of energy leads to a glassy dynamics.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1

Similar content being viewed by others

References

  1. Abanin, D.A., De Roeck, W., Huveneers, F.: Exponentially slow heating in periodically driven many-body systems. Phys. Rev. Lett. 115(25), 256803 (2015)

    Article  ADS  Google Scholar 

  2. Bols, A., De Roeck, W.: Asymptotic localization in the Bose-Hubbard model. J. Math. Phys. 59(2), 021901 (2018)

    Article  ADS  MathSciNet  Google Scholar 

  3. Callen, H.B.: Thermodynamics and an Introduction to Thermostatistics. Willey, New York (1985)

    MATH  Google Scholar 

  4. Chatterjee, S., Kirkpatrick, K.: Probabilistic methods for discrete nonlinear Schrödinger equations. Commun. Pure Appl. Math. 65(5), 727–757 (2012)

    Article  Google Scholar 

  5. Chatterjee, S.: Invariant measures and the soliton resolution conjecture. Commun. Pure Appl. Math. 67(11), 1737–1842 (2014)

    Article  MathSciNet  Google Scholar 

  6. Chatterjee, S.: A note about the uniform distribution on the intersection of a simplex and a sphere. J. Topol. Anal. 9(04), 717–738 (2017)

    Article  MathSciNet  Google Scholar 

  7. Chleboun, P., Grosskinsky, S.: Condensation in stochastic particle systems with stationary product measures. J. Stat. Phys. 154(1–2), 432–465 (2014)

    Article  MathSciNet  Google Scholar 

  8. Cherny, AYu., Engl, T., Flach, S.: Non-Gibbs states on a Bose-Hubbard lattice. Phys. Rev. A 99, 023603 (2019)

    Article  ADS  Google Scholar 

  9. Danieli, C., Mithun, T., Kati, Y., Campbell, D.K., Flach, S.: Dynamical glass in weakly non-integrable many-body systems, (2018) arXiv e-prints arXiv:1811.10832

  10. Deutsch, J.M.: Quantum statistical mechanics in a closed system. Phys. Rev. A 43(4), 2046 (1991)

    Article  ADS  Google Scholar 

  11. De Roeck, W., Huveneers, F.: Asymptotic quantum many-body localization from thermal disorder. Commun. Math. Phys. 332(3), 1017–1082 (2014)

    Article  ADS  MathSciNet  Google Scholar 

  12. De Roeck, W., Huveneers, F.: Asymptotic localization of energy in nondisordered oscillator chains. Commun. Pure Appl. Math. 68(9), 1532–1568 (2015)

    Article  MathSciNet  Google Scholar 

  13. Evans, M.R., Hanney, T.: Nonequilibrium statistical mechanics of the zero-range process and related models. J. Phys. A 38(19), R195–R240 (2005)

    Article  ADS  MathSciNet  Google Scholar 

  14. Grosskinsky, S.: Equivalence of ensembles for two-species zero-range invariant measures. Stoch. Process. Appl. 118(8), 1322–1350 (2008)

    Article  MathSciNet  Google Scholar 

  15. Grosskinsky, S., Schütz, G., Spohn, H.: Condensation in the zero range process: stationary and dynamical properties. J. Stat. Phys. 113(3–4), 389–410 (2003)

    Article  MathSciNet  Google Scholar 

  16. Iubini, S., Franzosi, R., Livi, R., Oppo, G.-L., Politi, A.: Discrete breathers and negative-temperature states. New J. Phys. 15(2), 023032 (2013)

    Article  ADS  Google Scholar 

  17. Lieb, E.H., Seiringer, R., Solovej, J.P., Yngvason, J.: The Mathematics of the Bose gas and Its Condensation, Oberwolfach Seminars, vol. 34. Birkhäuser Verlag, Basel (2005)

    MATH  Google Scholar 

  18. Majumdar, S.N.: Real-space Condensation in Stochastic Mass Transport Models, Exact Methods in Low-dimensional Statistical Physics and Quantum Computing: Lecture Notes of the Les Houches Summer School: Volume 89, July 2008, Oxford University Press (2010)

  19. Mithun, T., Kati, Y., Danieli, C., Flach, S.: Weakly nonergodic dynamics in the Gross-Pitaevskii lattice. Phys. Rev. Lett. 120(18), 184101 (2018)

    Article  ADS  Google Scholar 

  20. Mithun, T., Danieli, C., Kati, Y., Flach, S.: Dynamical glass and ergodization times in classical Josephson Junction Chains. Phys. Rev. Lett. 122(5), 054102 (2019)

    Article  ADS  Google Scholar 

  21. Nam, K.: Large deviations and localization of the microcanonical ensembles given by multiple constraints, (2018) arXiv e-prints, arXiv:1809.04138

  22. Rasmussen, K.Ø., Cretegny, T., Kevrekidis, P.G., Grønbech-Jensen, N.: Statistical mechanics of a discrete nonlinear system. Phys. Rev. Lett. 84(17), 3740–3743 (2000)

    Article  ADS  Google Scholar 

  23. Rumpf, B.: Simple statistical explanation for the localization of energy in nonlinear lattices with two conserved quantities. Phys. Rev. E 69, 016618 (2004)

    Article  ADS  Google Scholar 

  24. Srednicki, M.: Chaos and quantum thermalization. Phys. Rev. E 50(2), 888 (1994)

    Article  ADS  Google Scholar 

  25. Touchette, H.: Ensemble equivalence for general many-body systems. Europhys. Lett. 96(5), 50010 (2011)

    Article  ADS  Google Scholar 

  26. Touchette, H.: Equivalence and nonequivalence of ensembles: thermodynamic, macrostate, and measure levels. J. Stat. Phys. 159(5), 987–1016 (2015)

    Article  ADS  MathSciNet  Google Scholar 

Download references

Acknowledgements

We thank N. Starreveld for discussions at an early stage of this project, as well as C. Bernardin and S. Olla for pointing out relevant references to us. This work was partially supported by the grants ANR-15-CE40-0020-01 LSD and ANR-14-CE25-0011 EDNHS of the French National Research Agency (ANR).

Author information

Authors and Affiliations

  1. Ceremade, UMR-CNRS 7534, Université Paris Dauphine, PSL Research University, 75775, Paris Cedex 16, France

    François Huveneers

  2. Vienna University of Technology, 1040, Vienna, Austria

    Elias Theil

Authors
  1. François Huveneers
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Elias Theil
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to François Huveneers.

Additional information

Communicated by Alessandro Giuliani.

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Huveneers, F., Theil, E. Equivalence of Ensembles, Condensation and Glassy Dynamics in the Bose–Hubbard Hamiltonian. J Stat Phys 177, 917–935 (2019). https://doi.org/10.1007/s10955-019-02396-z

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10955-019-02396-z

Keywords

Search

Navigation