Skip to main content
SpringerLink
Log in

A spectral approach to simulating intrinsic random fields with power and spline generalized covariances

  • Original paper
  • Published:
Computational Geosciences Aims and scope Submit manuscript

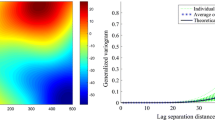
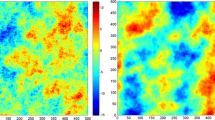
Abstract

This article presents a variant of the spectral turning bands method that allows fast and accurate simulation of intrinsic random fields with power, spline, or logarithmic generalized covariances. The method is applicable in any workspace dimension and is not restricted in the number and configuration of the locations where the random field is simulated; in particular, it does not require these locations to be regularly spaced. On the basis of the central limit and Berry–Esséen theorems, an upper bound is derived for the Kolmogorov distance between the distributions of generalized increments of the simulated random fields and the normal distribution.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Similar content being viewed by others

References

  1. Ahrens, J.H., Dieter, U.: Generating gamma variates by a modified rejection technique. Commun. ACM 25, (1), 47–54 (1982)

    Article  MATH  MathSciNet  Google Scholar 

  2. Besag, J., Mondal, D.: First-order intrinsic autoregressions and the de Wijs process. Biometrika 92, (4), 909–920 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  3. Box, G.E.P., Jenkins, G.M.: Time Series Analysis: Forecasting and Control. Holden-Day, San Francisco (1970)

    MATH  Google Scholar 

  4. Buttafuoco, G., Castrignano, A.: Study of the spatio-temporal variation of soil moisture under forest using intrinsic random functions of order k. Geoderma 128, (3–4), 208–220 (2005)

    Article  Google Scholar 

  5. Chauvet, P.: Processing data with a spatial support: Geostatistics and its methods. Cahiers de Géostatistique, Fascicule 4, 41 pp. Ecole des Mines de Paris, Fontainebleau (1993)

    Google Scholar 

  6. Chilès, J.P.: Quelques méthodes de simulation de fonctions aléatoires intrinsèques. In: de Fouquet, C. (ed.) Cahiers de Géostatistique, Fascicule 5, pp. 97–112. Ecole des Mines de Paris, Fontainebleau (1995)

    Google Scholar 

  7. Chilès, J.P., Allard, D.: Stochastic simulation of soil variations. In: Grunwald, S. (ed.) Environmental Soil-Landscape Modeling: Geographic Information Technologies and Pedometrics, pp. 289–321. CRC Press, Boca Raton (2005)

    Google Scholar 

  8. Chilès, J.P., Delfiner, P.: Geostatistics: Modeling Spatial Uncertainty. Wiley, New York (1999)

    MATH  Google Scholar 

  9. Chilès, J.P., Gable, R.: Three-dimensional modelling of a geothermal field. In: Verly, G., David, M., Journel, A.G., Maréchal, A. (eds.) Geostatistics for Natural Resources Characterization, pp. 587–598. Reidel, Dordrecht (1984)

    Google Scholar 

  10. Christakos, G., Thesing, G.A.: The intrinsic random field model in the study of sulfate deposition processes. Atmos. Environ., A–Gen. Topics 27, (10), 1521–1540 (1993)

    Article  Google Scholar 

  11. David, M.: Handbook of Applied Advanced Geostatistical Ore Reserve Estimation. Elsevier, Amsterdam (1988)

    Google Scholar 

  12. David, M., Crozel, D., Robb, J.M.: Automated mapping of the ocean floor using the theory of intrinsic random functions of order k. Mar. Geophys. Res. 8, (1), 49–74 (1986)

    Article  Google Scholar 

  13. Davis, M.W.: Production of conditional simulations via the LU triangular decomposition of the covariance matrix. Math. Geol. 19, (2), 91–98 (1987)

    Google Scholar 

  14. de Fouquet, C.: Joint simulation of a random function and its derivatives. In: Kleingeld, W.J., Krige, D.G. (eds.) Geostats 2000 Cape Town, vol. 1. Geostatistical Association of Southern Africa, Cape Town (2001)

    Google Scholar 

  15. Delfiner, P., Chilès, J.P.: Conditional simulations: A new Monte-Carlo approach to probabilistic evaluation of hydrocarbon in place. SPE paper 6985, pp. 32 (1977)

  16. Delhomme, J.P.: Spatial variability and uncertainty in groundwater flow parameters: a geostatistical approach. Water Resour. Res. 15, (2), 269–280 (1979)

    Article  Google Scholar 

  17. de Marsily, G.: Quantitative Hydrogeology: Groundwater Hydrology for Engineers. Academic, San Diego (1986)

    Google Scholar 

  18. Devroye, L.: Non-Uniform Random Variate Generation. Springer, New York (1986)

    MATH  Google Scholar 

  19. Dietrich, C.R., Newsam, G.N.: A fast and exact method for multidimensional Gaussian stochastic simulations. Water Resour. Res. 29, (8), 2861–2869 (1993)

    Article  Google Scholar 

  20. Dimitrakopoulos, R.: Conditional simulation of intrinsic random functions of order k. Math. Geol. 22, (3), 361–380 (1990)

    Article  Google Scholar 

  21. Dong, A.: Estimation géostatistique des phénomènes régis par des équations aux dérivées partielles. Doctoral thesis, Ecole des Mines de Paris, Paris (1990)

  22. Emery, X., Lantuéjoul, C.: TBSIM: a computer program for conditional simulation of three-dimensional Gaussian random fields via the turning bands method. Comput. Geosci. 32, (10), 1615–1628 (2006)

    Article  Google Scholar 

  23. Feller, W.: An Introduction to Probability Theory and Its Applications, vol. 2, 2nd edn. Wiley, New York (1971)

    Google Scholar 

  24. Flandrin, P.: Wavelet analysis and synthesis of fractional Brownian motion. IEEE Trans. Inf. Theory 38, (2), 910–917 (1992)

    Article  MathSciNet  Google Scholar 

  25. Fournier, A., Fussell, D., Carpenter, R.L.: Computer rendering of stochastic models. Commun. ACM 25, (6), 371–384 (1982)

    Article  Google Scholar 

  26. Gneiting, T., Sasvári, Z., Schlather, M.: Analogies and correspondences between variograms and covariance functions. Adv. Appl. Probab. 33, (3), 617–630 (2001)

    Article  MATH  Google Scholar 

  27. Gradshtein, I.S., Ryzhik, I.M.: Table of Integrals, Series and Products, 4th edn. Academic, New York (1965)

    Google Scholar 

  28. Journel, A.G.: Geostatistics for conditional simulation of orebodies. Econ. Geol. 69, (5), 673–687 (1974)

    Article  Google Scholar 

  29. Khinchin, A.Y.: Korrelationstheorie der stationären stochastischen Prozesse. Math. Ann. 109, (1), 604–615 (1934)

    Article  MATH  MathSciNet  Google Scholar 

  30. Kitanidis, P.K.: Statistical estimation of polynomial generalized covariance functions and hydrologic applications. Water Resour. Res. 19, (4), 909–921 (1983)

    Article  Google Scholar 

  31. Kitanidis, P.K.: Generalized covariance functions associated with the Laplace equation and their use in interpolation and inverse problems. Water Resour. Res. 35, (5), 1361–1367 (1999)

    Article  Google Scholar 

  32. Lantuéjoul, C.: Non conditional simulation of stationary isotropic multigaussian random functions. In: Armstrong, M., Dowd, P.A. (eds.) Geostatistical Simulations, pp. 147–177. Kluwer, Dordrecht (1994)

    Google Scholar 

  33. Lantuéjoul, C.: Geostatistical Simulation: Models and Algorithms. Springer, Berlin (2002)

    MATH  Google Scholar 

  34. Le Cointe, P.: Kriging with partial differential equations in hydrogeology. Master’s thesis, Université Pierre et Marie Curie, Ecole des Mines de Paris & Ecole Nationale du Génie Rural des Eaux et Forêts, Paris (2006)

  35. Mandelbrot, B.B., Van Ness, W.J.: Fractional Brownian motions, fractional noises and applications. SIAM Rev. 10, (4), 422–437 (1968)

    Article  MATH  MathSciNet  Google Scholar 

  36. Mantoglou, A.: Digital simulation of multivariate two- and three-dimensional stochastic processes with a spectral turning bands method. Math. Geol. 19, (2), 129–149 (1987)

    Google Scholar 

  37. Mantoglou, A., Wilson, J.L.: The turning bands method for simulation of random fields using line generation by a spectral method. Water Resour. Res. 18, (5), 1379–1394 (1982)

    Article  Google Scholar 

  38. Matheron, G.: The Theory of Regionalized Variables and its Applications. Ecole des Mines de Paris, Paris (1971)

    Google Scholar 

  39. Matheron, G.: The intrinsic random functions and their applications. Adv. Appl. Probab. 5, 439–468 (1973)

    Article  MATH  MathSciNet  Google Scholar 

  40. Matheron, G.: The internal consistency of models in geostatistics. In: Armstrong, M. (ed.) Geostatistics, vol. 1, pp. 21–38. Kluwer, Dordrecht (1989)

    Google Scholar 

  41. Pardo-Igúzquiza, E., Dowd, P.A.: IRFK2D: a computer program for simulating intrinsic random functions of order k. Comput. Geosci. 29, (6), 753–759 (2003)

    Article  Google Scholar 

  42. Saupe, D.: Algorithms for random fractals. In: Peitgen, H.O., Saupe, D. (eds.) The Science of Fractal Images, pp. 71–113. Springer, New York (1988)

    Google Scholar 

  43. Schlather, M.: An Introduction to Positive Definite Functions and to Unconditional Simulation of Random Fields. Technical Report ST-99–10, Lancaster University (1999)

  44. Sellan, F.: Wavelet transform based fractional Brownian-motion synthesis. Compte Rendu de l’Académie des Sciences, Série I Mathématique 321, (3), 351–358 (1995)

    MATH  MathSciNet  Google Scholar 

  45. Shiganov, I.S.: Refinement of the upper bound of the constant in the central limit theorem. J. Math. Sci. 35, (3), 2545–2550 (1986)

    Article  MATH  Google Scholar 

  46. Shinozuka, M.: Simulation of multivariate and multidimensional random processes. J. Acoust. Soc. Am. 49, (1), 357–367 (1971)

    Article  Google Scholar 

  47. Shinozuka, M., Jan, C.M.: Digital simulation of random processes and its applications. J. Sound Vib. 25, (1), 111–128 (1972)

    Article  Google Scholar 

  48. Stein, M.L.: Local stationarity and simulation of self-affine intrinsic random functions. IEEE Trans. Inf. Theory 47, (4), 1385–1390 (2001)

    Article  MATH  Google Scholar 

  49. Stein, M.L.: Fast and exact simulation of fractional Brownian surfaces. J. Comput. Graph. Stat. 11, (3), 587–599 (2002)

    Article  Google Scholar 

  50. Suárez Arriaga, M.C., Samaniego, F.: Intrinsic random functions of high order and their application to the modeling of non-stationary geothermal parameters. In: Proceedings of the Twenty-third Workshop on Geothermal Reservoir Engineering, pp. 169–175. Technical report SGP-TR-158, Stanford University, Stanford (1998)

  51. Tompson, A.F.B., Ababou, R., Gelhar, L.W.: Implementation of the three-dimensional turning bands random field generator. Water Resour. Res. 25, (8), 2227–2243 (1989)

    Article  Google Scholar 

  52. Voss, R.F.: Random fractal forgeries. In: Earnshaw, R.A. (ed.) Fundamental Algorithms for Computer Graphics, pp. 805–835. Springer, Berlin (1985))

    Google Scholar 

  53. Yin, Z.M.: New methods for simulation of fractional Brownian motion. J. Comput. Phys. 127, (1), 66–72 (1996)

    Article  MATH  MathSciNet  Google Scholar 

  54. Zeldin, B.A., Spanos, P.D.: Random field representation and synthesis using wavelet bases. J. Appl. Mech.—Trans ASME 63, (4), 946–952 (1996)

    MATH  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mining Engineering, University of Chile, Avenida Tupper 2069, Santiago, 837 0451, Chile

    Xavier Emery

  2. Equipe Géostatistique, Ecole des Mines–ParisTech, 35 Rue Saint-Honoré, 77300, Fontainebleau, France

    Christian Lantuéjoul

Authors
  1. Xavier Emery
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Christian Lantuéjoul
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Xavier Emery.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Emery, X., Lantuéjoul, C. A spectral approach to simulating intrinsic random fields with power and spline generalized covariances. Comput Geosci 12, 121–132 (2008). https://doi.org/10.1007/s10596-007-9064-8

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10596-007-9064-8

Keywords

Search

Navigation