Skip to main content
Springer Nature Link
Log in

Scaling laws of compressible turbulence

  • Published:
Applied Mathematics and Mechanics Aims and scope Submit manuscript

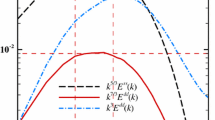
Abstract

Spatial scaling laws of velocity kinetic energy spectra for the compressible turbulence flow and the density-weighted counterparts are formulated in terms of the wavenumber, dissipation rate, and Mach number by using a dimensional analysis. We apply the Barenblatt’s incomplete similarity theory to both kinetic and density-weighted energy spectra. It shows that, within the initial subrange, both energy spectra approach the –5/3 and –2 power laws of the wavenumber when the Mach number tends to unity and infinity, respectively.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

Abbreviations

u :

flow velocity

v :

density-weighted velocity

u′i :

fluctuation of flow velocity

L :

length dimension

t :

time dimension

m :

mass dimension

k :

wavenumber

ν :

kinematic viscosity

ε :

dissipation rate

E :

kinetic energy spectrum

E ρ :

density-weighted energy spectrum

Ma :

Mach number

Re :

Reynolds number

c :

speed of sound

γ :

ratio of specific heat

η :

Kolmogorov length

C K :

Kolmogorov constant

C :

coefficient constant

C ρ :

coefficient constant

A :

adjustable parameter

σ :

adjustable parameter

β :

adjustable parameter

β ρ :

adjustable parameter

E c :

compressible part of E ρ

E s :

solenoidal part of E ρ

c c :

compressible part constant

c s :

solenoidal part constant

λ :

constant

d(Ma):

exponent

h(Ma):

exponent

α :

exponent

l :

box size

l ν :

box size of sequence ν

ρ :

mass density

ρ 0 :

local reservoir value of density

References

  1. Kritsuk, A. G., Norman, M. L., Padoan, P., and Wagner, R. The statistics of supersonic isothermal turbulence. The Astrophysical Journal, 665, 416–431 (2007)

    Article  Google Scholar 

  2. Frisch, U. Turbulence: The Legacy of A.N. Kolmogorov, Cambridge University Press, Cambridge (2008)

    Google Scholar 

  3. Lee, C. B. and Wu, J. Z. Transition in wall-bounded flows. Applied Mechanics Reviews, 61, 030802 (2008)

    Article  MATH  Google Scholar 

  4. She, Z. S., Chen, X., Wu, Y., and Hussain, F. New perspective in statistical modeling of wallbounded turbulence. Acta Mechanica Sinica, 26, 847–861 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  5. Zhang, Y. S., Bi, W. T., Hussain, F., Li, X. L., and She, Z. S. Mach-number-invariant meanvelocity profile of compressible turbulent boundary layers. Physical Review Letters, 109, 054502 (2012)

    Article  Google Scholar 

  6. Zhou, H. and Zhang, H. X. What is the essence of the so-called century lasting difficult problem in classic physics, the “problem of turbulence”? Scienta Sinica: Physica, Mechanica and Astronomica, 42, 1–5 (2012)

    Google Scholar 

  7. Wang, J., Yang, Y., Shi, Y., Xiao, Z., He, X. T., and Chen, S. Cascade of kinetic energy in three-dimensional compressible turbulence. Physical Review Letters, 110, 214505 (2013)

    Article  Google Scholar 

  8. Chen, S. Y., Xia, Z. H., Wang, J. C., and Yang, Y. T. Recent progress in compressible turbulence. Acta Mechanica Sinica, 31, 275–291 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  9. Kolmogorov, A. N. The local structure of turbulence in incompressible viscous fluid for very large Reynolds number. Proceedings of the Royal Society of London, 434, 9–13 (1991)

    Article  MathSciNet  MATH  Google Scholar 

  10. Kolmogorov, A. N. On degeneration (decay) of isotropic turbulence in an incompressible visous liquid. Doklady Akademii Nauk SSSR, 31, 538–540 (1941)

    Google Scholar 

  11. Kolmogorov, A. N. Dissipation of energy in locally isotropic turbulence. Proceedings of the Royal Society of London, 434, 15–17 (1991)

    Article  MathSciNet  MATH  Google Scholar 

  12. Aluie, H. Compressible turbulence: the cascade and its locality. Physical Review Letters, 106, 174502 (2011)

    Article  Google Scholar 

  13. Aluie, H., Li, S., and Li, H. Conservative cascade of kinetic energy in compressible turbulence. Astrophysical Journal Letters, 751, L29 (2012)

    Article  Google Scholar 

  14. Aluie, H. Scale decomposition in compressible turbulence. Physica D: Nonlinear Phenomena, 247, 54–65 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  15. Armstrong, J. W., Spangler, S. R., and Rickett, B. J. Electron density power spectrum in the local interstellar medium. The Astrophysical Journal, 443, 209–221 (1995)

    Article  Google Scholar 

  16. Cardy, J., Falkovich, G., and Gawedzki, K. Nonequilibrium Statistical Mechanics and Turbulence, Cambridge University Press, Cambridge (2008)

    Book  Google Scholar 

  17. Chu, B. T. and Kovasznay, L. S. G. Non-linear interactions in a viscous heatconducting compressible gas. Journal of Fluid Mechanics, 3, 494–514 (1958)

    Article  MathSciNet  Google Scholar 

  18. Federrath, C., Roman-Duval, J., Klessen, R. S., Schmidt, W., and Mac Low, M. M. Comparing the statistics of interstellar turbulence in simulations and observations: solenoidal versus compressive turbulence forcing. Astronomy and Astrophysics, 512, A81 (2010)

    Article  Google Scholar 

  19. Wang, J. Cascade of Kinetic Energy and Thermodynamic Process in Compressible Turbulence (in Chinese), Post-Doctoral Research Report, Peking University (2014)

    Google Scholar 

  20. Schmidt, W., Federrath, C., and Klessen, R. Is the scaling of supersonic turbulence universal? Physical Review Letters, 101, 194505 (2008)

    Article  Google Scholar 

  21. Galtier, S. and Banerjee, S. Exact relation for correlation functions in compressible isothermal turbulence. Physical Review Letters, 107, 134501 (2011)

    Article  Google Scholar 

  22. Sun, B. H. The spatial scaling laws of compressible turbulence. https://arxiv.org/ abs/1502.02815v5 (2016)

    Google Scholar 

  23. Kovasznay, L. S. G. Turbulence in supersonic flow. Journal of the Aeronautical Sciences, 20, 657–674 (1953)

    Article  MATH  Google Scholar 

  24. Lighthill, M. J. The effect of compressibility on turbulence. Proceedings from the 2nd International Astronomical Union Symposium on Gas Dynamics of Cosmic Clouds, North Holland Publishing Company, Amsterdam (1955)

    Google Scholar 

  25. Moiseev, S. S., Toor, A. V., and Yanovsky, V. V. The decay of turbulence in the Burgers model. Physica D: Nonlinear Phenomena, 2, 187–193 (1981)

    Article  Google Scholar 

  26. Kadomtsev, B. B. and Petviashvili, V. I. Acoustic turbulence. Soviet Physics Doklady, 18, 115–118 (1973)

    Google Scholar 

  27. Shivamoggi, B. K. Multifractal aspects of the scaling laws in fully developed compressible turbulence. Annals of Physics, 243, 169–176 (1995)

    Article  MathSciNet  MATH  Google Scholar 

  28. Bridgman, P. W. Dimensional Analysis, Yale University Press, New Haven (1922)

    MATH  Google Scholar 

  29. Sedov, L. I. Similarity and Dimensional Analysis in Mechanics, Academic Press, New York (1959)

    MATH  Google Scholar 

  30. Barenblatt, G. I. Similarity, Self-similarity and Intermediate Asymptotics, Cambridge University Press, Cambridge (1996)

    Book  MATH  Google Scholar 

  31. Cantwell, B. J. Introduction to Symmetry Analysis, Cambridge University Press, Cambridge (2002)

    MATH  Google Scholar 

  32. Sun, B. H. Dimensional Analysis and Lie Group (in Chinese), China High Education Press, Bejing (2016)

    Google Scholar 

  33. Liepmann, H.W. and Roshko, A. Elements of Gasdynamics, Dover Publications, New York (1993)

    MATH  Google Scholar 

  34. Von Weizsäcker, C. F. The evolution of galaxies and stars. The Astrophysical Journal, 114, 165–186 (1951)

    Article  MathSciNet  Google Scholar 

  35. Fleck, R. C., Jr. Scaling relations for the turbulenct, non-self-gravitating, neutral component of the interstellar medium. The Astrophysical Journal, 458, 739–741 (1996)

    Article  Google Scholar 

  36. Meneveau, C. and Sreenivasan, K. R. Interface dimension in intermittent turbulence. Physical Review A, 41, 2246–2248 (1990)

    Article  Google Scholar 

  37. Sun, B. H. The temporal scaling laws of compressible turbulence. Modern Physics Letters B, 30, 1650297 (2016)

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

This paper commemorates my beloved father, Zhongchuan SUN. Supports from the South Africa National Research Foundation (NRF), Cape Peninsula University of Technology (CPUT), and the State Key Laboratory for Turbulence and Complex Systems at Peking University are gratefully acknowledged. The author would like to express his most sincere thanks to reviewers for their high level academic comments and corrections, and their professionalism inspires me deeply. The author wishes to take this opportunity to appreciate the general discussion on turbulence with Professors Shiyi CHEN, Zhensu SHE, Cunbiao LEE, Yipeng SHI, and Jiancun WANG.

Author information

Authors and Affiliations

  1. Department of Mechanical Engineering, Cape Peninsula University of Technology, Cape Town, 7535, South Africa

    Bohua Sun

Authors
  1. Bohua Sun
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Bohua Sun.

Additional information

Project supported by the National Research Foundation of South Africa (No. 93918)

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Sun, B. Scaling laws of compressible turbulence. Appl. Math. Mech.-Engl. Ed. 38, 765–778 (2017). https://doi.org/10.1007/s10483-017-2204-8

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10483-017-2204-8

Key words

Chinese Library Classification

2010 Mathematics Subject Classification

Profiles

  1. Bohua Sun View author profile