Time Versus Energy in the Averaged Optimal Coplanar Kepler Transfer Towards Circular Orbits

Abstract

This article makes a study of the averaged optimal coplanar transfer towards circular orbits. Our objective is to compare this problem when the cost minimized is transfer time to the same problem when the cost minimized is energy consumption. While the minimum energy case leads to the analysis of a 2D-Riemannian metric using the standard tools of Riemannian geometry, the minimum time case is associated with a Finsler metric which is not smooth. Nevertheless a qualitative analysis of the geodesic flow is given in this article to describe the optimal transfers of the time minimal case.

Appendices

Appendix A: Proof of Proposition 5

Let us prove that the seven points in Proposition 5 are satisfied by a,b,c given by (45) (full control case) and also by a,b,c given by (46) (tangential thrust case).

1. Symmetries. Equations (30) and (31) imply

$$\widetilde{I}(\pi+ \psi, \varphi, E) = - \widetilde{I}( \psi, \varphi, E),\qquad \widetilde{I}(-\psi, -\varphi, \pi- E) = \widetilde{I}( \psi, \varphi, E) $$

while (33) implies

$$\widetilde{J}(\psi+ \pi, \varphi, E) = -\widetilde{J}(\psi, \varphi, E),\qquad \widetilde{J}(-\psi, -\varphi, \pi- E) = \widetilde{J}(\psi, \varphi, E). $$

Substituting in (29) and (32) yields, using the change of variable E→π−E in the integrals L(−ψ,−φ) and M(−ψ,−φ),

$$L(\pi+ \psi, \varphi) = L(-\psi, -\varphi) = L(\psi, \varphi),\qquad M(\pi+ \psi, \varphi) = M(-\psi, - \varphi) = M(\psi, \varphi). $$

This yields identities (53) with a,b,c given either by (45) or by (46).

2. Uniqueness of solutions. This follows from the classical Cauchy-Lipschitz theorem away from $\mathcal{S}$ (see Proposition 3). On $\mathcal{S}$,

• in the full control case (a,b given by (45)), as seen in [3], the regularity properties (35) of the right hand side of (54) guarantee the existence and uniqueness of solutions to the Cauchy problem (Kamke uniqueness Theorem [13, Chap. III, Theorem 6.1]),

• in the tangential thrust case (a,b given by (46)), the same argument does not apply but one may check that the derivative of $\tan\psi-\frac{1}{2}(\pm1+\sin\varphi)/\cos\varphi$ along $\dot{\psi}=a(\psi,\varphi)$, $\dot{\varphi}=b(\psi,\varphi)$ is nonzero along the curve $\tan\psi=\frac{1}{2}(\pm1+\sin\varphi)/\cos \varphi$, hence the vector field is transverse to $\mathcal{S}$ and this implies uniqueness of solutions starting from a point in $\mathcal{S}$ (see e.g. [10]).

Continuity of Φ in (55), is, according to [13, Chap. V, Theorem 2.1], guaranteed by uniqueness of solutions and continuity of a,b.

3. Sign and zeroes of b.

3.1. Full control case (a,b given by (45)). On the one hand, one has

$$\begin{aligned} b(0,\varphi) =& \frac{2\,\cos\varphi\sin\varphi}{\pi}\int_0^{\pi} \frac{\cos^2 E \,\mathrm{d}E }{ \sqrt{1-\sin^2\varphi\cos ^2 E }}, \qquad b(-\pi,\varphi) =-b(0,\varphi) . \end{aligned}$$

(71)

On the other hand, the derivative of b(ψ,φ) with respect to ψ is given by

$$\begin{aligned} \frac{\partial b}{\partial\psi}(\psi,\varphi) =& \frac{\cos\psi}{\pi}\int _0^{\pi} \frac{(1-\sin^2\varphi \cos^2 E)^{4}\,\sin^2 E }{\widetilde{I}(\psi,\varphi ,E)^{3/2}}\,\mathrm{d}E. \end{aligned}$$

(72)

The integrals in both equations are positive. Hence for any fixed φ≥0, b(ψ,φ) is increasing with respect to ψ on $(-\frac{\pi}{2},\frac{\pi}{2})$ and decreasing on $(\frac{\pi}{2},\frac{3\pi}{2})$; according to (71), it is positive in $(0,\frac{\pi}{2}]$ and negative in $(\pi,\frac{3\pi}{2}]$ (identified with $(-\pi,-\frac {\pi}{2}]$), hence it must vanish for a unique value of ψ between $-\frac{\pi}{2}$ and 0, that we call Z _b (ψ), thus defining $Z_{b}:[0,\frac{\pi}{2})\to(-\frac{\pi}{2},0]$. It also vanishes for a unique value of ψ between $\frac{\pi}{2}$ and π that must be equal to π+Z _b (ψ) according to (53). According to (71), Z _b (0)=0 and Z _b (0)<0 if φ>0.

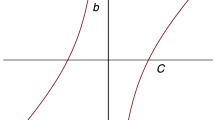
Proposition 3 says that L, and hence b, are smooth away from $\mathcal{S}$. The part of $\mathcal{S}$ that is contained in the square $(-\frac{\pi}{2},0]\times[0,\frac{\pi}{2})$ is the curve $\{\tan\psi=\frac{-1+\sin\varphi}{2\cos\varphi},\;0\leq \varphi<\frac{\pi}{2}\}$. We claim that $\gamma(\varphi)=b (\arctan\frac{-1+\sin\varphi }{2\cos\varphi},\varphi )$ does not vanish between 0 and $\frac{\pi}{2}$; this is numerically checked by plotting, on Fig. 4, the graph of the function e↦γ(arcsine) on [0,1], i.e.

$$ e\mapsto b \biggl(\arctan\frac{-1+e}{2\sqrt{1-e^2}},\ \arcsin e \biggr). $$

(73)

On the one hand, this proves that b is smooth at points where it vanishes; on the other hand the derivative of b with respect to ψ is (see (72)) strictly positive at (Z _b (φ),φ), φ>0. This implies smoothness of Z _b according to the inverse function theorem; and this extends to negative φ with Z _b (0)=0, hence point 3 of the proposition is satisfied with $\bar{\sigma}=0$; it is also easy to check that b(ψ,0) only if ψ=0 or ψ=π.

Fig. 4

Numerical plot of the function (73) on the interval [0,1). Obtained with Maple 15

3.2. Tangential thrust case (a,b given by (46)). In the region $\mathcal{R}_{1}$, one has

$$\begin{aligned} b(\psi, \varphi) = (\operatorname {sign}\cos\psi )\;\frac{2\cos\varphi\sin\varphi}{\pi}\int _{0}^{\pi}\frac{\cos^2E}{\sqrt{1-\sin^2\varphi \cos^2E}}\mathrm{d}E . \end{aligned}$$

(74)

The derivative of b(ψ,φ) with respect to ψ is zero in $\mathcal{R}_{1}$ because the above does not depend on ψ, and in $\mathcal{R}_{2}$ it is given by

$$\begin{aligned} b_{\psi}(\psi,\varphi) =& \frac{ \operatorname {sign}( P(\psi,\varphi) )\; 8\cos^2\varphi\;R(\psi,\varphi)\, (1-R(\psi,\varphi)\sin \varphi ) }{ \sqrt{1 - R(\psi,\varphi)^2}\sqrt{1 - R(\psi,\varphi)^2\sin^2 \varphi}\, (2\sin\varphi\cos\psi- \cos\varphi\sin\psi)^2}. \end{aligned}$$

(75)

Since |R|<1 on $\mathcal{R}_{2}$, all factors are positive except R(ψ,φ), hence b _ψ vanishes in $\mathcal{R}_{2}$ at points where R vanishes, and this is exactly, according to (39), on the lines $\{\psi= \pm\frac{\pi}{2}\}$, so that b _ψ (ψ,φ) has the sign of P(ψ,φ)R(ψ,φ), i.e. of cosψ. Hence, for fixed φ≥0, b(ψ,φ) is

• minimum and negative for ψ=−π/2,

• increasing for ψ in $(-\frac{\pi}{2},\arctan (\frac {-1+\sin\varphi}{2\cos\varphi} ))$,

• constant, positive if φ>0 and zero if φ=0, for ψ in $[\arctan (\frac{-1+\sin\varphi}{2\cos\varphi } ),\arctan (\frac{1+\sin\varphi}{2\cos\varphi} )]$,

• increasing, hence positive, for ψ in $(\arctan (\frac {1+\sin\varphi}{2\cos\varphi} ),\frac{\pi}{2})$,

• maximum and positive for $\psi=\frac{\pi}{2}$.

Hence, for any φ > 0, there is a unique ψ, $-\frac{\pi}{2}\,{<}\,\psi\,{<}\,\arctan (\frac{-1+\sin\varphi}{2\cos \varphi} )$ such that b(ψ,φ) = 0; we call it Z _b (φ), thus defining $Z_{b}:(0,\frac{\pi}{2})\to(-\frac{\pi}{2},0)$, satisfying $-\frac{\pi}{2}<Z_{b}(\varphi)< \arctan (\frac{-1+\sin\varphi }{2\cos\varphi} )$ satisfying (58)–(59) (situation on $[\frac{\pi}{2},\frac{3\pi}{2}]$ by symmetry, see (53)).

Since (74) is valid also on $\mathcal{S}$ by continuity, b does not vanish on $\mathcal{S}$ except at φ=0, hence b is smooth when it vanishes, away from φ=0; since we also proved that b _ψ is nonzero at these points, the inverse function theorem implies that Z _b is smooth on the open interval $(0,\frac{\pi}{2})$; also the monotonicity argument shows that $\lim_{\varphi\to0}Z_{b}(\varphi)=-\arctan\frac{1}{2}$, hence Z _b is defined $[0,\frac{\pi}{2})\to(-\frac{\pi}{2},0)$ with $Z_{b}(0)=-\arctan \frac{1}{2}$. Since our considerations above for φ≥0 imply that b(0,φ) is zero if and only if $\psi\in[-\arctan\frac{1}{2},\arctan\frac{1}{2}]\cup[\pi-\arctan\frac{1}{2},\pi+\arctan\frac{1}{2}]$, we have proved point 3 of the proposition with $\bar{\sigma}=\arctan\frac{1}{2}$.

4. Sign and zeroes of a. In (60), the part saying that a(ψ,0)>0 for $-\bar{\sigma}\leq\psi<0$ needs no proof in the full control case because $\bar{\sigma}=0$ and is easy in the tangential thrust case because, from (40) and (46), a(ψ,0)=(1+cosψ)sinψ in $\mathcal{R}_{2}$.

We give numerical evidence that a(Z _b (φ),φ) is positive if $0<\varphi<\frac{\pi}{2}$. Note that the map Z _b can only be determined numerically as the zero ψ=Z _b (φ) of b(ψ,φ)=0 between $-\frac{\pi}{2}$ and 0 for fixed φ, but the determination is very reliable for b is monotonous with respect to ψ=Z _b (φ) in the considered region; see point 3.

Figure 5 displays a numerical plot of the graph of the map φ↦a(Z _b (φ),φ) in the full control case; we also show φ↦Z _b (φ). Figure 6 displays a numerical plot of the graph of φ↦a(Z _b (φ),φ) in the tangential thrust case; we also show φ↦Z _b (φ) and $\varphi\mapsto\arctan (\frac{-1+\sin\varphi}{2\cos \varphi} )$ to show that it is very close to Z _b ($\psi=\arctan (\frac{-1+\sin\varphi}{2\cos\varphi} )$ is the curve where R(ψ,φ)=−1, the border between $\mathcal{R}_{1}$ and $\mathcal{R}_{2}$).

Fig. 5

Plots (obtained with Matlab) of the maps φ↦Z _b (φ) (dashed line) and φ↦a(Z _b (φ),φ) (solid line) in the full control case

Fig. 6

The plots (obtained with Matlab) of the functions φ↦Z _b (φ) (dashed) and ψ=a(Z _b (φ),φ) for φ∈[0,π/2]. Note that a(Z _b (φ),φ) is everywhere positive on this interval. The other curve shown is the curve R(ψ,φ)=−1

5. Hyperbolic saddle. Smoothness around the origin follows from Proposition 3. It is clear in both cases that a(0,0)=0, b(0,0)=0. The computation of the Jacobians is easy (in the tangential thrust case it takes place in the region $\mathcal{R}_{1}$ with cosψ≥0, see (74)).

In the full control case,

$$ \frac{\partial a}{\partial\psi}(0,0)=-2 ,\qquad \frac{\partial a}{\partial\varphi}(0,0)= \frac{1}{2} ,\qquad \frac{\partial b}{\partial\psi}(0,0)=\frac{1}{2} ,\qquad \frac{\partial b}{\partial\varphi}(0,0)=1 . $$

(76)

The eigenvalues of the Jacobian $\bigl( {\scriptsize\begin{matrix} -2&1/2\cr 1/2 & 1\end{matrix}} \bigr)$ are $(\sqrt{10}-1)/2$ (unstable) and $-(\sqrt{10}+1)/2$ (stable) associated to the eigenvectors $(\sqrt{10}-3,1)$ and $(-\sqrt {10}-3,1)$, respectively.

In the tangential thrust case,

$$ \frac{\partial a}{\partial\psi}(0,0) = -2,\qquad \frac{\partial a}{\partial\varphi}(0,0) = \frac{1}{2}, \qquad \frac{\partial b}{\partial \psi}(0,0) = 0,\qquad \frac{\partial b}{\partial\varphi}(0,0) = 1. $$

(77)

The eigenvalues of the Jacobian $\bigl( {\scriptsize\begin{matrix} -2&1/2\cr 0 & 1\end{matrix}} \bigr)$ at (0,0) are 1 (unstable) and −2 (stable), associated to the eigenvectors (1/6,1) and (1,0), respectively.

6. Values of c at equilibria. One deduces L(0,0)=L(π,0)=1 from (29), (30), (31) and M(0,0)=M(π,0)=1 from (32), (33). According to (45) and (46), this implies c(0,0)=1, c(0,π)=−1 in both cases.

7. Stable and unstable manifolds of (0,0).

7.1. Full control case. The unstable manifold is the union of the equilibrium (0,0) and two solutions that tend to (0,0) as time τ tends to −∞ and are, according to (76), both tangent to the line $\{\psi=(\sqrt{10}-3)\varphi\}$ at (0,0). One of the solutions approaches with positive ψ and φ and the other with negative ψ and φ. We consider only the first one and call it $\tau\mapsto(\bar{\psi}(\tau ),\bar{\varphi}(\tau))$, defined on the time interval $(-\infty,\bar{\tau}^{+})$, $\bar{\tau}^{+}\leq+\infty$; the result for the other one follows by symmetry. Let $\mathcal{D}_{1}$ be the rectangle

$$\mathcal{D}_1=\bigl\{ (\psi,\varphi)\in\mathcal{C},\ 0<\psi<\pi,\ 0< \varphi\bigr\} . $$

On the one hand, we have $\lim_{\tau\to-\infty}\bar{\psi}(\tau )=\lim_{\tau\to-\infty}\bar{\varphi}(\tau)=0$, $\lim_{\tau\to-\infty}({\bar{\psi}}(\tau)/{\bar{\varphi}}(\tau ))=\sqrt{10}-3$, hence $(\bar{\psi}(\tau),\bar{\varphi}(\tau))\in \mathcal{D}_{1}$ for τ close enough to −∞. On the other hand, the border of $\mathcal{D}_{1}$ is made of the two equilibria and three segments

$$\biggl\{ \psi=0,\,0<\varphi<\frac{\pi}{2}\biggr\} ,\qquad \biggl\{ \psi=\pi,\,0<\varphi< \frac{\pi}{2}\biggr\} ,\qquad \{0<\psi<\pi,\, \varphi=0\}. $$

A short computation shows that

$$ a(0,\varphi) =\frac{\cos\varphi\sin\varphi}{\pi}\int_0^{\pi} \frac{\cos^2 E \,\mathrm{d}E }{ \sqrt{1-\sin^2\varphi\cos^2 E }}, \qquad a(\pi,\varphi)=-a(0,\varphi), $$

(78)

hence a is positive on the first segment and negative on the second one; according to the proof of Point 3 above, b is positive on the last one; hence solutions starting on these segments all enter $\mathcal{D}_{1}$. This proves positive invariance of $\mathcal{D}_{1}$ (solutions may “exit” through the segment $\{\varphi=\frac{\pi}{2}\}$, but they are no longer defined). Hence the solution remains in $\mathcal{D}_{1}$ for all time in the open interval $(-\infty,\bar{\tau}^{+})$. According to Point 3, b(ψ,φ) is positive on $\mathcal{D}_{1}$. This solution cannot remain in a compact subset of $\mathcal{C}$ for all time because then it would have a non-empty ω-limit set that would have to be a union of equilibria and periodic solutions by Poincaré-Bendixon Theorem, but the fact that $\dot{\varphi}>0$ in $\mathcal{D}_{1}$ prevents periodic solutions from existing and the only equilibria are (0,0) and (π,0), that cannot be approached because $\bar {\varphi}(t)$ cannot become small for $\bar{\varphi}(\tau)$ is increasing. Hence necessarily, $\lim_{t\to\bar{\tau}^{+}}\bar{\varphi}(t)=\frac{\pi}{2}$. We have established that the parametrized curve $t\mapsto(\bar{\psi}(t),\bar{\varphi}(t))$, $-\infty<t<\bar{\tau}^{+}$ defines the graph of a function ψ=U(φ), $(0,\frac{\pi}{2})\to(0,\pi )$; it is continuously differentiable from the implicit function theorem: since the right-hand side of the differential equation is continuous, the parameterized curve is continuously differentiable, and we saw that the derivative of φ with respect to the parameter (time) remains positive (again because b>0 in $\mathcal{D}_{1}$).

Let us turn to the stable manifold. It is the union of the equilibrium (0,0) and two solutions that tend to (0,0) as time τ tends to +∞. According to the proof of point 5, both solutions are tangent to the line $\{\psi=-(\sqrt{10}+3)\varphi\}$ at the origin. We consider the solution that approaches (0,0) with negative ψ and positive φ, and call it $\tau\mapsto(\bar{\psi}(\tau ),\bar{\varphi}(\tau))$; the result for the other one follows by symmetry. The proof is now very similar to the one for the unstable manifold, reversing time and replacing $\mathcal{D}_{1}$ by the domain

$$\mathcal{D}_2=\bigl\{ (\psi,\varphi)\in\mathcal{C},\;-\pi<\psi <Z_b(\varphi),\ \varphi>0\bigr\} . $$

Firstly, b(ψ,φ) is negative in this domain. Secondly, the solution is in this domain for τ large enough (obviously ψ is negative and φ is positive, and it is on the right side of ψ=Z _b (φ) because φ tends to zero so the solution must spend some time in the region where b<0). Thirdly, the domain $\mathcal{D}_{2}$ is negatively invariant: its border is made of the equilibria, the segments $\{\psi=-\pi,\,0<\varphi< \frac{\pi}{2}\}$, {−π<ψ<0, φ=0} and the curve $\{\psi=Z_{b}(\varphi), 0<\varphi< \frac{\pi}{2}\}$. Solutions which start on the segments leave $\mathcal{D}_{2}$ because of (78) and the fact that b(ψ,0)<0 if −π<φ<0 (see Point 3 above). Solutions which start on the curve leave the domain $\mathcal{D}_{2}$ because of Point 4 above (at these points, Z _b is differentiable, $Z_{b}(\bar{\psi}(\tau ),\bar{\varphi}(\tau))=0$, $a(\bar{\psi}(\tau),\bar{\varphi}(\tau))>0$, $b(\bar{\psi}(\tau ),\bar{\varphi}(\tau))=0$). This with the second point implies that the solution is in the domain for all time. The end of the proof, i.e. definition of the continuously differentiable S is exactly the same as the previous proof, only with b<0 instead of b>0. Moreover, we get for free that −π<S(φ)<Z _b (φ) from the definition of $\mathcal{D}_{2}$; this and the above implies (66).

7.2. Tangential thrust case. Let us first compute some values of a and b on special lines. Making the change of variables y=cosE one gets

$$\begin{aligned} &a \biggl(\frac{\pi}{2},\varphi \biggr) = -\frac{2 \cos\varphi \operatorname {arctanh}(\sin\varphi)}{\sin\varphi}, \\ &a \biggl(-\frac{\pi}{2},\varphi \biggr) = \frac{2 \cos\varphi \operatorname {arctanh}(\sin\varphi)}{\sin\varphi}, \end{aligned}$$

(79)

$$\begin{aligned} &a (0,\varphi ) = \frac{1}{\pi}\int_{0}^{\pi} \frac{\cos ^2E\;\mathrm{d}E}{\sqrt{1-\sin^2\varphi\cos^2E}\sqrt{1-\cos ^2E}}, \\ & \begin{aligned}[c] b(\psi, 0) &= \operatorname {sign}\bigl( P(\psi,0) \bigr)\;\sqrt{4 - \cot^2\psi }\quad\mbox{in}\ \mathcal{R}_{2}, \\ b(\psi, 0) &= 0\quad\mbox{in}\ \mathcal{R}_{1}. \end{aligned} \end{aligned}$$

(80)

The unstable manifold comprises the equilibrium point (0,0) and two solutions that tend towards it as time τ tends to −∞, tangent, according to (77), to the line {ψ=1/6φ} at (0,0). Thus either both φ and ψ are positive as they approach (0,0) or they are both negative. Of the two solutions, we will only consider the one where φ,ψ are both positive, and call it $\tau\rightarrow(\bar {\psi}(\tau), \bar{\varphi}(\tau))$. Obviously, $(\bar{\psi}(\tau), \bar{\varphi}(\tau))$ is in the rectangle

$$\mathcal{D}_1 = \bigl\{ (\psi, \varphi)\in\mathcal{C}: 0 < \psi< \pi/2, \varphi> 0\bigr\} $$

for τ negative large enough. From Point 3.2 above, b(ψ,φ)>0 in the whole of $\mathcal{D}_{1}$. From (79) and (80), a(0,φ)>0, a(π/2,φ)<0, and b(ψ,0)≥0 for 0<ψ<π, φ>0, thus $\mathcal{D}_{1}$ is positively invariant. The rest of the proof follows exactly the same argument as for the case of the unstable manifold in Point 7.1 above.

We now consider the stable manifold. It comprises the equilibrium point (0,0) and two solutions that tend towards it as time τ tends to +∞. They are both tangent at (0,0) to the stable eigenvector, i.e. (see (77) and the sequel) to the line {φ=0}. Since $\dot{\varphi}$, i.e. b(ψ,φ), is zero on the segment $\{\varphi=0,\,-\arctan\frac{1}{2}\leq\psi\leq\arctan\frac{1}{2}\}$, these solutions follow this segment. We examine the one that approaches (0,0) with negative ψ, the other one follows by symmetry. Call $\tau\mapsto(\bar{\psi}(\tau), \bar{\varphi}(\tau))$ the solution such that $\bar{\psi}(0)=-\arctan\frac{1}{2}$, $\bar{\varphi}(0)=0$. One has $\bar{\varphi}(\tau)=0$ for all positive τ and $\bar{\psi}(\tau)$ is increasing for positive τ and tends to zero as τ→+∞. Define the domain

$$\mathcal{D}_2 = \biggl\{ (\psi, \varphi)\in\mathcal{C}:-\frac{\pi}{2} < \psi< Z_b(0), \varphi> 0\biggr\} . $$

The solution is outside $\mathcal{D}_{2}$ for positive τ but on its border at τ=0. From (79), $a(-\frac{\pi}{2},\varphi) > 0$ for all φ between 0 and $\frac{\pi}{2}$; from (79), b(ψ,0)<0 for $\psi\in(-\frac{\pi}{2},-\arctan\frac{1}{2})$ ($-\arctan\frac{1}{2}$ is Z _b (0)); from point 4 above, a(Z _b (φ),φ)>0 if $\psi\in( 0,\frac{\pi}{2})$. Hence $\mathcal{D}_{2}$, as well as its topological closure, are negatively invariant. Since $(\bar {\psi}(0), \bar{\varphi}(0))$ is on the boundary of $\mathcal{D}_{2}$, one has $(\bar{\psi}(\tau), \bar{\varphi }(\tau))\in\mathcal{D}_{2}$ for all τ∈(τ ⁻,0) where (τ ⁻,+∞) is the maximal interval of definition of the solution we consider. From (59) and (58), b(ψ,φ)<0 for all (ψ,φ) in $\mathcal{D}_{2}$. Then, following the same argument as in the proof concerning the stable manifold in Point 7.1 above, we obtain that the restriction to negative times of the solution is the graph φ=S(ψ) where $S:[0,\frac{\pi}{2})\to(-\frac{\pi}{2},0]$ is continuously differentiable on $(0,\frac{\pi}{2})$ and $S(0)=-\arctan\frac{1}{2}$. We already noticed that the other part of the solution covers the segment $\{\varphi=0,\,-\arctan\frac{1}{2}\leq\psi<0\}$. This ends the proof of Point 7 ((63) to (66)) in the tangential thrust case.  □

Appendix B: Proof of Theorem 3

Theorem 3 and this section are independent of the rest of the paper: here we only refer to the seven conditions ranging from Eq. (53) to Eq. (66).

Lemma 1

Assume that a,b,c satisfy (53) (i.e. point 1).

If $\tau\mapsto(\psi(\tau),\varphi(\tau))\in\mathcal {C}$ is a solution of (54) defined on the time interval [0,τ _fin], then τ↦(ψ ^♯(τ),φ ^♯(τ)) and τ↦(ψ ⁺(τ),φ ⁺(τ)) with

$$ \begin{aligned}[c] &\psi^\sharp(\tau)=-\psi(\tau),\qquad \psi^+(\tau)=\psi(\tau _{\mathrm{fin}}-\tau)+\pi, \\ &\varphi^\sharp(\tau)=-\varphi(\tau),\qquad \varphi^+(\tau )=\varphi(\tau_{\mathrm{fin}}-\tau), \end{aligned} $$

(81)

are also solutions of (54) on the same time interval [0,τ _fin] and they satisfy

$$ \int_0^{\tau_{\mathrm{fin}}}c\bigl( \psi^\sharp(\tau),\varphi^\sharp (\tau)\bigr)\mathrm{d}\tau=\int _0^{\tau_{\mathrm{fin}}}c\bigl(\psi^+(\tau ),\varphi^+(\tau)\bigr) \mathrm{d}\tau=\int_0^{\tau_{\mathrm {fin}}}c\bigl(\psi(\tau), \varphi(\tau)\bigr)\mathrm{d}\tau. $$

(82)

Proof

This is straightforward. □

We also use the “♯” and “+” notation to denote the transformations in $\mathcal{C}$:

$$ (\psi,\varphi)^\sharp=(-\psi,-\varphi),\qquad (\psi, \varphi )^+=(\pi+\psi,\varphi). $$

(83)

Let us make further constructions and remarks on the conditions (53)–(66) before proceeding with the proof per se.

Stable and Unstable Manifolds

Equation (61) implies that the Jacobian of the vector field at the equilibrium (0,0) has two real eigenvalues, of which one is positive and the other negative; i.e. (0,0) is an hyperbolic saddle (see e.g. [14, Sect. 8.3]). Thus it has a stable manifold $\mathcal{S}^{0}$ and an unstable manifold $\mathcal{U}^{0}$; these are curves passing through (0,0) tangent to the corresponding eigenvectors. Their existence is a consequence of (61) but point 7 assumes a more specific description.

The Number $\overline{\sigma}$

Everything may be stated in a much simpler if $\overline{\sigma}=0$: in particular in Points 3 and 7, S and Z _b may be continued into continuous even maps $(-\frac{\pi}{2},\frac{\pi}{2})\to\mathbb{R}$, and, for instance, the equations of $\mathcal{S}^{0}$ and $\mathcal{U}^{0}$, instead of (65), as $\{\psi=S(\varphi),-\frac{\pi}{2}<\varphi<\frac{\pi}{2}\}$ and $\{\psi =U(\varphi),-\frac{\pi}{2}<\varphi<\frac{\pi}{2}\}$. We would have preferred this simpler formulation but we do not assume $\overline{\sigma}=0$ because the proof of Theorem 2 in the tangential case uses Theorem 3 with a nonzero $\overline{\sigma}$ ($\overline{\sigma}=\arctan \frac{1}{2}$).

However, in order to avoid considering positive and negative φ’s as different cases in (65), (58) and (59), we define the functions $U^{0}:[-\frac{\pi}{2},\frac{\pi}{2}]\to[-\pi,\pi]$, $S^{0}:[-\frac{\pi}{2},\frac{\pi}{2}]\setminus\{0\}\to[-\pi,\pi]$, $Z_{b}^{0}:[-\frac{\pi}{2},\frac{\pi}{2}]\setminus\{0\}\to(-\frac{\pi}{2},\frac{\pi}{2})$ after S, U, Z _b ; these functions are odd and coincide with the former on $(0,\frac{\pi}{2}]$:

$$\begin{aligned} & S^0(-\varphi)=-S^0(\varphi) ,\qquad U^0(-\varphi)=-U^0(\varphi) ,\qquad Z_b^0(- \varphi)=-Z_b^0(\varphi), \end{aligned}$$

(84)

$$\begin{aligned} &0<\varphi\leq\frac{\pi}{2}\quad \Rightarrow\quad S^0( \varphi)=S(\varphi) ,\quad U^0(\varphi)=U(\varphi) ,\quad Z_b^0( \varphi )=Z_b(\varphi). \end{aligned}$$

(85)

Then, the description (65) of the stable and unstable manifolds of (0,0) may be replaced by

$$ \begin{aligned}[c] &\mathcal{S}^0=\bigl\{ (\psi,\varphi)\in\mathcal{C},\;\varphi\neq0\mbox { and }\psi=S^0(\varphi)\bigr\} \cup\,[-\overline{\sigma},\overline {\sigma}]\times\{0\}, \\ &\mathcal{U}^0=\bigl\{ (\psi,\varphi)\in\mathcal{C},\;\psi=U^0(\varphi )\bigr\} \end{aligned} $$

(86)

and (58) may be replaced by

$$b(\psi,\varphi)=0\quad \Leftrightarrow\quad \begin{cases} \varphi=0\quad\mbox{and}\quad\psi\in[-\overline{\sigma},\overline {\sigma}]\cup[\pi-\overline{\sigma},\pi+\overline{\sigma}] \\ \quad\mbox{or}\ \varphi\neq0\quad\mbox{and}\quad\psi\in\{Z_b^0(\varphi)\}\cup \{Z_b^\pi(\varphi)\}. \end{cases} $$

The Equilibrium Point (π,0)

The “+” symmetry (see (81)) obviously maps (0,0) to (π,0), the stable manifold of (0,0) to the unstable manifold of (π,0), the unstable one to the stable one and the set of zeroes of b to itself. Define $\mathcal{S}^{\pi}$, $\mathcal{U}^{\pi}$, $Z_{b}^{\pi}$ after $\mathcal{S}^{0}$, $\mathcal{U}^{0}$, $Z_{b}^{0}$ by

$$ S^\pi(\varphi)=\pi+U^0(\varphi),\qquad U^\pi(\varphi)=\pi +S^0(\varphi),\qquad Z_b^\pi( \varphi)=\pi+Z_b^0(\varphi). $$

(87)

From (84), (85) and (87), the relation (66) translates into

$$ \begin{aligned}[c] 0&<\varphi<\frac{\pi}{2}\quad\Rightarrow\quad \begin{cases} S^0(\varphi)<Z_b^0(\varphi)<0<U^0(\varphi),\\ U^\pi(\varphi )<Z_b^\pi(\varphi)<\pi<S^\pi(\varphi), \end{cases} \\ -\frac{\pi}{2}&<\varphi<0 \quad\Rightarrow\quad \begin{cases} U^0(\varphi) <0<Z_b^0(\varphi)<S^0(\varphi),\\ S^\pi(\varphi) <\pi<Z_b^\pi(\varphi)<U^\pi(\varphi). \end{cases} \end{aligned} $$

(88)

The stable and unstable manifolds of (π,0) are:

$$ \begin{aligned}[c] \mathcal{S}^\pi&=\bigl\{ (\psi,\varphi)\in\mathcal{C},\;\psi=S^\pi (\varphi)\bigr\} , \\ \mathcal{U}^\pi&=\bigl\{ (\psi,\varphi)\in\mathcal{C},\;\varphi\neq 0\mbox{ and }\psi=U^\pi(\varphi)\bigr\} \cup\,[-\overline{\sigma },\overline{\sigma}]\times\{0\}. \end{aligned} $$

(89)

Also, (58) and (59) become:

$$\begin{aligned} & b(\psi,\varphi)=0\quad \Leftrightarrow\quad \begin{cases} \varphi=0\quad\mbox{and}\quad\psi\in[-\overline{\sigma},\overline {\sigma}]\cup[\pi-\overline{\sigma},\pi+\overline{\sigma}] \\ \quad \mbox{or }\varphi\neq0\quad\mbox{and}\quad\psi\in\{Z_b^0(\varphi)\}\cup \{Z_b^\pi(\varphi)\}, \end{cases} \end{aligned}$$

(90)

$$\begin{aligned} & b(\psi,\varphi)>0 \quad\mbox{if } \begin{cases} \varphi=0\quad\mbox{and}\quad \overline{\sigma}<\psi<\pi+\overline {\sigma} \\ \quad\mbox{or } \varphi\neq0\quad\mbox{and}\quad Z_b^0(\varphi)<\psi<Z_b^\pi (\varphi). \end{cases} \end{aligned}$$

(91)

Invariant Regions of $\mathcal{C}$

The stable and unstable manifolds $\mathcal{S}^{0}$, $\mathcal{U}^{0}$, $\mathcal{S}^{\pi}$, $\mathcal{U}^{\pi}$, that intersect at the equilibria (0,0) and (π,0) are invariant sets that divide the cylinder $\mathcal{C}$ into six open regions:

$$\begin{aligned} F&=\bigl\{ (\psi,\varphi),\;0<\varphi<\pi/2 \ \mbox{and}\ S^0(\varphi) <\psi<U^0(\varphi)\bigr\} , \end{aligned}$$

(92)

$$\begin{aligned} F^+&=\bigl\{ (\psi,\varphi),\;0<\varphi<\pi/2 \ \mbox{and}\ U^\pi(\varphi)<\psi<S^\pi(\varphi)\bigr\} , \end{aligned}$$

(93)

$$\begin{aligned} F^\sharp&=\bigl\{ (\psi,\varphi),\;- \pi/2<\varphi<0 \ \mbox{and} \ U^0(\varphi)<\psi<S^0( \varphi)\bigr\} , \end{aligned}$$

(94)

$$\begin{aligned} F^{\sharp+}&=\bigl\{ (\psi,\varphi),\;- \pi/2<\varphi<0 \ \mbox{and}\ S^\pi(\varphi)< \psi<U^\pi(\varphi)\bigr\} , \end{aligned}$$

(95)

$$\begin{aligned} E&= \bigl\{ (\psi,\varphi),S^0(\varphi)<\psi<S^\pi(\varphi) \ \mbox{if}\ \varphi\leq0, \ U^0(\varphi)<\psi <U^\pi(\varphi) \ \mbox{if}\ \varphi\geq0\bigr\} , \end{aligned}$$

(96)

$$\begin{aligned} E^+&= \bigl\{ (\psi,\varphi),U^\pi(\varphi)<\psi<U^0(\varphi) \ \mbox{if} \ \varphi\leq0,\ S^\pi(\varphi )<\psi<S^0(\varphi) \ \mbox{if} \ \varphi\geq0\bigr\} . \end{aligned}$$

(97)

E is self-symmetric under the “♯” symmetry and E ⁺ is its own image under the “+” symmetry; F ⁺, F ^♯, F ^♯+ are the images of F by the “♯” and “+” symmetries; see Lemma 1. These regions are represented in Fig. 7.

Fig. 7

This picture reflects qualitatively the assumptions on a and b. It is provided as a help to follow the proof; a precise numerical drawing is provided in Figs. 1 and 2 for the specific expression of a,b in the case of full control or tangential thrust. The six invariant regions separated by the stable and unstable manifolds of (0,0) and (π,0) are shown. The other curves are $\psi=Z_{b}^{0}(\varphi)$ and $\psi=Z_{b}^{\pi}(\varphi)$, where b(ψ,φ) changes sign

We now state and prove two preliminary lemmas and give the proper proof of Theorem 3.

Lemma 2

Assume that a,b satisfy assumptions (53) to (60), and consider a solution t↦(ψ(t),φ(t)) of (54) defined on [0,τ _fin], τ _fin>0.

• 1. If it starts in E or in the upper part of the unstable manifold $\mathcal{U}^{0}$, φ(.) is monotonic increasing.

• 2. If it starts in F, then

  • if it starts in $\{(\psi,\varphi),\,Z_{b}^{0}(\varphi)\leq\psi<U^{0}(\varphi)\}$, it remains in this part of E and φ(.) is monotonic increasing,

  • if it starts in $\{(\psi,\varphi),\,S^{0}(\varphi)<\psi <Z_{b}^{0}(\varphi)\}$, either it remains in this part of E and φ(.) is monotonic decreasing, or there is some $\bar{\tau}$, $0<\bar{\tau}<\tau_{\mathrm{fin}}$ such that t↦φ(t) is monotonic decreasing for t between 0 and $\bar{\tau}$, minimum for $t=\bar{\tau}$ and monotonic increasing for t between $\bar{\tau}$ and τ _fin, with $\psi(\bar{\tau})-Z_{b}^{0}(\varphi(\bar{\tau}))=b(\psi(\bar{\tau}),\varphi(\bar{\tau}))=0$.

• 3. If it starts in the upper part of the stable manifold $\mathcal{S}^{0}$, φ(.) is monotonic non-increasing.

The behavior in the regions E ⁺, F ⁺, F ^♯, F ^♯+ and on the other pieces of stable or unstable curves are obtained by symmetry; see Lemma 1.

Proof

Points 1 and 3 are obvious because b is negative in E and in the upper part of $\mathcal{U}^{0}$ while it is positive in the upper part of $\mathcal{S}^{0}$ except, if $\overline {\sigma}$ is nonzero, on the segment {φ=0}, where it is zero. Let us prove point 2. In the region F, according to (59), b has the sign of $\psi-Z_{b}^{0}(\varphi)$. Using differentiability of $Z_{b}^{0}$ away from φ=0 (see (63)), one may compute the derivative of $\psi-Z_{b}^{0}(\varphi)$ with respect to time along a solution; it is $a(\psi,\varphi)-{Z_{b}^{0}}'(\varphi)b(\psi,\varphi )$, which, according to (60), is positive when $\psi-Z_{b}^{0}(\varphi)=0$, i.e. when b(ψ,φ)=0. Hence the region where b>0 is positively invariant, this accounts for the behavior of solutions starting in {b>0}, and no solution may stay on the locus where b=0, this accounts for solutions that start in {b<0}: either they stay in this part of F or they cross {b=0} at one time and then remain in {b>0}. □

For any number f, $0<f<\frac{\pi}{2}$, let

$$ \mathcal{C}_f=\bigl\{ (\psi,\varphi)\in\mathcal{C} ,\;|\varphi|<f\bigr\} . $$

(98)

Lemma 3

Assume that a,b,c satisfy assumptions (53) to (62). For any number f, $0<f<\frac{\pi}{2}$, there are two neighborhoods Ω ₀ and Ω _π of (0,0) and (π,0) respectively and, a number T(f)≥0 such that

$$\begin{aligned} & (\psi,\varphi)\in\varOmega_0\quad\Rightarrow\quad c (\psi, \varphi)> \frac{1}{2},\qquad (\psi,\varphi)\in \varOmega_\pi\quad\Rightarrow\quad c (\psi,\varphi )<- \frac{1}{2}, \end{aligned}$$

(99)

$$\begin{aligned} &\textit{no\ solution}\ t\mapsto\bigl(\psi(t),\varphi(t)\bigr)\ \textit{of}\ (54)\ \textit{may\ cross\ both}\ \varOmega_0\ \textit{and }\ \varOmega_\pi, \end{aligned}$$

(100)

and any solution t↦(ψ(t),φ(t)) defined on the time interval [0,τ _fin] such that $(\psi(t), \varphi (t))\in\mathcal{C}_{f}$ for all t∈[0,τ _fin] satisfies

$$ \operatorname{meas}\bigl\{ t\in[0,\tau_{\mathrm{fin}}],\ \bigl(\psi(t), \varphi (t)\bigr)\notin (\varOmega_0\cup\varOmega_\pi ) \bigr\} \leq T(f). $$

(101)

The left-hand side ($\operatorname {meas}$ stands for the Lebesgue measure of a subset of $\mathbb{R}$) is simply the time spent by the solution outside the neighborhoods Ω ₀ and Ω _π of (0,0) and (π,0); this bound depends on $f<\frac{\pi}{2}$ because a and b could tend to zero as φ tends to $\pm\frac{\pi}{2}$.

Proof of Lemma 3

To deal with the case where the stable or unstable manifolds contain a segment of the ψ-axis, we must account for both the cases where $\overline{\sigma} = 0$ and where $\overline{\sigma} \neq0$. We define the set $\mathcal{K}^{\varepsilon}$ (ε>0) as follows:

$$ \mathcal{K}^{\varepsilon}=\varnothing\quad \mbox{if}\ \overline {\sigma}=0 $$

(102)

and, if $\overline{\sigma}\neq0$,

$$\begin{aligned} \mathcal{K}^{\varepsilon}&= [\varepsilon,\overline{\sigma}+\varepsilon] \times [-\bar{k} \varepsilon,\bar{k} \varepsilon] \,\cup\, [-\overline{\sigma}-\varepsilon,-\varepsilon] \times [-\bar{k} \varepsilon,\bar{k} \varepsilon] \\ &\quad \cup [\pi+\varepsilon,\pi+\overline{\sigma}+\varepsilon] \times [-\bar{k} \varepsilon,\bar{k} \varepsilon] \cup [\pi-\overline{\sigma}-\varepsilon,\pi-\varepsilon] \times [-\bar{k} \varepsilon,\bar{k} \varepsilon] \end{aligned}$$

(103)

with

$$ 0<\bar{k}\leq\min\biggl\{ \,1,\frac{1}{2} \bigg| \frac{\partial a}{\partial\psi}(0,0) \Big/ \frac{\partial a}{\partial\varphi}(0,0)\bigg| \biggr\} . $$

(104)

Since $\frac{\partial b}{\partial\psi}(0,0)$ is zero, (61), (59) and (60) imply $\frac {\partial b}{\partial\varphi}(0,0)>0$, $\frac{\partial a}{\partial\psi}(0,0)<0$, and (66) implies $\frac{\partial a}{\partial\varphi}(0,0)\geq0$. The slope of the tangent to the curve a=0 is $- \frac{\partial a}{\partial\psi}(0,0) / \frac{\partial a}{\partial\varphi}(0,0)$, and the slope of the unstable manifold {ψ=U ⁰(φ)} (i.e. the slope of the eigenvector corresponding to the negative eigenvalue $\frac{\partial a}{\partial\psi}(0,0)$) is $( \partial b/\partial\varphi(0,0)-\frac{\partial a}{\partial\psi}(0,0) ) / \frac{\partial a}{\partial\varphi}(0,0)$, larger than the previous slope. Hence, for some open ball B around the origin,

$$ |\varphi|\leq\bar{k}|\psi|,\quad \psi\neq0,\qquad (\psi,\varphi)\in B \quad \Rightarrow\quad \bigl|a(\psi,\varphi)\bigr|\neq0 \quad\mbox{and}\quad \bigl|\psi-U^0(\varphi)\bigr| \neq0 . $$

(105)

This implies that a does not vanish on $B\cap([\varepsilon ,\overline{\sigma}+\varepsilon]\times[-\bar{k} \varepsilon,\bar{k} \varepsilon])$. On the compact segment $\{(\psi,0),\, 0\leq\psi\leq\overline {\sigma},\, (\psi,0)\notin B\}$, |a(ψ,φ)| has a positive lower bound $\underline{a}$; hence, for ε small enough, a(ψ,φ) is larger that $\frac{1}{2}\underline{a}/2$ on the part of the compact rectangle $[\varepsilon,\overline{\sigma}+\varepsilon]\times[-\bar{k} \varepsilon,\bar{k} \varepsilon]$ that is outside B. Gluing the piece inside B and the piece outside B together, we get that, for ε small enough, a does not vanish on the compact rectangle $[\varepsilon,\overline{\sigma}+\varepsilon ]\times[-\bar{k} \varepsilon,\bar{k} \varepsilon]$. By symmetry, i.e. from (53) and (103), we get that, for ε small enough,

$$ a \text{ does not vanish on } \mathcal{K}^{\varepsilon}. $$

(106)

By a similar argument, since ψ−U ⁰(φ) does not vanish on the compact segment $\{(\psi,0),\, 0\leq\psi\leq\overline{\sigma},\, (\psi,0)\notin B\}$, (105) implies that it also does not vanish on $\mathcal{K}^{\varepsilon}$ for ε small enough either, hence

$$ \mathcal{U}^0\cap\mathcal{K}^{\varepsilon}= \mathcal{U}^\pi\cap \mathcal{K}^{\varepsilon}=\varnothing $$

(107)

for ε small enough. Define the neighborhoods $\varOmega_{0}^{\varepsilon}$ and $\varOmega_{\pi}^{\varepsilon}$ of (0,0) and (π,0) as:

$$\begin{aligned} \begin{aligned}[c] \varOmega_0^{\varepsilon}&= \; \bigl\{ (\psi,\varphi),\; |\psi|<\varepsilon , |\varphi|<\varepsilon\bigr\} , \\ \varOmega_\pi^{\varepsilon}&= \bigl(\varOmega_0^{\varepsilon} \bigr)^+= \bigl\{ (\psi,\varphi),\; |\psi-\pi|<\varepsilon, |\varphi |< \varepsilon\bigr\} . \end{aligned} \end{aligned}$$

(108)

Consider the two distinct solutions going through $(\frac{\pi}{2},0)$ and $(-\frac{\pi}{2},0)$; for ε small enough they cross neither $\varOmega_{\pi}^{\varepsilon}$ nor $\varOmega_{\pi}^{0}$, and hence they separate $\mathcal{C}$ into two regions, one containing $\varOmega_{\pi}^{\varepsilon}$ and the other one $\varOmega_{\pi}^{\varepsilon}$. Hence, for ε small enough, no solution can cross both $\varOmega_{\pi}^{\varepsilon}$ and $\varOmega _{0}^{\varepsilon}$. From (62), it is also clear that, for ε small enough,

$$\min_{(\psi,\varphi)\in\varOmega_0^{\varepsilon}}c (\psi,\varphi )>\frac{1}{2}\quad \mbox{and}\quad \max_{(\psi,\varphi)\in\varOmega_\pi ^{\varepsilon}}c (\psi,\varphi)<-\frac{1}{2}. $$

Let us now fix some ε small enough that this is true, (106) and (107) hold and no solution can cross both $\varOmega_{\pi}^{\varepsilon}$ and $\varOmega _{0}^{\varepsilon}$. Take

$$ \varOmega_0=\varOmega_0^{\varepsilon},\qquad \varOmega_\pi=\varOmega_\pi ^{\varepsilon},\qquad \mathcal{K}= \mathcal{K}^{\varepsilon} $$

(109)

for this fixed value of ε. With this choice, one has

$$ \mathcal{U}^0\cap\mathcal{K}=\mathcal{U}^\pi \cap\mathcal {K}=\varnothing, $$

(110)

and there is some $\underline{a}>0$ such that

$$\begin{aligned} (\psi,\varphi)\in \mathcal{K}\quad \Rightarrow\quad \bigl|a(\psi,\varphi )\bigr|> \underline{a} \end{aligned}$$

(111)

and (99) and (100) hold: we only need to prove that (101) holds as well.

First, “thicken” the curves $\psi=Z_{b}^{0}(\varphi)$ and $\psi =Z_{b}^{\pi}(\varphi)$ where b vanishes, using the flow Φ (see (55)):

$$ \begin{aligned}[c] \varSigma_0&= \biggl\{ \varPhi\bigl(Z_b^0(\varphi),\varphi,t\bigr) ,\; - \frac{\pi}{2}< \varphi< \frac{\pi}{2},\, \varphi\neq0,\, -\frac{1}{2}< t<\frac{1}{2} \biggr\} \cap\mathcal{C}_f, \\ \varSigma_\pi&=(\varSigma_0)^+= \biggl\{ \varPhi \bigl(Z_b^\pi(\varphi),\varphi,t\bigr) ,\; - \frac{\pi}{2}< \varphi< \frac{\pi}{2},\, \varphi\neq0, - \frac{1}{2}< t< \frac{1}{2} \biggr\} \cap\mathcal{C}_f . \end{aligned} $$

(112)

Note

If, for the initial condition $(\psi^{o} ,\varphi ^{o})=(Z_{b}^{\pi}(\varphi),\varphi)$, either $\tau^{-}>-\frac{1}{2}$ or $\tau^{+}<\frac{1}{2}$ (see (54)–(61)), then $\varPhi(Z_{b}^{\pi}(\varphi),\varphi,t)$ is not defined up to $-\frac{1}{2}$ or $\frac{1}{2}$; we however kept, for the sake of simplicity, “$-\frac{1}{2}<t<\frac{1}{2}$” instead of “$\max\{-\frac{1}{2},\tau^{-}\}<t<\min\{\frac{1}{2},\tau^{+}\}$”.

The topological closure of $\mathcal{C}_{f} \setminus ( \varOmega_{0}\cup\varOmega_{\pi}\cup\mathcal{K} \cup\varSigma_{0}\cup\varSigma _{\pi})$ does not contain any zero of b, and is obviously compact. Hence b has a positive lower bound m(f) on that compact set:

$$ (\psi,\varphi)\in \mathcal{C}_f \setminus ( \varOmega_0\cup\varOmega_\pi\cup\mathcal {K}\cup \varSigma_0\cup\varSigma_\pi ) \quad \Rightarrow\quad \bigl|b(\psi, \varphi)\bigr|>m(f)>0. $$

(113)

Now consider a solution $[0,\tau_{\mathrm{fin}}]\to\mathcal{C}_{f}$, and partition [0,τ _fin] as follows:

$$\begin{aligned} &[0,\tau_{\mathrm{fin}}]=I_0\cup I_1\cup I_2 \cup I_3\quad \mbox{with}\quad \\ & \quad I_0= \bigl\{ t\in[0,\tau_{\mathrm{fin}}],\; \bigl(\psi(t), \varphi(t)\bigr)\in\varOmega_0\cup\varOmega_\pi \bigr\} , \\ &\quad I_1= \bigl\{ t\in[0,\tau_{\mathrm{fin}}],\; \bigl(\psi(t), \varphi(t)\bigr) \in\mathcal{K} \bigr\} , \\ & \quad I_2= \bigl\{ t\in[0,\tau_{\mathrm{fin}}],\; \bigl(\psi(t), \varphi(t)\bigr) \in\varSigma_0\cup\varSigma_\pi\mbox{ and } t\notin I_0\cup I_1 \bigr\} , \\ &\quad I_3= \bigl\{ t\in[0,\tau_{\mathrm{fin}}],\; \bigl(\psi(t), \varphi(t)\bigr)\notin \varOmega_0\cup\varOmega_\pi\cup \mathcal {K}\cup\varSigma_0\cup\varSigma_\pi \bigr\} . \end{aligned}$$

(114)

Obviously,

$$ \operatorname {meas}\,\bigl\{ t\in[0,\tau_{\mathrm{fin}}],\ \bigl(\psi(t), \varphi (t)\bigr)\notin (\varOmega_0\cup\varOmega_\pi ) \bigr\} = \operatorname {meas}I_1 + \operatorname {meas}I_2 + \operatorname {meas}I_3 . $$

(115)

Either the solution is one of the two equilibria or it stays in one of the stable or unstable manifolds or in one of the six regions E, E ⁺, F, F ⁺, F ^♯, F ^♯+. According to Lemma 1, and seen that the neighborhoods are invariant by the ♯ symmetry and exchanged by the + symmetry, it is enough to prove the property for solutions in the regions E and F, the equilibrium (0,0) and the upper parts of its stable and unstable manifolds $\mathcal{S}^{0}$ and $\mathcal{U}^{0}$.

In order to bound $\operatorname {meas}I_{1}$ if $\overline{\sigma}\neq0$ (it is zero if $\overline{\sigma}=0$), let us prove that

$$ \begin{array}[b]{l} \text{either the solution does not cross}\ \mathcal{K} \\ \text{or there are times}\ t_1,t_2,\ 0\leq t_1<t_2\leq\tau_{\mathrm {fin}}\ \mbox{such that the solution is} \\ \text{in}\ \mathcal{K}\ \mbox{on the time interval}\ [t_1,t_2]\ \mbox{and outside}\ \mathcal{K}\ \mbox{on}\ [0,\tau_{\mathrm{fin}}]\setminus[t_1,t_2]. \end{array} $$

(116)

• This is obvious if the solution is an equilibrium or is on the unstable manifold $\mathcal{U}^{0}$, that do not cross $\mathcal{K}$ (see (110)).

• In E: among the rectangles in (103), only $[\varepsilon,\overline{\sigma}+\varepsilon]\times [-\bar{k} \varepsilon,\bar{k} \varepsilon]$ and $[\pi-\overline{\sigma}-\varepsilon,\pi-\varepsilon] \times [-\bar{k} \varepsilon,\bar{k} \varepsilon]$ intersect E; a solution that lies in E cannot cross both rectangles because the solution passing through $(0,\frac{\pi}{2})$ separates them. Consider a solution that crosses one of them, say $[\varepsilon ,\overline{\sigma}+\varepsilon]\times [-\bar{k} \varepsilon,\bar{k} \varepsilon]$ (the situation around $[\pi -\overline{\sigma}-\varepsilon,\pi-\varepsilon] \times [-\bar{k} \varepsilon,\bar{k} \varepsilon]$ is similar). Since $\dot{\psi}$ is negative in the rectangle (a does not change sign according to (106) and $a(0,\overline{\sigma})<0$ according to (60)) and $\dot{\varphi}$ is positive in E, a solution may only exit through the top or left-hand edge; if it exits through the top edge $\{\varphi=\bar{k} \varepsilon\}$, it will not enter again because φ will remain larger than $\bar{k} \varepsilon$. If it exits through the left-hand edge, the fact that $\dot{\varphi}>0$ in E only allows it to enter again through the same edge, but this is impossible because $\dot{\psi}>0$ on this edge; this proves (116).

• In F: from (110), $[-\overline{\sigma}-\varepsilon,-\varepsilon ] \times [-\bar{k} \varepsilon,\bar{k} \varepsilon]$ is the only rectangle in (103) that intersects F; hence the solution may only cross this rectangle. Since, according to (106), a does not change sign in the rectangle and, according to (60), $a(0,\overline{\sigma})>0$, a is positive on the rectangle, then the vector field points inwards on the left-hand edge $\{\psi=-\overline{\sigma}-\varepsilon\}$ and outwards on the right-hand edge {ψ=−ε}. The bottom edge is not in F. A solution may only exit through the top or right-hand edge; if it exits through the top edge, it means that φ(t) is increasing at the exit time, and so, according to Lemma 2, it will continue increasing and cannot go back to the rectangle. Also, if it exits through the right-hand edge, re-entering the rectangle through the top or left-hand edge would require φ to increase and reach at least kε, making it impossible to reach the rectangle again because φ will continue increasing. This proves (116) for solutions that remain in F.

• A solution in the upper part of the stable manifold $\mathcal{S}^{0}$ also satisfies (116) because it enters the rectangle through the left-hand edge or the top edge and exits it through the right-hand edge and then goes asymptotically to (0,0).

We have proved (116) for any solution. Either I ₁=∅ or I ₁=[t ₁,t ₂] and connectedness implies that the solution stays in a single rectangle of $\mathcal{K}$. Using (111), ψ(t) varies monotonically in [t ₁,t ₂], and so its variation is at most $\overline{\sigma}$: $\underline{a}(t_{2}-t_{1})<\overline{\sigma}$. This yields

$$ \operatorname {meas}I_1\leq\overline{\sigma}/\underline{a}. $$

(117)

Solutions in E or in the stable and unstable manifolds cross neither Σ ₀ nor Σ _π ; hence I ₂=∅ for these solutions. Solutions in F may cross Σ ₀ but not Σ _π , and they cannot enter Σ ₀ again after leaving it because the region between Σ ₀ and the unstable manifold $\mathcal{U}^{0}$ is invariant in positive time. They stay in Σ ₀ on a time-interval of length at most 1 from the definition (112). Hence, for any solution,

$$ \operatorname {meas}I_2\leq1. $$

(118)

Using Lemma 2, for any solutions in E or F or the upper part of $\mathcal{S}^{0}$ or $\mathcal{U}^{0}$, the total variation of φ on the interval [0,τ _fin] is at most π−2ℓ. The inequality (113) then implies

$$ \operatorname {meas}I_3\leq(\pi-2\ell) / m(f). $$

(119)

Setting $T(f)= \overline{\sigma} /\underline{a} +1+ (\pi-2\ell ) / m(f)$, (115), (117), (118) and (119) imply (101). □

Proof of Theorem 3

From Lemma 1, the “+” symmetry allows one to interchange φ ¹ and φ ⁰ while the “♯” symmetry changes their sign: we may assume

$$ -\frac{\pi}{2}<\varphi^0\leq\varphi^1<\frac{\pi}{2}\quad \mbox{and}\quad \varphi^1\geq0 $$

(120)

in the proof without loss of generality. We distinguish four cases.

Case a: $-\frac{\pi}{2}< \varphi^{0}\leq0<\varphi ^{1}<\frac{\pi}{2}$. In this paragraph, by convention,

$$ \text{if}\ \varphi^0=0,\quad \mbox{then}\ S^0\bigl( \varphi^0\bigr)\ \mbox{stands for}\ \overline {\sigma}\ \mbox{and}\ S^\pi \bigl(\varphi^0\bigr)\ \mbox{stands for}\ \pi-\overline{\sigma}. $$

(121)

The solutions such that φ(0)=φ ⁰, φ(τ _fin)=φ ¹, must be in the region E (see Lemma 2 and Fig. 7), and satisfy S ⁰(φ ⁰)<ψ(0)<S ^π(φ ⁰). For any χ in the open interval (S ⁰(φ ⁰),S ^π(φ ⁰)), let (ψ ^χ(.),φ ^χ(.)) be the unique solution to the Cauchy problem (54) with initial condition

$$ \psi^\chi(0)=\chi,\qquad \varphi^\chi(0)= \varphi^0. $$

(122)

It is—see (55)—continuous with respect to χ and continuously differentiable with respect to τ. Since this solution is in E, φ ^χ is an increasing function of time, and so there is a unique time $\tau_{\mathrm {fin}}^{\chi}>0$ such that

$$ \varphi^\chi\bigl(\tau_{\mathrm{fin}}^\chi \bigr)=\varphi^1. $$

(123)

Since $b(\,\varphi^{\chi}(\tau_{\mathrm{fin}}^{\chi}),\,\psi^{\chi}(\tau_{\mathrm{fin}}^{\chi}))>0$, there is a constant k>0 such that |φ ^χ(t′)−φ ^χ(t″)|>k|t′−t″| for t′,t″ in a neighborhood of $\tau_{\mathrm{fin}}^{\chi}$. This implies that $\tau_{\mathrm{fin}}^{\chi}$ depends continuously on χ. This allows us to define a continuous map $\varLambda:(S^{0}(\varphi ^{0}),S^{\pi}(\varphi^{0})) \to\mathbb{R}$ by

$$ \varLambda(\chi)=\int_0^{\tau_{\mathrm{fin}}^\chi} c \bigl(\psi^\chi (s),\varphi^\chi(s)\bigr)\mathrm{d}s. $$

(124)

All we need to prove is that, for any $\bar{\lambda}\in\mathbb{R}$, there is at least one χ in (S ⁰(φ ⁰),S ^π(φ ⁰)) such that $\varLambda(\chi)=\bar{\lambda}$, i.e. that Λ is onto. Since Λ is continuous, it is sufficient to prove that

$$ \lim_{\substack{\chi\to S^0(\varphi^0)\\\chi>S^0(\varphi ^0)}}\varLambda(\chi)=+\infty ,\qquad \lim _{\substack{\chi\to S^\pi(\varphi^0)\\\chi< S^\pi(\varphi ^0)}}\varLambda(\chi)=-\infty . $$

(125)

The solution $t\mapsto(\psi^{S^{0}(\varphi^{0})}(t),\varphi ^{S^{0}(\varphi^{0})}(t))$ of (54) with initial condition (S ⁰(φ ⁰), φ ⁰) is on the stable manifold of (0,0); it is defined on [0,+∞); $\varphi^{S^{0}(\varphi^{0})}(t)$ is negative for all time and tends to zero as t→+∞. By continuity with respect to initial conditions, the solutions (ψ ^χ(.),φ ^χ(.)) starting from (χ,φ ⁰) with χ close enough to S ⁰(φ ⁰) are also defined on $[0,\overline{\tau}]$ for arbitrarily large fixed $\overline{\tau}>0$, and converge uniformly to $(\psi^{S^{0}(\varphi^{0})}(.),\varphi^{S^{0}(\varphi^{0})}(.))$ on the compact interval $[0,\overline{\tau}]$ as χ→S ⁰(φ ⁰). This proves that, for χ close enough to S ⁰(φ ⁰), $\varphi^{\chi}(\overline{\tau})<0$ and hence $\tau_{\mathrm{fin}}>\overline{\tau}$. The situation near S ^π(φ ⁰) being similar, we have proved that

$$ \lim_{\substack{\chi\to S^0(\varphi^0)\\\chi>S^0(\varphi^0)}}\tau _{\mathrm{fin}}^\chi= \lim_{\substack{\chi\to S^\pi(\varphi^0)\\\chi< S^\pi(\varphi ^0)}}\tau_{\mathrm{fin}}^\chi= +\infty. $$

(126)

Now define Ω ₀ and Ω _π according to Lemma 3, and $\overline{\tau}$ large enough that $(\psi^{S^{0}(\varphi^{0})}(\overline{\tau}), \varphi^{S^{0}(\varphi ^{0})}(\overline{\tau}))$ is in Ω ₀. For χ close enough to S ⁰(φ ⁰), we also have $(\psi^{\chi}(\overline{\tau}),\varphi^{\chi}(\overline{\tau})) \in S^{0}(\varphi^{0})$; hence, according to (100), solutions (ψ ^χ(.),φ ^χ(.)) with χ close enough to S ⁰(φ ⁰) never cross Ω _π . The interval $[0,\tau_{\mathrm{fin}}^{\chi}]$ is partitioned into times t such that (ψ ^χ(t),φ ^χ(t))∈Ω ₀ and also (ψ ^χ(t),φ ^χ(t))∉(Ω ₀∪Ω _π ). Since, according to (101) (with f=max{|φ ⁰|,|φ ¹|}) $\operatorname {meas}\{t\in[0,\tau_{\mathrm {fin}}^{\chi}],\ (\psi^{\chi}(t),\varphi^{\chi}(t))\notin (\varOmega_{0}\cup\varOmega_{\pi})\}\leq T(f)$ and $\operatorname {meas}\,\{t\in[0,\tau_{\mathrm{fin}}^{\chi}],\ (\psi^{\chi}(t), \varphi^{\chi}(t))\in\varOmega_{0}\} \geq\tau_{\mathrm {fin}}^{\chi}-T(f)$, then (124) and (99) imply

$$ \varLambda(\chi)\geq\frac{1}{2} \bigl( \tau_{\mathrm {fin}}^\chi-T(f) \bigr) -c_f\,T(f) \quad \mbox{with}\ c_f=\max_{(\psi,\varphi) \in\mathcal{C}, |\varphi|\leq f} \bigl|c (\psi, \varphi)\bigr|, $$

(127)

and this does imply, using (126), the first limit in (125). Similarly, for χ close enough to S ^π(φ ⁰), one has $\operatorname {meas}\{t\in[0,\tau_{\mathrm{fin}}^{\chi}], (\psi^{\chi}(t),\varphi^{\chi}(t))\in\varOmega_{\pi}\} \geq\tau_{\mathrm {fin}}^{\chi}-T(f)$, $\operatorname {meas}\,\{t\in[0,\tau_{\mathrm{fin}}^{\chi}], (\psi^{\chi}(t),\varphi^{\chi}(t))\notin (\varOmega_{0}\cup\varOmega_{\pi})\} \leq T(f)$ and hence, using (127) again,

$$ \varLambda(\chi)\leq-\frac{1}{2} \bigl( \tau_{\mathrm {fin}}^\chi-T(f) \bigr) + c_f\,\,T(f) $$

(128)

with c _f as in (9). This implies, according to (126), the second limit in (125).

Case b: $-\frac{\pi}{2}< \varphi^{0}<0$ and φ ¹=0. If $\overline{\sigma}=0$, the solutions such that φ(0)=φ ⁰, φ(τ _fin)=φ ¹ must be in the region E, and the proof from case (a) applies, where φ ¹ is replaced with zero. If $\overline{\sigma}>0$, the solutions on the stable manifolds {ψ=S ⁰(φ)} and {ψ=S ^π(φ)} (see Fig. 7) also qualify for this case, because φ reaches zero in finite time. Hence we have to examine the solutions such that (ψ(0),φ(0))=(χ,φ ⁰) with χ∈[S ⁰(φ ⁰),S ^π(φ ⁰)] instead of the open interval; the solutions (ψ ^χ(.),φ ^χ(.)) to the Cauchy problem (54)–(122) are still well defined and depend continuously on χ; however, uniqueness of $\tau_{\mathrm{fin}}^{\chi}$ such that (123) holds for χ∈(S ⁰(φ ⁰),S ^π(φ ⁰)) but not for χ=S ⁰(φ ⁰) or χ=S ^π(φ ⁰). If we call $\tau_{\mathrm{fin}}^{S^{0}(\varphi^{0})}$ (resp. $\tau_{\mathrm {fin}}^{S^{\pi}(\varphi^{0})}$) the first time t such that $\varphi^{S^{0}(\varphi^{0})}(t)=0$ (resp. $\varphi^{S^{\pi}(\varphi ^{0})}(t)=0$), the solutions to be considered are these with initial condition (ψ(0),φ(0))=(χ,φ ⁰), S ⁰(φ ⁰)<χ<S ^π(φ ⁰) on the time interval $[0,\tau_{\mathrm{fin}}^{\chi}]$ and these with initial condition (ψ(0),φ(0))=(χ,φ ⁰), χ∈{S ⁰(φ ⁰),S ^π(φ ⁰)} on the time intervals [0,τ], $\tau_{\mathrm{fin}}^{\chi}\leq\tau<+\infty$. With the first set of solutions, one reaches $\bar{\lambda}\in[\varLambda (S^{0}(\varphi^{0})),\varLambda(S^{\pi}(\varphi^{0}))]$; the set of solutions with initial condition (S ⁰(φ ⁰),φ ⁰) allows one to reach $\bar{\lambda}$ larger than Λ(S ⁰(φ ⁰)) as the value of $\int_{0}^{\tau} c(\psi^{S^{0}(\varphi^{0})}(s),\varphi^{S^{0}(\varphi ^{0})}(s)) \mathrm{d}s$ varies from Λ(S ⁰(φ ⁰)) to +∞ as τ varies from $\tau^{S^{0}(\varphi^{0})}$ (the smallest such value such that φS ⁰(φ ⁰)(τ)=0) to +∞; these solutions with initial condition (S ^π(φ ⁰),φ ⁰) allow one to reach $\bar{\lambda}$ smaller than Λ(S ^π(φ ⁰)).

Case c: φ ⁰=φ ¹=0. It suffices to chose $\tau_{\mathrm{fin}}=|\bar{\lambda}|$ and the solution to be the equilibrium (0,0) if $\bar{\lambda}\geq0$ or the equilibrium (π,0) if $\bar{\lambda}\geq0$. Then (67) is satisfied because c(0,0)=1, c(π,0)=−1.

Case d: $0< \varphi^{0}<\varphi^{1}<\frac{\pi}{2}$. This is the other “generic” case, with case (a). According to Lemma 2, the solutions such that φ(0)=φ ⁰, φ(τ _fin)=φ ¹ must lie in one of the regions F, E, or F ⁺ or in the unstable manifolds $\mathcal{U}^{0}$ or $\mathcal{U}^{\pi}$ that separate them (see Fig. 7). These solutions satisfy φ(0)=φ ⁰, hence S ⁰(φ ⁰)<ψ(0)<S ^π(φ ⁰). For any χ in the open interval (S ⁰(φ ⁰),S ^π(φ ⁰)), let (ψ ^χ(.),φ ^χ(.)) be the solution to the Cauchy problem (54)–(122). According to Lemma 2:

• If $S^{0}(\varphi^{0})<\chi< Z_{b}^{0}(\varphi^{0})$ or $Z_{b}^{0}(\varphi^{\pi})<\chi<S^{\pi}(\varphi^{0})$, t↦φ ^χ(t) is first decreasing, then crosses the set of zeroes of b at some time t ^o: $\psi^{\chi}(t^{o})=Z_{b}^{0}(\varphi^{\chi}(t^{o}))$ or $\psi^{\chi}(t^{o})=Z_{b}^{\pi}(\varphi^{\chi}(t^{o}))$ and is increasing for t larger than t ^o. Hence, since φ ^χ(t ^o)<φ ⁰<φ ¹, there is a unique $\tau _{\mathrm{fin}}^{\chi}$ (larger than t ^o) such that $\varphi^{\chi}(\tau_{\mathrm{fin}}^{\chi})=\varphi^{1}$.

• If $Z_{b}^{0}(\varphi^{0})\leq\chi\leq Z_{b}^{\pi}(\varphi^{0})$, t↦φ ^χ(t) is monotonic increasing for positive times and cannot have a limit, hence it takes all the values between φ ⁰ and $\frac{\pi}{2}$ only once and there is a unique $\tau_{\mathrm{fin}}^{\chi}$ such that (123) holds.

In both cases, $Z_{b}^{0}(\varphi^{1})<\psi^{\chi}(\tau_{\mathrm {fin}}^{\chi})<Z_{b}^{\pi}(\varphi^{1})$, hence $b(\,\widehat{\varphi}^{\chi}(\tau_{\mathrm{fin}}^{\chi}),\,\widehat{\psi}^{\chi}(\tau_{\mathrm{fin}}^{\chi}))>0$, and so there is a constant k>0 such that |φ ^χ(t′)−φ ^χ(t″)|>k|t′−t″| for t′,t″ in a neighborhood of $\tau_{\mathrm{fin}}^{\chi}$. This implies that $\tau_{\mathrm{fin}}^{\chi}$ depends continuously on χ. This continuous dependence on χ allows us to define the continuous map $\varLambda:(U^{0}(\varphi^{0}),U^{\pi}(\varphi^{0})) \to\mathbb{R}$ by (124); as in case (a), let us prove the following limits, sufficient to imply that Λ is onto:

$$ \lim_{\substack{\chi\to U^0(\varphi^0)\\\chi>U^0(\varphi ^0)}}\varLambda(\chi)=+\infty ,\qquad \lim _{\substack{\chi\to U^\pi(\varphi^0)\\\chi< U^\pi(\varphi ^0)}}\varLambda(\chi)=-\infty . $$

(129)

This follows as in case (a): first we get

$$ \lim_{\substack{\chi\to U^0(\varphi^0)\\\chi>S^0(\varphi^0)}}\tau _{\mathrm{fin}}^\chi= \lim _{\substack{\chi\to U^\pi(\varphi^0)\\\chi< S^\pi(\varphi ^0)}}\tau_{\mathrm{fin}}^\chi= +\infty $$

and then, with f=|φ ₁|, (127) holds for χ close to U ⁰(φ ⁰) and (128) for χ close to U ^π(φ ⁰).

Case e: $0< \varphi^{0}=\varphi^{1}<\frac{\pi}{2}$. This is a similar to the previous case but degenerate in the sense that $\tau_{\mathrm{fin}}^{\chi}=0$ if $Z_{b}^{0}(\varphi^{0})\leq\chi\leq Z_{b}^{\pi}(\varphi^{0})$. The only nontrivial trajectories that display the same initial and final values of φ lie in the regions F or F ⁺, and they join points on one side of the curve where b vanishes ($\psi=Z_{b}^{0}(\varphi)$ or $\psi=Z_{b}^{\pi}(\varphi )$) to points on the other side. We have

$$\varLambda(\chi) \begin{cases} >0&\text{if}\ U^0(\varphi^1)<\chi<Z_b^0(\varphi^1), \\ =0&\text{if}\ Z_b^0(\varphi^1)\leq\chi\leq Z_b^\pi(\varphi^1), \\ <0&\text{if}\ Z_b^\pi(\varphi^1)<\chi<U^\pi(\varphi^1); \end{cases} $$

(129) still holds and the same arguments prove that Λ is onto.  □