Modelling secondary lymphatic valves with a flexible vessel wall: how geometry and material properties combine to provide function

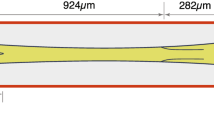
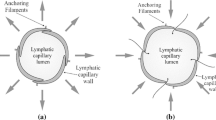
Abstract

A three-dimensional finite-element fluid/structure interaction model of an intravascular lymphatic valve was constructed, and its properties were investigated under both favourable and adverse pressure differences, simulating valve opening and valve closure, respectively. The shear modulus of the neo-Hookean material of both vascular wall and valve leaflet was varied, as was the degree of valve opening at rest. Also investigated was how the valve characteristics were affected by prior application of pressure inflating the whole valve. The characteristics were parameterised by the volume flow rate through the valve, the hydraulic resistance to flow, and the maximum sinus radius and inter-leaflet-tip gap on the plane of symmetry bisecting the leaflet, all as functions of the applied pressure difference. Maximum sinus radius on the leaflet-bisection plane increased with increasing pressure applied to either end of the valve segment, but also reflected the non-circular deformation of the sinus cross section caused by the leaflet, such that it passed through a minimum at small favourable pressure differences. When the wall was stiff, the inter-leaflet gap increased sigmoidally during valve opening; when it was as flexible as the leaflet, the gap increased more linearly. Less pressure difference was required both to open and to close the valve when either the wall or the leaflet material was more flexible. The degree of bias of the valve characteristics to the open position increased with the inter-leaflet gap in the resting position and with valve inflation pressure. The characteristics of the simulated valve were compared with those specified in an existing lumped-parameter model of one or more collecting lymphangions and used to estimate a revised value for the constant in that model which controls the rate of valve opening/closure with variation in applied pressure difference. The effects of the revised value on the lymph pumping efficacy predicted by the lumped-parameter model were evaluated.

Appendix

Equations of the model

$$ {{Mass}} \, {{conservation}}:\frac{{{\text{d}}D}}{{{\text{d}}t}} = \frac{{2\left( {Q_{1} - Q_{2} } \right)}}{\pi LD}. $$

(5)

$$ {{Momentum}} \, {{conservation}}:p_{{\text{a}}} {-}p_{0} = R_{{\text{a}}} Q_{{1}} ;\,\,p_{0} {-}p_{{1}} = R_{{{\text{V1}}}} Q_{{1}} ;\,\,p_{1} - p_{m} = \frac{64}{\pi }\frac{{\mu LQ_{1} }}{{D^{4} }}; $$

(6)

$$ p_{m} - p_{2} = \frac{64}{\pi }\frac{{\mu LQ_{2} }}{{D^{4} }};\,p_{{2}} {-}p_{{\text{p}}} = R_{{{\text{V2}}}} Q_{{2}} ;\,p_{{\text{p}}} {-}p_{{\text{b}}} = R_{{\text{b}}} Q_{{2}} , $$

(7)

where p₁, p_m, and p₂ = pressure at the upstream end, centre, and downstream end of the lymphangion, p_a and p_b = upstream and downstream reservoir pressures, p₀ = pressure upstream of the inlet valve but downstream of the inlet cannula, and p_p = pressure downstream of the outlet valve but upstream of the outlet cannula; Q₁ and Q₂ = flow-rates into and out of the lymphangion, and D = lymphangion diameter. The resistances of the inlet and outlet valves, R_V1 and R_V2, are given by

$$ R_{{{\text{V}}1}} = R_{{{\text{Vn}}}} + \frac{{R_{{{\text{Vx}}}} }}{{1 + {\exp}\left[ { - s_{{\text{o}}} \left( {\Delta p_{{{\text{V}}1}} - \Delta p_{{{\text{o}}1}} } \right)} \right]}};\;R_{{{\text{V}}2}} = R_{{{\text{Vn}}}} + \frac{{R_{{{\text{Vx}}}} }}{{1 + {\exp}\left[ { - s_{{\text{o}}} \left( {\Delta p_{{{\text{V}}2}} - \Delta p_{{{\text{o}}2}} } \right)} \right]}}, $$

(8)

where ∆p_V1 = p₀ − p₁, ∆p_V2 = p₂ − p_p, the open/close threshold ∆p_o1 depends on either p₀ − p_e or p₁ − p_e according to the current valve state, and ∆p_o2 depends on either p₂ − p_e or p_p − p_e (Bertram et al. 2014a). The constants in eqns. A1 − 4 are the lymphangion length L (0.3 cm), the lymph viscosity µ (1 cP), the resistances of the inlet and outlet cannulae (R_a and R_b, respectively), the external pressure p_e, and R_Vn, R_Vx and s_o as defined in the main text.

Constitutive relation Two curves fitted to twitch-contraction data [see Bertram et al. (2017)] describe the maximally active and passive states as

$$ D_{{{\text{act}}}} = f_{{{\text{act}}}} \left( {p_{{{\text{tm}}}} } \right);\;\;D_{{{\text{psv}}}} = f_{{{\text{psv}}}} \left( {p_{{{\text{tm}}}} } \right) $$

(9)

where subscripts act and psv indicate the peak-twitch and pre-twitch states, respectively.

Time course of active contraction:

$$ D = D_{{{\text{psv}}}} - M\left( t \right)\left[ {D_{{{\text{psv}}}} - D_{{{\text{act}}}} } \right], $$

(10)

where the activation waveform M(t) is calculated in two steps; in the first,

$$ M\left( t \right) = 0.5\left\{ {1 - \cos \left[ {2\pi f_{m} \left( {t - t_{{\text{c}}} } \right)} \right]} \right\}, $$

(11a)

where f_m = mf, t_c is the beginning of a contraction, and 1/f is its duration. A function M_j(t) modifies the preliminary M(t) to its final form, where

$$ M_{{\text{j}}} \left( t \right) = m_{{\text{g}}} \left\{ {1 - \cos \left[ {2\pi f\left( {t - t_{{\text{c}}} } \right)} \right]} \right\},\;\;{\text{with}}\;m_{{\text{g}}} = 1/\left( {1 - \cos \frac{\pi }{m}} \right)\;;\quad {\text{if}}\;\;M_{{\text{j}}} \left( t \right) \, > { 1},M\left( t \right) \, = { 1}. $$

(11b)

Constant m multiplies the rate of onset and decay of the active state; with m = 1, M(t) is a sinusoid.

Pressure-dependent diastole The duration of the period of relaxation t_r that starts at t = t_c + 1/f is

$$ t_{r} = \frac{1}{{f_{{{\text{tw}}}} }} - \frac{1}{f}, $$

(12)

where $f_{{{\text{tw}}}} = 60\left( { - 1.39q^{2} + 12.6q + 0.647} \right)$, with $q = {\ln}\left( {1 + {\overline {\Delta {p}}}_{{{\text{tm}}}} } \right)$, ${\overline {\Delta {p}}}_{{{\text{tm}}}}$ being the average transmural pressure over the immediately preceding systole, defined as

$${\overline{\Delta {p}}}_{{{\text{tm}}}} = f.\mathop \int \limits_{{t_{c} }}^{{t_{c} + 1/f}} \Delta p_{{{\text{tm}}}} {\text{d}}t, $$

(13)

where ∆p_tm = p_m − p_e (in cm H₂O).

Valve characteristics The twin curves that determine ∆p_o (Eqs. 4 and 8) for opening and closure of each valve are shown in Fig. 

Fig. 13

Variation of ∆p_o with valve transmural pressure. Solid black and magenta: curves fitted to data measured by Davis et al. (2011) for opening and closing, respectively. Broken magenta: closure curve, times constant cfact (Bertram et al. 2014b). Solid and broken green: opening and closing characteristics, respectively, offset by − 0.6 cm H₂O as for right-hand panels of Fig. 12. Pressure p_int = p_in for opening, p_out for closure

13.