Skip to main content

Advertisement

SpringerLink
Log in

Nonlinear dynamics of slender structures: a new object-oriented framework

  • Original Paper
  • Published:
Computational Mechanics Aims and scope Submit manuscript

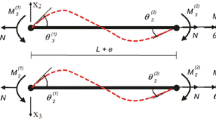
Abstract

With this work, we present a new object-oriented framework to study the nonlinear dynamics of slender structures made of composite multilayer and hyperelastic materials, which combines finite element method and multibody system formalism with a robust integration scheme. Each mechanical system under consideration is represented as a collection of infinitely stiff components, such as rigid bodies, and flexible components like geometrically exact beams and solid-degenerate shells, which are spatially discretized into finite elements. The semi-discrete equations are temporally discretized for a fixed time increment with a momentum-preserving, energy-preserving/dissipative method, which allows the systematic annihilation of unresolved high-frequency content. As usual in multibody system dynamics, kinematic constraints are employed to render supports, joints and structural connections. The presented ideas are implemented following the object-oriented programming philosophy. The approach, which is perfectly suitable for wind energy or aeronautic applications, is finally tested and its potential is illustrated by means of numerical examples.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20
Fig. 21
Fig. 22
Fig. 23

Similar content being viewed by others

References

  1. Reissner E (1972) On one-dimensional finite-strain beam theory: the plane problem. J Appl Math Phys 23:795–804

    MATH  Google Scholar 

  2. Bathe K-J, Bolourchi S (1979) Large displacement analysis of three-dimensional beam structures. Int J Numer Methods Eng 14:961–986

    Article  MATH  Google Scholar 

  3. Simo JC (1985) A finite strain beam formulation. The three-dimensional dynamic problem. Part I. Comput Methods Appl Mech Eng 49:55–70

    Article  MATH  Google Scholar 

  4. Cardona A, Géradin M (1988) A beam finite element non-linear theory with finite rotations. Int J Numer Methods Eng 26:2403–2438

    Article  MATH  Google Scholar 

  5. Romero I, Armero F (2002) An objective finite element approximation of the kinematics of geometrically exact rods and its use in the formulation of an energy-momentum conserving scheme in dynamics. Int J Numer Methods Eng 54:1683–1716

    Article  MathSciNet  MATH  Google Scholar 

  6. Armero F, Romero I (2001) On the formulation of high-frequency dissipative time-stepping algorithms for nonlinear dynamics. Part I: low-order methods for two model problems and nonlinear elastodynamics. Comput Methods Appl Mech Eng 190:2603–2649

    Article  MATH  Google Scholar 

  7. Armero F, Romero I (2001) On the formulation of high-frequency dissipative time-stepping algorithms for nonlinear dynamics. Part II: second-order methods. Comput Methods Appl Mech Eng 190:6783–6824

    Article  MATH  Google Scholar 

  8. Armero F, Romero I (2003) Energy-dissipative momentum-conserving time-stepping algorithms for the dynamics of nonlinear Cosserat rods. Comput Mech 31:3–26

    Article  MathSciNet  MATH  Google Scholar 

  9. Betsch P, Steinmann P (2003) Constrained dynamics of geometrically exact beams. Comput Mech 31:49–59

    Article  MATH  Google Scholar 

  10. Yu W, Liao L, Hodges DH, Volovoi VV (2005) Theory of initially twisted, composite, thin-walled beams. Thin-Walled Struct 43:1296–1311

    Article  Google Scholar 

  11. Mäkinen J (2007) Total lagrangian Reissner’s geometrically exact beam element without singularities. Int J Numer Methods Eng 70:1009–1048

    Article  MathSciNet  MATH  Google Scholar 

  12. Auricchio F, Carotenuto P, Reali A (2008) On the geometrically exact beam model: a consistent, effective and simple derivation from three-dimensional finite-elasticity. Int J Solids Struct 45:4766–4781

    Article  MATH  Google Scholar 

  13. Pimenta PM, Campello EM, Wriggers P (2008) An exact conserving algorithm for nonlinear dynamics with rotational dofs and general hyperelasticity. Part 1: rods. Comput Mech 42:715–732

    Article  MathSciNet  MATH  Google Scholar 

  14. Romero I (2008) A comparison of finite elements for nonlinear beams: the absolute nodal coordinate and geometrically exact formulations. Multibody Syst Dyn 20:51–68

    Article  MathSciNet  MATH  Google Scholar 

  15. Pai PF (2014) Problems in geometrically exact modeling of highly flexible beams. Thin-Walled Struct 76:65–76

    Article  Google Scholar 

  16. de Miranda S, Gutierrez A, Melchionda D, Patruno L (2015) Linearly elastic constitutive relations and consistency for GBT-based thin-walled beams. Thin-Walled Struct 92:55–64

    Article  Google Scholar 

  17. Sprague MA, Jonkman JM, Jonkman B (2015) FAST modular framework for wind turbine simulation: new algorithms and numerical examples. In: 33rd Wind energy symposium, AIAA SciTech Forum, American Institute of Aeronautics and Astronautics

  18. Wang Q, Sprague MA, Jonkman J, Johnson N, Jonkman B (2017) Beamdyn: a high-fidelity wind turbine blade solver in the fast modular framework. Wind Energy 20:1439–1462

    Article  Google Scholar 

  19. Dvorkin EN, Bathe K-J (1984) A continuum mechanics based four-node shell element for general non-linear analysis. Eng Comput 1:77–88

    Article  Google Scholar 

  20. Bathe K-J, Dvorkin EN (1986) A formulation of general shell elements—the use of mixed interpolation of tensorial components. Int J Numer Methods Eng 22:697–722

    Article  MATH  Google Scholar 

  21. Choi CK, Paik JG (1996) An effective four node degenerated shell element for geometrically nonlinear analysis. Thin-Walled Struct 24:261–283

    Article  Google Scholar 

  22. Betsch P, Stein E (1995) An assumed strain approach avoiding artificial thickness straining for a non-linear 4-node shell element. Commun Numer Methods Eng 11:899–909

    Article  MATH  Google Scholar 

  23. Betsch P, Stein E (1996) A nonlinear extensible 4-node shell element based on continuum theory and assumed strain interpolations. J Nonlinear Sci 6:169–199

    Article  MathSciNet  MATH  Google Scholar 

  24. Simo JC, Armero F (1992) Geometrically non-linear enhanced strain mixed methods and the method of incompatible modes. Int J Numer Methods Eng 33:1413–1449

    Article  MATH  Google Scholar 

  25. Bischoff M, Ramm E (1997) Shear deformable shell elements for large strains and rotations. Int J Numer Methods Eng 40:4427–4449

    Article  MATH  Google Scholar 

  26. Sansour C, Wriggers P, Sansour J (1997) Nonlinear dynamics of shells: theory, finite element formulation, and integration schemes. Nonlinear Dyn 13:279–305

    Article  MathSciNet  MATH  Google Scholar 

  27. Betsch P, Menzel A, Stein E (1998) On the parametrization of finite rotations in computational mechanics: a classification of concepts with application to smooth shells. Comput Methods Appl Mech Eng 155:273–305

    Article  MathSciNet  MATH  Google Scholar 

  28. Sansour C, Wagner W, Wriggers P, Sansour J (2002) An energy-momentum integration scheme and enhanced strain finite elements for the non-linear dynamics of shells. Int J Non-Linear Mech 37:951–966

    Article  MATH  Google Scholar 

  29. Romero I, Armero F (2002) Numerical integration of the stiff dynamics of geometrically exact shells: an energy-dissipative momentum-conserving scheme. Int J Numer Meth Eng 54:1043–1086

    Article  MathSciNet  MATH  Google Scholar 

  30. Bauchau OA, Choi J-Y, Bottasso CL (2002) On the modeling of shells in multibody dynamics. Multibody Syst Dyn 8:459–489

    Article  MATH  Google Scholar 

  31. Aksu Ozkul T (2004) A finite element formulation for dynamic analysis of shells of general shape by using the Wilson-\(\theta \) method. Thin-Walled Struct 42:497–513

    Article  Google Scholar 

  32. Betsch P, Sänger N (2009) On the use of geometrically exact shells in a conserving framework for flexible multibody dynamics. Comput Methods Appl Mech Eng 198:1609–1630

    Article  MathSciNet  MATH  Google Scholar 

  33. Vaziri A (2009) Mechanics of highly deformed elastic shells. Thin-Walled Struct 47:692–700

    Article  Google Scholar 

  34. Campello EM, Pimenta PM, Wriggers P (2011) An exact conserving algorithm for nonlinear dynamics with rotational dofs and general hyperelasticity. Part 2: shells. Comput Mech 48:195–211

    Article  MathSciNet  MATH  Google Scholar 

  35. Wu T-Y (2013) Dynamic nonlinear analysis of shell structures using a vector form intrinsic finite element. Eng Struct 56:2028–2040

    Article  Google Scholar 

  36. Ahmed A, Sluys LJ (2015) Implicit/explicit elastodynamics of isotropic and anisotropic plates and shells using a solid-like shell element. Eur J Mech A Solids 43:118–132

    Article  MathSciNet  MATH  Google Scholar 

  37. Pietraszkiewicz W, Konopińska V (2015) Junctions in shell structures: a review. Thin-Walled Struct 95:310–334

    Article  Google Scholar 

  38. Reinoso J, Blázquez A (2016) Application and finite element implementation of 7-parameter shell element for geometrically nonlinear analysis of layered CFRP composites. Compos Struct 139:263–276

    Article  Google Scholar 

  39. Caliri MF Jr, Ferreira AJM, Tita V (2016) A review on plate and shell theories for laminated and sandwich structures highlighting the finite element method. Compos Struct 156:63–77

    Article  Google Scholar 

  40. Ota NSN, Wilson L, Gay Neto A, Pellegrino S, Pimenta PM (2016) Nonlinear dynamic analysis of creased shells. Finite Elem Anal Des 121:64–74

    Article  MathSciNet  Google Scholar 

  41. Gebhardt CG, Rolfes R (2017) On the nonlinear dynamics of shell structures: combining a mixed finite element formulation and a robust integration scheme. Thin-Walled Struct 118:56–72

    Article  Google Scholar 

  42. Bucalem ML, Bathe K-J (2011) The mechanics of solids and structures—hierarchical modeling and the finite element solution. Springer, Berlin

    Book  MATH  Google Scholar 

  43. Eisenberg M, Guy R (1979) A proof of the hairy ball theorem. Am Math Monthly 86:571–574

    Article  MathSciNet  MATH  Google Scholar 

  44. Arnold VI (1989) Mathematical methods of classical mechanics. Springer, Berlin

    Book  Google Scholar 

  45. Heard WB (2006) Rigid body mechanics: mathematics, physics and applications. Wiley, Hoboken

    Google Scholar 

  46. Romero I (2001) Formulation and analysis of dissipative algorithms for nonlinear elastodynamics. Ph.D. thesis, University of California, Berkeley

  47. Gebhardt CG (2012) Desarrollo de simulaciones numéricas del comportamiento aeroelástico de grandes turbinas eólicas de eje horizontal. Ph.D. thesis, Universidad Nacional de Córdoba

  48. Betsch P, Steinmann P (2001) Frame-indifferent beam finite elements based upon the geometrically exact beam theory. Int J Numer Methods Eng 54:1775–1788

    Article  MathSciNet  MATH  Google Scholar 

  49. Gebhardt CG, Matusevich AE, Inaudi JA (2018) Coupled transverse and axial vibrations including warping effect in asymmetric short beams. J Eng Mech 144(6):04018043

    Article  Google Scholar 

  50. Gay Neto A (2017) Simulation of mechanisms modeled by geometrically-exact beams using rodrigues rotation parameters. Comput Mech 59:459–481

    Article  MathSciNet  Google Scholar 

  51. Ghosh S, Roy D (2009) A frame-invariant scheme for the geometrically exact beam using rotation vector parametrization. Comput Mech 44:103–118

    Article  MATH  Google Scholar 

  52. Romero I (2004) The interpolation of rotations and its application to finite element models of geometrically exact rods. Comput Mech 34:121–133

    Article  MathSciNet  MATH  Google Scholar 

  53. Simo JC, Rifai MS (1990) A class of mixed assumed strain methods and the method of incompatible modes. Int J Numer Methods Eng 29:1595–1638

    Article  MathSciNet  MATH  Google Scholar 

  54. Ko Y, Lee P-S, Bathe K-J (2017) A new mitc4+ shell element. Comput Struct 182:404–418

    Article  Google Scholar 

  55. Kane C, Marsden JE, Ortiz M (1999) Symplectic-energy-momentum preserving variational integrators. J Math Phys 40:3353–3371

    Article  MathSciNet  MATH  Google Scholar 

  56. Simo JC, Tarnow N (1994) A new energy and momentum conserving algorithm for the non-linear dynamics of shells. Int J Numer Methods Eng 37:2527–2549

    Article  MathSciNet  MATH  Google Scholar 

  57. Harten A, Lax B, Leer P (1983) On upstream differencing and Godunov-type schemes for hyperbolic conservation laws. SIAM Rev 25:35–61

    Article  MathSciNet  MATH  Google Scholar 

  58. McLachlan RI, Quispel GRW, Robideux N (1999) Geometric integration using discrete gradients. Philos Trans Math Phys Eng Sci 357:1021–1045

    Article  MathSciNet  MATH  Google Scholar 

  59. Gonzalez O (1996) Time integration and discrete Hamiltonian systems. J Nonlinear Sci 6:449–467

    Article  MathSciNet  MATH  Google Scholar 

  60. Romero I (2012) An analysis of the stress formula for energy-momentum methods in nonlinear elastodynamics. Comput Mech 50:603–610

    Article  MathSciNet  MATH  Google Scholar 

  61. Romero I (2008) Formulation and performance of variational integrators for rotating bodies. Comput Mech 42:825–836

    Article  MathSciNet  MATH  Google Scholar 

  62. Leyendecker S, Marsden J, Ortiz M (2008) Variational integrators for constrained dynamical systems. Zeitschrift für Angewandte Mathematik und Mechanik 88:677–708

    Article  MathSciNet  MATH  Google Scholar 

  63. Betsch P (2005) The discrete null space method for the energy consistent integration of constrained mechanical systems. Part i: holonomic constraints. Comput Methods Appl Mech Eng 194:5159–5190

    Article  MATH  Google Scholar 

  64. Betsch P, Leyendecker S (2006) The discrete null space method for the energy consistent integration of constrained mechanical systems. Part ii: multibody dynamics. Int J Numer Methods Eng 67:499–552

    Article  MATH  Google Scholar 

  65. Leyendecker S, Betsch P, Steinmann P (2008) The discrete null space method for the energy-consistent integration of constrained mechanical systems. Part iii: flexible multibody dynamics. Multibody Syst Dyn 19:45–72

    Article  MathSciNet  MATH  Google Scholar 

  66. Betsch P (2016) Structure-preserving integrators in nonlinear structural dynamics and flexible multibody dynamics. Springer, Berlin

    Book  MATH  Google Scholar 

  67. Klöppel T, Gee MW, Wall WA (2011) A scaled thickness conditioning for solid- and solid-shell discretizations of thin-walled structures. Comput Methods Appl Mech Eng 200:1301–1310

    Article  MathSciNet  MATH  Google Scholar 

  68. Simo JC, Tarnow N (1992) The discrete energy-momentum method. Conserving algorithms for nonlinear elastodynamics. Zeitschrift für Angewandte Mathematik und Physik 43:757–792

    Article  MathSciNet  MATH  Google Scholar 

  69. Romero I (2018) Coupling nonlinear beams and continua: Variational principles and finite element approximations. Int J Numer Methods Eng (in press)

  70. Wagner W, Gruttmann F (2002) Modeling of shell-beam transitions in the presence of finite rotations. Comput Assist Mech Eng Sci 9:4005–4018

    MATH  Google Scholar 

  71. Lang H, Linn J, Arnold M (2011) Multi-body dynamics simulation of geometrically exact Cosserat rods. Multibody Syst Dyn 25:285–312

    Article  MathSciNet  MATH  Google Scholar 

  72. Masud A, Tham CL, Liu WK (2000) A stabilized 3-D co-rotational formulation for geometrically nonlinear analysis of multi-layered composite shells. Comput Mech 26:1–12

    Article  MATH  Google Scholar 

  73. Kuhl D, Crisfield M (1999) Energy-conserving and decaying algorithms in non-linear structural dynamics. Int J Numer Methods Eng 45:569–599

    Article  MathSciNet  MATH  Google Scholar 

  74. Jonkman J, Butterfield S, Musial W, Scott G (2009) Definition of a 5-MW reference wind turbine for offshore system development. Technical report, National Renewable Energy Laboratory (NREL) Golden, CO

  75. Gebhardt CG, Preidikman S, Massa JC (2010) Numerical simulations of the aerodynamic behavior of large horizontal-axis wind turbines. Int J Hydrog Energy 35:6005–6011

    Article  Google Scholar 

  76. Gebhardt CG, Preidikman S, Jørgensen MH, Massa JC (2012) Non-linear aeroelastic behavior of large horizontal-axis wind turbines: a multibody system approach. Int J Hydrog Energy 37:14719–14724

    Article  Google Scholar 

  77. Gebhardt CG, Roccia BA (2014) Non-linear aeroelasticity: an approach to compute the response of three-blade large-scale horizontal-axis wind turbines. Renew Energy 66:495–514

    Article  Google Scholar 

  78. Häfele J, Hübler C, Gebhardt CG, Rolfes R (2016) An improved two-step soil-structure interaction modeling method for dynamical analyses of offshore wind turbines. Appl Ocean Res 55:141–150

    Article  Google Scholar 

  79. Hübler C, Häfele J, Gebhardt CG, Rolfes R (2018) Experimentally supported consideration of operating point dependent soil properties in coupled dynamics of offshore wind turbines. Mar Struct 57:18–37

    Article  Google Scholar 

  80. Hübler C, Gebhardt CG, Rolfes R (2017) Hierarchical four-step global sensitivity analysis of offshore wind turbines based on aeroelastic time domain simulations. Renew Energy 111:878–891

    Article  Google Scholar 

  81. Hübler C, Gebhardt CG, Rolfes R (2017) Development of a comprehensive data basis of scattering environmental conditions and simulation constraints for offshore wind turbines. Wind Energy Sci 2:491–505

    Article  Google Scholar 

  82. Häfele J, Hübler C, Gebhardt CG, Rolfes R (2018) A comprehensive fatigue load set reduction study for offshore wind turbines with jacket substructures. Renew Energy 118:99–112

    Article  Google Scholar 

  83. Intel Corporation (2015) Intel\(^{\textregistered }\) Math Kernel Library 11.3 Developer Reference. https://software.intel.com/en-us/mkl. Accessed Oct 2017

Download references

Acknowledgements

We greatly acknowledge the financial support of the Lower Saxony Ministry of Science and Culture (research Project ventus efficiens, FKZ ZN3024) and the German Federal Ministry for Economic Affairs and Energy (research Project Deutsche Forschungsplattform für Windenergie, FKZ 0325936E) that enabled this work. We also thank the reviewers for their valuable comments.

Author information

Authors and Affiliations

  1. Institute of Structural Analysis, Leibniz Universität Hannover and ForWind Hannover, Appelstr. 9A, 30167, Hannover, Germany

    Cristian Guillermo Gebhardt, Benedikt Hofmeister, Christian Hente & Raimund Rolfes

Authors
  1. Cristian Guillermo Gebhardt
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Benedikt Hofmeister
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Christian Hente
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Raimund Rolfes
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Cristian Guillermo Gebhardt.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendix: Summary: numerical implementation

Appendix: Summary: numerical implementation

To achive very competitive numerical performance, the adopted formulations ought to be generalized in regard to the implementation aspects, for example avoiding redundant code, storing the information to facilitate an optimized handling of data, minimize the amount of required operations, taking advantage of sparse structures, among others. At the same time reusability and extensibility of the produced code must be warranted. Satisfing several criteria at the same time can result very complicated. Therefore, we think that presenting our scheme for efficient numerical implementation to that kind of problems is as important as the formulation aspects already presented. In the scope of this section, we describe how our self-developed object-oriented finite element method, multibody system software, dubbed DeSiO (Design and Simulation Framework for Offshore Support Structures) was conceived.

1.1 A.1 Object-oriented programming

The presented ideas are implemented in object-oriented Fortran 2008 by following the design rules of object-oriented programming. Data belonging to the entities modeled in the program like structures and finite elements are confined to the respective Fortran modules. The control flow is designed in such a way that the solver module drives the time integration scheme. The “model” object is called to update force, stiffness and constraint data for every increment.

In Fig. 24, the object hierarchy and information about data and functionality is shown in brief. The following subsections give a detailed description of these entities.

1.1.1 A.1.1 Class “solver”

The “solver” class is responsible for step control, assembling and solving the system of equations for every iteration. After assembly of the iteration matrix and the residual vector, the linear system of equations is solved. According to the Newton–Raphson iteration scheme, the nodal positions and velocities, elemental strains and Lagrange’s multipliers are updated. This also encompasses check of convergence by a numerical tolerance criterion and code to abort the simulation in case of divergence.

Fig. 24
figure 24

Object hierarchy and functionality

Full size image

Since the structure of the iteration matrix is invariant to the geometrical configuration and loading, reordering and memory organization can be done once before the actual finite element calculation. Nonzero elements are identified by running the iteration matrix assembly using specially conditioned input data for all variables of the formulation that numerically change the iteration matrix:

  • Node positions at the beginning of the time step

  • Node positions at the current time

  • Material loads

  • Lagrange multipliers

Parameter coefficients of the employed material law like density and moduli are invariant during the simulation, so zero entries in the iteration matrix arising for example from material symmetries are not considered for the solution.

Considering the “three intersecting plates” problem [56], for a finite element mesh containing 7896 degrees of freedom, the iteration matrix has a fraction of \(99.54\%\) zero elements. Figure 25 displays the structure of the iteration matrix in this case.

Generally, the fraction of zero elements increases with degrees of freedom, which means that the speed advantage gained by employing a sparse solver is pronounced in problems with large numbers of elements. The present code solves the system of equations using the PARDISO [83] parallel sparse solver code.

1.1.2 A.1.2 Class “model”

The “model” class contains every entity in the mechanical system considered in the simulation. When the code starts, it calls the constructor of the “model” class which will in turn open simulation input files. The input files are parsed and instances of structure modules are created according to the information provided. Also constraint input is processed and constraint objects are created accordingly.

During the simulation, the model class is called to assemble the iteration matrix by passing the respective row and column coordinates of the iteration matrix to the simulation entities.

1.1.3 A.1.3 Class “structure”

The “structure” class is a master class to provide an external force calculation routine and assembly handling for entities in the mechanical system considered in the simulation. The external force calculation is placed outside of the element formulation to prevent redundant code which would arise, if this was done in the actual beam and shell classes. Since this simulation framework is intended to be mostly used in context of fluid-structure interactions with non-matching grids (flow field computed with boundary elements), information is tranferred using an extended version of the approach exposed in [77], which warrants the primary consistency condition (equivalency at the level of the virtual work) during the data trasnferring for loads located at fixed isoparametric coordinates and therefore, the calculation can be performed outside the elemental scope. This assumption could result very restrictive for other situations, in which requierements on invariant preservation along the data transefernce from one field to another field are not requiered. However, a broad exposure of this topic is outside the reach of this manuscript.

Fig. 25
figure 25

Skyline plot of the iteration matrix for the “three intersecting plates” problem [41, 56]

Full size image

1.1.4 A.1.4 Class “rigid body”, “beam”, and “shell”

The “rigid body” class provides a means to simulate concentrated masses and inertias. During the simulation, stiffness matrices corresponding to the inertia of the rigid body are provided for assembly into the iteration matrix.

The “beam” class contains the actual finite elements and element connectivity. During the update for every iteration, the update function for stiffness and internal forces for each element is called. Updated element stiffness and internal forces are then assembled according to the element connectivity stored in the “beam” class.

The “shell” class has the same functionality as the “beam” class. Additional complexity arises from the fact that for quadrilateral elements more connected neighboring elements have to be considered during assembly than during beam assembly and the existence of elemental degree of freedom due to the enhanced assumed strains (these are not condensed).

1.1.5 A.1.5 Class “beam element” and “shell element”

The “element” classes provide the actual mathematical implementation of the finite element as proposed in sections 2.2 and 2.3. Due to the object-oriented implementation, each finite element is an instance of the corresponding Fortran module. This makes passing stiffness and mass information easy by making member variables of the class accessible from the outside. After an update to the internal terms of the element and hence the update of the member variables, all subsequent data queries to the element will provide internal terms for the latest time step.

In every iteration the stiffness and internal forces are updated using the current configuration in generalized coordinates and velocities.

1.1.6 A.1.6 Class “constraint”

The “constraint” class is a master class which is inherited to create a specific kinematic constraint of the type described in Sect. 4. It provides the block vector \({\varvec{h}}\) and block matrix \({\varvec{H}}\) for the coupling terms between Lagrange’s multipliers and generalized coordinates. A method is provided for the Lagrange multiplier update based on the configuration at the current iteration. To enable internal constraints and certain types of joint connections, optionally a stiffness block matrix contribution can be provided.

As discussed in Sect. 4, there are three classes of constraint to couple the “Node 6” and “Node 12” node types: “constraint6”, “constraint12” and “constraint6to12”.

1.1.7 A.1.7 Class “node”

For both “Node 6” and “Node 12”, a separate class exists in the code. These classes are derived from the “node” class and provide the routine to calculate external nodal loads. Loads which should follow the structure, also called material loads, further provide a stiffness block matrix contribution.

1.2 A.2 Pre- and post-processing

1.2.1 A.2.1 Pre-processing

Since geometry definition and meshing routines are outside the scope of the finite element kernel, pre-processing is done in external software. Import routines are written in the language Python to read node and element list formats from various commercial finite element solvers and convert geometry and material definitions into the simple format used by the present finite element code. Import of constraints, forces and boundary conditions is semi-automated, since in some cases, the present formulation needs extra information regarding the director-based approach.

1.2.2 A.2.2 Post-processing

For graphical post-processing, a script was developed which enables interactive video display of the transient simulation results. Since commercially available finite element solvers do not implement the concept of directors in the formulation, there is no simple way to convert analysis output to formats used by established post-processors. The in-house visualization tool thus enables a quick check for model sanity by making directors and mesh visible.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Gebhardt, C.G., Hofmeister, B., Hente, C. et al. Nonlinear dynamics of slender structures: a new object-oriented framework. Comput Mech 63, 219–252 (2019). https://doi.org/10.1007/s00466-018-1592-7

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00466-018-1592-7

Keywords

Search

Navigation