Single-compartment model of a pyramidal neuron, fitted to recordings with current and conductance injection

Abstract

For single neuron models, reproducing characteristics of neuronal activity such as the firing rate, amplitude of spikes, and threshold potentials as functions of both synaptic current and conductance is a challenging task. In the present work, we measure these characteristics of regular spiking cortical neurons using the dynamic patch-clamp technique, compare the data with predictions from the standard Hodgkin-Huxley and Izhikevich models, and propose a relatively simple five-dimensional dynamical system model, based on threshold criteria. The model contains a single sodium channel with slow inactivation, fast activation and moderate deactivation, as well as, two fast repolarizing and slow shunting potassium channels. The model quantitatively reproduces characteristics of steady-state activity that are typical for a cortical pyramidal neuron, namely firing rate not exceeding 30 Hz; critical values of the stimulating current and conductance which induce the depolarization block not exceeding 80 mV and 3, respectively (both values are scaled by the resting input conductance); extremum of hyperpolarization close to the midpoint between spikes. The analysis of the model reveals that the spiking regime appears through a saddle-node-on-invariant-circle bifurcation, and the depolarization block is reached through a saddle-node bifurcation of cycles. The model can be used for realistic network simulations, and it can also be implemented within the so-called mean-field, refractory density framework.

Appendices

Appendix

Experimental technique

2.1 The whole-cell patch-clamp recordings in rat brain slices

Animal handling and experimentation were performed in accordance with European Community Council directives 86/609/EEC. Male Wistar albino rats were used for the experiments (N = 4, 18–21 days postnatal). Rats were sacrificed by decapitation, each brain was rapidly removed and immersed in ice-cold, preoxygenated (95% O2 and 5% CO2) artificial cerebrospinal fluid (ACSF) of the following composition (in mM): 126 NaCl, 2.5 KCl, 1.25 Na2HPO4, 24 NaHCO3, 2 CaCl2, 1 MgSO4, 10 D-glucose; pH 7.3–7.4. Coronal slices (300 μm thick) of medial frontal (prelimbic) cortex were cut with a vibratome (Microm HM 650 V; Microm, Walldorf, Germany). Slices were incubated at room temperature for at least 1 h before recordings. Neurons were visualized using transmitted illumination on a fixed-stage upright Axioscop 2 microscope (Zeiss, Oberkochen, Germany) equipped with differential interference contrast optics and a video camera (Grasshopper 3 GS3-U3-23S6M-C; FLIR Integrated Imaging Solutions Inc., Wilsonville, OR, USA). Pyramidal neurons were identified by their apical dendrites and triangular somata. Whole-cell recordings were made from layer 3 pyramidal neurons using Model 2400 (AM-Systems; Sequim, WA, USA) patch-clamp amplifier. Patch electrodes (3–5 MΩ) were pulled from borosilicate capillary glass (Sutter Instrument, Novato, CA, USA). The following internal solution was used (in mM): 136 K-gluconate, 10 NaCl, 5 EGTA, 10 HEPES, 4 ATP-Mg, 0.3 GTP, pH 7.25 (adjusted with KOH).

2.2 Acquisition and dynamic-clamp

Signals were digitized with a sampling frequency of 33 kHz for acquisition with NI DAQ PCI-6221-37pin (National Instruments, Austin, TX) and filtered with 5 kHz by the amplifier. Whole-cell recordings were done in the dynamic-clamp mode to introduce additional leaky channel. The custom software “DC-project” was used.¹ The applied current is calculated as $u(t)-s(t)(V(t)-{V}_{US})$, where $u(t)$ and $s(t)$ are the voltage-independent input signals, current and conductance, respectively; $V(t)$ is the membrane voltage and ${V}_{US}$ is the reference voltage which was set to -60 mV, close to the resting membrane potential ${V}_{\mathrm{rest}}$. This reference voltage level is not essential, because its shift is equivalent to the shift of u. We included it for analysis only cells with stable input conductance and membrane potential, and with high access conductance. The access resistance was compensated in real time. For every neuron the recordings were started from estimations of the resting membrane potential ${V}_{\mathrm{rest}}$, input conductance ${G}_{L}$, and the membrane time constant ${\tau }_{m}$. Intrinsic membrane properties were assessed from the voltage responses to the series of 500-ms current steps providing hyperpolarization up to 5–10 mV.

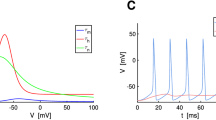
To estimate the f-u-s-function in the whole domain of u and s that provide spike generation, a series of recordings were performed with the injected steps of input signal calculated for different values of current $u$ and conductance $s$ and lasting for 0.5 s with the frequency 0.5 Hz. The increments of u and s were constant, the typical grid was 25 by 12 for u and s, respectively. For each step of stimulation, the firing rate ν was calculated as a total number of spikes per the last 2/3 of step duration. The values of u and s in the plots were scaled by the input conductance ${G}_{L}$, in order to compare Ω-domains for different neurons, following (Graham and Schramm 2009).

The threshold was measured at the point where $\mathrm{d}V/\mathrm{d}t$ reaches $5 \mathrm{mV}/\mathrm{ms}$ or ${\mathrm{d}}^{2}V/\mathrm{d}{t}^{2}$ reaches $2 \mathrm{mV}/{\mathrm{ms}}^{2}$. The spike half-width was measured for each spike at the half-height defined as the middle voltage level between the threshold and the peak, and averaged across all spikes of a trace. The post-spike hyperpolarization potential (PHP) was measured as a minimum value between spikes, and averages across spikes. The average membrane potential was calculated for each trace on interspike intervals since the time moment of the peak plus 2 ms to the time of the next crossing of threshold.

Mathematical models

3.1 Hodgkin–Huxley model

We considered the classical one-compartmental Hodgkin-Huxley model, described by the following equations (Hodgkin and Huxley 1952):

$$C\frac{\mathrm{d}U}{\mathrm{d}t}=-{g}_{L}\left(U-{V}_{L}\right)-{\overline{g}}_{Na} {m}^{3} h \left(U-{V}_{Na}\right)-{\overline{g}}_{K} {n}^{4}\left(U-{V}_{K}\right)+u\left(t\right)-s\left(t\right)\left(U-{V}_{us}\right)$$

$$\frac{\mathrm{d}m}{\mathrm{d}t}=\frac{{m}_{\infty }-m}{{\tau }_{m}}, \frac{\mathrm{d}h}{\mathrm{d}t}=\frac{{h}_{\infty }-h}{{\tau }_{h}}, \frac{dn}{\mathrm{d}t}=\frac{{n}_{\infty }-n}{{\tau }_{n}},$$

$${\tau }_{m}=\frac{1}{{\alpha }_{m}+{\beta }_{m}}, {\tau }_{h}=\frac{1}{{\alpha }_{h}+{\beta }_{h}}, {\tau }_{n}=\frac{1}{{\alpha }_{n}+{\beta }_{n}},$$

$${m}_{\infty }=\frac{{\alpha }_{m}}{{\alpha }_{m}+{\beta }_{m}},{ h}_{\infty }=\frac{{\alpha }_{h}}{{\alpha }_{h}+{\beta }_{h}}, {n}_{\infty }=\frac{{\alpha }_{n}}{{\alpha }_{n}+{\beta }_{n}},$$

$${\alpha }_{m}=\frac{(U+35)/10}{1-\mathrm{exp}\left(-(U+35)/10\right)}, {\beta }_{m}=4\mathrm{exp}\left(-\frac{U+60}{18}\right),$$

$${\alpha }_{h}=0.07 \mathrm{exp}\left(-\frac{U+60}{20}\right), {\beta }_{m}=\frac{1}{1+\mathrm{exp}(-(U+30)/10)},$$

$${\alpha }_{n}=\frac{(U+50)/100}{1-\mathrm{exp}(-(U+50)/10)}, {\beta }_{m}=0.125\mathrm{exp}\left(-\frac{U+60}{80}\right)$$

where $U(t)$ is the membrane potential, $C$ is the capacitance, ${V}_{L}, {V}_{\mathrm{Na}}$ and ${V}_{K}$ are the leak, sodium and potassium reversal potentials, $m(U,t)$ is the sodium channel activation, $h(U,t)$ is the sodium channel activation, $n(U,t)$ is the potessium channel activation, ${g}_{L}$ is the leak conductance, ${\overline{g}}_{\mathrm{Na}}$ and ${\overline{g}}_{K}$ are the sodium and potassium channel maximum conductances.

$$C=1\frac{\mu F}{{\mathrm{cm}}^{2}}, {g}_{L}=0.3\frac{\mathrm{mS}}{{\mathrm{cm}}^{2}}, {\overline{g}}_{\mathrm{Na}}=120\frac{\mathrm{mS}}{{\mathrm{cm}}^{2}},\hspace{1em}{\overline{g}}_{K}=36\frac{\mathrm{mS}}{{\mathrm{cm}}^{2}}$$

$$S=1.4\cdot {10}^{-5} {\mathrm{cm}}^{2},\left({G}_{L}={g}_{\mathrm{tot}}^{0} S=5 \,\mathrm{nS}\right),$$

$$\begin{aligned} &V_{{{\text{rest}}}} = - 65\,{\text{mV}},\left( {V_{{\text{L}}} = - 63\,{\text{mV}}} \right),\\ & V_{{{\text{us}}}} = - \,60\,{\text{mV}},V_{{\text{K}}} = - 72\,{\text{mV}},V_{{{\text{Na}}}} = 55\,mV \end{aligned}$$

Here, in comparison with standard parameterization, the leak reversal potential ${V}_{L}$ was modified to provide the desired resting potential ${V}_{\mathrm{rest}}$, and the membrane area was set such that the input conductance at rest, ${G}_{L}$, would be equal to 5nS as in the other model.

3.2 Izhikevich model

The simple model of a regular spiking cell was used according to (Izhikevich 2003), where to we introduced the input terms $u\left(t\right)-s\left(t\right)\left(U-{V}_{us}\right)$:

$$C \frac{\mathrm{d}U}{\mathrm{d}t}=k(U-{V}_{r})(U-{V}_{t})-w+u\left(t\right)-s\left(t\right)\left(U-{V}_{us}\right)$$

$$\frac{\mathrm{d}w}{\mathrm{d}t}=a \left(b (U-{V}_{r})-w\right)$$

with the after-spike resetting.

if $U\ge 30\, \mathrm{mV}$, then $U=c$, $w=w+d$.

The parameters were normalized so that the neuron has an input conductance of 5nS, namely: $C=\left(100*\frac{5}{35}\right)\, \mathrm{pF}$, $k=\left(3*\frac{5}{35}\right)\,\mathrm{nS}/\mathrm{mV}$, ${V}_{r}=-60 \,\mathrm{mV}$, ${V}_{t}=-50\, \mathrm{mV}$, $a=\left(0.01*\frac{35}{5}\right) \,{\mathrm{ms}}^{-1}$, $b=\left(5*\frac{5}{35}\right)\,\mathrm{nS}$, $c=-60 \,\mathrm{mV}$, $d=400\, \mathrm{pA}$.

3.3 Minimal Hodgkin–Huxley-like model for rodent’s cortical regular spiking neuron

The model is taken from (Pospischil et al. 2008). A slow potassium channel with the activalion variable $p(U,t)$ was added to the original Hodgkin–Huxley model:

$$C\frac{\mathrm{d}U}{\mathrm{d}t}=-{g}_{L}\left(U-{V}_{L}\right)-{\overline{g}}_{\mathrm{Na}} {m}^{3} h \left(U-{V}_{Na}\right)-{\overline{g}}_{K} {n}^{4}\left(U-{V}_{K}\right)-{\overline{g}}_{M}p(U-{V}_{K})+u\left(t\right)-s\left(t\right)\left(U-{V}_{us}\right)$$

$$ \begin{aligned} \frac{{{\text{d}}m}}{{{\text{d}}t}} = & \frac{{m_{\infty } - m}}{{\tau_{m} }}, \frac{{{\text{d}}h}}{{{\text{d}}t}} = \frac{{h_{\infty } - h}}{{\tau_{h} }}, \\ \frac{{{\text{d}}n}}{{{\text{d}}t}} =& \frac{{n_{\infty } - n}}{{\tau_{n} }}, \frac{{{\text{d}}p}}{{{\text{d}}t}} = \frac{{p_{\infty } - p}}{{\tau_{p} }}, \\ \tau_{m} = & \frac{1}{{\alpha_{m} + \beta_{m} }}, \tau_{h} = \frac{1}{{\alpha_{h} + \beta_{h} }}, \tau_{n} = \frac{1}{{\alpha_{n} + \beta_{n} }}, \\ \end{aligned} $$

$${\tau }_{m}=\frac{1}{{\alpha }_{m}+{\beta }_{m}}, {\tau }_{h}=\frac{1}{{\alpha }_{h}+{\beta }_{h}}, {\tau }_{n}=\frac{1}{{\alpha }_{n}+{\beta }_{n}},$$

$${m}_{\infty }=\frac{{\alpha }_{m}}{{\alpha }_{m}+{\beta }_{m}},{ h}_{\infty }=\frac{{\alpha }_{h}}{{\alpha }_{h}+{\beta }_{h}}, {n}_{\infty }=\frac{{\alpha }_{n}}{{\alpha }_{n}+{\beta }_{n}},$$

$$\begin{aligned} {\alpha }_{m} & =\frac{0.32(U-{V}_{Tr}-13)}{1-\mathrm{exp}\left(-(U-{V}_{Tr}-13)/4\right)}, \\ {\beta }_{m} & =\frac{-0.28(U-{V}_{Tr}-40)}{1-\mathrm{exp}\left(-(U-{V}_{Tr}-40)/5\right)},\end{aligned}$$

$$\begin{aligned} {\alpha }_{h} & =0.128 \mathrm{exp}\left(-\frac{U-{V}_{Tr}-17}{18}\right), \\ {\beta }_{m} & =\frac{4}{1+\mathrm{exp}(-(U-{V}_{Tr}-40)/5)}, \end{aligned} $$

$$\begin{aligned} {\alpha }_{n} & =\frac{0.032(U-{V}_{Tr}-15)}{1-\mathrm{exp}(-(U-{V}_{Tr}-15)/5)}, \\ {\beta }_{m} & =0.5\mathrm{exp}\left(-\frac{U-{V}_{Tr}-10}{40}\right),\end{aligned}$$

$${p}_{\infty }=\frac{1}{1+\mathrm{exp}(-(U+35)/10)}, {\tau }_{p}=\frac{{\tau }_{\mathrm{max}} }{3.3 \mathrm{exp}((U+35)/20)+\mathrm{exp}(-(U+35)/20)},$$

$$C=1\frac{\mu F}{{\mathrm{cm}}^{2}}, {g}_{L}=0.1\frac{\mathrm{mS}}{{\mathrm{cm}}^{2}}, {\overline{g}}_{\mathrm{Na}}=50\frac{\mathrm{mS}}{{\mathrm{cm}}^{2}},\hspace{1em}{\overline{g}}_{K}=5\frac{\mathrm{mS}}{{\mathrm{cm}}^{2}},\hspace{1em}{\overline{g}}_{M}=0.07\frac{\mathrm{mS}}{{\mathrm{cm}}^{2}}, S=29\cdot {10}^{-5}{\mathrm{cm}}^{2}, {V}_{L}=-70\hspace{0.33em}\mathrm{mV}, {V}_{us}=-60\hspace{0.33em}mV, {V}_{K}=-90\hspace{0.33em}\mathrm{mV}, {V}_{\mathrm{Na}}=50\hspace{0.33em}\mathrm{mV}, {V}_{Tr}=-60\hspace{0.33em}\mathrm{mV}, {\tau }_{\mathrm{max}}=1\hspace{0.33em}s.$$

3.4 The proposed hybrid model

The proposed model contains three channels: one sodium channel ${I}_{\mathrm{Na}}$ and two potassium channels, one fast ${I}_{K,f}$ and one slow ${I}_{K,s}$. The voltage equation hence reads:

$$C\frac{\mathrm{d}U}{\mathrm{d}t}=-{g}_{L}\left(U-{V}_{L}\right)-{I}_{Na}-{I}_{K,f}-{I}_{K,s}+u\left(t\right)-s\left(t\right)\left(U-{V}_{us}\right)$$

(1)

The sodium current is defined by:

$${I}_{Na}\left(U,t\right)={\overline{g}}_{\mathrm{Na}} {m}^{2}\left(U,t\right) i\left(U,t\right) \left(U\left(t\right)-{V}_{\mathrm{Na}}\right),$$

(2)

$$\mathrm{if }\left({U\left(t\right)>V}^{T}\left(t\right)\right)\, \mathrm{and} \,\left(h>0.5\right)\,\mathrm{ then }\,m=1, h=0$$

(3)

$${V}^{T}\left(t\right)=-51+{\left(({i}_{\infty }^{-1}\left(i(t)\right)+60)/5\right)}^{2} \mathrm{mV}$$

(4)

$$\frac{\mathrm{d}m}{\mathrm{d}t}=\frac{{m}_{\infty }-m}{{\tau }_{mm}}$$

(5)

$$\frac{\mathrm{d}h}{\mathrm{d}t}=\frac{{h}_{\infty }-h}{{\tau }_{h}}$$

(6)

$$\frac{\mathrm{d}i}{\mathrm{d}t}=\frac{{i}_{\infty }(U)-i}{{\tau }_{i}(U)}$$

(7)

$${\tau }_{mm}=7\,\mathrm{ ms}, {\tau }_{h}=10\,\mathrm{ ms},{\tau }_{i}=40\,\mathrm{ ms},{m}_{\infty }=0,{h}_{\infty }=1,$$

${i}_{\infty }=1/(1+\mathrm{exp} ((U+44)/4)),$ Hence ${i}_{\infty }^{-1}\left(x\right)=-44+4\, \mathrm{ln}\left(1/x-1\right)$

The fast voltage-dependent potassium current ${I}_{K,f}$ is defined by:

$${I}_{K,f}\left(U,t\right)={\overline{g}}_{K,f} n\left(U,t\right)\left(U\left(t\right)-{V}_{K}\right),$$

(8)

$$\frac{\mathrm{d}n}{\mathrm{d}t}=\frac{{n}_{\infty }(U)-n}{{\tau }_{n}(U)},$$

(9)

$${\tau }_{n}=\frac{1}{a+b}+2\, \mathrm{ms}; {n}_{\infty }=a/(a+b),$$

$$\begin{aligned} & a=0.1\,\mathrm{exp} ((U+25)/7)\, {\mathrm{ms}}^{-1}, \\ & b=0.1\,\mathrm{exp} (-(U+25)/7)\, {\mathrm{ms}}^{-1} \end{aligned}$$

The slow voltage-dependent potassium current ${I}_{K,s}$ is defined by:

$${I}_{K,s}\left(U,t\right)={\overline{g}}_{K,s} w\left(U,t\right) \left(U\left(t\right)-{V}_{K}\right),$$

(10)

$$\frac{\mathrm{d}w}{\mathrm{d}t}=\frac{{w}_{\infty }\left(U\right)-w}{{\tau }_{w}\left(U\right)},$$

(11)

$${\tau }_{w}=\frac{1}{a+b}+4 \mathrm{ms},{w}_{\infty }=a/(a+b),$$

$$a=5\mathrm{exp}\left(\frac{U}{4}\right)\,{\mathrm{ms}}^{-1}, b=0.05\, {\mathrm{ms}}^{-1}$$

Parameters values are given by:

$$C=0.7\,\frac{\mu F}{{\mathrm{cm}}^{2}}, {\tau }_{m}=\frac{C}{{g}_{\mathrm{tot}}^{0}}=14.4\, \mathrm{ms}, \left({g}_{L}=0.048\,\frac{\mu S}{{\mathrm{cm}}^{2}}\right),$$

$$S=1{0}^{-4} \,{\mathrm{cm}}^{2},{(G}_{L}={g}_{\mathrm{tot}}^{0} S=5\, \mathrm{nS}),$$

$$ V_{{{\text{rest}}}} = - 65\,{\text{mV}},\left( {V_{L} = - 65\,{\text{mV}}} \right),V_{{us}} = - 60\,{\text{mV}},V_{K} = - 80\,{\text{mV}},V_{{{\text{Na}}}} = 55\,{\text{mV}}, $$

$${\overline{g}}_{\mathrm{Na}}=2\,\frac{\mu S}{{\mathrm{cm}}^{2}},\hspace{1em}{\overline{g}}_{K,f}=2\,\frac{\mu S}{{\mathrm{cm}}^{2}}, {\overline{g}}_{K,s}=0.5\,\frac{\mu S}{{\mathrm{cm}}^{2}}.$$

For a fast-spiking interneuron, not considered in the present paper, we modified two parameters: ${\overline{g}}_{K,s}=0.1\frac{\mu S}{{\mathrm{cm}}^{2}}$ and ${\tau }_{mm}=3 \mathrm{ms}$, which increases the gain of the f-I curve, increases the maximum firing rate and approaches the DB limit.

3.5 The proposed continuous model

A continuous version of the sodium channel approximation is obtained from the system of Eqs. (1–11) by omitting the threshold condition, Eq. (3), and introducing in Eqs. (5, 6) the variable time constants and steady-state functions in the following form:

$${\tau }_{\mathrm{mm}}=0.1\, \mathrm{ms}+7 \,\mathrm{ms} (1-{S}_{V}{S}_{h}),$$

$${\tau }_{h}=0.1 \,\mathrm{ms}+10\, \mathrm{ms} (1-{S}_{V}{S}_{m}),$$

$${m}_{\infty }={S}_{V}{S}_{h},$$

$${h}_{\infty }={(1-S}_{V}{S}_{m}) {i}_{\infty }(U(t)),$$

$${S}_{V}=\frac{1}{\left(1+\mathrm{exp}\left(-\frac{\left({U\left(t\right)-V}^{T}\left(t\right)\right)}{0.1\mathrm{ mV}}\right)\right)},$$

$${S}_{h}=\frac{1}{\left(1+\mathrm{exp}\left(-\frac{\left(h\left(t\right)-0.5\right)}{0.01}\right)\right)},$$

$${S}_{m}=\frac{1}{\left(1+\mathrm{exp}\left(-\frac{\left(m\left(t\right)-0.8\right)}{0.01}\right)\right)}.$$

3.5.1 Statistical analysis

For each spike, the threshold potential in the model was measured at 1.2 ms before the peak of the spike. The spike half-width was measured for each spike at the voltage level − 20 mV, and averaged across all spikes of a trace. The post-spike hyperpolarization potential (PHP) was measured as a minimum value between spikes, and averages across spikes. The average membrane potential was calculated for each trace on interspike intervals since the time moment of the peak plus 2 ms to the point where $\mathrm{d}V/\mathrm{d}t$ reaches $5 \mathrm{mV}/\mathrm{ms}$.