Unimodularity and Preservation of Volumes in Nonholonomic Mechanics

Abstract

The equations of motion of a mechanical system subjected to nonholonomic linear constraints can be formulated in terms of a linear almost Poisson structure in a vector bundle. We study the existence of invariant measures for the system in terms of the unimodularity of this structure. In the presence of symmetries, our approach allows us to give necessary and sufficient conditions for the existence of an invariant volume, which unify and improve results existing in the literature. We present an algorithm to study the existence of a smooth invariant volume for nonholonomic mechanical systems with symmetry and we apply it to several concrete mechanical examples.

Appendix: Volume Forms on Vector Bundles

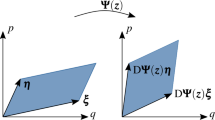
Let $\tau : E \rightarrow Q$ be an orientable vector bundle, over an orientable manifold $Q$. If $\alpha$ is a section of $\tau : E \rightarrow Q$, we can define its vertical lift $\alpha ^\mathbf{v}$, which is the vector field on $E$ given by

$$\begin{aligned} \alpha ^\mathbf{v}(\gamma _{q}) = \frac{d}{dt}_{|t=0}(\gamma _{q} + t\alpha (q)), \text{ for } \gamma _{q} \in E_q. \end{aligned}$$

If $\{e^{\beta }\}$ is a local basis of sections of $E$ and $\alpha = \alpha _{\beta }e^{\beta }$ then

$$\begin{aligned} \alpha ^\mathbf{v} = \alpha _{\beta }\frac{\partial }{\partial p_{\beta }}, \end{aligned}$$

(7.1)

where $p_{\beta }$ are the coordinates on the fibers of $E$ obtained using the basis $\{e^{\beta }\}$.

Lemma 7.1

Marrero (2010) Let $\nu$ be a volume form on $Q$ and $\Omega$ be a volume form on the fibers of $E^*$. Then, there exists a unique volume form $\nu \wedge \Omega$ on $E$ such that

$$\begin{aligned} \nu \wedge \Omega (\tilde{Z}_{1}, \dots , \tilde{Z}_{m}, \alpha _{1}^\mathbf{v}, \dots , \alpha _{n}^\mathbf{v}) = \nu (Z_{1}, \dots , Z_{m}) \Omega (\alpha _{1}, \dots , \alpha _{n}), \end{aligned}$$

(7.2)

for $\alpha _{1}, \dots , \alpha _{n} \in \Gamma (\tau )$ and $\tilde{Z}_{1}, \dots , \tilde{Z}_{m}$ vector fields on $E$ which are $\tau$-projectable on the vector fields $Z_{1}, \dots , Z_{m}$ on $Q$.

Locally, if $(q^i)$ are local coordinates on an open subset $U \subseteq Q$ and $\{e_{\alpha }\}$ is a basis of sections of $E^*$ such that

$$\begin{aligned} \nu = dq^1 \wedge \dots \wedge dq^m, \text{[ }.75cm]{} \Omega = e_{1} \wedge \dots \wedge e_{n}, \end{aligned}$$

then

$$\begin{aligned} \nu \wedge \Omega = dq^1 \wedge \dots \wedge dq^m \wedge dp_{1} \wedge \dots \wedge dp_{n}. \end{aligned}$$

(7.3)

A volume form $\Phi$ on $E$ is said to be of basic type if

$$\begin{aligned} {\mathcal L}_{\alpha ^\mathbf{v}}\Phi = 0, \; \; \; \forall \alpha \in \Gamma (\tau ). \end{aligned}$$

(7.4)

Using (7.3), it is easy to prove that the volume form $\nu \wedge \Omega$ is of basic type. In fact, we have the following result:

Proposition 7.2

A volume form $\Phi$ on $E$ is of basic type if and only if there exists a volume form $\nu$ on $Q$ and a volume form $\Omega$ on the fibers of $E^*$ such that

$$\begin{aligned} \Phi = \nu \wedge \Omega . \end{aligned}$$

Proof

Suppose that $\Phi$ is a volume form on $E$ of basic type.

Let $\nu _0$ be an arbitrary volume form on $Q$ and $\Omega _0$ a volume form on the fibers of $E^*$. Then we can assume, without the loss of generality, that

$$\begin{aligned} \Phi = e^{\tilde{\sigma }} \nu _{0} \wedge \Omega _0, \; \; \; \text{ with } \tilde{\sigma } \in C^{\infty }(E). \end{aligned}$$

Now, using (7.4), it follows that

$$\begin{aligned} d\tilde{\sigma }(\gamma ^\mathbf{v}) = 0, \; \; \; \forall \gamma \in \Gamma (\tau ), \end{aligned}$$

which implies that $\tilde{\sigma }$ is a basic function with respect to the vector bundle projection $\tau : E \rightarrow Q$. In other words, there exists $\sigma \in C^{\infty }(Q)$ such that $\tilde{\sigma } = \sigma \circ \tau$.

Thus, if we take

$$\begin{aligned} \nu = e^{\sigma } \nu _0, \; \; \; \Omega = \Omega _0, \end{aligned}$$

we have that $\Phi = \nu \wedge \Omega$. $\square$