Abstract
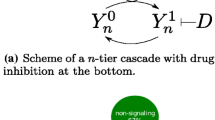
A modified Goldbeter–Koshland (GK) type model is proposed to study the open signaling cascade with forward activation. Three different regimes related to the ultrasensitivity are clearly defined. When the cascade is sufficiently long, the steady states in the downstream cycles exhibit a limiting behavior. Meanwhile, a temporal switch-like behavior is revealed in the pre-ultrasensitivity regime without any feedback circuitry. A detailed analysis of this phenomenon is provided.
Similar content being viewed by others
References
Alberts B, Johnson A, Lewis J, Raff M, Roberts K et al (2001) Molecular biology of the cell, 4th edn. Garland Science
Behar M, Hao N, Dohlman H, Elston T (2007) Mathematical and computational analysis of adaptation via feedback inhibition in signal transduction pathways. Biophys J 93(3): 806–821
Binder B, Heinrich R (2004) Interrelations between dynamical properties and structural characteristics of signal transduction networks. Genome Inform 15(1): 13–23
Bowden AC (1995) Fundamentals of enzyme kinetics. Revised edn. Portland Press, London
Chaves M, Sontag ED, Dinerstein RJ (2004) Optimal length and signal amplification in weakly activated signal transduction cascades. J Phys Chem B 108(39): 15311–15320
Criswell T, Beman M, Araki S, Leskov K, Cataldo E, Mayo L, Boothman D (2005) Delayed activation of insulin-like growth factor-1 receptor/Src/MAPK/Egr-1 signaling regulates clusterin expression, a pro-survival factor. J Biol Chem 280(14): 14212–14221
Goldbeter A (1991) A minimal cascade model for the mitotic oscillator involving cyclin and cdc2 kinase. Proc Natl Acad Sci USA 88: 9107–9111
Goldbeter A, Koshland DE (1981) An amplified sensitivity arising from covalent modification in biological systems. Proc Natl Acad Sci USA 78(11): 6840–6844
Gonze D, Goldbeter A (2000) A model for a network of phosphorylation-dephosphorylation cycles displaying the dynamics of dominoes and clocks. J Theor Biol 210: 167–186
Heinrich R, Neel BG, Rapoport TA (2002) Mathematical models of protein kinase signal transduction. Mol Cell 9(5): 957–970
Huang CY, Ferrell JE (1996) Ultrasensitivity in the mitogen-activated protein kinase cascade. Proc Natl Acad Sci USA 93(19): 10078–10083
Kholodenko B (2006) Cell-signalling dynamics in time and space. Nat Rev Mol Cell Biol 7(3): 165–176
Kim J, Copley S (2007) Why metabolic enzymes are essential or nonessential for growth of Escherichia coli K12 on Glucose. Biochemistry 46(44): 12501–12511
Markevich N, Moehren G, Demin O, Kiyatkin A, Hoek J, Kholodenko B (2004) Signal processing at the Ras circuit: what shapes Ras activation patterns?. IEE Proc Syst Biol 1(1): 104–113
Okamoto M, Takeda Y, Aso Y, Hayashi K (1983) Steady-state approximation of enzyme activation and inhibition. Biotechnol Bioeng 25(6): 1453–1463
Papadia S, Stevenson P, Hardingham NR, Bading H, Hardingham GE (2005) Nuclear Ca2+ and the cAMP response element-binding protein family mediate a late phase of activity-dependent neuroprotection. J Neurosci 25(17): 4279–4287
Qu Z, Vondriska TM (2009) The effects of cascade length, kinetics and feedback loops on biological signal transduction dynamics in a simplified cascade model. Phys Biol 6(1):016007(10pp)
Salazar C, Höfer T (2006) Kinetic models of phosphorylation cycles: a systematic approach using the rapid-equilibrium approximation for protein-protein interactions. Biosystems 83(2–3):195–206. In: 5th international conference on systems biology-ICSB 2004
Segel IH (1975) Enzyme kinetics. Behavior and analysis of rapid-equilibrium and steady-state enzyme systems. Wiley-Interscience
Stalmans W, Wulf H, Hue L, Hers HG (1974) The sequential inactivation of glycogen phosphorylase and activation of glycogen synthetase in liver after the administration of glucose to mice and rats. Euro J Biochem 41(1): 127–134
Strauss A, Yorke JA (1967) On asymptotically autonomous differential equations. Math Syst Theory 1: 175–182
Su M, Young A, He C, West C, Swanson S, Mentzer S (2001) Biphasic response of the regional lymphatics in the normal lymphocyte transfer reaction 1. Transplantation 72(3): 516–522
Tsai T, Choi Y, Ma W, Pomerening J, Tang C, Ferrell J Jr (2008) Robust, tunable biological oscillations from interlinked positive and negative feedback loops. Science’s STKE 321(5885): 126
Ventura AC, Sepulchre JA, Merajver SD (2008) A hidden feedback in signaling cascades is revealed. PLoS Comput Biol 4(3): e1000041
Wolkenhauer O, Sreenath S, Wellstead P, Ullah M, Cho K (2005) A systems-and signal-oriented approach to intracellular dynamics. Biochem Soc Trans 33: 507–515
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Li, Y., Srividhya, J. Goldbeter–Koshland model for open signaling cascades: a mathematical study. J. Math. Biol. 61, 781–803 (2010). https://doi.org/10.1007/s00285-009-0322-3
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00285-009-0322-3