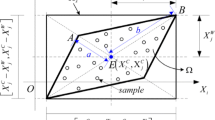
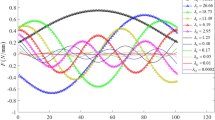
Abstract
This paper presents a single-loop algorithm for system reliability-based topology optimization (SRBTO) that can account for statistical dependence between multiple limit-states, and its applications to computationally demanding topology optimization (TO) problems. A single-loop reliability-based design optimization (RBDO) algorithm replaces the inner-loop iterations to evaluate probabilistic constraints by a non-iterative approximation. The proposed single-loop SRBTO algorithm accounts for the statistical dependence between the limit-states by using the matrix-based system reliability (MSR) method to compute the system failure probability and its parameter sensitivities. The SRBTO/MSR approach is applicable to general system events including series, parallel, cut-set and link-set systems and provides the gradients of the system failure probability to facilitate gradient-based optimization. In most RBTO applications, probabilistic constraints are evaluated by use of the first-order reliability method for efficiency. In order to improve the accuracy of the reliability calculations for RBDO or RBTO problems with high nonlinearity, we introduce a new single-loop RBDO scheme utilizing the second-order reliability method and implement it to the proposed SRBTO algorithm. Moreover, in order to overcome challenges in applying the proposed algorithm to computationally demanding topology optimization problems, we utilize the multiresolution topology optimization (MTOP) method, which achieves computational efficiency in topology optimization by assigning different levels of resolutions to three meshes representing finite element analysis, design variables and material density distribution respectively. The paper provides numerical examples of two- and three-dimensional topology optimization problems to demonstrate the proposed SRBTO algorithm and its applications. The optimal topologies from deterministic, component and system RBTOs are compared with one another to investigate the impact of optimization schemes on final topologies. Monte Carlo simulations are also performed to verify the accuracy of the failure probabilities computed by the proposed approach.
















Similar content being viewed by others
Explore related subjects
Discover the latest articles and news from researchers in related subjects, suggested using machine learning.References
Allen M, Raulli M, Maute K, Frangopol DM (2004) Reliability-based analysis and design optimization of electrostatically actuated MEMS. Comput Struct 82(13–14):1007–1020
Almeida SRM, Paulino GH, Silva ECN (2010) Material gradation and layout in topology optimization of functionally graded structures: a global–local approach. Struct Multidiscipl Optim 42(6):855–868
Amir O, Bendsoe MP, Sigmund O (2009) Approximate reanalysis in topology optimization. Int J Numer Methods Eng 78(12):1474–1491
Amir O, Stolpe M, Sigmund O (2010) Efficient use of iterative solvers in nested topology optimization. Struct Multidiscipl Optim 42(1):55–72
Bae K, Wang S, Choi KK (2002) Reliability-based topology optimization. In: AIAA 2002-5542, 9th AIAA/ISSMO symposium on multidisciplinary analysis and optimization, 4–6 September 2002, Atlanta, USA
Bendsøe MP (1989) Optimal shape design as a material distribution problem. Struct Multidiscipl Optim 1(4):193–202
Bendsøe MP, Sigmund O (2003) Topology optimization: theory, methods, and applications. Springer, Berlin
Bjerager P, Krenk S (1989) Parametric sensitivity in first order reliability theory. J Eng Mech 115(7):1577–1582
Borrvall T, Petersson J (2001) Large-scale topology optimization in 3D using parallel computing. Comput Methods Appl Mech Eng 190(46–47):6201–6229
Breitung K (1984) Asymptotic approximations for multinormal integrals. J Eng Mech 110:357–366
Chen S, Chen W, Lee S (2010) Level set based robust shape and topology optimization under random field uncertainties. Struct Multidiscipl Optim 41(4):507–524
Der Kiureghian A (2005) First-and second-order reliability methods. Engineering design reliability handbook, Ch 14. CRC, Boca Raton, Florida
Du XP, Chen W (2004) Sequential optimization and reliability assessment method for efficient probabilistic design. J Mech Des 126(2):225–233
Dunnett CW, Sobel M (1955) Approximations to the probability integral and certain percentage points of a multivariate analogue of student’s t-distribution. Biometrika 42(1–2):258–260
Enevoldsen I, Sørensen JD (1994) Reliability-based optimization in structural engineering. Struct Saf 15(3):169–196
Eschenauer HA, Olhoff N (2001) Topology optimization of continuum structures: a review. Appl Mech Rev 54(4):331–389
Evgrafov A, Rupp CJ, Maute K, Dunn ML (2008) Large-scale parallel topology optimization using a dual-primal substructuring solver. Struct Multidiscipl Optim 36(4):329–345
Guest JK, Igusa T (2008) Structural optimization under uncertain loads and nodal locations. Comput Methods Appl Mech Eng 198(1):116–124
Guest JK, Prévost JH, Belytschko T (2004) Achieving minimum length scale in topology optimization using nodal design variables and projection functions. Int J Numer Methods Eng 61(2):238–254
Jung HS, Cho S (2004) Reliability-based topology optimization of geometrically nonlinear structures with loading and material uncertainties. Finite Elem Anal Des 41(3):311–331
Kang J, Kim C, Wang S (2004) Reliability-based topology optimization for electromagnetic systems. Compel Int J Comput Math Elect Electr Eng 23(3):715–723
Kang W-H, Song J, Gardoni P (2008) Matrix-based system reliability method and applications to bridge networks. Reliab Eng Syst Saf 93:1584–1593
Kang W-H, Lee Y-J, Song J, Gencturk B (2011) Further development of matrix-based system reliability method and applications to structural systems. Struct Infrastruct Eng, in print. doi:10.1080/15732479.2010.539060
Kharmanda G, Olhoff N, Mohamed A, Lemaire M (2004) Reliability-based topology optimization. Struct Multidiscipl Optim 26(5):295–307
Kim C, Wang S, Rae K, Moon H, Choi KK (2006) Reliability-based topology optimization with uncertainties. J Mech Sci Technol 20(4):494–504
Lee I, Choi KK, Gorsich D (2010) System reliability-based design optimization using the MPP-based dimension reduction method. Struct Multidiscipl Optim 41(6):823–839
Lee Y-J, Song J, Gardoni P, Lim H-W (2011) Post-hazard flow capacity of bridge transportation network considering structural deterioration of bridges. Struct Infrastruct Eng 7(7):509–521
Liang J, Mourelatos ZP, Nikolaidis E (2007) A single-loop approach for system reliability-based design optimization. J Mech Des 129(12):1215–1224
Liang J, Mourelatos ZP, Tu J (2008) A single-loop method for reliability-based design optimisation. Int J Prod Dev 5(1–2):76–92
Lógó J, Ghaemi M, Rad MM (2009) Optimal topologies in case of probabilistic loading: the influence of load correlation. Mech Based Des Struct Mach 37(3):327–348
Luo Y, Kang Z, Luo Z, Li A (2009) Continuum topology optimization with non-probabilistic reliability constraints based on multi-ellipsoid convex model. Struct Multidiscipl Optim 39(3):297–310
Maute K, Frangopol DM (2003) Reliability-based design of MEMS mechanisms by topology optimization. Comput Struct 81(8–11):813–824
McDonald M, Mahadevan S (2008) Design optimization with system-level reliability constraints. J Mech Des 130(2):021403-1-10
Mogami K, Nishiwaki S, Izui K, Yoshimura M, Kogiso N (2006) Reliability-based structural optimization of frame structures for multiple failure criteria using topology optimization techniques. Struct Multidiscipl Optim 32(4):299–311
Nguyen TH (2010) System reliability-based design and multiresolution topology optimization. PhD thesis, Department of Civil and Environmental Engineering, University of Illinois at Urbana-Champaign
Nguyen TH, Paulino GH, Song J, Le CH (2010a) A computational paradigm for multiresolution topology optimization (MTOP). Struct Multidiscipl Optim 41(4):525–539
Nguyen TH, Song J, Paulino GH (2010b) Single-loop system reliability-based design optimization using matrix-based system reliability method: theory and applications. J Mech Des 132(1):011005-1-11
Rahman S, Wei D (2008) Design sensitivity and reliability-based structural optimization by univariate decomposition. Struct Multidiscipl Optim 35(3):245–261
Royset JO, Der Kiureghian A, Polak E (2001) Reliability-based optimal structural design by the decoupling approach. Reliab Eng Syst Saf 73(3):213–221
Royset JO, Der Kiureghian A, Polak E (2006) Optimal design with probabilistic objective and constraints. J Eng Mech 132(1):107–118
Rozvany GIN (2001) Aims, scope, methods, history and unified terminology of computer-aided topology optimization in structural mechanics. Struct Multidiscipl Optim 21(2):90–108
Rozvany GIN (2008) Exact analytical solutions for benchmark problems in probabilistic topology optimization. In: EngOpt 2008–international conference on engineering optimization, Rio de Janeiro
Rozvany GIN, Zhou M, Birker T (1992) Generalized shape optimization without homogenization. Struct Multidiscipl Optim 4(3):250—252
Shan S, Wang GG (2008) Reliable design space and complete single-loop reliability-based design optimization. Reliab Eng Syst Saf 93(8):1218–1230
Silva M, Tortorelli DA, Norato JA, Ha C, Bae HR (2010) Component and system reliability-based topology optimization using a single-loop method. Struct Multidiscipl Optim 41(1):87–106
Song J, Kang W-H (2009) System reliability and sensitivity under statistical dependence by matrix-based system reliability method. Struct Saf 31(2):148–156
Song J, Ok S-Y (2010) Multi-scale system reliability analysis of lifeline networks under earthquake hazards. Earthquake Eng Struct Dyn 39(3):259–279
Sutradhar A, Paulino GH, Miller MJ, Nguyen TH (2010) Topological optimization for designing patient-specific large craniofacial segmental bone replacements. Proc Nat Acad Sci USA 107(30):13222–13227
Svanberg K (1987) The method of moving asymptotes—a new method for structural optimization. Int J Numer Methods Eng 24(2):359–373
Tu J, Choi KK, Park YH (1999) A new study on reliability-based design optimization. J Mech Des 121(4):557–564
Wang S, de Sturler E, Paulino GH (2007) Large-scale topology optimization using preconditioned Krylov subspace methods with recycling. Int J Numer Methods Eng 69(12):2441–2468
Wu YT, Wang W (1998) Efficient probabilistic design by converting reliability constraints to approximately equivalent deterministic constraints. J Integr Des Process Sci 2(4):13–21
Xu H, Rahman S (2005) Decomposition methods for structural reliability analysis. Probab Eng Mech 20(3):239–250
Yang RJ, Chuang C, Gu L, Li G (2005) Experience with approximate reliability-based optimization methods II: an exhaust system problem. Struct Multidiscipl Optim 29(6):488–497
Acknowledgments
This research was funded in part by a grant from the Vietnam Education Foundation (VEF) and the National Science Foundation. The supports are gratefully acknowledged. The opinions, findings, and conclusions stated herein are those of the authors and do not necessarily reflect those of sponsors.
Nomenclature
- B :
-
strain-displacement matrix of shape function derivatives
- c :
-
“event” vector
- d :
-
vector of design variables
- D(·):
-
constitutive matrix determined by material density function
- d n :
-
the n-th design variable
- D 0 :
-
constitutive matrix corresponding to the solid material
- E i :
-
the i-th failure event
- E sys :
-
the system failure event
- E 0 :
-
Young’s modulus corresponding to the solid material
- f(·):
-
objective function
- f :
-
global load vector
- \(g_{i}({\uprho}, {\rm \textbf{X}}\)):
-
limit-state (or performance) function of the i-th failure mode
- \(g_{P_i^t}\) :
-
performance function of the i-th failure mode
- Jx,u :
-
Jacobian matrix of the transformation x = x(u)
- K :
-
global stiffness matrix
- K e :
-
element stiffness matrix
- p :
-
penalization parameter
- p :
-
“probability” vector
- P i :
-
actual failure probability of the i-th mode
- \(P_i^t\) :
-
target failure probability of the i-th mode
- P sys :
-
actual system failure probability
- \(P_{sys}^t\) :
-
target system failure probability
- r ik :
-
Dunnett-Sobel correlation parameter
- r min :
-
minimum length scale
- r ni :
-
distance from the point associated design variable to the centroid
- S :
-
common source random variables
- u d :
-
displacement vector
- \({\boldsymbol{\tilde u}}_i^t\) :
-
approximate location for the performance function value for the i-th failure mode
- \({\boldsymbol{\tilde u}}_i\) :
-
approximate location for the performance function value by the KKT condition
- volfrac :
-
volume fraction
- V s :
-
prescribed volume constraint
- X :
-
random variables
- \(\hat{\pmb{\upalpha}}_i\) :
-
negative normalized gradient vector
- β i :
-
reliability index
- \({\upbeta}_i^t\) :
-
target reliability index
- \({\upbeta} _i^{t(k)}\) :
-
updated target reliability index at the k-th design iteration
- \({\upbeta}_i^{t(k)(SORM)}\) :
-
improved reliability index using Breitung’s formula at the k-th design iteration
- \(\pmb{\rm \mu}_{\bf x}\) :
-
vector of means of x
- \({\uprho} (\cdot )\) :
-
material density function
- ρ i :
-
density of element i
- \(\pmb{\uppsi}\) :
-
position vector
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Nguyen, T.H., Song, J. & Paulino, G.H. Single-loop system reliability-based topology optimization considering statistical dependence between limit-states. Struct Multidisc Optim 44, 593–611 (2011). https://doi.org/10.1007/s00158-011-0669-0
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00158-011-0669-0