Delay-Dependent Stability for Discrete 2D Switched Systems with State Delays in the Roesser Model

Abstract

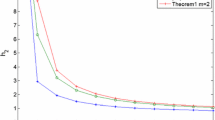
This paper is concerned with the problem of delay-dependent stability analysis for a class of two-dimensional (2D) discrete switched systems described by the Roesser model with state delays. First, the concept of average dwell time is extended to 2D switched systems with state delays. Then, based on the average dwell time approach, a delay-dependent sufficient condition for the exponential stability of the addressed systems is derived. All the results are formulated in terms of linear matrix inequalities (LMIs), which can be solved efficiently. A numerical example is given to illustrate the effectiveness of the proposed method.

Appendix: The proof of Theorem 1

Proof

Without loss of generality, we assume that the kth subsystem is active. We consider the following Lyapunov–Krasovskii functional candidate for the kth subsystem:

$$ V_{k} \bigl( x ( i,j ) \bigr) = V_{k}^{h} \bigl( x^{h} ( i,j ) \bigr) + V_{k}^{v} \bigl( x^{v} ( i,j ) \bigr), $$

(14)

where

$$V_{k}^{h} \bigl( x^{h} ( i,j ) \bigr) = \sum _{g = 1}^{5} V_{gk}^{h} \bigl( x^{h} ( i,j ) \bigr),\qquad V_{k}^{v} \bigl( x^{v} ( i,j ) \bigr) = \sum_{g = 1}^{5} V_{gk}^{v} \bigl( x^{v} ( i,j ) \bigr), $$

$$V_{1k}^{h} \bigl( x^{h} ( i,j ) \bigr) = x^{h} ( i,j )^{T}P_{h}^{k}x^{h} ( i,j ),$$

$$V_{2k}^{h} \bigl( x^{h} ( i,j ) \bigr) = \sum_{r = i - d_{h} ( i )}^{i} x^{h} ( r,j )^{T}Q_{h}^{k}x^{h} ( r,j ) \alpha ^{i - r}, $$

$$V_{3k}^{h} \bigl( x^{h} ( i,j ) \bigr) = \sum _{r = i - d_{hH}}^{i - 1} x^{h} ( r,j )^{T}W_{h}^{k}x^{h} ( r,j ) \alpha ^{i - r}, $$

$$V_{4k}^{h} \bigl( x^{h} ( i,j ) \bigr) = \sum _{s = - d_{hH} + 1}^{ - d_{hL}} \sum _{r = i + s}^{i - 1} x^{h} ( r,j )^{T}Q_{h}^{k}x^{h} ( r,j ) \alpha ^{i - r}, $$

$$V_{5k}^{h} \bigl( x^{h} ( i,j ) \bigr) = d_{hH}\sum_{s = - d_{hH}}^{ - 1} \sum _{r = i + s}^{i - 1} \eta ^{h} ( r,j )^{T}R_{h}^{k}\eta ^{h} ( r,j ) \alpha ^{i - r}, $$

$$V_{1k}^{v} \bigl( x^{v} ( i,j ) \bigr) = x^{v} ( i,j )^{T}P_{v}^{k}x^{v} ( i,j ),$$

$$V_{2k}^{v} \bigl( x^{v} ( i,j ) \bigr) = \sum_{t = j - d_{v} ( j )}^{j} x^{v} ( i,s )^{T}Q_{v}^{k}x^{v} ( i,s ) \alpha ^{j - t}, $$

$$V_{3k}^{v} \bigl( x^{v} ( i,j ) \bigr) = \sum _{t = j - d_{vH}}^{j - 1} x^{v} ( i,t )^{T}W_{v}^{k}x^{v} ( i,t ) \alpha ^{j - t}, $$

$$V_{4k}^{v} \bigl( x^{v} ( i,j ) \bigr) = \sum _{s = - d_{vH} + 1}^{ - d_{vL}} \sum _{t = j + s}^{j - 1} x^{v} ( i,t )^{T}Q_{v}^{k}x^{v} ( i,t ) \alpha ^{j - t}, $$

$$V_{5k}^{v} \bigl( x^{v} ( i,j ) \bigr) = d_{vH}\sum_{s = - d_{vH}}^{ - 1} \sum _{t = j + s}^{j - 1} \eta ^{v} ( i,t )^{T}R_{v}^{k}\eta ^{v} ( i,t ) \alpha ^{j - t}, $$

$$\eta ^{h} ( r,j ) = \left [ \begin{array}{c@{\quad }c} x^{h} ( r,j )^{T} & \delta ^{h} ( r,j )^{T} \end{array} \right ]^{T}, \qquad \eta ^{v} ( i,t ) = \left [ \begin{array}{c@{\quad }c} x^{v} ( i,t )^{T} & \delta ^{v} ( i,t )^{T} \end{array} \right ]^{T}, $$

$$\delta ^{h} ( r,j ) = x^{h} ( r + 1,j ) - x^{h} ( r,j ),\qquad \delta ^{v} ( i,t ) = x^{v} ( i,t + 1 ) - x^{v} ( i,t ), $$

where

are real matrices to be determined.

Then we have

(15)

(16)

(17)

(18)

(19)

(20)

(21)

(22)

(23)

(24)

By Lemma 2, it can be obtained from (19) and (24) that

(25)

(26)

Denote

$$\varLambda _{1} = \operatorname{diag} \bigl\{ ( d_{hH} - d_{hL} )I_{h}, ( d_{vH} - d_{vL} )I_{v} \bigr\}, $$

$$\varLambda _{2} = \operatorname{diag} \bigl\{ \alpha ^{1 + d_{hH}}I_{h},\alpha ^{1 + d_{vH}}I_{v} \bigr\}, \qquad \varLambda _{3} = \operatorname{diag} \bigl\{ \alpha d_{hH}^{2}I_{h},\alpha d_{vH}^{2}I_{v} \bigr\}. $$

From (25) and (26), we obtain the following relationship:

(27)

where

In addition, applying Lemma 1, inequality (8) is equivalent to the following inequality:

$$\left ( \begin{array}{c@{\quad }c@{\quad }c@{\quad }c} \varPsi _{11} & \varPsi _{12} & \varLambda _{2}R_{3}^{k} & - \varLambda _{2}R_{2}^{k} \\ * & \varPsi _{22} & 0 & 0 \\ * & * & \varPsi _{33} & \varLambda _{2}R_{2}^{k} \\ \noalign{\vspace*{4pt}} * & * & * & - \varLambda _{2}R_{1}^{k} \end{array} \right ) < 0. $$

Thus, it is easy to obtain

$$ V_{k}^{h} ( i + 1,j ) + V_{k}^{v} ( i,j + 1 ) < \alpha \bigl( V_{k}^{h} ( i,j ) + V_{k}^{v} ( i,j ) \bigr). $$

(28)

Notice that for any nonnegative integer D>z=max(z ₁,z ₂), one has that V ^h(0,D)=V ^v(D,0)=0. Then summing up both sides of (28) from D−1 to 0 with respect to j and 0 to D−1 with respect to i, one gets

(29)

Assume that the switching number of σ(i,j) on an interval [z,D) is υ=N _σ(i,j)(z,D), and let (i _κ−υ+1,j _κ−υ+1),(i _κ−υ+2,j _κ−υ+2),…,(i _κ ,j _κ ) denote the switching points of σ(i,j) over the interval [z,D). Thus, denoting m _i =i _i +j _i , i=κ−υ+1,…,κ, it follows from (10) and (29) that

(30)

Notice from (14) that there exist two positive constants a and b(a<b) such that

(31)

Combining (30) and (31), we obtain

$$ \sum_{i + j = D} \bigl \Vert x ( i,j ) \bigr \Vert ^{2} < \frac{b}{a}e^{ - ( - \frac{\ln \chi}{\tau _{a}} - \ln \alpha ) ( D - z )}\sum _{i + j = z} \bigl \Vert x ( i,j ) \bigr \Vert _{C}^{2}. $$

(32)

By Definition 1, it follows from (9) that 2D discrete switched system (1) is exponentially stable. The proof is completed. □